9744:
11493:
9367:
11044:
28:
12707:
9739:{\displaystyle {\begin{aligned}{\begin{pmatrix}1\\0\end{pmatrix}}\cdot {\begin{pmatrix}0\\-e\end{pmatrix}}=0&&{\begin{pmatrix}\tau _{1}\\\tau _{2}\end{pmatrix}}\cdot {\begin{pmatrix}0\\-e\end{pmatrix}}=-e\tau _{2}\\{\begin{pmatrix}1\\0\end{pmatrix}}\cdot {\begin{pmatrix}e\tau _{2}\\-e\tau _{1}\end{pmatrix}}=e\tau _{2}&&{\begin{pmatrix}\tau _{1}\\\tau _{2}\end{pmatrix}}\cdot {\begin{pmatrix}e\tau _{2}\\-e\tau _{1}\end{pmatrix}}=0\end{aligned}}}
11488:{\displaystyle {\begin{aligned}\chi _{1}\chi _{2}(\lambda +\mu )&=\chi _{1}(\lambda +\mu )\chi _{2}(\lambda +\mu )\\&=\chi _{1}(\lambda )\chi _{1}(\mu )\chi _{2}(\lambda )\chi _{2}(\mu )\exp(i\pi {\text{Im}}H_{1}(\lambda ,\mu ))\exp(i\pi {\text{Im}}H_{2}(\lambda ,\mu ))\\&=\chi _{1}\chi _{2}(\lambda )\chi _{1}\chi _{2}(\mu )\exp(i\pi {\text{Im}}H_{1}(\lambda ,\mu )+i\pi {\text{Im}}H_{2}(\lambda ,\mu ))\end{aligned}}}
12489:
7668:
9336:
7205:
14153:
7466:
1416:
9151:
12702:{\displaystyle {\begin{matrix}1&\to &{\text{Hom}}(\Lambda ,U(1))&\to &{\mathcal {P}}(\Lambda )&\to &NS(X)&\to 0\\&&\downarrow &&\downarrow &&\downarrow \\1&\to &{\text{Pic}}^{0}(X)&\to &{\text{Pic}}(X)&\to &{\text{NS}}(X)&\to 0\end{matrix}}}
2069:
771:
5587:
6906:
14291:
13931:
1238:
1898:
6790:
3705:
636:
13070:
2912:
The class of homomorphic maps between complex tori have a very simple structure. Of course, every homomorphism induces a holomorphic map, but every holomorphic map is the composition of a special kind of holomorphic map with a homomorphism. For an element
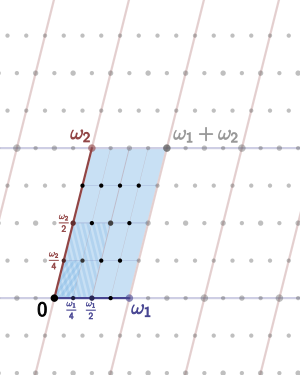
5379:
7663:{\displaystyle {\mathcal {L}}(U)=\left\{\theta :\pi ^{-1}(U)\to \mathbb {C} :{\begin{matrix}\theta {\text{ holomorphic with }}\theta (v+\lambda )=f(\lambda ,v)\theta (v)\\{\text{for all }}(\lambda ,v)\in \Lambda \times \pi ^{-1}(U)\end{matrix}}\right\}}
5816:
2202:
5374:
11980:
2309:
2826:
10281:
9331:{\displaystyle {\begin{aligned}E\cdot {\begin{pmatrix}1\\0\end{pmatrix}}={\begin{pmatrix}0\\-e\end{pmatrix}}&&E\cdot {\begin{pmatrix}\tau _{1}\\\tau _{2}\end{pmatrix}}={\begin{pmatrix}e\tau _{2}\\-e\tau _{1}\end{pmatrix}}\end{aligned}}}
6593:
14158:
12938:
4258:
1501:
2734:
11666:
7798:
which are exactly the theta functions on the plane. Conversely, this process can be done backwards where the automorphic factor in the theta function is in fact the factor of automorphy defining a line bundle on a complex torus.
6070:
11002:
10800:
1893:
10082:
10600:
4756:
8665:
6165:
252:. These are Lie groups where the structure maps are holomorphic maps of complex manifolds. It turns out that all such compact connected Lie groups are commutative, and are isomorphic to a quotient of their Lie algebra
5675:
4632:
4092:
1150:
13152:
9996:
8570:
7200:{\displaystyle E_{L}={\begin{pmatrix}0&e_{2,1}&e_{3,1}&e_{4,1}\\-e_{2,1}&0&e_{3,2}&e_{4,2}\\-e_{3,1}&-e_{2,3}&0&e_{4,3}\\-e_{4,1}&-e_{4,2}&-e_{4,3}&0\end{pmatrix}}}
13363:
12270:
4366:
12805:
10676:
7372:
11866:
9120:
4954:
12066:
13304:
13154:
which has the special property that that dual of the dual complex torus is the original complex torus. Moreover, from the discussion above, we can identify the dual complex torus with the Picard group of
12123:
5966:
5160:
14148:{\displaystyle {\begin{matrix}H:(V\times {\overline {\Omega }})\times (V\times {\overline {\Omega }})\to \mathbb {C} \\H((v_{1},l_{1}),(v_{2},l_{2}))={\overline {l_{2}(v_{1})}}+l_{1}(v_{2})\end{matrix}}}
8901:
3343:
12990:
12484:
7796:
8748:
8016:
6664:
3487:
8821:
3590:
13898:
12995:
9787:
926:
834:
14678:
2642:
7280:
13779:
5680:
5073:
2082:
9058:
1411:{\displaystyle \Pi ={\begin{pmatrix}\lambda _{1,1}&\lambda _{1,2}&\lambda _{1,3}&\lambda _{1,4}\\\lambda _{2,1}&\lambda _{2,2}&\lambda _{2,3}&\lambda _{2,4}\end{pmatrix}}}
13225:
11049:
9372:
9156:
5238:
2865:
1903:
9836:
4854:
3987:
545:
11871:
4452:
2207:
13365:
which factors through the dual complex torus. There are other constructions of the dual complex torus using techniques from the theory of
Abelian varieties. Essentially, taking a line bundle
6854:
985:
358:
320:
6283:
2473:
1047:
7437:
10183:
11571:
10539:
8216:
7970:
3211:
1699:
10391:
7728:
6625:
6460:
3873:
1799:
1739:
10869:
8280:
10178:
8364:
5233:
290:
12845:
10716:
8849:
6213:
4814:
3531:
12877:
12171:
4162:
3767:
1421:
13644:
13525:
10453:
2064:{\displaystyle {\begin{aligned}\det P&=\det(1_{g})\det(Z-1_{g}1_{g}{\overline {Z}})\\&=\det(Z-{\overline {Z}})\\&\Rightarrow \det({\text{Im}}(Z))\neq 0\end{aligned}}}
611:
407:
13577:
11039:
4500:
3582:
3374:
1074:
11576:
10110:
4535:
3414:
3165:
6819:
3029:
2990:
1203:
9898:
8079:
6455:
2902:
13926:
13820:
13676:
8780:
7461:
3117:
2578:
2541:
2340:
12344:
11780:
6002:
2370:
12872:
8963:
8447:
7930:
7908:
7833:
6341:
4157:
3250:
2424:
1543:
1225:
868:
766:{\displaystyle \Pi ={\begin{pmatrix}\lambda _{1,1}&\cdots &\lambda _{1,2g}\\\vdots &&\vdots \\\lambda _{g,1}&\cdots &\lambda _{g,2g}\end{pmatrix}}}
10874:
8941:
5852:
4990:
4294:
4128:
3913:
3279:
1824:
13606:
12305:
11701:
7694:
5582:{\displaystyle H^{1}(\pi _{1}(X),H^{0}({\mathcal {O}}_{\tilde {X}}^{*}))\to \ker(H^{1}(X,{\mathcal {O}}_{X}^{*})\to H^{1}({\tilde {X}},{\mathcal {O}}_{\tilde {X}}^{*}))}
2944:
476:
435:
137:
14343:
11741:
10832:
3943:
3080:
2504:
1622:
13090:
12143:
11800:
10415:
8399:
8150:
8111:
6901:
6388:
6319:
6233:
5872:
5192:
2828:
which are called the analytic and rational representations of the space of homomorphisms. These are useful to determining some information about the endomorphism ring
2647:
565:
10001:
9362:
9146:
13478:
12394:
8176:
7886:
6651:
3828:
2739:
11525:
10357:
10301:
9945:
8482:
7860:
6881:
6368:
4693:
1649:
13432:
1583:
1174:
1094:
496:
8575:
6075:
3175:
One distinct class of homomorphisms of complex tori are called isogenies. These are endomorphisms of complex tori with a non-zero kernel. For example, if we let
14311:
13840:
13716:
13696:
13452:
13403:
13383:
13245:
13173:
12434:
12414:
12368:
10493:
10473:
10325:
10137:
9918:
8705:
8685:
8522:
8502:
7233:
6408:
5986:
5892:
5093:
4902:
4882:
4688:
4656:
3799:
3551:
3049:
2598:
1819:
1759:
1563:
631:
381:
250:
4000:
1099:
13098:
9950:
8527:
3585:
13309:
12176:
10721:
12734:
11805:
14286:{\displaystyle {\begin{matrix}\chi :\Lambda \times {\hat {\Lambda }}\to U(1)\\\chi (\lambda ,l_{0})=\exp(i\pi {\text{Im}}l_{0}(\lambda ))\end{matrix}}}
9063:
8903:
by looking at a generic alternating matrix and finding the correct compatibility conditions for it to behave as expected. If we use the standard basis
10544:
11985:
211:
13250:
12071:
5897:
5098:
3284:
14478:
12439:
7733:
2543:
such that the group structure is preserved. This has a number of consequences, such as every homomorphism induces a map of their covering spaces
5600:
3419:
4540:
873:
776:
2380:
To get a period matrix which gives a projective complex manifold, hence an algebraic variety, the period matrix needs to further satisfy the
4995:
4299:
13177:
10605:
7285:
9792:
4819:
2603:
437:
has a complex Lie group structure, and is also compact and connected. This implies the two definitions for complex tori are equivalent.
4371:
12486:
Moreover, this is a group homomorphism with a trivial kernel. These facts can all be summarized in the following commutative diagram
6171:
map, sending an isomorphism class of a line bundle to its associated first Chern class. It turns out there is an isomorphism between
4907:
14313:. Showing this data constructs a line bundle with the desired properties follows from looking at the associated canonical factor of
8854:
12947:
5818:
representing line bundles on complex tori as 1-cocyles in the associated group cohomology. It is typical to write down the group
12847:. If we want to put a complex structure on the real torus of all characters, we need to start with a complex vector space which
8710:
7978:
8785:
13846:
9749:
1895:
since this must be a non-singular matrix. This is because if we calculate the determinant of the block matrix, this is simply
14637:
14516:
14447:
7238:
292:
whose covering map is the exponential map of a Lie algebra to its associated Lie group. The kernel of this map is a lattice
13724:
4769:
179:
8968:
3710:
13483:
2831:
13530:
10802:
of the set of characters with a real torus. The set of all pairs of semi-characters and their associated
Hermitian form
3948:
501:
14753:
Felder, Giovanni; Henriques, André; Rossi, Carlo A.; Zhu, Chenchang (2008). "A gerbe for the elliptic gamma function".
14407:
3122:
6824:
2949:
931:
325:
295:
6238:
990:
13904:
where the first is the property discussed above, and the second acts as a normalization property. We can construct
11004:
This group structure comes from applying the previous commutation law for semi-characters to the new semicharacter
7377:
6785:{\displaystyle \Pi ={\begin{pmatrix}z_{1,1}&z_{1,2}&1&0\\z_{2,1}&z_{2,2}&0&1\end{pmatrix}}}
3700:{\displaystyle {\mathcal {T}}_{g}\cong GL_{\mathbb {Z} }(2g)\backslash GL_{\mathbb {R} }(2g)/GL_{\mathbb {C} }(g)}
3216:
13065:{\displaystyle {\hat {\Lambda }}=\{l\in {\overline {\Omega }}:\langle l,\Lambda \rangle \subseteq \mathbb {Z} \}}
11530:
10498:
8181:
7935:
8667:
from the first Chern class. We can also identify it with the group of alternating real-valued alternating forms
3178:
1654:
114:
10362:
7699:
6598:
3833:
2546:
2509:
1764:
1704:
1588:
12992:, which is part of the factorization for writing down characters. Furthermore, there is an associated lattice
10841:
8224:
5811:{\displaystyle H^{1}(\pi _{1}(X),H^{0}({\mathcal {O}}_{\tilde {X}}^{*}))\cong H^{1}(X,{\mathcal {O}}_{X}^{*})}
2197:{\displaystyle {\begin{pmatrix}z_{1,1}&z_{1,2}&1&0\\z_{2,1}&z_{2,2}&0&1\end{pmatrix}}}
10142:
8286:
5369:{\displaystyle Z^{1}(\pi _{1}(X),H^{0}({\mathcal {O}}_{\tilde {X}}^{*}))\to H^{1}(X,{\mathcal {O}}_{X}^{*})}
5197:
255:
12810:
10681:
8826:
6174:
3492:
17:
14799:
12148:
11975:{\displaystyle a(\lambda ,v)=\chi (\lambda )\exp(\pi H(v,\lambda )+{\frac {\pi }{2}}H(\lambda ,\lambda ))}
2304:{\displaystyle {\begin{pmatrix}1+i&1-i&1&0\\1+2i&1+{\sqrt {2}}i&0&1\end{pmatrix}}}
13611:
10420:
4763:
3770:
570:
386:
11007:
4457:
3556:
3348:
1052:
14824:
14814:
14541:
10087:
7808:
5997:
4505:
3387:
2381:
14471:
6795:
2995:
1179:
14819:
10276:{\displaystyle \chi (\lambda +\mu )=\chi (\lambda )\chi (\mu )\exp(i\pi {\text{Im}}H(\lambda ,\mu ))}
9841:
8022:
6413:
2429:
13907:
13784:
13657:
9338:
Then, taking the inner products (with the standard inner product) of these vectors with the vectors
8761:
8410:
7442:
2314:
12310:
11746:
2345:
191:
168:
13654:
From the construction of the dual complex torus, it is suggested there should exist a line bundle
12850:
11668:
This surjection can be constructed through associating to every semi-character pair a line bundle
8946:
8418:
7913:
7891:
7816:
6588:{\displaystyle E_{L}(\lambda ,\mu )=g(\mu ,v+\lambda )+g(\lambda ,v)-g(\lambda ,v+\mu )-g(\mu ,v)}
6324:
4133:
2395:
1514:
1208:
839:
14374:
10304:
8906:
5821:
4959:
4263:
4097:
3882:
3255:
13582:
12275:
11671:
7673:
3167:
showing the holomorphic maps are not much larger than the set of homomorphisms of complex tori.
2916:
2870:
452:
412:
14316:
11714:
10805:
3918:
3085:
2821:{\displaystyle \rho _{r}:{\text{Hom}}(X,X')\to {\text{Hom}}_{\mathbb {Z} }(\Lambda ,\Lambda ')}
1153:
14442:. Herbert Lange (Second, augmented ed.). Berlin, Heidelberg: Springer Berlin Heidelberg.
13698:
and its dual which can be used to present all isomorphism classes of degree 0 line bundles on
13075:
12709:
where the vertical arrows are isomorphisms, or equality. This diagram is typically called the
12128:
11785:
10400:
8372:
8119:
8084:
6886:
6373:
6288:
6218:
5857:
5165:
550:
14402:. C. P. Ramanujam, I︠U︡. I. Manin. Published for the Tata Institute of Fundamental Research.
14369:
12933:{\displaystyle {\overline {\Omega }}={\text{Hom}}_{\overline {\mathbb {C} }}(V,\mathbb {C} )}
9341:
9125:
4253:{\displaystyle H^{0}({\mathcal {O}}_{\tilde {X}}^{*})=\{f:{\tilde {X}}\to \mathbb {C} ^{*}\}}
1496:{\displaystyle \Pi ={\begin{pmatrix}1&0&i&2i\\1&-i&1&1\end{pmatrix}}}
13457:
12373:
8155:
7865:
6630:
3807:
14725:
14526:
14364:
12726:
11498:
10330:
10286:
9923:
8455:
7838:
6859:
6346:
4759:
3489:
and isomorphic tori can be given by a change of basis of their lattices, hence a matrix in
1627:
13408:
11661:{\displaystyle 1\to {\text{Hom}}(\Lambda ,U(1))\to {\mathcal {P}}(\Lambda )\to NS(X)\to 1}
10495:, or equivalently, its dual torus, which can be seen by computing the group of characters
3054:
2478:
2079:
For example, we can write a normalized period matrix for a 2-dimensional complex torus as
1568:
1159:
1079:
481:
8:
14686:
12068:
can be easily verified by direct computation. Hence the cocycle determines a line bundle
14729:
14780:
14762:
14741:
14715:
14691:
14625:
14607:
14561:
14296:
13825:
13701:
13681:
13437:
13388:
13368:
13230:
13158:
12419:
12399:
12353:
10478:
10458:
10310:
10122:
9903:
8690:
8670:
8507:
8487:
7218:
6393:
5971:
5877:
5078:
4887:
4867:
4673:
4641:
3784:
3536:
3034:
2583:
1804:
1744:
1548:
616:
366:
235:
14542:"When is an abelian surface isomorphic or isogeneous to a product of elliptic curves?"
6065:{\displaystyle 0\to \mathbb {Z} \to {\mathcal {O}}_{X}\to {\mathcal {O}}_{X}^{*}\to 0}
14745:
14684:
Block, Jonathan; Daenzer, Calder (2008). "Mukai duality for gerbes with connection".
14565:
14512:
14453:
14443:
14413:
14403:
14359:
227:
172:
164:
143:
complex manifold. All complex tori, up to isomorphism, are obtained in this way. For
90:
67:
14629:
14598:
Ben-Bassat, Oren (2012). "Gerbes and the holomorphic Brauer group of complex tori".
10997:{\displaystyle (H_{1},\chi _{1})*(H_{2},\chi _{2})=(H_{1}+H_{2},\chi _{1}\chi _{2})}
10795:{\displaystyle {\text{Hom}}(\Lambda ,U(1))\cong \mathbb {R} ^{2g}/\mathbb {Z} ^{2g}}
1888:{\displaystyle {\begin{pmatrix}Z&1_{g}\\{\overline {Z}}&1_{g}\end{pmatrix}}}
14772:
14737:
14733:
14617:
14553:
7696:
open. Then, evaluated on global sections, this is the set of holomorphic functions
3876:
215:
203:
160:
52:
14784:
14776:
14522:
14354:
12722:
10394:
2729:{\displaystyle \rho _{a}:{\text{Hom}}(X,X')\to {\text{Hom}}_{\mathbb {C} }(V,V')}
101:
59:
10077:{\displaystyle H_{a}(z,w)=a\cdot {\frac {z{\overline {w}}}{{\text{Im}}(\tau )}}}
4856:
which is useful for computing invariants related to the associated line bundle.
4762:, more precisely, the automorphic functions used in the transformation laws for
12941:
12731:
As mentioned before, a character on the lattice can be expressed as a function
10595:{\displaystyle \Lambda \to \mathbb {R} \to \mathbb {R} /\mathbb {Z} \cong U(1)}
3801:, in particular complex tori, there is a construction relating the holomorphic
187:
152:
148:
109:
4751:{\displaystyle f:\mathbb {C} ^{n}\times \mathbb {Z} ^{2n}\to \mathbb {C} ^{*}}
14808:
14457:
14417:
12416:, and a semi-character can be constructed using the factor of automorphy for
2600:
induces a group homomorphism, it must restrict to a morphism of the lattices
140:
14496:"How Appell-Humbert theorem works in the simplest case of an elliptic curve"
8660:{\displaystyle c_{1}:H^{1}({\mathcal {O}}_{X}^{*})\to H^{2}(X,\mathbb {Z} )}
6160:{\displaystyle c_{1}:H^{1}({\mathcal {O}}_{X}^{*})\to H^{2}(X,\mathbb {Z} )}
5095:. Since this action is free and properly discontinuous, the quotient bundle
1503:
forms a period matrix since the associated period matrix has determinant 4.
987:, it corresponds to a period matrix if and only if the corresponding matrix
14495:
14437:
14397:
5670:{\displaystyle H^{1}({\tilde {X}},{\mathcal {O}}_{\tilde {X}}^{*})\cong 0}
14511:, Progress in Mathematics, vol. 177, Boston, MA: Birkhäuser Boston,
6168:
4627:{\displaystyle Z^{1}(\pi _{1}(X),H^{0}({\mathcal {O}}_{\tilde {X}}^{*}))}
4087:{\displaystyle H^{1}(\pi _{1}(X),H^{0}({\mathcal {O}}_{\tilde {X}}^{*}))}
3802:
3533:. This gives the set of isomorphism classes of complex tori of dimension
195:
44:
27:
1235:
For a two-dimensional complex torus, it has a period matrix of the form
1145:{\displaystyle P={\begin{pmatrix}\Pi \\{\overline {\Pi }}\end{pmatrix}}}
14557:
13147:{\displaystyle {\hat {X}}\cong {\overline {\Omega }}/{\hat {\Lambda }}}
3384:
There is an isomorphism of complex structures on the real vector space
163:
found necessary and sufficient conditions for a complex torus to be an
14621:
3773:, which shows there are far more complex tori than Abelian varieties.
14767:
12272:
Note this action can be used to show the sections of the line bundle
9991:{\displaystyle H_{a}:\mathbb {C} \times \mathbb {C} \to \mathbb {C} }
8565:{\displaystyle {\text{Im}}H(\Lambda ,\Lambda )\subseteq \mathbb {Z} }
230:
14696:
13358:{\displaystyle {\overline {\Omega }}\to {\text{Hom}}(\Lambda ,U(1))}
12265:{\displaystyle \lambda \circ (v,t)=(v+t,a_{(H,\chi )}(\lambda ,v)t)}
5991:
4361:{\displaystyle f:\pi _{1}(X)\times {\tilde {X}}\to \mathbb {C} ^{*}}
1741:. We can get this from taking a change of basis of the vector space
12800:{\displaystyle \chi (\cdot )=\exp \left(2\pi iv^{*}(\cdot )\right)}
10671:{\displaystyle \chi (\cdot )=\exp \left(2\pi iv^{*}(\cdot )\right)}
9122:
and calculate the associated products on the vectors associated to
8753:
7367:{\displaystyle \in H^{1}(\Lambda ,H^{0}(V,{\mathcal {O}}_{V}^{*}))}
2580:
which is compatible with their covering maps. Furthermore, because
214:, no complex torus other than the abelian varieties can 'fit' into
31:
The complex torus associated to a lattice spanned by two periods, ω
14720:
14612:
14570:- Gives tools to find complex tori which are not Abelian varieties
11861:{\displaystyle a_{(H,\chi )}:\Lambda \times V\to \mathbb {C} ^{*}}
9115:{\displaystyle E={\begin{pmatrix}0&e\\-e&0\end{pmatrix}}}
7802:
13718:. We can encode this behavior with the following two properties
13646:, hence is in fact the dual complex torus (or Abelian variety).
14706:
Ben-Bassat, Oren (2013). "Equivariant gerbes on complex tori".
14575:
7210:
4949:{\displaystyle {\tilde {X}}\times \mathbb {C} \to {\tilde {X}}}
100:
All such complex structures can be obtained as follows: take a
74:
12061:{\displaystyle a(\lambda +\mu ,v)=a(\lambda ,v+\mu )a(\mu ,v)}
7207:
where a number of compatibility conditions must be satisfied.
13299:{\displaystyle l\mapsto \exp(2\pi i\langle l,\cdot \rangle )}
12118:{\displaystyle L(H,\chi )\cong V\times \mathbb {C} /\Lambda }
5961:{\displaystyle H^{1}(\Lambda ,H^{0}({\mathcal {O}}_{V}^{*}))}
5155:{\displaystyle L={\tilde {X}}\times \mathbb {C} /\pi _{1}(X)}
63:
14634:- Extends idea of using alternating forms on the lattice to
8896:{\displaystyle E\in {\text{Alt}}^{2}(\Lambda ,\mathbb {Z} )}
3338:{\displaystyle X_{n}\cong (\mathbb {Z} /n\mathbb {Z} )^{2g}}
13579:
presenting it as an isogeny. It can be shown that defining
12985:{\displaystyle {\text{Hom}}_{\mathbb {R} }(V,\mathbb {R} )}
12479:{\displaystyle {\mathcal {P}}(\Lambda )\to {\text{Pic}}(X)}
12307:
are given by the theta functions with factor of automorphy
10114:
7791:{\displaystyle \theta (v+\lambda )=f(\lambda ,v)\theta (v)}
4159:
there is an induced action on all of its sheaves, hence on
1761:
giving a block matrix of the form above. The condition for
8743:{\displaystyle E(\Lambda ,\Lambda )\subseteq \mathbb {Z} }
8011:{\displaystyle E(\Lambda ,\Lambda )\subseteq \mathbb {Z} }
4859:
3915:. Fortunately for complex tori, every complex line bundle
3482:{\displaystyle GL_{\mathbb {R} }(2g)/GL_{\mathbb {C} }(g)}
8816:{\displaystyle {\begin{pmatrix}1&\tau \end{pmatrix}}}
226:
One way to define complex tori is as a compact connected
13893:{\displaystyle {\mathcal {P}}|_{\{0\}\times {\hat {X}}}}
12874:
embeds into. It turns out that the complex vector space
11706:
9782:{\displaystyle E(\Lambda ,\Lambda )\subset \mathbb {Z} }
928:
If we go in the reverse direction by selecting a matrix
921:{\displaystyle X=\mathbb {C} ^{g}/\Pi \mathbb {Z} ^{2g}}
829:{\displaystyle \lambda _{i}=\sum _{j}\lambda _{ji}e_{j}}
194:(with fixed modulus). There is nothing as simple as the
14752:
14673:{\displaystyle {\text{Alt}}^{3}(\Lambda ,\mathbb {Z} )}
2375:
14345:, and observing its behavior at various restrictions.
14163:
13936:
12494:
9682:
9639:
9571:
9542:
9490:
9447:
9409:
9380:
9280:
9237:
9199:
9170:
9078:
8794:
7534:
7275:{\displaystyle f:\Lambda \times V\to \mathbb {C} ^{*}}
6928:
6679:
3638:
2216:
2091:
1833:
1436:
1253:
1114:
1049:
constructed by adjoining the complex conjugate matrix
651:
186:> 1, and are really coextensive with the theory of
178:
The actual projective embeddings are complicated (see
14640:
14319:
14299:
14161:
13934:
13910:
13849:
13828:
13787:
13774:{\displaystyle {\mathcal {P}}|_{X\times \{\}}\cong L}
13727:
13704:
13684:
13660:
13614:
13585:
13533:
13486:
13460:
13440:
13411:
13391:
13371:
13312:
13253:
13233:
13180:
13161:
13101:
13078:
12998:
12950:
12880:
12853:
12813:
12737:
12492:
12442:
12422:
12402:
12376:
12356:
12313:
12278:
12179:
12151:
12131:
12074:
11988:
11874:
11808:
11788:
11749:
11717:
11674:
11579:
11533:
11501:
11047:
11010:
10877:
10844:
10808:
10724:
10684:
10608:
10547:
10501:
10481:
10461:
10423:
10403:
10365:
10333:
10313:
10289:
10186:
10145:
10125:
10090:
10004:
9953:
9926:
9906:
9844:
9795:
9752:
9370:
9344:
9154:
9128:
9066:
8971:
8949:
8909:
8857:
8829:
8788:
8764:
8713:
8693:
8673:
8578:
8530:
8510:
8490:
8458:
8421:
8375:
8289:
8227:
8184:
8158:
8122:
8087:
8025:
7981:
7938:
7916:
7894:
7868:
7841:
7819:
7736:
7702:
7676:
7469:
7445:
7380:
7288:
7241:
7221:
6909:
6889:
6862:
6827:
6798:
6667:
6633:
6601:
6463:
6416:
6396:
6376:
6349:
6327:
6291:
6241:
6221:
6177:
6078:
6005:
5974:
5900:
5880:
5860:
5824:
5683:
5603:
5382:
5241:
5200:
5168:
5101:
5081:
5068:{\displaystyle a\cdot (x,t)=(a\cdot x,f(a,x)\cdot t)}
4998:
4962:
4910:
4890:
4870:
4822:
4772:
4696:
4676:
4644:
4543:
4508:
4460:
4374:
4302:
4296:-action can then be represented as a holomorphic map
4266:
4165:
4136:
4100:
4094:
we recall how its elements can be represented. Since
4003:
3951:
3921:
3885:
3836:
3810:
3787:
3776:
3769:
this is important when considering the dimensions of
3713:
3593:
3559:
3539:
3495:
3422:
3390:
3351:
3287:
3258:
3219:
3181:
3125:
3088:
3057:
3037:
2998:
2952:
2919:
2873:
2834:
2742:
2650:
2606:
2586:
2549:
2512:
2481:
2432:
2398:
2348:
2317:
2210:
2085:
1901:
1827:
1807:
1767:
1747:
1707:
1657:
1630:
1591:
1571:
1551:
1517:
1424:
1241:
1211:
1182:
1162:
1102:
1082:
1055:
993:
934:
876:
842:
779:
639:
619:
573:
553:
504:
484:
455:
415:
389:
369:
328:
298:
258:
238:
117:
9053:{\displaystyle z=z_{1}+iz_{2}=z_{1}x_{1}+z_{2}y_{1}}
5968:
contains the isomorphism classes of line bundles on
13527:Then, the dual complex torus can be constructed as
13220:{\displaystyle {\hat {X}}\cong {\text{Pic}}^{0}(X)}
6215:and the module of alternating forms on the lattice
5162:is a complex manifold. Furthermore, the projection
2907:
2860:{\displaystyle {\text{End}}(X)\otimes \mathbb {Q} }
2506:then a homomorphism of complex tori is a function
440:
14672:
14576:"Abelian surfaces and products of elliptic curves"
14337:
14305:
14285:
14147:
13920:
13892:
13834:
13814:
13773:
13710:
13690:
13670:
13638:
13600:
13571:
13519:
13472:
13446:
13426:
13397:
13377:
13357:
13298:
13239:
13219:
13167:
13146:
13084:
13064:
12984:
12944:maps, is isomorphic to the real dual vector space
12932:
12866:
12839:
12799:
12701:
12478:
12428:
12408:
12388:
12362:
12338:
12299:
12264:
12165:
12137:
12117:
12060:
11974:
11860:
11794:
11774:
11735:
11695:
11660:
11565:
11519:
11487:
11033:
10996:
10863:
10826:
10794:
10710:
10670:
10594:
10533:
10487:
10467:
10447:
10409:
10385:
10351:
10319:
10295:
10275:
10172:
10131:
10104:
10076:
9990:
9939:
9912:
9892:
9831:{\displaystyle e=a{\frac {1}{{\text{Im}}(\tau )}}}
9830:
9781:
9738:
9356:
9330:
9140:
9114:
9052:
8957:
8935:
8895:
8843:
8815:
8774:
8742:
8699:
8679:
8659:
8572:Equivalently, it is the image of the homomorphism
8564:
8516:
8496:
8476:
8441:
8393:
8358:
8274:
8210:
8178:. Moreover, there is an associated Hermitian form
8170:
8144:
8105:
8073:
8010:
7964:
7924:
7902:
7880:
7854:
7827:
7790:
7722:
7688:
7662:
7455:
7431:
7366:
7274:
7227:
7199:
6895:
6875:
6848:
6813:
6784:
6645:
6619:
6587:
6449:
6402:
6382:
6362:
6335:
6313:
6277:
6227:
6207:
6159:
6064:
5980:
5960:
5886:
5866:
5846:
5810:
5669:
5581:
5368:
5227:
5186:
5154:
5087:
5067:
4984:
4948:
4896:
4876:
4849:{\displaystyle g:V\times \Lambda \to \mathbb {C} }
4848:
4808:
4750:
4682:
4650:
4626:
4529:
4494:
4446:
4360:
4288:
4252:
4151:
4122:
4086:
3982:{\displaystyle {\tilde {X}}\cong \mathbb {C} ^{n}}
3981:
3937:
3907:
3867:
3822:
3793:
3761:
3699:
3576:
3545:
3525:
3481:
3408:
3368:
3337:
3273:
3244:
3205:
3159:
3111:
3074:
3043:
3023:
2984:
2938:
2896:
2859:
2820:
2728:
2637:{\displaystyle F_{\Lambda }:\Lambda \to \Lambda '}
2636:
2592:
2572:
2535:
2498:
2467:
2418:
2364:
2334:
2303:
2196:
2063:
1887:
1813:
1793:
1753:
1733:
1693:
1643:
1616:
1577:
1557:
1537:
1495:
1410:
1219:
1197:
1168:
1144:
1088:
1068:
1041:
979:
920:
862:
828:
765:
625:
605:
559:
540:{\displaystyle \lambda _{1},\ldots ,\lambda _{2g}}
539:
490:
470:
429:
401:
375:
352:
314:
284:
244:
131:
14800:p-adic Abelian Integrals: from Theory to Practice
10417:. It will turn out this corresponds to the group
5992:First chern class of line bundles on complex tori
4447:{\displaystyle f(a\cdot b,x)=f(a,b\cdot x)f(b,x)}
3707:Note that as a real manifold, this has dimension
2204:one such example is the normalized period matrix
14806:
9900:, which holds for the matrix above. For a fixed
8754:Example of a Hermitian form on an elliptic curve
6849:{\displaystyle \Lambda \subset \mathbb {C} ^{2}}
6821:we have the column vectors defining the lattice
2387:
2028:
1993:
1938:
1919:
1906:
1768:
1708:
1205:hence must be linearly independent vectors over
980:{\displaystyle \Pi \in Mat_{\mathbb {C} }(g,2g)}
167:; those that are varieties can be embedded into
14506:
13608:this way satisfied the universal properties of
10359:, so it corresponds to the trivial line bundle
9060:), then we can write out an alternating matrix
3997:Starting from the first group cohomology group
3213:be an integer, then there is an associated map
409:of maximal rank, the quotient complex manifold
353:{\displaystyle {\mathfrak {g}}/\Lambda \cong U}
315:{\displaystyle \Lambda \subset {\mathfrak {g}}}
14507:Birkenhake, Christina; Lange, Herbert (1999),
10393:, then the associated semi-characters are the
9947:. Then, there is an associated Hermitian form
7803:Hermitian forms and the Appell-Humbert theorem
6457:then the alternating form can be expressed as
6278:{\displaystyle Alt^{2}(\Lambda ,\mathbb {Z} )}
4758:which follow the cocycle condition. These are
4368:. This map satisfies the cocycle condition if
1042:{\displaystyle P\in Mat_{\mathbb {C} }(2g,2g)}
14702:- includes examples of gerbes on complex tori
14533:
13480:where their translations are invariant, i.e.
7432:{\displaystyle \phi _{1}=\in {\text{Pic}}(X)}
14683:
13870:
13864:
13760:
13748:
13290:
13278:
13059:
13048:
13036:
13014:
10307:twisted by the Hermitian form. Note that if
7211:Sections of line bundles and theta functions
4247:
4211:
11566:{\displaystyle {\text{Hom}}(\Lambda ,U(1))}
10534:{\displaystyle {\text{Hom}}(\Lambda ,U(1))}
8211:{\displaystyle H:V\times V\to \mathbb {C} }
8116:is the extension of some first Chern class
7965:{\displaystyle E:V\times V\to \mathbb {R} }
7439:, there is an associated sheaf of sections
1506:
14705:
14597:
14580:Bollettino dell'unione Matematica Italiana
14435:
13385:over a complex torus (or Abelian variety)
3379:
3206:{\displaystyle n\in \mathbb {Z} _{\neq 0}}
3051:is a holomorphic map between complex tori
1801:follows from looking at the corresponding
1694:{\displaystyle Z\in Mat_{\mathbb {C} }(g)}
449:-dimensional complex torus is by using a
108:considered as real vector space; then the
14766:
14719:
14695:
14663:
14611:
14591:
14573:
13996:
13055:
12975:
12959:
12923:
12903:
12159:
12103:
11848:
10779:
10759:
10573:
10563:
10555:
10386:{\displaystyle \mathbb {C} \times X\to X}
10367:
10098:
9984:
9976:
9968:
9775:
8951:
8886:
8837:
8736:
8650:
8558:
8204:
8004:
7958:
7918:
7896:
7821:
7723:{\displaystyle \theta :V\to \mathbb {C} }
7716:
7526:
7262:
6836:
6801:
6620:{\displaystyle \mu ,\lambda \in \Lambda }
6329:
6268:
6198:
6150:
6013:
5124:
4927:
4842:
4738:
4720:
4705:
4348:
4237:
3969:
3868:{\displaystyle \pi ^{*}L\to {\tilde {X}}}
3682:
3650:
3620:
3505:
3464:
3432:
3393:
3318:
3305:
3190:
2853:
2792:
2700:
1794:{\displaystyle \det {\text{Im}}(Z)\neq 0}
1734:{\displaystyle \det {\text{Im}}(Z)\neq 0}
1676:
1213:
1185:
1012:
953:
905:
885:
363:Conversely, given a complex vector space
14680:, to construct gerbes on a complex torus
10864:{\displaystyle {\mathcal {P}}(\Lambda )}
10115:Semi-character pairs for Hermitian forms
8275:{\displaystyle {\text{Im}}H(v,w)=E(v,w)}
3992:
1156:. This guarantees the column vectors of
26:
14539:
14395:
10541:whose elements can be factored as maps
4860:Line bundles from factors of automorphy
4766:. Also, any such map can be written as
14:
14807:
13227:by sending an anti-linear dual vector
12370:. Note that because every line bundle
11495:It turns out this group surjects onto
10173:{\displaystyle \chi :\Lambda \to U(1)}
8359:{\displaystyle H(v,w)=E(iv,w)+iE(v,w)}
5228:{\displaystyle \pi :{\tilde {X}}\to X}
285:{\displaystyle {\mathfrak {g}}=T_{0}G}
12840:{\displaystyle v^{*}\in \Lambda ^{*}}
12716:
11707:Semi-character pairs and line bundles
10711:{\displaystyle v^{*}\in \Lambda ^{*}}
9920:, we will write the integral form as
8844:{\displaystyle \tau \in \mathbb {H} }
8404:
6792:expanded using the standard basis of
6208:{\displaystyle H^{2}(X,\mathbb {Z} )}
5597:In the case of complex tori, we have
5194:induced from the covering projection
4809:{\displaystyle f=\exp(2\pi i\cdot g)}
3526:{\displaystyle GL_{\mathbb {Z} }(2g)}
39:. Corresponding edges are identified.
14431:
14429:
14427:
14391:
14389:
12166:{\displaystyle V\times \mathbb {C} }
7972:satisfying the following conditions
6321:can be considered as an alternating
4904:as follows: the trivial line bundle
3762:{\displaystyle 4g^{2}-2g^{2}=2g^{2}}
2644:In particular, there are injections
2376:Period matrices of Abelian varieties
498:whose columns correspond to a basis
180:equations defining abelian varieties
104:Λ in a vector space V isomorphic to
14789:- could be extended to complex tori
13928:using the following hermitian form
13639:{\displaystyle {\text{Pic}}^{0}(X)}
13520:{\displaystyle T_{x}^{*}(L)\cong L}
10678:for some fixed dual lattice vector
10602:showing a character is of the form
10448:{\displaystyle {\text{Pic}}^{0}(X)}
5592:
606:{\displaystyle e_{1},\ldots ,e_{g}}
402:{\displaystyle \Lambda \subseteq V}
331:
307:
261:
24:
14656:
14600:Journal of Noncommutative Geometry
14574:Marchisio, Marina Rosanna (1998).
14181:
14172:
13981:
13956:
13913:
13852:
13730:
13663:
13649:
13572:{\displaystyle {\hat {X}}:=X/K(L)}
13334:
13315:
13135:
13119:
13079:
13045:
13025:
13002:
12883:
12855:
12828:
12553:
12545:
12515:
12453:
12445:
12132:
12112:
11834:
11789:
11628:
11620:
11594:
11542:
11034:{\displaystyle \chi _{1}\chi _{2}}
10855:
10847:
10733:
10699:
10548:
10510:
10404:
10152:
9765:
9759:
8879:
8767:
8726:
8720:
8608:
8548:
8542:
8436:
7994:
7988:
7623:
7472:
7448:
7342:
7314:
7248:
6890:
6828:
6668:
6614:
6377:
6261:
6222:
6108:
6040:
6023:
5936:
5914:
5861:
5789:
5735:
5635:
5550:
5497:
5434:
5347:
5293:
4835:
4670:For complex tori, these functions
4665:
4595:
4537:. The abelian group of 1-cocycles
4495:{\displaystyle a,b\in \pi _{1}(X)}
4182:
4055:
3777:Line bundles and automorphic forms
3597:
3577:{\displaystyle {\mathcal {T}}_{g}}
3563:
3369:{\displaystyle \Lambda /n\Lambda }
3363:
3352:
2808:
2801:
2627:
2620:
2612:
2458:
2413:
1572:
1532:
1425:
1242:
1163:
1126:
1117:
1083:
1069:{\displaystyle {\overline {\Pi }}}
1058:
935:
900:
857:
640:
554:
485:
424:
390:
341:
299:
126:
25:
14836:
14484:from the original on 31 May 2021.
14424:
14386:
12396:has an associated Hermitian form
10105:{\displaystyle a\in \mathbb {Z} }
4530:{\displaystyle x\in {\tilde {X}}}
3409:{\displaystyle \mathbb {R} ^{2g}}
3160:{\displaystyle h=t_{h(0)}\circ f}
3082:, there is a unique homomorphism
12346:. Sometimes, this is called the
11573:, giving a short exact sequence
8484:as the group of Hermitian forms
7910:-valued. Then, it turns out any
7235:given by a factor of automorphy
6814:{\displaystyle \mathbb {C} ^{2}}
3024:{\displaystyle x\mapsto x+x_{0}}
2985:{\displaystyle t_{x_{0}}:X\to X}
2908:Holomorphic maps of complex tori
1198:{\displaystyle \mathbb {C} ^{g}}
441:Period matrix of a complex torus
14708:Journal of Geometry and Physics
9893:{\displaystyle E(v,w)=E(iv,iw)}
8074:{\displaystyle E(iv,iw)=E(v,w)}
6661:For a normalized period matrix
6450:{\displaystyle f=\exp(2\pi ig)}
4884:we can define a line bundle on
2468:{\displaystyle X'=V'/\Lambda '}
14793:
14738:10.1016/j.geomphys.2012.10.012
14667:
14653:
14488:
14464:
14436:Birkenhake, Christina (2004).
14332:
14320:
14276:
14273:
14267:
14243:
14231:
14212:
14202:
14196:
14190:
14184:
14138:
14125:
14103:
14090:
14071:
14068:
14042:
14036:
14010:
14007:
13992:
13989:
13970:
13964:
13945:
13921:{\displaystyle {\mathcal {P}}}
13882:
13859:
13815:{\displaystyle \in {\hat {X}}}
13806:
13794:
13788:
13757:
13751:
13737:
13678:over the product of the torus
13671:{\displaystyle {\mathcal {P}}}
13633:
13627:
13592:
13566:
13560:
13540:
13508:
13502:
13421:
13415:
13352:
13349:
13343:
13331:
13323:
13293:
13266:
13257:
13214:
13208:
13187:
13138:
13108:
13005:
12979:
12965:
12927:
12913:
12789:
12783:
12747:
12741:
12689:
12684:
12678:
12668:
12663:
12657:
12647:
12642:
12636:
12619:
12607:
12601:
12595:
12583:
12578:
12572:
12561:
12556:
12550:
12538:
12533:
12530:
12524:
12512:
12502:
12473:
12467:
12459:
12456:
12450:
12380:
12348:canonical factor of automorphy
12331:
12319:
12294:
12282:
12259:
12253:
12241:
12236:
12224:
12204:
12198:
12186:
12090:
12078:
12055:
12043:
12037:
12019:
12010:
11992:
11969:
11966:
11954:
11935:
11923:
11914:
11905:
11899:
11890:
11878:
11843:
11826:
11814:
11767:
11755:
11730:
11718:
11690:
11678:
11652:
11649:
11643:
11634:
11631:
11625:
11615:
11612:
11609:
11603:
11591:
11583:
11560:
11557:
11551:
11539:
11514:
11508:
11478:
11475:
11463:
11436:
11424:
11400:
11391:
11385:
11362:
11356:
11323:
11320:
11308:
11284:
11275:
11272:
11260:
11236:
11227:
11221:
11208:
11202:
11189:
11183:
11170:
11164:
11141:
11129:
11116:
11104:
11084:
11072:
10991:
10942:
10936:
10910:
10904:
10878:
10858:
10852:
10821:
10809:
10751:
10748:
10742:
10730:
10660:
10654:
10618:
10612:
10589:
10583:
10559:
10551:
10528:
10525:
10519:
10507:
10442:
10436:
10377:
10346:
10340:
10270:
10267:
10255:
10238:
10229:
10223:
10217:
10211:
10202:
10190:
10167:
10161:
10155:
10068:
10062:
10027:
10015:
9980:
9887:
9869:
9860:
9848:
9822:
9816:
9768:
9756:
8890:
8876:
8851:we can find the integral form
8775:{\displaystyle {\mathcal {E}}}
8729:
8717:
8654:
8640:
8627:
8624:
8602:
8551:
8539:
8471:
8465:
8353:
8341:
8329:
8314:
8305:
8293:
8269:
8257:
8248:
8236:
8200:
8162:
8139:
8133:
8068:
8056:
8047:
8029:
7997:
7985:
7954:
7872:
7862:associated to the line bundle
7785:
7779:
7773:
7761:
7752:
7740:
7712:
7648:
7642:
7617:
7605:
7593:
7587:
7581:
7569:
7560:
7548:
7522:
7519:
7513:
7483:
7477:
7456:{\displaystyle {\mathcal {L}}}
7426:
7420:
7409:
7403:
7397:
7391:
7361:
7358:
7330:
7311:
7295:
7289:
7257:
6582:
6570:
6561:
6543:
6534:
6522:
6513:
6495:
6486:
6474:
6444:
6429:
6308:
6302:
6272:
6258:
6202:
6188:
6154:
6140:
6127:
6124:
6102:
6056:
6034:
6017:
6009:
5955:
5952:
5930:
5911:
5841:
5835:
5805:
5777:
5761:
5758:
5746:
5729:
5713:
5707:
5694:
5677:hence there is an isomorphism
5658:
5646:
5623:
5614:
5576:
5573:
5561:
5538:
5529:
5516:
5513:
5485:
5472:
5463:
5460:
5457:
5445:
5428:
5412:
5406:
5393:
5363:
5335:
5322:
5319:
5316:
5304:
5287:
5271:
5265:
5252:
5219:
5213:
5178:
5149:
5143:
5114:
5062:
5053:
5041:
5023:
5017:
5005:
4979:
4973:
4940:
4931:
4917:
4838:
4803:
4785:
4733:
4621:
4618:
4606:
4589:
4573:
4567:
4554:
4521:
4489:
4483:
4441:
4429:
4423:
4405:
4396:
4378:
4343:
4337:
4325:
4319:
4283:
4277:
4232:
4226:
4205:
4193:
4176:
4143:
4117:
4111:
4081:
4078:
4066:
4049:
4033:
4027:
4014:
3958:
3902:
3896:
3859:
3850:
3814:
3694:
3688:
3665:
3656:
3635:
3626:
3520:
3511:
3476:
3470:
3447:
3438:
3323:
3301:
3262:
3236:
3146:
3140:
3098:
3002:
2976:
2946:we define the translation map
2846:
2840:
2815:
2798:
2781:
2778:
2761:
2723:
2706:
2689:
2686:
2669:
2623:
2559:
2522:
2335:{\displaystyle {\text{Im}}(Z)}
2329:
2323:
2048:
2045:
2039:
2031:
2025:
2015:
1996:
1980:
1941:
1935:
1922:
1782:
1776:
1722:
1716:
1688:
1682:
1611:
1592:
1036:
1018:
974:
959:
13:
1:
14777:10.1215/S0012-7094-08-14111-0
14540:Ruppert, Wolfgang M. (1990).
14380:
12339:{\displaystyle a_{(H,\chi )}}
11775:{\displaystyle a_{(H,\chi )}}
11743:we can construct a 1-cocycle
10718:. This gives the isomorphism
6856:. Then, any alternating form
5376:which induces an isomorphism
4864:Given a factor of automorphy
2867:which has rational dimension
2388:Homomorphisms of complex tori
2365:{\displaystyle 2+{\sqrt {2}}}
2071:which gives the implication.
221:
66:in the usual sense (i.e. the
14472:"Riemann bilinear relations"
14107:
13984:
13959:
13318:
13122:
13028:
12907:
12886:
12867:{\displaystyle \Lambda ^{*}}
10050:
9838:We can then directly verify
8958:{\displaystyle \mathbb {C} }
8442:{\displaystyle X=V/\Lambda }
7925:{\displaystyle \mathbb {R} }
7903:{\displaystyle \mathbb {R} }
7828:{\displaystyle \mathbb {Z} }
7542: holomorphic with
6656:
6336:{\displaystyle \mathbb {Z} }
4152:{\displaystyle {\tilde {X}}}
3245:{\displaystyle n_{X}:X\to X}
3170:
2419:{\displaystyle X=V/\Lambda }
2074:
2010:
1975:
1860:
1538:{\displaystyle X=V/\Lambda }
1220:{\displaystyle \mathbb {R} }
1129:
1061:
863:{\displaystyle X=V/\Lambda }
836:We can then write the torus
7:
14348:
13405:, there is a closed subset
13072:called the dual lattice of
12807:for some fixed dual vector
8965:as a real vector space (so
8936:{\displaystyle x_{1},y_{1}}
7888:, it can be extended to be
5847:{\displaystyle \pi _{1}(X)}
5589:giving the desired result.
4985:{\displaystyle \pi _{1}(X)}
4638:. Note that such functions
4289:{\displaystyle \pi _{1}(X)}
4123:{\displaystyle \pi _{1}(X)}
3908:{\displaystyle \pi _{1}(X)}
3771:moduli of Abelian varieties
3274:{\displaystyle x\mapsto nx}
1651:is the identity matrix and
567:expanded out using a basis
206:can handle cases for small
10:
14841:
14534:Complex 2-dimensional tori
13601:{\displaystyle {\hat {X}}}
12720:
12300:{\displaystyle L(H,\chi )}
11711:For a semi-character pair
11696:{\displaystyle L(H,\chi )}
10139:a semi-character is a map
8408:
7806:
7689:{\displaystyle U\subset X}
5998:exponential exact sequence
2939:{\displaystyle x_{0}\in X}
2897:{\displaystyle m\leq 4gg'}
2382:Riemann bilinear relations
1230:
471:{\displaystyle g\times 2g}
430:{\displaystyle V/\Lambda }
147:= 1 this is the classical
132:{\displaystyle V/\Lambda }
14755:Duke Mathematical Journal
14546:Mathematische Zeitschrift
14439:Complex Abelian Varieties
14338:{\displaystyle (H,\chi )}
13454:defined as the points of
11736:{\displaystyle (H,\chi )}
10827:{\displaystyle (\chi ,H)}
7932:-valued alternating form
6410:has factor of automorphy
3938:{\displaystyle \pi ^{*}L}
3112:{\displaystyle f:X\to X'}
2573:{\displaystyle F:V\to V'}
2536:{\displaystyle f:X\to X'}
2311:since the determinant of
1617:{\displaystyle (Z,1_{g})}
192:several complex variables
81:must be the even number 2
13900:is a trivial line bundle
13092:. Then, we can form the
13085:{\displaystyle \Lambda }
12138:{\displaystyle \Lambda }
11795:{\displaystyle \Lambda }
10410:{\displaystyle \Lambda }
8394:{\displaystyle v,w\in V}
8145:{\displaystyle c_{1}(L)}
8106:{\displaystyle v,w\in V}
6896:{\displaystyle \Lambda }
6383:{\displaystyle \Lambda }
6314:{\displaystyle c_{1}(L)}
6228:{\displaystyle \Lambda }
6072:the connecting morphism
5867:{\displaystyle \Lambda }
5187:{\displaystyle p:L\to X}
2392:If we have complex tori
1507:Normalized period matrix
1418:for example, the matrix
560:{\displaystyle \Lambda }
169:complex projective space
51:is a particular kind of
14396:Mumford, David (2008).
14375:Elliptic gamma function
14155:and the semi-character
13822:giving the line bundle
10327:is the zero element in
9357:{\displaystyle 1,\tau }
9141:{\displaystyle 1,\tau }
4690:are given by functions
4634:is called the group of
3380:Isomorphic complex tori
1565:it has a period matrix
14674:
14592:Gerbes on complex tori
14339:
14307:
14287:
14149:
13922:
13894:
13836:
13816:
13775:
13712:
13692:
13672:
13640:
13602:
13573:
13521:
13474:
13473:{\displaystyle x\in X}
13448:
13428:
13399:
13379:
13359:
13300:
13241:
13221:
13169:
13148:
13086:
13066:
12986:
12934:
12868:
12841:
12801:
12711:Appell-Humbert theorem
12703:
12480:
12436:, we get a surjection
12430:
12410:
12390:
12389:{\displaystyle L\to X}
12364:
12340:
12301:
12266:
12167:
12139:
12119:
12062:
11976:
11862:
11796:
11776:
11737:
11697:
11662:
11567:
11521:
11489:
11035:
10998:
10865:
10828:
10796:
10712:
10672:
10596:
10535:
10489:
10469:
10449:
10411:
10387:
10353:
10321:
10297:
10277:
10174:
10133:
10106:
10078:
9992:
9941:
9914:
9894:
9832:
9783:
9740:
9358:
9332:
9142:
9116:
9054:
8959:
8937:
8897:
8845:
8817:
8776:
8758:For an elliptic curve
8744:
8701:
8681:
8661:
8566:
8518:
8498:
8478:
8443:
8395:
8360:
8276:
8212:
8172:
8171:{\displaystyle L\to X}
8146:
8107:
8075:
8012:
7966:
7926:
7904:
7882:
7881:{\displaystyle L\to X}
7856:
7829:
7809:Appell–Humbert theorem
7792:
7724:
7690:
7664:
7457:
7433:
7368:
7276:
7229:
7201:
6897:
6877:
6850:
6815:
6786:
6647:
6646:{\displaystyle v\in V}
6621:
6589:
6451:
6404:
6384:
6364:
6337:
6315:
6279:
6229:
6209:
6161:
6066:
5982:
5962:
5888:
5868:
5848:
5812:
5671:
5583:
5370:
5229:
5188:
5156:
5089:
5069:
4986:
4950:
4898:
4878:
4850:
4810:
4752:
4684:
4652:
4628:
4531:
4496:
4448:
4362:
4290:
4254:
4153:
4124:
4088:
3983:
3945:becomes trivial since
3939:
3909:
3875:are trivial using the
3869:
3824:
3823:{\displaystyle L\to X}
3795:
3781:For complex manifolds
3763:
3701:
3578:
3547:
3527:
3483:
3410:
3370:
3339:
3275:
3246:
3207:
3161:
3113:
3076:
3045:
3025:
2986:
2940:
2898:
2861:
2822:
2730:
2638:
2594:
2574:
2537:
2500:
2469:
2420:
2366:
2336:
2305:
2198:
2065:
1889:
1815:
1795:
1755:
1735:
1695:
1645:
1618:
1579:
1559:
1539:
1511:For any complex torus
1497:
1412:
1221:
1199:
1170:
1146:
1090:
1070:
1043:
981:
922:
864:
830:
767:
627:
607:
561:
541:
492:
472:
445:One way to describe a
431:
403:
377:
354:
316:
286:
246:
133:
40:
14675:
14370:Intermediate Jacobian
14340:
14308:
14288:
14150:
13923:
13895:
13837:
13817:
13776:
13713:
13693:
13673:
13641:
13603:
13574:
13522:
13475:
13449:
13429:
13400:
13380:
13360:
13301:
13242:
13222:
13170:
13149:
13087:
13067:
12987:
12935:
12869:
12842:
12802:
12704:
12481:
12431:
12411:
12391:
12365:
12341:
12302:
12267:
12168:
12140:
12120:
12063:
11982:The cocycle relation
11977:
11863:
11797:
11777:
11738:
11698:
11663:
11568:
11522:
11520:{\displaystyle NS(X)}
11490:
11036:
10999:
10866:
10829:
10797:
10713:
10673:
10597:
10536:
10490:
10470:
10450:
10412:
10388:
10354:
10352:{\displaystyle NS(X)}
10322:
10298:
10296:{\displaystyle \chi }
10278:
10175:
10134:
10119:For a Hermitian form
10107:
10079:
9993:
9942:
9940:{\displaystyle E_{a}}
9915:
9895:
9833:
9784:
9741:
9359:
9333:
9143:
9117:
9055:
8960:
8938:
8898:
8846:
8818:
8782:given by the lattice
8777:
8745:
8702:
8682:
8662:
8567:
8519:
8499:
8479:
8477:{\displaystyle NS(X)}
8444:
8396:
8361:
8277:
8213:
8173:
8147:
8108:
8076:
8013:
7967:
7927:
7905:
7883:
7857:
7855:{\displaystyle E_{L}}
7830:
7793:
7725:
7691:
7665:
7458:
7434:
7369:
7277:
7230:
7202:
6898:
6878:
6876:{\displaystyle E_{L}}
6851:
6816:
6787:
6648:
6622:
6590:
6452:
6405:
6385:
6365:
6363:{\displaystyle E_{L}}
6338:
6316:
6280:
6230:
6210:
6162:
6067:
5983:
5963:
5889:
5869:
5849:
5813:
5672:
5584:
5371:
5230:
5189:
5157:
5090:
5070:
4987:
4951:
4899:
4879:
4851:
4811:
4760:automorphic functions
4753:
4685:
4658:are also just called
4653:
4636:factors of automorphy
4629:
4532:
4497:
4449:
4363:
4291:
4255:
4154:
4125:
4089:
3993:Factors of automorphy
3984:
3940:
3910:
3870:
3825:
3796:
3764:
3702:
3579:
3548:
3528:
3484:
3411:
3371:
3340:
3276:
3247:
3208:
3162:
3114:
3077:
3046:
3026:
2987:
2941:
2899:
2862:
2823:
2731:
2639:
2595:
2575:
2538:
2501:
2470:
2421:
2367:
2342:is nonzero, equal to
2337:
2306:
2199:
2066:
1890:
1816:
1796:
1756:
1736:
1696:
1646:
1644:{\displaystyle 1_{g}}
1619:
1580:
1560:
1540:
1498:
1413:
1222:
1200:
1171:
1147:
1091:
1071:
1044:
982:
923:
865:
831:
768:
628:
608:
562:
542:
493:
473:
432:
404:
378:
355:
317:
287:
247:
134:
30:
14638:
14365:Automorphic function
14317:
14297:
14159:
13932:
13908:
13847:
13826:
13785:
13725:
13702:
13682:
13658:
13612:
13583:
13531:
13484:
13458:
13438:
13427:{\displaystyle K(L)}
13409:
13389:
13369:
13310:
13251:
13231:
13178:
13159:
13099:
13076:
12996:
12948:
12878:
12851:
12811:
12735:
12727:Dual abelian variety
12490:
12440:
12420:
12400:
12374:
12354:
12311:
12276:
12177:
12149:
12129:
12072:
11986:
11872:
11806:
11786:
11747:
11715:
11672:
11577:
11531:
11499:
11045:
11008:
10875:
10842:
10836:semi-character pairs
10806:
10722:
10682:
10606:
10545:
10499:
10479:
10459:
10421:
10401:
10363:
10331:
10311:
10287:
10184:
10143:
10123:
10088:
10002:
9951:
9924:
9904:
9842:
9793:
9750:
9368:
9342:
9152:
9126:
9064:
8969:
8947:
8907:
8855:
8827:
8786:
8762:
8711:
8691:
8671:
8576:
8528:
8508:
8488:
8456:
8419:
8415:For a complex torus
8373:
8287:
8225:
8182:
8156:
8120:
8085:
8023:
7979:
7936:
7914:
7892:
7866:
7839:
7817:
7813:For the alternating
7734:
7700:
7674:
7467:
7443:
7378:
7286:
7239:
7219:
6907:
6887:
6860:
6825:
6796:
6665:
6631:
6599:
6461:
6414:
6394:
6374:
6347:
6325:
6289:
6239:
6219:
6175:
6076:
6003:
5972:
5898:
5878:
5858:
5822:
5681:
5601:
5380:
5239:
5198:
5166:
5099:
5079:
4996:
4960:
4908:
4888:
4868:
4820:
4770:
4694:
4674:
4642:
4541:
4506:
4458:
4372:
4300:
4264:
4163:
4134:
4098:
4001:
3949:
3919:
3883:
3834:
3808:
3785:
3711:
3591:
3557:
3537:
3493:
3420:
3388:
3349:
3285:
3256:
3217:
3179:
3123:
3086:
3075:{\displaystyle X,X'}
3055:
3035:
2996:
2950:
2917:
2871:
2832:
2740:
2648:
2604:
2584:
2547:
2510:
2499:{\displaystyle g,g'}
2479:
2430:
2396:
2346:
2315:
2208:
2083:
1899:
1825:
1805:
1765:
1745:
1705:
1655:
1628:
1589:
1578:{\displaystyle \Pi }
1569:
1549:
1515:
1422:
1239:
1209:
1180:
1169:{\displaystyle \Pi }
1160:
1100:
1089:{\displaystyle \Pi }
1080:
1053:
991:
932:
874:
840:
777:
637:
633:. That is, we write
617:
571:
551:
502:
491:{\displaystyle \Pi }
482:
453:
413:
387:
367:
326:
296:
256:
236:
210:reasonably well. By
115:
14730:2013JGP....64..209B
13501:
10395:group of characters
8623:
8451:Neron-Serveri group
7357:
6123:
6055:
5951:
5804:
5757:
5657:
5572:
5512:
5456:
5362:
5315:
5235:. This gives a map
4617:
4204:
4077:
14670:
14558:10.1007/BF02570737
14335:
14303:
14283:
14281:
14145:
14143:
13918:
13890:
13832:
13812:
13771:
13708:
13688:
13668:
13636:
13598:
13569:
13517:
13487:
13470:
13444:
13424:
13395:
13375:
13355:
13296:
13237:
13217:
13165:
13144:
13094:dual complex torus
13082:
13062:
12982:
12930:
12864:
12837:
12797:
12717:Dual complex torus
12699:
12697:
12476:
12426:
12406:
12386:
12360:
12336:
12297:
12262:
12163:
12135:
12115:
12058:
11972:
11858:
11792:
11772:
11733:
11693:
11658:
11563:
11517:
11485:
11483:
11031:
10994:
10861:
10824:
10792:
10708:
10668:
10592:
10531:
10485:
10465:
10445:
10407:
10383:
10349:
10317:
10293:
10273:
10170:
10129:
10102:
10074:
9988:
9937:
9910:
9890:
9828:
9779:
9736:
9734:
9720:
9668:
9609:
9557:
9508:
9476:
9427:
9395:
9354:
9328:
9326:
9318:
9266:
9217:
9185:
9138:
9112:
9106:
9050:
8955:
8933:
8893:
8841:
8813:
8807:
8772:
8740:
8697:
8677:
8657:
8605:
8562:
8514:
8494:
8474:
8449:we can define the
8439:
8411:Néron–Severi group
8405:Neron-Severi group
8391:
8356:
8272:
8208:
8168:
8142:
8103:
8071:
8008:
7962:
7922:
7900:
7878:
7852:
7825:
7788:
7720:
7686:
7660:
7653:
7453:
7429:
7364:
7339:
7272:
7225:
7215:For a line bundle
7197:
7191:
6893:
6873:
6846:
6811:
6782:
6776:
6643:
6617:
6585:
6447:
6400:
6380:
6360:
6333:
6311:
6275:
6225:
6205:
6157:
6105:
6062:
6037:
5978:
5958:
5933:
5884:
5864:
5844:
5808:
5786:
5732:
5667:
5632:
5579:
5547:
5494:
5431:
5366:
5344:
5290:
5225:
5184:
5152:
5085:
5065:
4982:
4946:
4894:
4874:
4846:
4806:
4748:
4680:
4648:
4624:
4592:
4527:
4492:
4444:
4358:
4286:
4250:
4179:
4149:
4120:
4084:
4052:
3979:
3935:
3905:
3865:
3820:
3791:
3759:
3697:
3586:Double coset space
3574:
3543:
3523:
3479:
3406:
3366:
3335:
3271:
3242:
3203:
3157:
3109:
3072:
3041:
3021:
2982:
2936:
2894:
2857:
2818:
2726:
2634:
2590:
2570:
2533:
2496:
2465:
2416:
2362:
2332:
2301:
2295:
2194:
2188:
2061:
2059:
1885:
1879:
1811:
1791:
1751:
1731:
1691:
1641:
1614:
1575:
1555:
1535:
1493:
1487:
1408:
1402:
1217:
1195:
1176:span a lattice in
1166:
1142:
1136:
1086:
1066:
1039:
977:
918:
860:
826:
802:
763:
757:
623:
603:
557:
537:
488:
468:
427:
399:
373:
350:
312:
282:
242:
129:
41:
14825:Abelian varieties
14815:Complex manifolds
14645:
14518:978-0-8176-4103-0
14449:978-3-662-06307-1
14399:Abelian varieties
14360:Complex Lie group
14306:{\displaystyle H}
14255:
14187:
14110:
13987:
13962:
13885:
13835:{\displaystyle L}
13809:
13711:{\displaystyle X}
13691:{\displaystyle X}
13619:
13595:
13543:
13447:{\displaystyle X}
13398:{\displaystyle X}
13378:{\displaystyle L}
13329:
13321:
13240:{\displaystyle l}
13200:
13190:
13168:{\displaystyle X}
13141:
13125:
13111:
13031:
13008:
12955:
12910:
12898:
12889:
12676:
12655:
12628:
12510:
12465:
12429:{\displaystyle L}
12409:{\displaystyle H}
12363:{\displaystyle L}
11949:
11589:
11537:
11451:
11412:
11296:
11248:
10728:
10505:
10488:{\displaystyle X}
10468:{\displaystyle 0}
10428:
10320:{\displaystyle H}
10250:
10132:{\displaystyle H}
10072:
10060:
10053:
9913:{\displaystyle a}
9826:
9814:
8868:
8700:{\displaystyle V}
8680:{\displaystyle E}
8534:
8517:{\displaystyle V}
8497:{\displaystyle H}
8231:
8152:of a line bundle
7603:
7543:
7418:
7228:{\displaystyle L}
6403:{\displaystyle L}
5981:{\displaystyle X}
5887:{\displaystyle X}
5749:
5649:
5626:
5564:
5541:
5448:
5307:
5216:
5117:
5088:{\displaystyle f}
4992:-action given by
4943:
4920:
4897:{\displaystyle X}
4877:{\displaystyle f}
4683:{\displaystyle f}
4651:{\displaystyle f}
4609:
4524:
4340:
4229:
4196:
4146:
4069:
3961:
3862:
3794:{\displaystyle X}
3546:{\displaystyle g}
3281:which has kernel
3044:{\displaystyle h}
2838:
2788:
2759:
2696:
2667:
2593:{\displaystyle F}
2360:
2321:
2278:
2037:
2013:
1978:
1863:
1814:{\displaystyle P}
1774:
1754:{\displaystyle V}
1714:
1558:{\displaystyle g}
1132:
1064:
793:
626:{\displaystyle V}
376:{\displaystyle V}
245:{\displaystyle G}
173:abelian varieties
165:algebraic variety
91:complex dimension
68:cartesian product
58:whose underlying
16:(Redirected from
14832:
14820:Complex surfaces
14788:
14770:
14749:
14723:
14701:
14699:
14687:Crelle's Journal
14679:
14677:
14676:
14671:
14666:
14652:
14651:
14646:
14643:
14633:
14615:
14587:
14569:
14529:
14500:
14499:
14492:
14486:
14485:
14483:
14476:
14468:
14462:
14461:
14433:
14422:
14421:
14393:
14344:
14342:
14341:
14336:
14312:
14310:
14309:
14304:
14292:
14290:
14289:
14284:
14282:
14266:
14265:
14256:
14253:
14230:
14229:
14189:
14188:
14180:
14154:
14152:
14151:
14146:
14144:
14137:
14136:
14124:
14123:
14111:
14106:
14102:
14101:
14089:
14088:
14078:
14067:
14066:
14054:
14053:
14035:
14034:
14022:
14021:
13999:
13988:
13980:
13963:
13955:
13927:
13925:
13924:
13919:
13917:
13916:
13899:
13897:
13896:
13891:
13889:
13888:
13887:
13886:
13878:
13862:
13856:
13855:
13841:
13839:
13838:
13833:
13821:
13819:
13818:
13813:
13811:
13810:
13802:
13780:
13778:
13777:
13772:
13764:
13763:
13740:
13734:
13733:
13717:
13715:
13714:
13709:
13697:
13695:
13694:
13689:
13677:
13675:
13674:
13669:
13667:
13666:
13645:
13643:
13642:
13637:
13626:
13625:
13620:
13617:
13607:
13605:
13604:
13599:
13597:
13596:
13588:
13578:
13576:
13575:
13570:
13556:
13545:
13544:
13536:
13526:
13524:
13523:
13518:
13500:
13495:
13479:
13477:
13476:
13471:
13453:
13451:
13450:
13445:
13433:
13431:
13430:
13425:
13404:
13402:
13401:
13396:
13384:
13382:
13381:
13376:
13364:
13362:
13361:
13356:
13330:
13327:
13322:
13314:
13305:
13303:
13302:
13297:
13246:
13244:
13243:
13238:
13226:
13224:
13223:
13218:
13207:
13206:
13201:
13198:
13192:
13191:
13183:
13174:
13172:
13171:
13166:
13153:
13151:
13150:
13145:
13143:
13142:
13134:
13131:
13126:
13118:
13113:
13112:
13104:
13091:
13089:
13088:
13083:
13071:
13069:
13068:
13063:
13058:
13032:
13024:
13010:
13009:
13001:
12991:
12989:
12988:
12983:
12978:
12964:
12963:
12962:
12956:
12953:
12939:
12937:
12936:
12931:
12926:
12912:
12911:
12906:
12901:
12899:
12896:
12890:
12882:
12873:
12871:
12870:
12865:
12863:
12862:
12846:
12844:
12843:
12838:
12836:
12835:
12823:
12822:
12806:
12804:
12803:
12798:
12796:
12792:
12782:
12781:
12708:
12706:
12705:
12700:
12698:
12677:
12674:
12656:
12653:
12635:
12634:
12629:
12626:
12605:
12599:
12593:
12592:
12549:
12548:
12511:
12508:
12485:
12483:
12482:
12477:
12466:
12463:
12449:
12448:
12435:
12433:
12432:
12427:
12415:
12413:
12412:
12407:
12395:
12393:
12392:
12387:
12369:
12367:
12366:
12361:
12345:
12343:
12342:
12337:
12335:
12334:
12306:
12304:
12303:
12298:
12271:
12269:
12268:
12263:
12240:
12239:
12172:
12170:
12169:
12164:
12162:
12144:
12142:
12141:
12136:
12124:
12122:
12121:
12116:
12111:
12106:
12067:
12065:
12064:
12059:
11981:
11979:
11978:
11973:
11950:
11942:
11867:
11865:
11864:
11859:
11857:
11856:
11851:
11830:
11829:
11801:
11799:
11798:
11793:
11781:
11779:
11778:
11773:
11771:
11770:
11742:
11740:
11739:
11734:
11702:
11700:
11699:
11694:
11667:
11665:
11664:
11659:
11624:
11623:
11590:
11587:
11572:
11570:
11569:
11564:
11538:
11535:
11526:
11524:
11523:
11518:
11494:
11492:
11491:
11486:
11484:
11462:
11461:
11452:
11449:
11423:
11422:
11413:
11410:
11384:
11383:
11374:
11373:
11355:
11354:
11345:
11344:
11329:
11307:
11306:
11297:
11294:
11259:
11258:
11249:
11246:
11220:
11219:
11201:
11200:
11182:
11181:
11163:
11162:
11147:
11128:
11127:
11103:
11102:
11071:
11070:
11061:
11060:
11040:
11038:
11037:
11032:
11030:
11029:
11020:
11019:
11003:
11001:
11000:
10995:
10990:
10989:
10980:
10979:
10967:
10966:
10954:
10953:
10935:
10934:
10922:
10921:
10903:
10902:
10890:
10889:
10870:
10868:
10867:
10862:
10851:
10850:
10838:, forms a group
10833:
10831:
10830:
10825:
10801:
10799:
10798:
10793:
10791:
10790:
10782:
10776:
10771:
10770:
10762:
10729:
10726:
10717:
10715:
10714:
10709:
10707:
10706:
10694:
10693:
10677:
10675:
10674:
10669:
10667:
10663:
10653:
10652:
10601:
10599:
10598:
10593:
10576:
10571:
10566:
10558:
10540:
10538:
10537:
10532:
10506:
10503:
10494:
10492:
10491:
10486:
10475:line bundles on
10474:
10472:
10471:
10466:
10454:
10452:
10451:
10446:
10435:
10434:
10429:
10426:
10416:
10414:
10413:
10408:
10392:
10390:
10389:
10384:
10370:
10358:
10356:
10355:
10350:
10326:
10324:
10323:
10318:
10302:
10300:
10299:
10294:
10282:
10280:
10279:
10274:
10251:
10248:
10179:
10177:
10176:
10171:
10138:
10136:
10135:
10130:
10111:
10109:
10108:
10103:
10101:
10083:
10081:
10080:
10075:
10073:
10071:
10061:
10058:
10055:
10054:
10046:
10040:
10014:
10013:
9997:
9995:
9994:
9989:
9987:
9979:
9971:
9963:
9962:
9946:
9944:
9943:
9938:
9936:
9935:
9919:
9917:
9916:
9911:
9899:
9897:
9896:
9891:
9837:
9835:
9834:
9829:
9827:
9825:
9815:
9812:
9806:
9788:
9786:
9785:
9780:
9778:
9745:
9743:
9742:
9737:
9735:
9725:
9724:
9717:
9716:
9697:
9696:
9673:
9672:
9665:
9664:
9651:
9650:
9632:
9630:
9629:
9614:
9613:
9606:
9605:
9586:
9585:
9562:
9561:
9532:
9531:
9513:
9512:
9481:
9480:
9473:
9472:
9459:
9458:
9440:
9432:
9431:
9400:
9399:
9363:
9361:
9360:
9355:
9337:
9335:
9334:
9329:
9327:
9323:
9322:
9315:
9314:
9295:
9294:
9271:
9270:
9263:
9262:
9249:
9248:
9224:
9222:
9221:
9190:
9189:
9147:
9145:
9144:
9139:
9121:
9119:
9118:
9113:
9111:
9110:
9059:
9057:
9056:
9051:
9049:
9048:
9039:
9038:
9026:
9025:
9016:
9015:
9003:
9002:
8987:
8986:
8964:
8962:
8961:
8956:
8954:
8942:
8940:
8939:
8934:
8932:
8931:
8919:
8918:
8902:
8900:
8899:
8894:
8889:
8875:
8874:
8869:
8866:
8850:
8848:
8847:
8842:
8840:
8822:
8820:
8819:
8814:
8812:
8811:
8781:
8779:
8778:
8773:
8771:
8770:
8749:
8747:
8746:
8741:
8739:
8706:
8704:
8703:
8698:
8686:
8684:
8683:
8678:
8666:
8664:
8663:
8658:
8653:
8639:
8638:
8622:
8617:
8612:
8611:
8601:
8600:
8588:
8587:
8571:
8569:
8568:
8563:
8561:
8535:
8532:
8523:
8521:
8520:
8515:
8503:
8501:
8500:
8495:
8483:
8481:
8480:
8475:
8448:
8446:
8445:
8440:
8435:
8400:
8398:
8397:
8392:
8365:
8363:
8362:
8357:
8281:
8279:
8278:
8273:
8232:
8229:
8217:
8215:
8214:
8209:
8207:
8177:
8175:
8174:
8169:
8151:
8149:
8148:
8143:
8132:
8131:
8112:
8110:
8109:
8104:
8080:
8078:
8077:
8072:
8017:
8015:
8014:
8009:
8007:
7971:
7969:
7968:
7963:
7961:
7931:
7929:
7928:
7923:
7921:
7909:
7907:
7906:
7901:
7899:
7887:
7885:
7884:
7879:
7861:
7859:
7858:
7853:
7851:
7850:
7834:
7832:
7831:
7826:
7824:
7797:
7795:
7794:
7789:
7729:
7727:
7726:
7721:
7719:
7695:
7693:
7692:
7687:
7669:
7667:
7666:
7661:
7659:
7655:
7654:
7641:
7640:
7604:
7601:
7544:
7541:
7529:
7512:
7511:
7476:
7475:
7462:
7460:
7459:
7454:
7452:
7451:
7438:
7436:
7435:
7430:
7419:
7416:
7390:
7389:
7373:
7371:
7370:
7365:
7356:
7351:
7346:
7345:
7329:
7328:
7310:
7309:
7281:
7279:
7278:
7273:
7271:
7270:
7265:
7234:
7232:
7231:
7226:
7206:
7204:
7203:
7198:
7196:
7195:
7183:
7182:
7162:
7161:
7141:
7140:
7118:
7117:
7095:
7094:
7074:
7073:
7051:
7050:
7033:
7032:
7010:
7009:
6987:
6986:
6969:
6968:
6951:
6950:
6919:
6918:
6902:
6900:
6899:
6894:
6882:
6880:
6879:
6874:
6872:
6871:
6855:
6853:
6852:
6847:
6845:
6844:
6839:
6820:
6818:
6817:
6812:
6810:
6809:
6804:
6791:
6789:
6788:
6783:
6781:
6780:
6763:
6762:
6745:
6744:
6715:
6714:
6697:
6696:
6652:
6650:
6649:
6644:
6626:
6624:
6623:
6618:
6594:
6592:
6591:
6586:
6473:
6472:
6456:
6454:
6453:
6448:
6409:
6407:
6406:
6401:
6389:
6387:
6386:
6381:
6369:
6367:
6366:
6361:
6359:
6358:
6342:
6340:
6339:
6334:
6332:
6320:
6318:
6317:
6312:
6301:
6300:
6284:
6282:
6281:
6276:
6271:
6257:
6256:
6234:
6232:
6231:
6226:
6214:
6212:
6211:
6206:
6201:
6187:
6186:
6166:
6164:
6163:
6158:
6153:
6139:
6138:
6122:
6117:
6112:
6111:
6101:
6100:
6088:
6087:
6071:
6069:
6068:
6063:
6054:
6049:
6044:
6043:
6033:
6032:
6027:
6026:
6016:
5987:
5985:
5984:
5979:
5967:
5965:
5964:
5959:
5950:
5945:
5940:
5939:
5929:
5928:
5910:
5909:
5893:
5891:
5890:
5885:
5873:
5871:
5870:
5865:
5853:
5851:
5850:
5845:
5834:
5833:
5817:
5815:
5814:
5809:
5803:
5798:
5793:
5792:
5776:
5775:
5756:
5751:
5750:
5742:
5739:
5738:
5728:
5727:
5706:
5705:
5693:
5692:
5676:
5674:
5673:
5668:
5656:
5651:
5650:
5642:
5639:
5638:
5628:
5627:
5619:
5613:
5612:
5593:For complex tori
5588:
5586:
5585:
5580:
5571:
5566:
5565:
5557:
5554:
5553:
5543:
5542:
5534:
5528:
5527:
5511:
5506:
5501:
5500:
5484:
5483:
5455:
5450:
5449:
5441:
5438:
5437:
5427:
5426:
5405:
5404:
5392:
5391:
5375:
5373:
5372:
5367:
5361:
5356:
5351:
5350:
5334:
5333:
5314:
5309:
5308:
5300:
5297:
5296:
5286:
5285:
5264:
5263:
5251:
5250:
5234:
5232:
5231:
5226:
5218:
5217:
5209:
5193:
5191:
5190:
5185:
5161:
5159:
5158:
5153:
5142:
5141:
5132:
5127:
5119:
5118:
5110:
5094:
5092:
5091:
5086:
5074:
5072:
5071:
5066:
4991:
4989:
4988:
4983:
4972:
4971:
4955:
4953:
4952:
4947:
4945:
4944:
4936:
4930:
4922:
4921:
4913:
4903:
4901:
4900:
4895:
4883:
4881:
4880:
4875:
4855:
4853:
4852:
4847:
4845:
4815:
4813:
4812:
4807:
4757:
4755:
4754:
4749:
4747:
4746:
4741:
4732:
4731:
4723:
4714:
4713:
4708:
4689:
4687:
4686:
4681:
4657:
4655:
4654:
4649:
4633:
4631:
4630:
4625:
4616:
4611:
4610:
4602:
4599:
4598:
4588:
4587:
4566:
4565:
4553:
4552:
4536:
4534:
4533:
4528:
4526:
4525:
4517:
4501:
4499:
4498:
4493:
4482:
4481:
4453:
4451:
4450:
4445:
4367:
4365:
4364:
4359:
4357:
4356:
4351:
4342:
4341:
4333:
4318:
4317:
4295:
4293:
4292:
4287:
4276:
4275:
4259:
4257:
4256:
4251:
4246:
4245:
4240:
4231:
4230:
4222:
4203:
4198:
4197:
4189:
4186:
4185:
4175:
4174:
4158:
4156:
4155:
4150:
4148:
4147:
4139:
4129:
4127:
4126:
4121:
4110:
4109:
4093:
4091:
4090:
4085:
4076:
4071:
4070:
4062:
4059:
4058:
4048:
4047:
4026:
4025:
4013:
4012:
3988:
3986:
3985:
3980:
3978:
3977:
3972:
3963:
3962:
3954:
3944:
3942:
3941:
3936:
3931:
3930:
3914:
3912:
3911:
3906:
3895:
3894:
3877:group cohomology
3874:
3872:
3871:
3866:
3864:
3863:
3855:
3846:
3845:
3829:
3827:
3826:
3821:
3800:
3798:
3797:
3792:
3768:
3766:
3765:
3760:
3758:
3757:
3742:
3741:
3726:
3725:
3706:
3704:
3703:
3698:
3687:
3686:
3685:
3672:
3655:
3654:
3653:
3625:
3624:
3623:
3607:
3606:
3601:
3600:
3583:
3581:
3580:
3575:
3573:
3572:
3567:
3566:
3552:
3550:
3549:
3544:
3532:
3530:
3529:
3524:
3510:
3509:
3508:
3488:
3486:
3485:
3480:
3469:
3468:
3467:
3454:
3437:
3436:
3435:
3415:
3413:
3412:
3407:
3405:
3404:
3396:
3375:
3373:
3372:
3367:
3359:
3344:
3342:
3341:
3336:
3334:
3333:
3321:
3313:
3308:
3297:
3296:
3280:
3278:
3277:
3272:
3251:
3249:
3248:
3243:
3229:
3228:
3212:
3210:
3209:
3204:
3202:
3201:
3193:
3166:
3164:
3163:
3158:
3150:
3149:
3118:
3116:
3115:
3110:
3108:
3081:
3079:
3078:
3073:
3071:
3050:
3048:
3047:
3042:
3030:
3028:
3027:
3022:
3020:
3019:
2991:
2989:
2988:
2983:
2969:
2968:
2967:
2966:
2945:
2943:
2942:
2937:
2929:
2928:
2903:
2901:
2900:
2895:
2893:
2866:
2864:
2863:
2858:
2856:
2839:
2836:
2827:
2825:
2824:
2819:
2814:
2797:
2796:
2795:
2789:
2786:
2777:
2760:
2757:
2752:
2751:
2735:
2733:
2732:
2727:
2722:
2705:
2704:
2703:
2697:
2694:
2685:
2668:
2665:
2660:
2659:
2643:
2641:
2640:
2635:
2633:
2616:
2615:
2599:
2597:
2596:
2591:
2579:
2577:
2576:
2571:
2569:
2542:
2540:
2539:
2534:
2532:
2505:
2503:
2502:
2497:
2495:
2474:
2472:
2471:
2466:
2464:
2456:
2451:
2440:
2425:
2423:
2422:
2417:
2412:
2371:
2369:
2368:
2363:
2361:
2356:
2341:
2339:
2338:
2333:
2322:
2319:
2310:
2308:
2307:
2302:
2300:
2299:
2279:
2274:
2203:
2201:
2200:
2195:
2193:
2192:
2175:
2174:
2157:
2156:
2127:
2126:
2109:
2108:
2070:
2068:
2067:
2062:
2060:
2038:
2035:
2021:
2014:
2006:
1986:
1979:
1971:
1969:
1968:
1959:
1958:
1934:
1933:
1894:
1892:
1891:
1886:
1884:
1883:
1876:
1875:
1864:
1856:
1850:
1849:
1820:
1818:
1817:
1812:
1800:
1798:
1797:
1792:
1775:
1772:
1760:
1758:
1757:
1752:
1740:
1738:
1737:
1732:
1715:
1712:
1700:
1698:
1697:
1692:
1681:
1680:
1679:
1650:
1648:
1647:
1642:
1640:
1639:
1623:
1621:
1620:
1615:
1610:
1609:
1584:
1582:
1581:
1576:
1564:
1562:
1561:
1556:
1544:
1542:
1541:
1536:
1531:
1502:
1500:
1499:
1494:
1492:
1491:
1417:
1415:
1414:
1409:
1407:
1406:
1399:
1398:
1381:
1380:
1363:
1362:
1345:
1344:
1325:
1324:
1307:
1306:
1289:
1288:
1271:
1270:
1226:
1224:
1223:
1218:
1216:
1204:
1202:
1201:
1196:
1194:
1193:
1188:
1175:
1173:
1172:
1167:
1151:
1149:
1148:
1143:
1141:
1140:
1133:
1125:
1095:
1093:
1092:
1087:
1075:
1073:
1072:
1067:
1065:
1057:
1048:
1046:
1045:
1040:
1017:
1016:
1015:
986:
984:
983:
978:
958:
957:
956:
927:
925:
924:
919:
917:
916:
908:
899:
894:
893:
888:
869:
867:
866:
861:
856:
835:
833:
832:
827:
825:
824:
815:
814:
801:
789:
788:
772:
770:
769:
764:
762:
761:
754:
753:
728:
727:
704:
695:
694:
669:
668:
632:
630:
629:
624:
612:
610:
609:
604:
602:
601:
583:
582:
566:
564:
563:
558:
546:
544:
543:
538:
536:
535:
514:
513:
497:
495:
494:
489:
477:
475:
474:
469:
436:
434:
433:
428:
423:
408:
406:
405:
400:
382:
380:
379:
374:
359:
357:
356:
351:
340:
335:
334:
321:
319:
318:
313:
311:
310:
291:
289:
288:
283:
278:
277:
265:
264:
251:
249:
248:
243:
216:projective space
204:Computer algebra
198:description for
161:Bernhard Riemann
151:construction of
138:
136:
135:
130:
125:
53:complex manifold
21:
14840:
14839:
14835:
14834:
14833:
14831:
14830:
14829:
14805:
14804:
14796:
14662:
14647:
14642:
14641:
14639:
14636:
14635:
14622:10.4171/JNCG/96
14594:
14536:
14519:
14503:
14494:
14493:
14489:
14481:
14474:
14470:
14469:
14465:
14450:
14434:
14425:
14410:
14394:
14387:
14383:
14355:Poincare bundle
14351:
14318:
14315:
14314:
14298:
14295:
14294:
14280:
14279:
14261:
14257:
14252:
14225:
14221:
14206:
14205:
14179:
14178:
14162:
14160:
14157:
14156:
14142:
14141:
14132:
14128:
14119:
14115:
14097:
14093:
14084:
14080:
14079:
14077:
14062:
14058:
14049:
14045:
14030:
14026:
14017:
14013:
14001:
14000:
13995:
13979:
13954:
13935:
13933:
13930:
13929:
13912:
13911:
13909:
13906:
13905:
13877:
13876:
13863:
13858:
13857:
13851:
13850:
13848:
13845:
13844:
13827:
13824:
13823:
13801:
13800:
13786:
13783:
13782:
13741:
13736:
13735:
13729:
13728:
13726:
13723:
13722:
13703:
13700:
13699:
13683:
13680:
13679:
13662:
13661:
13659:
13656:
13655:
13652:
13650:Poincare bundle
13621:
13616:
13615:
13613:
13610:
13609:
13587:
13586:
13584:
13581:
13580:
13552:
13535:
13534:
13532:
13529:
13528:
13496:
13491:
13485:
13482:
13481:
13459:
13456:
13455:
13439:
13436:
13435:
13410:
13407:
13406:
13390:
13387:
13386:
13370:
13367:
13366:
13326:
13313:
13311:
13308:
13307:
13306:giving the map
13252:
13249:
13248:
13232:
13229:
13228:
13202:
13197:
13196:
13182:
13181:
13179:
13176:
13175:
13160:
13157:
13156:
13133:
13132:
13127:
13117:
13103:
13102:
13100:
13097:
13096:
13077:
13074:
13073:
13054:
13023:
13000:
12999:
12997:
12994:
12993:
12974:
12958:
12957:
12952:
12951:
12949:
12946:
12945:
12922:
12902:
12900:
12895:
12894:
12881:
12879:
12876:
12875:
12858:
12854:
12852:
12849:
12848:
12831:
12827:
12818:
12814:
12812:
12809:
12808:
12777:
12773:
12763:
12759:
12736:
12733:
12732:
12729:
12723:Poincare bundle
12719:
12696:
12695:
12687:
12673:
12671:
12666:
12652:
12650:
12645:
12630:
12625:
12624:
12622:
12617:
12611:
12610:
12604:
12598:
12590:
12589:
12581:
12564:
12559:
12544:
12543:
12541:
12536:
12507:
12505:
12500:
12493:
12491:
12488:
12487:
12462:
12444:
12443:
12441:
12438:
12437:
12421:
12418:
12417:
12401:
12398:
12397:
12375:
12372:
12371:
12355:
12352:
12351:
12318:
12314:
12312:
12309:
12308:
12277:
12274:
12273:
12223:
12219:
12178:
12175:
12174:
12158:
12150:
12147:
12146:
12130:
12127:
12126:
12107:
12102:
12073:
12070:
12069:
11987:
11984:
11983:
11941:
11873:
11870:
11869:
11852:
11847:
11846:
11813:
11809:
11807:
11804:
11803:
11787:
11784:
11783:
11754:
11750:
11748:
11745:
11744:
11716:
11713:
11712:
11709:
11673:
11670:
11669:
11619:
11618:
11586:
11578:
11575:
11574:
11534:
11532:
11529:
11528:
11527:and has kernel
11500:
11497:
11496:
11482:
11481:
11457:
11453:
11448:
11418:
11414:
11409:
11379:
11375:
11369:
11365:
11350:
11346:
11340:
11336:
11327:
11326:
11302:
11298:
11293:
11254:
11250:
11245:
11215:
11211:
11196:
11192:
11177:
11173:
11158:
11154:
11145:
11144:
11123:
11119:
11098:
11094:
11087:
11066:
11062:
11056:
11052:
11048:
11046:
11043:
11042:
11025:
11021:
11015:
11011:
11009:
11006:
11005:
10985:
10981:
10975:
10971:
10962:
10958:
10949:
10945:
10930:
10926:
10917:
10913:
10898:
10894:
10885:
10881:
10876:
10873:
10872:
10846:
10845:
10843:
10840:
10839:
10807:
10804:
10803:
10783:
10778:
10777:
10772:
10763:
10758:
10757:
10725:
10723:
10720:
10719:
10702:
10698:
10689:
10685:
10683:
10680:
10679:
10648:
10644:
10634:
10630:
10607:
10604:
10603:
10572:
10567:
10562:
10554:
10546:
10543:
10542:
10502:
10500:
10497:
10496:
10480:
10477:
10476:
10460:
10457:
10456:
10430:
10425:
10424:
10422:
10419:
10418:
10402:
10399:
10398:
10366:
10364:
10361:
10360:
10332:
10329:
10328:
10312:
10309:
10308:
10303:behaves like a
10288:
10285:
10284:
10247:
10185:
10182:
10181:
10144:
10141:
10140:
10124:
10121:
10120:
10117:
10097:
10089:
10086:
10085:
10057:
10056:
10045:
10041:
10039:
10009:
10005:
10003:
10000:
9999:
9983:
9975:
9967:
9958:
9954:
9952:
9949:
9948:
9931:
9927:
9925:
9922:
9921:
9905:
9902:
9901:
9843:
9840:
9839:
9811:
9810:
9805:
9794:
9791:
9790:
9774:
9751:
9748:
9747:
9733:
9732:
9719:
9718:
9712:
9708:
9699:
9698:
9692:
9688:
9678:
9677:
9667:
9666:
9660:
9656:
9653:
9652:
9646:
9642:
9635:
9634:
9631:
9625:
9621:
9608:
9607:
9601:
9597:
9588:
9587:
9581:
9577:
9567:
9566:
9556:
9555:
9549:
9548:
9538:
9537:
9534:
9533:
9527:
9523:
9507:
9506:
9497:
9496:
9486:
9485:
9475:
9474:
9468:
9464:
9461:
9460:
9454:
9450:
9443:
9442:
9439:
9426:
9425:
9416:
9415:
9405:
9404:
9394:
9393:
9387:
9386:
9376:
9375:
9371:
9369:
9366:
9365:
9343:
9340:
9339:
9325:
9324:
9317:
9316:
9310:
9306:
9297:
9296:
9290:
9286:
9276:
9275:
9265:
9264:
9258:
9254:
9251:
9250:
9244:
9240:
9233:
9232:
9223:
9216:
9215:
9206:
9205:
9195:
9194:
9184:
9183:
9177:
9176:
9166:
9165:
9155:
9153:
9150:
9149:
9127:
9124:
9123:
9105:
9104:
9099:
9090:
9089:
9084:
9074:
9073:
9065:
9062:
9061:
9044:
9040:
9034:
9030:
9021:
9017:
9011:
9007:
8998:
8994:
8982:
8978:
8970:
8967:
8966:
8950:
8948:
8945:
8944:
8927:
8923:
8914:
8910:
8908:
8905:
8904:
8885:
8870:
8865:
8864:
8856:
8853:
8852:
8836:
8828:
8825:
8824:
8806:
8805:
8800:
8790:
8789:
8787:
8784:
8783:
8766:
8765:
8763:
8760:
8759:
8756:
8735:
8712:
8709:
8708:
8692:
8689:
8688:
8672:
8669:
8668:
8649:
8634:
8630:
8618:
8613:
8607:
8606:
8596:
8592:
8583:
8579:
8577:
8574:
8573:
8557:
8531:
8529:
8526:
8525:
8509:
8506:
8505:
8489:
8486:
8485:
8457:
8454:
8453:
8431:
8420:
8417:
8416:
8413:
8407:
8374:
8371:
8370:
8288:
8285:
8284:
8228:
8226:
8223:
8222:
8203:
8183:
8180:
8179:
8157:
8154:
8153:
8127:
8123:
8121:
8118:
8117:
8086:
8083:
8082:
8024:
8021:
8020:
8003:
7980:
7977:
7976:
7957:
7937:
7934:
7933:
7917:
7915:
7912:
7911:
7895:
7893:
7890:
7889:
7867:
7864:
7863:
7846:
7842:
7840:
7837:
7836:
7835:-valued 2-form
7820:
7818:
7815:
7814:
7811:
7805:
7735:
7732:
7731:
7715:
7701:
7698:
7697:
7675:
7672:
7671:
7652:
7651:
7633:
7629:
7600:
7597:
7596:
7540:
7533:
7525:
7504:
7500:
7493:
7489:
7471:
7470:
7468:
7465:
7464:
7447:
7446:
7444:
7441:
7440:
7415:
7385:
7381:
7379:
7376:
7375:
7352:
7347:
7341:
7340:
7324:
7320:
7305:
7301:
7287:
7284:
7283:
7266:
7261:
7260:
7240:
7237:
7236:
7220:
7217:
7216:
7213:
7190:
7189:
7184:
7172:
7168:
7163:
7151:
7147:
7142:
7130:
7126:
7120:
7119:
7107:
7103:
7101:
7096:
7084:
7080:
7075:
7063:
7059:
7053:
7052:
7040:
7036:
7034:
7022:
7018:
7016:
7011:
6999:
6995:
6989:
6988:
6976:
6972:
6970:
6958:
6954:
6952:
6940:
6936:
6934:
6924:
6923:
6914:
6910:
6908:
6905:
6904:
6903:is of the form
6888:
6885:
6884:
6867:
6863:
6861:
6858:
6857:
6840:
6835:
6834:
6826:
6823:
6822:
6805:
6800:
6799:
6797:
6794:
6793:
6775:
6774:
6769:
6764:
6752:
6748:
6746:
6734:
6730:
6727:
6726:
6721:
6716:
6704:
6700:
6698:
6686:
6682:
6675:
6674:
6666:
6663:
6662:
6659:
6632:
6629:
6628:
6600:
6597:
6596:
6468:
6464:
6462:
6459:
6458:
6415:
6412:
6411:
6395:
6392:
6391:
6375:
6372:
6371:
6354:
6350:
6348:
6345:
6344:
6343:-valued 2-form
6328:
6326:
6323:
6322:
6296:
6292:
6290:
6287:
6286:
6267:
6252:
6248:
6240:
6237:
6236:
6220:
6217:
6216:
6197:
6182:
6178:
6176:
6173:
6172:
6149:
6134:
6130:
6118:
6113:
6107:
6106:
6096:
6092:
6083:
6079:
6077:
6074:
6073:
6050:
6045:
6039:
6038:
6028:
6022:
6021:
6020:
6012:
6004:
6001:
6000:
5994:
5973:
5970:
5969:
5946:
5941:
5935:
5934:
5924:
5920:
5905:
5901:
5899:
5896:
5895:
5879:
5876:
5875:
5859:
5856:
5855:
5854:as the lattice
5829:
5825:
5823:
5820:
5819:
5799:
5794:
5788:
5787:
5771:
5767:
5752:
5741:
5740:
5734:
5733:
5723:
5719:
5701:
5697:
5688:
5684:
5682:
5679:
5678:
5652:
5641:
5640:
5634:
5633:
5618:
5617:
5608:
5604:
5602:
5599:
5598:
5595:
5567:
5556:
5555:
5549:
5548:
5533:
5532:
5523:
5519:
5507:
5502:
5496:
5495:
5479:
5475:
5451:
5440:
5439:
5433:
5432:
5422:
5418:
5400:
5396:
5387:
5383:
5381:
5378:
5377:
5357:
5352:
5346:
5345:
5329:
5325:
5310:
5299:
5298:
5292:
5291:
5281:
5277:
5259:
5255:
5246:
5242:
5240:
5237:
5236:
5208:
5207:
5199:
5196:
5195:
5167:
5164:
5163:
5137:
5133:
5128:
5123:
5109:
5108:
5100:
5097:
5096:
5080:
5077:
5076:
5075:for the factor
4997:
4994:
4993:
4967:
4963:
4961:
4958:
4957:
4935:
4934:
4926:
4912:
4911:
4909:
4906:
4905:
4889:
4886:
4885:
4869:
4866:
4865:
4862:
4841:
4821:
4818:
4817:
4771:
4768:
4767:
4764:theta functions
4742:
4737:
4736:
4724:
4719:
4718:
4709:
4704:
4703:
4695:
4692:
4691:
4675:
4672:
4671:
4668:
4666:On complex tori
4643:
4640:
4639:
4612:
4601:
4600:
4594:
4593:
4583:
4579:
4561:
4557:
4548:
4544:
4542:
4539:
4538:
4516:
4515:
4507:
4504:
4503:
4477:
4473:
4459:
4456:
4455:
4373:
4370:
4369:
4352:
4347:
4346:
4332:
4331:
4313:
4309:
4301:
4298:
4297:
4271:
4267:
4265:
4262:
4261:
4241:
4236:
4235:
4221:
4220:
4199:
4188:
4187:
4181:
4180:
4170:
4166:
4164:
4161:
4160:
4138:
4137:
4135:
4132:
4131:
4105:
4101:
4099:
4096:
4095:
4072:
4061:
4060:
4054:
4053:
4043:
4039:
4021:
4017:
4008:
4004:
4002:
3999:
3998:
3995:
3973:
3968:
3967:
3953:
3952:
3950:
3947:
3946:
3926:
3922:
3920:
3917:
3916:
3890:
3886:
3884:
3881:
3880:
3854:
3853:
3841:
3837:
3835:
3832:
3831:
3830:whose pullback
3809:
3806:
3805:
3786:
3783:
3782:
3779:
3753:
3749:
3737:
3733:
3721:
3717:
3712:
3709:
3708:
3681:
3680:
3676:
3668:
3649:
3648:
3644:
3619:
3618:
3614:
3602:
3596:
3595:
3594:
3592:
3589:
3588:
3568:
3562:
3561:
3560:
3558:
3555:
3554:
3538:
3535:
3534:
3504:
3503:
3499:
3494:
3491:
3490:
3463:
3462:
3458:
3450:
3431:
3430:
3426:
3421:
3418:
3417:
3397:
3392:
3391:
3389:
3386:
3385:
3382:
3355:
3350:
3347:
3346:
3326:
3322:
3317:
3309:
3304:
3292:
3288:
3286:
3283:
3282:
3257:
3254:
3253:
3224:
3220:
3218:
3215:
3214:
3194:
3189:
3188:
3180:
3177:
3176:
3173:
3136:
3132:
3124:
3121:
3120:
3101:
3087:
3084:
3083:
3064:
3056:
3053:
3052:
3036:
3033:
3032:
3015:
3011:
2997:
2994:
2993:
2962:
2958:
2957:
2953:
2951:
2948:
2947:
2924:
2920:
2918:
2915:
2914:
2910:
2886:
2872:
2869:
2868:
2852:
2835:
2833:
2830:
2829:
2807:
2791:
2790:
2785:
2784:
2770:
2756:
2747:
2743:
2741:
2738:
2737:
2715:
2699:
2698:
2693:
2692:
2678:
2664:
2655:
2651:
2649:
2646:
2645:
2626:
2611:
2607:
2605:
2602:
2601:
2585:
2582:
2581:
2562:
2548:
2545:
2544:
2525:
2511:
2508:
2507:
2488:
2480:
2477:
2476:
2457:
2452:
2444:
2433:
2431:
2428:
2427:
2408:
2397:
2394:
2393:
2390:
2378:
2355:
2347:
2344:
2343:
2318:
2316:
2313:
2312:
2294:
2293:
2288:
2283:
2273:
2265:
2250:
2249:
2244:
2239:
2228:
2212:
2211:
2209:
2206:
2205:
2187:
2186:
2181:
2176:
2164:
2160:
2158:
2146:
2142:
2139:
2138:
2133:
2128:
2116:
2112:
2110:
2098:
2094:
2087:
2086:
2084:
2081:
2080:
2077:
2058:
2057:
2034:
2019:
2018:
2005:
1984:
1983:
1970:
1964:
1960:
1954:
1950:
1929:
1925:
1912:
1902:
1900:
1897:
1896:
1878:
1877:
1871:
1867:
1865:
1855:
1852:
1851:
1845:
1841:
1839:
1829:
1828:
1826:
1823:
1822:
1806:
1803:
1802:
1771:
1766:
1763:
1762:
1746:
1743:
1742:
1711:
1706:
1703:
1702:
1675:
1674:
1670:
1656:
1653:
1652:
1635:
1631:
1629:
1626:
1625:
1605:
1601:
1590:
1587:
1586:
1570:
1567:
1566:
1550:
1547:
1546:
1527:
1516:
1513:
1512:
1509:
1486:
1485:
1480:
1475:
1467:
1461:
1460:
1452:
1447:
1442:
1432:
1431:
1423:
1420:
1419:
1401:
1400:
1388:
1384:
1382:
1370:
1366:
1364:
1352:
1348:
1346:
1334:
1330:
1327:
1326:
1314:
1310:
1308:
1296:
1292:
1290:
1278:
1274:
1272:
1260:
1256:
1249:
1248:
1240:
1237:
1236:
1233:
1212:
1210:
1207:
1206:
1189:
1184:
1183:
1181:
1178:
1177:
1161:
1158:
1157:
1135:
1134:
1124:
1121:
1120:
1110:
1109:
1101:
1098:
1097:
1081:
1078:
1077:
1056:
1054:
1051:
1050:
1011:
1010:
1006:
992:
989:
988:
952:
951:
947:
933:
930:
929:
909:
904:
903:
895:
889:
884:
883:
875:
872:
871:
852:
841:
838:
837:
820:
816:
807:
803:
797:
784:
780:
778:
775:
774:
756:
755:
740:
736:
734:
729:
717:
713:
710:
709:
703:
697:
696:
681:
677:
675:
670:
658:
654:
647:
646:
638:
635:
634:
618:
615:
614:
597:
593:
578:
574:
572:
569:
568:
552:
549:
548:
547:of the lattice
528:
524:
509:
505:
503:
500:
499:
483:
480:
479:
454:
451:
450:
443:
419:
414:
411:
410:
388:
385:
384:
368:
365:
364:
336:
330:
329:
327:
324:
323:
306:
305:
297:
294:
293:
273:
269:
260:
259:
257:
254:
253:
237:
234:
233:
224:
188:theta-functions
153:elliptic curves
121:
116:
113:
112:
70:of some number
60:smooth manifold
38:
34:
23:
22:
15:
12:
11:
5:
14838:
14828:
14827:
14822:
14817:
14803:
14802:
14795:
14792:
14791:
14790:
14750:
14703:
14681:
14669:
14665:
14661:
14658:
14655:
14650:
14606:(3): 407–455.
14593:
14590:
14589:
14588:
14571:
14535:
14532:
14531:
14530:
14517:
14502:
14501:
14487:
14463:
14448:
14423:
14409:978-8185931869
14408:
14384:
14382:
14379:
14378:
14377:
14372:
14367:
14362:
14357:
14350:
14347:
14334:
14331:
14328:
14325:
14322:
14302:
14278:
14275:
14272:
14269:
14264:
14260:
14251:
14248:
14245:
14242:
14239:
14236:
14233:
14228:
14224:
14220:
14217:
14214:
14211:
14208:
14207:
14204:
14201:
14198:
14195:
14192:
14186:
14183:
14177:
14174:
14171:
14168:
14165:
14164:
14140:
14135:
14131:
14127:
14122:
14118:
14114:
14109:
14105:
14100:
14096:
14092:
14087:
14083:
14076:
14073:
14070:
14065:
14061:
14057:
14052:
14048:
14044:
14041:
14038:
14033:
14029:
14025:
14020:
14016:
14012:
14009:
14006:
14003:
14002:
13998:
13994:
13991:
13986:
13983:
13978:
13975:
13972:
13969:
13966:
13961:
13958:
13953:
13950:
13947:
13944:
13941:
13938:
13937:
13915:
13902:
13901:
13884:
13881:
13875:
13872:
13869:
13866:
13861:
13854:
13842:
13831:
13808:
13805:
13799:
13796:
13793:
13790:
13781:for any point
13770:
13767:
13762:
13759:
13756:
13753:
13750:
13747:
13744:
13739:
13732:
13707:
13687:
13665:
13651:
13648:
13635:
13632:
13629:
13624:
13594:
13591:
13568:
13565:
13562:
13559:
13555:
13551:
13548:
13542:
13539:
13516:
13513:
13510:
13507:
13504:
13499:
13494:
13490:
13469:
13466:
13463:
13443:
13423:
13420:
13417:
13414:
13394:
13374:
13354:
13351:
13348:
13345:
13342:
13339:
13336:
13333:
13325:
13320:
13317:
13295:
13292:
13289:
13286:
13283:
13280:
13277:
13274:
13271:
13268:
13265:
13262:
13259:
13256:
13236:
13216:
13213:
13210:
13205:
13195:
13189:
13186:
13164:
13140:
13137:
13130:
13124:
13121:
13116:
13110:
13107:
13081:
13061:
13057:
13053:
13050:
13047:
13044:
13041:
13038:
13035:
13030:
13027:
13022:
13019:
13016:
13013:
13007:
13004:
12981:
12977:
12973:
12970:
12967:
12961:
12929:
12925:
12921:
12918:
12915:
12909:
12905:
12893:
12888:
12885:
12861:
12857:
12834:
12830:
12826:
12821:
12817:
12795:
12791:
12788:
12785:
12780:
12776:
12772:
12769:
12766:
12762:
12758:
12755:
12752:
12749:
12746:
12743:
12740:
12718:
12715:
12694:
12691:
12688:
12686:
12683:
12680:
12672:
12670:
12667:
12665:
12662:
12659:
12651:
12649:
12646:
12644:
12641:
12638:
12633:
12623:
12621:
12618:
12616:
12613:
12612:
12609:
12606:
12603:
12600:
12597:
12594:
12591:
12588:
12585:
12582:
12580:
12577:
12574:
12571:
12568:
12565:
12563:
12560:
12558:
12555:
12552:
12547:
12542:
12540:
12537:
12535:
12532:
12529:
12526:
12523:
12520:
12517:
12514:
12506:
12504:
12501:
12499:
12496:
12495:
12475:
12472:
12469:
12461:
12458:
12455:
12452:
12447:
12425:
12405:
12385:
12382:
12379:
12359:
12333:
12330:
12327:
12324:
12321:
12317:
12296:
12293:
12290:
12287:
12284:
12281:
12261:
12258:
12255:
12252:
12249:
12246:
12243:
12238:
12235:
12232:
12229:
12226:
12222:
12218:
12215:
12212:
12209:
12206:
12203:
12200:
12197:
12194:
12191:
12188:
12185:
12182:
12161:
12157:
12154:
12134:
12114:
12110:
12105:
12101:
12098:
12095:
12092:
12089:
12086:
12083:
12080:
12077:
12057:
12054:
12051:
12048:
12045:
12042:
12039:
12036:
12033:
12030:
12027:
12024:
12021:
12018:
12015:
12012:
12009:
12006:
12003:
12000:
11997:
11994:
11991:
11971:
11968:
11965:
11962:
11959:
11956:
11953:
11948:
11945:
11940:
11937:
11934:
11931:
11928:
11925:
11922:
11919:
11916:
11913:
11910:
11907:
11904:
11901:
11898:
11895:
11892:
11889:
11886:
11883:
11880:
11877:
11855:
11850:
11845:
11842:
11839:
11836:
11833:
11828:
11825:
11822:
11819:
11816:
11812:
11791:
11769:
11766:
11763:
11760:
11757:
11753:
11732:
11729:
11726:
11723:
11720:
11708:
11705:
11692:
11689:
11686:
11683:
11680:
11677:
11657:
11654:
11651:
11648:
11645:
11642:
11639:
11636:
11633:
11630:
11627:
11622:
11617:
11614:
11611:
11608:
11605:
11602:
11599:
11596:
11593:
11585:
11582:
11562:
11559:
11556:
11553:
11550:
11547:
11544:
11541:
11516:
11513:
11510:
11507:
11504:
11480:
11477:
11474:
11471:
11468:
11465:
11460:
11456:
11447:
11444:
11441:
11438:
11435:
11432:
11429:
11426:
11421:
11417:
11408:
11405:
11402:
11399:
11396:
11393:
11390:
11387:
11382:
11378:
11372:
11368:
11364:
11361:
11358:
11353:
11349:
11343:
11339:
11335:
11332:
11330:
11328:
11325:
11322:
11319:
11316:
11313:
11310:
11305:
11301:
11292:
11289:
11286:
11283:
11280:
11277:
11274:
11271:
11268:
11265:
11262:
11257:
11253:
11244:
11241:
11238:
11235:
11232:
11229:
11226:
11223:
11218:
11214:
11210:
11207:
11204:
11199:
11195:
11191:
11188:
11185:
11180:
11176:
11172:
11169:
11166:
11161:
11157:
11153:
11150:
11148:
11146:
11143:
11140:
11137:
11134:
11131:
11126:
11122:
11118:
11115:
11112:
11109:
11106:
11101:
11097:
11093:
11090:
11088:
11086:
11083:
11080:
11077:
11074:
11069:
11065:
11059:
11055:
11051:
11050:
11028:
11024:
11018:
11014:
10993:
10988:
10984:
10978:
10974:
10970:
10965:
10961:
10957:
10952:
10948:
10944:
10941:
10938:
10933:
10929:
10925:
10920:
10916:
10912:
10909:
10906:
10901:
10897:
10893:
10888:
10884:
10880:
10860:
10857:
10854:
10849:
10823:
10820:
10817:
10814:
10811:
10789:
10786:
10781:
10775:
10769:
10766:
10761:
10756:
10753:
10750:
10747:
10744:
10741:
10738:
10735:
10732:
10705:
10701:
10697:
10692:
10688:
10666:
10662:
10659:
10656:
10651:
10647:
10643:
10640:
10637:
10633:
10629:
10626:
10623:
10620:
10617:
10614:
10611:
10591:
10588:
10585:
10582:
10579:
10575:
10570:
10565:
10561:
10557:
10553:
10550:
10530:
10527:
10524:
10521:
10518:
10515:
10512:
10509:
10484:
10464:
10444:
10441:
10438:
10433:
10406:
10382:
10379:
10376:
10373:
10369:
10348:
10345:
10342:
10339:
10336:
10316:
10292:
10283:hence the map
10272:
10269:
10266:
10263:
10260:
10257:
10254:
10246:
10243:
10240:
10237:
10234:
10231:
10228:
10225:
10222:
10219:
10216:
10213:
10210:
10207:
10204:
10201:
10198:
10195:
10192:
10189:
10169:
10166:
10163:
10160:
10157:
10154:
10151:
10148:
10128:
10116:
10113:
10100:
10096:
10093:
10070:
10067:
10064:
10052:
10049:
10044:
10038:
10035:
10032:
10029:
10026:
10023:
10020:
10017:
10012:
10008:
9986:
9982:
9978:
9974:
9970:
9966:
9961:
9957:
9934:
9930:
9909:
9889:
9886:
9883:
9880:
9877:
9874:
9871:
9868:
9865:
9862:
9859:
9856:
9853:
9850:
9847:
9824:
9821:
9818:
9809:
9804:
9801:
9798:
9777:
9773:
9770:
9767:
9764:
9761:
9758:
9755:
9731:
9728:
9723:
9715:
9711:
9707:
9704:
9701:
9700:
9695:
9691:
9687:
9684:
9683:
9681:
9676:
9671:
9663:
9659:
9655:
9654:
9649:
9645:
9641:
9640:
9638:
9633:
9628:
9624:
9620:
9617:
9612:
9604:
9600:
9596:
9593:
9590:
9589:
9584:
9580:
9576:
9573:
9572:
9570:
9565:
9560:
9554:
9551:
9550:
9547:
9544:
9543:
9541:
9536:
9535:
9530:
9526:
9522:
9519:
9516:
9511:
9505:
9502:
9499:
9498:
9495:
9492:
9491:
9489:
9484:
9479:
9471:
9467:
9463:
9462:
9457:
9453:
9449:
9448:
9446:
9441:
9438:
9435:
9430:
9424:
9421:
9418:
9417:
9414:
9411:
9410:
9408:
9403:
9398:
9392:
9389:
9388:
9385:
9382:
9381:
9379:
9374:
9373:
9353:
9350:
9347:
9321:
9313:
9309:
9305:
9302:
9299:
9298:
9293:
9289:
9285:
9282:
9281:
9279:
9274:
9269:
9261:
9257:
9253:
9252:
9247:
9243:
9239:
9238:
9236:
9231:
9228:
9225:
9220:
9214:
9211:
9208:
9207:
9204:
9201:
9200:
9198:
9193:
9188:
9182:
9179:
9178:
9175:
9172:
9171:
9169:
9164:
9161:
9158:
9157:
9137:
9134:
9131:
9109:
9103:
9100:
9098:
9095:
9092:
9091:
9088:
9085:
9083:
9080:
9079:
9077:
9072:
9069:
9047:
9043:
9037:
9033:
9029:
9024:
9020:
9014:
9010:
9006:
9001:
8997:
8993:
8990:
8985:
8981:
8977:
8974:
8953:
8930:
8926:
8922:
8917:
8913:
8892:
8888:
8884:
8881:
8878:
8873:
8863:
8860:
8839:
8835:
8832:
8810:
8804:
8801:
8799:
8796:
8795:
8793:
8769:
8755:
8752:
8738:
8734:
8731:
8728:
8725:
8722:
8719:
8716:
8696:
8676:
8656:
8652:
8648:
8645:
8642:
8637:
8633:
8629:
8626:
8621:
8616:
8610:
8604:
8599:
8595:
8591:
8586:
8582:
8560:
8556:
8553:
8550:
8547:
8544:
8541:
8538:
8513:
8493:
8473:
8470:
8467:
8464:
8461:
8438:
8434:
8430:
8427:
8424:
8406:
8403:
8390:
8387:
8384:
8381:
8378:
8367:
8366:
8355:
8352:
8349:
8346:
8343:
8340:
8337:
8334:
8331:
8328:
8325:
8322:
8319:
8316:
8313:
8310:
8307:
8304:
8301:
8298:
8295:
8292:
8282:
8271:
8268:
8265:
8262:
8259:
8256:
8253:
8250:
8247:
8244:
8241:
8238:
8235:
8206:
8202:
8199:
8196:
8193:
8190:
8187:
8167:
8164:
8161:
8141:
8138:
8135:
8130:
8126:
8114:
8113:
8102:
8099:
8096:
8093:
8090:
8070:
8067:
8064:
8061:
8058:
8055:
8052:
8049:
8046:
8043:
8040:
8037:
8034:
8031:
8028:
8018:
8006:
8002:
7999:
7996:
7993:
7990:
7987:
7984:
7960:
7956:
7953:
7950:
7947:
7944:
7941:
7920:
7898:
7877:
7874:
7871:
7849:
7845:
7823:
7804:
7801:
7787:
7784:
7781:
7778:
7775:
7772:
7769:
7766:
7763:
7760:
7757:
7754:
7751:
7748:
7745:
7742:
7739:
7718:
7714:
7711:
7708:
7705:
7685:
7682:
7679:
7658:
7650:
7647:
7644:
7639:
7636:
7632:
7628:
7625:
7622:
7619:
7616:
7613:
7610:
7607:
7599:
7598:
7595:
7592:
7589:
7586:
7583:
7580:
7577:
7574:
7571:
7568:
7565:
7562:
7559:
7556:
7553:
7550:
7547:
7539:
7536:
7535:
7532:
7528:
7524:
7521:
7518:
7515:
7510:
7507:
7503:
7499:
7496:
7492:
7488:
7485:
7482:
7479:
7474:
7450:
7428:
7425:
7422:
7414:
7411:
7408:
7405:
7402:
7399:
7396:
7393:
7388:
7384:
7363:
7360:
7355:
7350:
7344:
7338:
7335:
7332:
7327:
7323:
7319:
7316:
7313:
7308:
7304:
7300:
7297:
7294:
7291:
7269:
7264:
7259:
7256:
7253:
7250:
7247:
7244:
7224:
7212:
7209:
7194:
7188:
7185:
7181:
7178:
7175:
7171:
7167:
7164:
7160:
7157:
7154:
7150:
7146:
7143:
7139:
7136:
7133:
7129:
7125:
7122:
7121:
7116:
7113:
7110:
7106:
7102:
7100:
7097:
7093:
7090:
7087:
7083:
7079:
7076:
7072:
7069:
7066:
7062:
7058:
7055:
7054:
7049:
7046:
7043:
7039:
7035:
7031:
7028:
7025:
7021:
7017:
7015:
7012:
7008:
7005:
7002:
6998:
6994:
6991:
6990:
6985:
6982:
6979:
6975:
6971:
6967:
6964:
6961:
6957:
6953:
6949:
6946:
6943:
6939:
6935:
6933:
6930:
6929:
6927:
6922:
6917:
6913:
6892:
6870:
6866:
6843:
6838:
6833:
6830:
6808:
6803:
6779:
6773:
6770:
6768:
6765:
6761:
6758:
6755:
6751:
6747:
6743:
6740:
6737:
6733:
6729:
6728:
6725:
6722:
6720:
6717:
6713:
6710:
6707:
6703:
6699:
6695:
6692:
6689:
6685:
6681:
6680:
6678:
6673:
6670:
6658:
6655:
6642:
6639:
6636:
6616:
6613:
6610:
6607:
6604:
6584:
6581:
6578:
6575:
6572:
6569:
6566:
6563:
6560:
6557:
6554:
6551:
6548:
6545:
6542:
6539:
6536:
6533:
6530:
6527:
6524:
6521:
6518:
6515:
6512:
6509:
6506:
6503:
6500:
6497:
6494:
6491:
6488:
6485:
6482:
6479:
6476:
6471:
6467:
6446:
6443:
6440:
6437:
6434:
6431:
6428:
6425:
6422:
6419:
6399:
6379:
6357:
6353:
6331:
6310:
6307:
6304:
6299:
6295:
6274:
6270:
6266:
6263:
6260:
6255:
6251:
6247:
6244:
6224:
6204:
6200:
6196:
6193:
6190:
6185:
6181:
6156:
6152:
6148:
6145:
6142:
6137:
6133:
6129:
6126:
6121:
6116:
6110:
6104:
6099:
6095:
6091:
6086:
6082:
6061:
6058:
6053:
6048:
6042:
6036:
6031:
6025:
6019:
6015:
6011:
6008:
5993:
5990:
5977:
5957:
5954:
5949:
5944:
5938:
5932:
5927:
5923:
5919:
5916:
5913:
5908:
5904:
5883:
5863:
5843:
5840:
5837:
5832:
5828:
5807:
5802:
5797:
5791:
5785:
5782:
5779:
5774:
5770:
5766:
5763:
5760:
5755:
5748:
5745:
5737:
5731:
5726:
5722:
5718:
5715:
5712:
5709:
5704:
5700:
5696:
5691:
5687:
5666:
5663:
5660:
5655:
5648:
5645:
5637:
5631:
5625:
5622:
5616:
5611:
5607:
5594:
5591:
5578:
5575:
5570:
5563:
5560:
5552:
5546:
5540:
5537:
5531:
5526:
5522:
5518:
5515:
5510:
5505:
5499:
5493:
5490:
5487:
5482:
5478:
5474:
5471:
5468:
5465:
5462:
5459:
5454:
5447:
5444:
5436:
5430:
5425:
5421:
5417:
5414:
5411:
5408:
5403:
5399:
5395:
5390:
5386:
5365:
5360:
5355:
5349:
5343:
5340:
5337:
5332:
5328:
5324:
5321:
5318:
5313:
5306:
5303:
5295:
5289:
5284:
5280:
5276:
5273:
5270:
5267:
5262:
5258:
5254:
5249:
5245:
5224:
5221:
5215:
5212:
5206:
5203:
5183:
5180:
5177:
5174:
5171:
5151:
5148:
5145:
5140:
5136:
5131:
5126:
5122:
5116:
5113:
5107:
5104:
5084:
5064:
5061:
5058:
5055:
5052:
5049:
5046:
5043:
5040:
5037:
5034:
5031:
5028:
5025:
5022:
5019:
5016:
5013:
5010:
5007:
5004:
5001:
4981:
4978:
4975:
4970:
4966:
4942:
4939:
4933:
4929:
4925:
4919:
4916:
4893:
4873:
4861:
4858:
4844:
4840:
4837:
4834:
4831:
4828:
4825:
4805:
4802:
4799:
4796:
4793:
4790:
4787:
4784:
4781:
4778:
4775:
4745:
4740:
4735:
4730:
4727:
4722:
4717:
4712:
4707:
4702:
4699:
4679:
4667:
4664:
4647:
4623:
4620:
4615:
4608:
4605:
4597:
4591:
4586:
4582:
4578:
4575:
4572:
4569:
4564:
4560:
4556:
4551:
4547:
4523:
4520:
4514:
4511:
4491:
4488:
4485:
4480:
4476:
4472:
4469:
4466:
4463:
4443:
4440:
4437:
4434:
4431:
4428:
4425:
4422:
4419:
4416:
4413:
4410:
4407:
4404:
4401:
4398:
4395:
4392:
4389:
4386:
4383:
4380:
4377:
4355:
4350:
4345:
4339:
4336:
4330:
4327:
4324:
4321:
4316:
4312:
4308:
4305:
4285:
4282:
4279:
4274:
4270:
4249:
4244:
4239:
4234:
4228:
4225:
4219:
4216:
4213:
4210:
4207:
4202:
4195:
4192:
4184:
4178:
4173:
4169:
4145:
4142:
4119:
4116:
4113:
4108:
4104:
4083:
4080:
4075:
4068:
4065:
4057:
4051:
4046:
4042:
4038:
4035:
4032:
4029:
4024:
4020:
4016:
4011:
4007:
3994:
3991:
3976:
3971:
3966:
3960:
3957:
3934:
3929:
3925:
3904:
3901:
3898:
3893:
3889:
3861:
3858:
3852:
3849:
3844:
3840:
3819:
3816:
3813:
3790:
3778:
3775:
3756:
3752:
3748:
3745:
3740:
3736:
3732:
3729:
3724:
3720:
3716:
3696:
3693:
3690:
3684:
3679:
3675:
3671:
3667:
3664:
3661:
3658:
3652:
3647:
3643:
3640:
3637:
3634:
3631:
3628:
3622:
3617:
3613:
3610:
3605:
3599:
3571:
3565:
3542:
3522:
3519:
3516:
3513:
3507:
3502:
3498:
3478:
3475:
3472:
3466:
3461:
3457:
3453:
3449:
3446:
3443:
3440:
3434:
3429:
3425:
3403:
3400:
3395:
3381:
3378:
3365:
3362:
3358:
3354:
3345:isomorphic to
3332:
3329:
3325:
3320:
3316:
3312:
3307:
3303:
3300:
3295:
3291:
3270:
3267:
3264:
3261:
3241:
3238:
3235:
3232:
3227:
3223:
3200:
3197:
3192:
3187:
3184:
3172:
3169:
3156:
3153:
3148:
3145:
3142:
3139:
3135:
3131:
3128:
3107:
3104:
3100:
3097:
3094:
3091:
3070:
3067:
3063:
3060:
3040:
3018:
3014:
3010:
3007:
3004:
3001:
2981:
2978:
2975:
2972:
2965:
2961:
2956:
2935:
2932:
2927:
2923:
2909:
2906:
2892:
2889:
2885:
2882:
2879:
2876:
2855:
2851:
2848:
2845:
2842:
2817:
2813:
2810:
2806:
2803:
2800:
2794:
2783:
2780:
2776:
2773:
2769:
2766:
2763:
2755:
2750:
2746:
2725:
2721:
2718:
2714:
2711:
2708:
2702:
2691:
2688:
2684:
2681:
2677:
2674:
2671:
2663:
2658:
2654:
2632:
2629:
2625:
2622:
2619:
2614:
2610:
2589:
2568:
2565:
2561:
2558:
2555:
2552:
2531:
2528:
2524:
2521:
2518:
2515:
2494:
2491:
2487:
2484:
2475:of dimensions
2463:
2460:
2455:
2450:
2447:
2443:
2439:
2436:
2415:
2411:
2407:
2404:
2401:
2389:
2386:
2377:
2374:
2359:
2354:
2351:
2331:
2328:
2325:
2298:
2292:
2289:
2287:
2284:
2282:
2277:
2272:
2269:
2266:
2264:
2261:
2258:
2255:
2252:
2251:
2248:
2245:
2243:
2240:
2238:
2235:
2232:
2229:
2227:
2224:
2221:
2218:
2217:
2215:
2191:
2185:
2182:
2180:
2177:
2173:
2170:
2167:
2163:
2159:
2155:
2152:
2149:
2145:
2141:
2140:
2137:
2134:
2132:
2129:
2125:
2122:
2119:
2115:
2111:
2107:
2104:
2101:
2097:
2093:
2092:
2090:
2076:
2073:
2056:
2053:
2050:
2047:
2044:
2041:
2033:
2030:
2027:
2024:
2022:
2020:
2017:
2012:
2009:
2004:
2001:
1998:
1995:
1992:
1989:
1987:
1985:
1982:
1977:
1974:
1967:
1963:
1957:
1953:
1949:
1946:
1943:
1940:
1937:
1932:
1928:
1924:
1921:
1918:
1915:
1913:
1911:
1908:
1905:
1904:
1882:
1874:
1870:
1866:
1862:
1859:
1854:
1853:
1848:
1844:
1840:
1838:
1835:
1834:
1832:
1810:
1790:
1787:
1784:
1781:
1778:
1770:
1750:
1730:
1727:
1724:
1721:
1718:
1710:
1690:
1687:
1684:
1678:
1673:
1669:
1666:
1663:
1660:
1638:
1634:
1613:
1608:
1604:
1600:
1597:
1594:
1574:
1554:
1534:
1530:
1526:
1523:
1520:
1508:
1505:
1490:
1484:
1481:
1479:
1476:
1474:
1471:
1468:
1466:
1463:
1462:
1459:
1456:
1453:
1451:
1448:
1446:
1443:
1441:
1438:
1437:
1435:
1430:
1427:
1405:
1397:
1394:
1391:
1387:
1383:
1379:
1376:
1373:
1369:
1365:
1361:
1358:
1355:
1351:
1347:
1343:
1340:
1337:
1333:
1329:
1328:
1323:
1320:
1317:
1313:
1309:
1305:
1302:
1299:
1295:
1291:
1287:
1284:
1281:
1277:
1273:
1269:
1266:
1263:
1259:
1255:
1254:
1252:
1247:
1244:
1232:
1229:
1215:
1192:
1187:
1165:
1139:
1131:
1128:
1123:
1122:
1119:
1116:
1115:
1113:
1108:
1105:
1085:
1063:
1060:
1038:
1035:
1032:
1029:
1026:
1023:
1020:
1014:
1009:
1005:
1002:
999:
996:
976:
973:
970:
967:
964:
961:
955:
950:
946:
943:
940:
937:
915:
912:
907:
902:
898:
892:
887:
882:
879:
859:
855:
851:
848:
845:
823:
819:
813:
810:
806:
800:
796:
792:
787:
783:
760:
752:
749:
746:
743:
739:
735:
733:
730:
726:
723:
720:
716:
712:
711:
708:
705:
702:
699:
698:
693:
690:
687:
684:
680:
676:
674:
671:
667:
664:
661:
657:
653:
652:
650:
645:
642:
622:
600:
596:
592:
589:
586:
581:
577:
556:
534:
531:
527:
523:
520:
517:
512:
508:
487:
467:
464:
461:
458:
442:
439:
426:
422:
418:
398:
395:
392:
383:and a lattice
372:
349:
346:
343:
339:
333:
309:
304:
301:
281:
276:
272:
268:
263:
241:
223:
220:
212:Chow's theorem
171:, and are the
149:period lattice
128:
124:
120:
110:quotient group
36:
32:
9:
6:
4:
3:
2:
14837:
14826:
14823:
14821:
14818:
14816:
14813:
14812:
14810:
14801:
14798:
14797:
14786:
14782:
14778:
14774:
14769:
14764:
14760:
14756:
14751:
14747:
14743:
14739:
14735:
14731:
14727:
14722:
14717:
14713:
14709:
14704:
14698:
14693:
14689:
14688:
14682:
14659:
14648:
14631:
14627:
14623:
14619:
14614:
14609:
14605:
14601:
14596:
14595:
14586:(2): 407–427.
14585:
14581:
14577:
14572:
14567:
14563:
14559:
14555:
14551:
14547:
14543:
14538:
14537:
14528:
14524:
14520:
14514:
14510:
14505:
14504:
14497:
14491:
14480:
14473:
14467:
14459:
14455:
14451:
14445:
14441:
14440:
14432:
14430:
14428:
14419:
14415:
14411:
14405:
14401:
14400:
14392:
14390:
14385:
14376:
14373:
14371:
14368:
14366:
14363:
14361:
14358:
14356:
14353:
14352:
14346:
14329:
14326:
14323:
14300:
14270:
14262:
14258:
14249:
14246:
14240:
14237:
14234:
14226:
14222:
14218:
14215:
14209:
14199:
14193:
14175:
14169:
14166:
14133:
14129:
14120:
14116:
14112:
14098:
14094:
14085:
14081:
14074:
14063:
14059:
14055:
14050:
14046:
14039:
14031:
14027:
14023:
14018:
14014:
14004:
13976:
13973:
13967:
13951:
13948:
13942:
13939:
13879:
13873:
13867:
13843:
13829:
13803:
13797:
13791:
13768:
13765:
13754:
13745:
13742:
13721:
13720:
13719:
13705:
13685:
13647:
13630:
13622:
13589:
13563:
13557:
13553:
13549:
13546:
13537:
13514:
13511:
13505:
13497:
13492:
13488:
13467:
13464:
13461:
13441:
13418:
13412:
13392:
13372:
13346:
13340:
13337:
13287:
13284:
13281:
13275:
13272:
13269:
13263:
13260:
13254:
13234:
13211:
13203:
13193:
13184:
13162:
13128:
13114:
13105:
13095:
13051:
13042:
13039:
13033:
13020:
13017:
13011:
12971:
12968:
12943:
12919:
12916:
12891:
12859:
12832:
12824:
12819:
12815:
12793:
12786:
12778:
12774:
12770:
12767:
12764:
12760:
12756:
12753:
12750:
12744:
12738:
12728:
12724:
12714:
12712:
12692:
12681:
12660:
12639:
12631:
12614:
12586:
12575:
12569:
12566:
12527:
12521:
12518:
12497:
12470:
12423:
12403:
12383:
12377:
12357:
12349:
12328:
12325:
12322:
12315:
12291:
12288:
12285:
12279:
12256:
12250:
12247:
12244:
12233:
12230:
12227:
12220:
12216:
12213:
12210:
12207:
12201:
12195:
12192:
12189:
12183:
12180:
12155:
12152:
12108:
12099:
12096:
12093:
12087:
12084:
12081:
12075:
12052:
12049:
12046:
12040:
12034:
12031:
12028:
12025:
12022:
12016:
12013:
12007:
12004:
12001:
11998:
11995:
11989:
11963:
11960:
11957:
11951:
11946:
11943:
11938:
11932:
11929:
11926:
11920:
11917:
11911:
11908:
11902:
11896:
11893:
11887:
11884:
11881:
11875:
11853:
11840:
11837:
11831:
11823:
11820:
11817:
11810:
11764:
11761:
11758:
11751:
11727:
11724:
11721:
11704:
11687:
11684:
11681:
11675:
11655:
11646:
11640:
11637:
11606:
11600:
11597:
11580:
11554:
11548:
11545:
11511:
11505:
11502:
11472:
11469:
11466:
11458:
11454:
11445:
11442:
11439:
11433:
11430:
11427:
11419:
11415:
11406:
11403:
11397:
11394:
11388:
11380:
11376:
11370:
11366:
11359:
11351:
11347:
11341:
11337:
11333:
11331:
11317:
11314:
11311:
11303:
11299:
11290:
11287:
11281:
11278:
11269:
11266:
11263:
11255:
11251:
11242:
11239:
11233:
11230:
11224:
11216:
11212:
11205:
11197:
11193:
11186:
11178:
11174:
11167:
11159:
11155:
11151:
11149:
11138:
11135:
11132:
11124:
11120:
11113:
11110:
11107:
11099:
11095:
11091:
11089:
11081:
11078:
11075:
11067:
11063:
11057:
11053:
11026:
11022:
11016:
11012:
10986:
10982:
10976:
10972:
10968:
10963:
10959:
10955:
10950:
10946:
10939:
10931:
10927:
10923:
10918:
10914:
10907:
10899:
10895:
10891:
10886:
10882:
10837:
10818:
10815:
10812:
10787:
10784:
10773:
10767:
10764:
10754:
10745:
10739:
10736:
10703:
10695:
10690:
10686:
10664:
10657:
10649:
10645:
10641:
10638:
10635:
10631:
10627:
10624:
10621:
10615:
10609:
10586:
10580:
10577:
10568:
10522:
10516:
10513:
10482:
10462:
10439:
10431:
10396:
10380:
10374:
10371:
10343:
10337:
10334:
10314:
10306:
10290:
10264:
10261:
10258:
10252:
10244:
10241:
10235:
10232:
10226:
10220:
10214:
10208:
10205:
10199:
10196:
10193:
10187:
10164:
10158:
10149:
10146:
10126:
10112:
10094:
10091:
10065:
10047:
10042:
10036:
10033:
10030:
10024:
10021:
10018:
10010:
10006:
9972:
9964:
9959:
9955:
9932:
9928:
9907:
9884:
9881:
9878:
9875:
9872:
9866:
9863:
9857:
9854:
9851:
9845:
9819:
9807:
9802:
9799:
9796:
9771:
9762:
9753:
9729:
9726:
9721:
9713:
9709:
9705:
9702:
9693:
9689:
9685:
9679:
9674:
9669:
9661:
9657:
9647:
9643:
9636:
9626:
9622:
9618:
9615:
9610:
9602:
9598:
9594:
9591:
9582:
9578:
9574:
9568:
9563:
9558:
9552:
9545:
9539:
9528:
9524:
9520:
9517:
9514:
9509:
9503:
9500:
9493:
9487:
9482:
9477:
9469:
9465:
9455:
9451:
9444:
9436:
9433:
9428:
9422:
9419:
9412:
9406:
9401:
9396:
9390:
9383:
9377:
9351:
9348:
9345:
9319:
9311:
9307:
9303:
9300:
9291:
9287:
9283:
9277:
9272:
9267:
9259:
9255:
9245:
9241:
9234:
9229:
9226:
9218:
9212:
9209:
9202:
9196:
9191:
9186:
9180:
9173:
9167:
9162:
9159:
9135:
9132:
9129:
9107:
9101:
9096:
9093:
9086:
9081:
9075:
9070:
9067:
9045:
9041:
9035:
9031:
9027:
9022:
9018:
9012:
9008:
9004:
8999:
8995:
8991:
8988:
8983:
8979:
8975:
8972:
8928:
8924:
8920:
8915:
8911:
8882:
8871:
8861:
8858:
8833:
8830:
8808:
8802:
8797:
8791:
8751:
8732:
8723:
8714:
8694:
8674:
8646:
8643:
8635:
8631:
8619:
8614:
8597:
8593:
8589:
8584:
8580:
8554:
8545:
8536:
8511:
8491:
8468:
8462:
8459:
8452:
8432:
8428:
8425:
8422:
8412:
8402:
8388:
8385:
8382:
8379:
8376:
8350:
8347:
8344:
8338:
8335:
8332:
8326:
8323:
8320:
8317:
8311:
8308:
8302:
8299:
8296:
8290:
8283:
8266:
8263:
8260:
8254:
8251:
8245:
8242:
8239:
8233:
8221:
8220:
8219:
8197:
8194:
8191:
8188:
8185:
8165:
8159:
8136:
8128:
8124:
8100:
8097:
8094:
8091:
8088:
8065:
8062:
8059:
8053:
8050:
8044:
8041:
8038:
8035:
8032:
8026:
8019:
8000:
7991:
7982:
7975:
7974:
7973:
7951:
7948:
7945:
7942:
7939:
7875:
7869:
7847:
7843:
7810:
7800:
7782:
7776:
7770:
7767:
7764:
7758:
7755:
7749:
7746:
7743:
7737:
7709:
7706:
7703:
7683:
7680:
7677:
7656:
7645:
7637:
7634:
7630:
7626:
7620:
7614:
7611:
7608:
7602:for all
7590:
7584:
7578:
7575:
7572:
7566:
7563:
7557:
7554:
7551:
7545:
7537:
7530:
7516:
7508:
7505:
7501:
7497:
7494:
7490:
7486:
7480:
7423:
7412:
7406:
7400:
7394:
7386:
7382:
7353:
7348:
7336:
7333:
7325:
7321:
7317:
7306:
7302:
7298:
7292:
7267:
7254:
7251:
7245:
7242:
7222:
7208:
7192:
7186:
7179:
7176:
7173:
7169:
7165:
7158:
7155:
7152:
7148:
7144:
7137:
7134:
7131:
7127:
7123:
7114:
7111:
7108:
7104:
7098:
7091:
7088:
7085:
7081:
7077:
7070:
7067:
7064:
7060:
7056:
7047:
7044:
7041:
7037:
7029:
7026:
7023:
7019:
7013:
7006:
7003:
7000:
6996:
6992:
6983:
6980:
6977:
6973:
6965:
6962:
6959:
6955:
6947:
6944:
6941:
6937:
6931:
6925:
6920:
6915:
6911:
6868:
6864:
6841:
6831:
6806:
6777:
6771:
6766:
6759:
6756:
6753:
6749:
6741:
6738:
6735:
6731:
6723:
6718:
6711:
6708:
6705:
6701:
6693:
6690:
6687:
6683:
6676:
6671:
6654:
6640:
6637:
6634:
6611:
6608:
6605:
6602:
6579:
6576:
6573:
6567:
6564:
6558:
6555:
6552:
6549:
6546:
6540:
6537:
6531:
6528:
6525:
6519:
6516:
6510:
6507:
6504:
6501:
6498:
6492:
6489:
6483:
6480:
6477:
6469:
6465:
6441:
6438:
6435:
6432:
6426:
6423:
6420:
6417:
6397:
6355:
6351:
6305:
6297:
6293:
6285:. Therefore,
6264:
6253:
6249:
6245:
6242:
6194:
6191:
6183:
6179:
6170:
6167:is the first
6146:
6143:
6135:
6131:
6119:
6114:
6097:
6093:
6089:
6084:
6080:
6059:
6051:
6046:
6029:
6006:
5999:
5989:
5975:
5947:
5942:
5925:
5921:
5917:
5906:
5902:
5881:
5838:
5830:
5826:
5800:
5795:
5783:
5780:
5772:
5768:
5764:
5753:
5743:
5724:
5720:
5716:
5710:
5702:
5698:
5689:
5685:
5664:
5661:
5653:
5643:
5629:
5620:
5609:
5605:
5590:
5568:
5558:
5544:
5535:
5524:
5520:
5508:
5503:
5491:
5488:
5480:
5476:
5469:
5466:
5452:
5442:
5423:
5419:
5415:
5409:
5401:
5397:
5388:
5384:
5358:
5353:
5341:
5338:
5330:
5326:
5311:
5301:
5282:
5278:
5274:
5268:
5260:
5256:
5247:
5243:
5222:
5210:
5204:
5201:
5181:
5175:
5172:
5169:
5146:
5138:
5134:
5129:
5120:
5111:
5105:
5102:
5082:
5059:
5056:
5050:
5047:
5044:
5038:
5035:
5032:
5029:
5026:
5020:
5014:
5011:
5008:
5002:
4999:
4976:
4968:
4964:
4937:
4923:
4914:
4891:
4871:
4857:
4832:
4829:
4826:
4823:
4800:
4797:
4794:
4791:
4788:
4782:
4779:
4776:
4773:
4765:
4761:
4743:
4728:
4725:
4715:
4710:
4700:
4697:
4677:
4663:
4661:
4645:
4637:
4613:
4603:
4584:
4580:
4576:
4570:
4562:
4558:
4549:
4545:
4518:
4512:
4509:
4486:
4478:
4474:
4470:
4467:
4464:
4461:
4438:
4435:
4432:
4426:
4420:
4417:
4414:
4411:
4408:
4402:
4399:
4393:
4390:
4387:
4384:
4381:
4375:
4353:
4334:
4328:
4322:
4314:
4310:
4306:
4303:
4280:
4272:
4268:
4242:
4223:
4217:
4214:
4208:
4200:
4190:
4171:
4167:
4140:
4114:
4106:
4102:
4073:
4063:
4044:
4040:
4036:
4030:
4022:
4018:
4009:
4005:
3990:
3974:
3964:
3955:
3932:
3927:
3923:
3899:
3891:
3887:
3878:
3856:
3847:
3842:
3838:
3817:
3811:
3804:
3788:
3774:
3772:
3754:
3750:
3746:
3743:
3738:
3734:
3730:
3727:
3722:
3718:
3714:
3691:
3677:
3673:
3669:
3662:
3659:
3645:
3641:
3632:
3629:
3615:
3611:
3608:
3603:
3587:
3569:
3540:
3517:
3514:
3500:
3496:
3473:
3459:
3455:
3451:
3444:
3441:
3427:
3423:
3401:
3398:
3377:
3360:
3356:
3330:
3327:
3314:
3310:
3298:
3293:
3289:
3268:
3265:
3259:
3239:
3233:
3230:
3225:
3221:
3198:
3195:
3185:
3182:
3168:
3154:
3151:
3143:
3137:
3133:
3129:
3126:
3105:
3102:
3095:
3092:
3089:
3068:
3065:
3061:
3058:
3038:
3016:
3012:
3008:
3005:
2999:
2979:
2973:
2970:
2963:
2959:
2954:
2933:
2930:
2925:
2921:
2905:
2890:
2887:
2883:
2880:
2877:
2874:
2849:
2843:
2811:
2804:
2774:
2771:
2767:
2764:
2753:
2748:
2744:
2719:
2716:
2712:
2709:
2682:
2679:
2675:
2672:
2661:
2656:
2652:
2630:
2617:
2608:
2587:
2566:
2563:
2556:
2553:
2550:
2529:
2526:
2519:
2516:
2513:
2492:
2489:
2485:
2482:
2461:
2453:
2448:
2445:
2441:
2437:
2434:
2409:
2405:
2402:
2399:
2385:
2383:
2373:
2357:
2352:
2349:
2326:
2296:
2290:
2285:
2280:
2275:
2270:
2267:
2262:
2259:
2256:
2253:
2246:
2241:
2236:
2233:
2230:
2225:
2222:
2219:
2213:
2189:
2183:
2178:
2171:
2168:
2165:
2161:
2153:
2150:
2147:
2143:
2135:
2130:
2123:
2120:
2117:
2113:
2105:
2102:
2099:
2095:
2088:
2072:
2054:
2051:
2042:
2023:
2007:
2002:
1999:
1990:
1988:
1972:
1965:
1961:
1955:
1951:
1947:
1944:
1930:
1926:
1916:
1914:
1909:
1880:
1872:
1868:
1857:
1846:
1842:
1836:
1830:
1808:
1788:
1785:
1779:
1748:
1728:
1725:
1719:
1685:
1671:
1667:
1664:
1661:
1658:
1636:
1632:
1606:
1602:
1598:
1595:
1552:
1545:of dimension
1528:
1524:
1521:
1518:
1504:
1488:
1482:
1477:
1472:
1469:
1464:
1457:
1454:
1449:
1444:
1439:
1433:
1428:
1403:
1395:
1392:
1389:
1385:
1377:
1374:
1371:
1367:
1359:
1356:
1353:
1349:
1341:
1338:
1335:
1331:
1321:
1318:
1315:
1311:
1303:
1300:
1297:
1293:
1285:
1282:
1279:
1275:
1267:
1264:
1261:
1257:
1250:
1245:
1228:
1190:
1155:
1137:
1111:
1106:
1103:
1033:
1030:
1027:
1024:
1021:
1007:
1003:
1000:
997:
994:
971:
968:
965:
962:
948:
944:
941:
938:
913:
910:
896:
890:
880:
877:
853:
849:
846:
843:
821:
817:
811:
808:
804:
798:
794:
790:
785:
781:
758:
750:
747:
744:
741:
737:
731:
724:
721:
718:
714:
706:
700:
691:
688:
685:
682:
678:
672:
665:
662:
659:
655:
648:
643:
620:
598:
594:
590:
587:
584:
579:
575:
532:
529:
525:
521:
518:
515:
510:
506:
465:
462:
459:
456:
448:
438:
420:
416:
396:
393:
370:
361:
347:
344:
337:
302:
279:
274:
270:
266:
239:
232:
229:
219:
217:
213:
209:
205:
201:
197:
193:
189:
185:
181:
176:
174:
170:
166:
162:
158:
154:
150:
146:
142:
122:
118:
111:
107:
103:
98:
96:
92:
88:
84:
80:
76:
73:
69:
65:
61:
57:
54:
50:
49:complex torus
46:
29:
19:
14768:math/0601337
14758:
14754:
14711:
14707:
14685:
14603:
14599:
14583:
14579:
14549:
14545:
14509:Complex tori
14508:
14490:
14466:
14438:
14398:
13903:
13653:
13093:
12730:
12710:
12347:
12173:is given by
11710:
10835:
10118:
9148:. These are
8757:
8450:
8414:
8368:
8115:
7812:
7214:
6660:
5995:
5596:
4863:
4669:
4659:
4635:
3996:
3803:line bundles
3780:
3416:and the set
3383:
3174:
2911:
2391:
2379:
2078:
1585:of the form
1510:
1234:
446:
444:
362:
225:
207:
199:
183:
177:
156:
144:
105:
99:
94:
86:
82:
78:
71:
55:
48:
42:
18:Complex tori
14794:P-adic tori
14714:: 209–221.
14697:0803.1529v2
14552:: 293–299.
12940:of complex
12145:-action on
11868:defined as
8218:satisfying
6169:Chern class
1154:nonsingular
196:cubic curve
45:mathematics
14809:Categories
14381:References
12942:antilinear
12721:See also:
12125:where the
10455:of degree
10180:such that
8707:such that
8409:See also:
7807:See also:
7730:such that
4454:for every
3119:such that
222:Definition
14746:119599648
14721:1102.2312
14657:Λ
14613:0811.2746
14566:120799085
14458:851380558
14418:297809496
14330:χ
14271:λ
14250:π
14241:
14216:λ
14210:χ
14191:→
14185:^
14182:Λ
14176:×
14173:Λ
14167:χ
14108:¯
13993:→
13985:¯
13982:Ω
13977:×
13968:×
13960:¯
13957:Ω
13952:×
13883:^
13874:×
13807:^
13798:∈
13766:≅
13746:×
13593:^
13541:^
13512:≅
13498:∗
13465:∈
13335:Λ
13324:→
13319:¯
13316:Ω
13291:⟩
13288:⋅
13279:⟨
13273:π
13264:
13258:↦
13194:≅
13188:^
13139:^
13136:Λ
13123:¯
13120:Ω
13115:≅
13109:^
13080:Λ
13052:⊆
13049:⟩
13046:Λ
13037:⟨
13029:¯
13026:Ω
13021:∈
13006:^
13003:Λ
12908:¯
12887:¯
12884:Ω
12860:∗
12856:Λ
12833:∗
12829:Λ
12825:∈
12820:∗
12787:⋅
12779:∗
12768:π
12757:
12745:⋅
12739:χ
12690:→
12669:→
12648:→
12620:→
12608:↓
12602:↓
12596:↓
12584:→
12562:→
12554:Λ
12539:→
12516:Λ
12503:→
12460:→
12454:Λ
12381:→
12329:χ
12292:χ
12245:λ
12234:χ
12184:∘
12181:λ
12156:×
12133:Λ
12113:Λ
12100:×
12094:≅
12088:χ
12047:μ
12035:μ
12023:λ
12002:μ
11996:λ
11964:λ
11958:λ
11944:π
11933:λ
11918:π
11912:
11903:λ
11897:χ
11882:λ
11854:∗
11844:→
11838:×
11835:Λ
11824:χ
11802:as a map
11790:Λ
11765:χ
11728:χ
11688:χ
11653:→
11635:→
11629:Λ
11616:→
11595:Λ
11584:→
11543:Λ
11473:μ
11467:λ
11446:π
11434:μ
11428:λ
11407:π
11398:
11389:μ
11377:χ
11367:χ
11360:λ
11348:χ
11338:χ
11318:μ
11312:λ
11291:π
11282:
11270:μ
11264:λ
11243:π
11234:
11225:μ
11213:χ
11206:λ
11194:χ
11187:μ
11175:χ
11168:λ
11156:χ
11139:μ
11133:λ
11121:χ
11114:μ
11108:λ
11096:χ
11082:μ
11076:λ
11064:χ
11054:χ
11023:χ
11013:χ
10983:χ
10973:χ
10928:χ
10908:∗
10896:χ
10856:Λ
10813:χ
10755:≅
10734:Λ
10704:∗
10700:Λ
10696:∈
10691:∗
10658:⋅
10650:∗
10639:π
10628:
10616:⋅
10610:χ
10578:≅
10560:→
10552:→
10549:Λ
10511:Λ
10405:Λ
10378:→
10372:×
10305:character
10291:χ
10265:μ
10259:λ
10245:π
10236:
10227:μ
10221:χ
10215:λ
10209:χ
10200:μ
10194:λ
10188:χ
10156:→
10153:Λ
10147:χ
10095:∈
10066:τ
10051:¯
10037:⋅
9998:given by
9981:→
9973:×
9820:τ
9772:⊂
9766:Λ
9760:Λ
9710:τ
9703:−
9690:τ
9675:⋅
9658:τ
9644:τ
9623:τ
9599:τ
9592:−
9579:τ
9564:⋅
9525:τ
9518:−
9501:−
9483:⋅
9466:τ
9452:τ
9420:−
9402:⋅
9352:τ
9308:τ
9301:−
9288:τ
9256:τ
9242:τ
9230:⋅
9210:−
9163:⋅
9136:τ
9094:−
8880:Λ
8862:∈
8834:∈
8831:τ
8803:τ
8733:⊆
8727:Λ
8721:Λ
8628:→
8620:∗
8555:⊆
8549:Λ
8543:Λ
8437:Λ
8386:∈
8201:→
8195:×
8163:→
8098:∈
8001:⊆
7995:Λ
7989:Λ
7955:→
7949:×
7873:→
7777:θ
7765:λ
7750:λ
7738:θ
7713:→
7704:θ
7681:⊂
7635:−
7631:π
7627:×
7624:Λ
7621:∈
7609:λ
7585:θ
7573:λ
7558:λ
7546:θ
7538:θ
7523:→
7506:−
7502:π
7495:θ
7413:∈
7383:ϕ
7354:∗
7315:Λ
7299:∈
7268:∗
7258:→
7252:×
7249:Λ
7166:−
7145:−
7124:−
7078:−
7057:−
6993:−
6891:Λ
6832:⊂
6829:Λ
6669:Π
6638:∈
6615:Λ
6612:∈
6609:λ
6603:μ
6574:μ
6565:−
6559:μ
6547:λ
6538:−
6526:λ
6511:λ
6499:μ
6484:μ
6478:λ
6436:π
6427:
6378:Λ
6262:Λ
6223:Λ
6128:→
6120:∗
6057:→
6052:∗
6035:→
6018:→
6010:→
5996:From the
5948:∗
5915:Λ
5874:defining
5862:Λ
5827:π
5801:∗
5765:≅
5754:∗
5747:~
5699:π
5662:≅
5654:∗
5647:~
5624:~
5569:∗
5562:~
5539:~
5517:→
5509:∗
5470:
5464:→
5453:∗
5446:~
5398:π
5359:∗
5323:→
5312:∗
5305:~
5257:π
5220:→
5214:~
5202:π
5179:→
5135:π
5121:×
5115:~
5057:⋅
5030:⋅
5003:⋅
4965:π
4941:~
4932:→
4924:×
4918:~
4839:→
4836:Λ
4833:×
4798:⋅
4792:π
4783:
4744:∗
4734:→
4716:×
4614:∗
4607:~
4559:π
4522:~
4513:∈
4475:π
4471:∈
4418:⋅
4385:⋅
4354:∗
4344:→
4338:~
4329:×
4311:π
4269:π
4243:∗
4233:→
4227:~
4201:∗
4194:~
4144:~
4103:π
4074:∗
4067:~
4019:π
3965:≅
3959:~
3928:∗
3924:π
3888:π
3860:~
3851:→
3843:∗
3839:π
3815:→
3728:−
3639:∖
3609:≅
3584:, as the
3364:Λ
3353:Λ
3299:≅
3263:↦
3237:→
3196:≠
3186:∈
3171:Isogenies
3152:∘
3099:→
3031:Then, if
3003:↦
2977:→
2931:∈
2878:≤
2850:⊗
2809:Λ
2802:Λ
2782:→
2745:ρ
2690:→
2653:ρ
2628:Λ
2624:→
2621:Λ
2613:Λ
2560:→
2523:→
2459:Λ
2414:Λ
2234:−
2052:≠
2026:⇒
2011:¯
2003:−
1976:¯
1948:−
1861:¯
1786:≠
1726:≠
1662:∈
1573:Π
1533:Λ
1470:−
1426:Π
1386:λ
1368:λ
1350:λ
1332:λ
1312:λ
1294:λ
1276:λ
1258:λ
1243:Π
1164:Π
1130:¯
1127:Π
1118:Π
1084:Π
1062:¯
1059:Π
998:∈
939:∈
936:Π
901:Π
858:Λ
805:λ
795:∑
782:λ
738:λ
732:⋯
715:λ
707:⋮
701:⋮
679:λ
673:⋯
656:λ
641:Π
588:…
555:Λ
526:λ
519:…
507:λ
486:Π
460:×
425:Λ
394:⊆
391:Λ
345:≅
342:Λ
303:⊂
300:Λ
231:Lie group
127:Λ
14630:15049025
14479:Archived
14349:See also
8369:for any
8081:for any
5894:, hence
4130:acts on
3252:sending
3106:′
3069:′
2992:sending
2891:′
2812:′
2775:′
2720:′
2683:′
2631:′
2567:′
2530:′
2493:′
2462:′
2449:′
2438:′
1821:-matrix
85:, where
77:). Here
14726:Bibcode
14527:1713785
9789:, then
9364:we get
6657:Example
4660:factors
2075:Example
1231:Example
478:matrix
228:complex
182:) when
159:> 1
141:compact
102:lattice
89:is the
75:circles
14785:817920
14783:
14744:
14628:
14564:
14525:
14515:
14456:
14446:
14416:
14406:
10871:where
10084:where
9746:so if
8823:where
7463:where
4956:has a
1701:where
1624:where
155:. For
14781:S2CID
14763:arXiv
14742:S2CID
14716:arXiv
14692:arXiv
14626:S2CID
14608:arXiv
14562:S2CID
14482:(PDF)
14475:(PDF)
10834:, or
8524:with
7670:with
7282:, so
6390:. If
1096:, so
202:= 1.
139:is a
64:torus
62:is a
35:and ω
14513:ISBN
14454:OCLC
14444:ISBN
14414:OCLC
14404:ISBN
14293:for
12725:and
12350:for
7374:and
6627:and
6595:for
4816:for
4502:and
4260:The
2736:and
2426:and
322:and
47:, a
14773:doi
14759:141
14734:doi
14644:Alt
14618:doi
14584:1-B
14554:doi
14550:203
14238:exp
13618:Pic
13434:of
13328:Hom
13261:exp
13247:to
13199:Pic
12954:Hom
12897:Hom
12754:exp
12654:Pic
12627:Pic
12509:Hom
12464:Pic
11909:exp
11782:on
11588:Hom
11536:Hom
11395:exp
11279:exp
11231:exp
10727:Hom
10625:exp
10504:Hom
10427:Pic
10397:on
10233:exp
8943:of
8867:Alt
8687:on
8504:on
7417:Pic
6883:on
6424:exp
6370:on
5467:ker
4780:exp
3879:of
2837:End
2787:Hom
2758:Hom
2695:Hom
2666:Hom
2029:det
1994:det
1939:det
1920:det
1907:det
1769:det
1709:det
1152:is
1076:to
870:as
773:so
613:of
190:of
93:of
43:In
14811::
14779:.
14771:.
14761:.
14757:.
14740:.
14732:.
14724:.
14712:64
14710:.
14690:.
14624:.
14616:.
14602:.
14582:.
14578:.
14560:.
14548:.
14544:.
14523:MR
14521:,
14477:.
14452:.
14426:^
14412:.
14388:^
14254:Im
13547::=
12713:.
12675:NS
11703:.
11450:Im
11411:Im
11295:Im
11247:Im
11041::
10249:Im
10059:Im
9813:Im
8750:.
8533:Im
8401:.
8230:Im
6653:.
6235:,
5988:.
4662:.
3989:.
3553:,
3376:.
2904:.
2384:.
2372:.
2320:Im
2036:Im
1773:Im
1713:Im
1227:.
360:.
218:.
175:.
97:.
14787:.
14775::
14765::
14748:.
14736::
14728::
14718::
14700:.
14694::
14668:)
14664:Z
14660:,
14654:(
14649:3
14632:.
14620::
14610::
14604:6
14568:.
14556::
14498:.
14460:.
14420:.
14333:)
14327:,
14324:H
14321:(
14301:H
14277:)
14274:)
14268:(
14263:0
14259:l
14247:i
14244:(
14235:=
14232:)
14227:0
14223:l
14219:,
14213:(
14203:)
14200:1
14197:(
14194:U
14170::
14139:)
14134:2
14130:v
14126:(
14121:1
14117:l
14113:+
14104:)
14099:1
14095:v
14091:(
14086:2
14082:l
14075:=
14072:)
14069:)
14064:2
14060:l
14056:,
14051:2
14047:v
14043:(
14040:,
14037:)
14032:1
14028:l
14024:,
14019:1
14015:v
14011:(
14008:(
14005:H
13997:C
13990:)
13974:V
13971:(
13965:)
13949:V
13946:(
13943::
13940:H
13914:P
13880:X
13871:}
13868:0
13865:{
13860:|
13853:P
13830:L
13804:X
13795:]
13792:L
13789:[
13769:L
13761:}
13758:]
13755:L
13752:[
13749:{
13743:X
13738:|
13731:P
13706:X
13686:X
13664:P
13634:)
13631:X
13628:(
13623:0
13590:X
13567:)
13564:L
13561:(
13558:K
13554:/
13550:X
13538:X
13515:L
13509:)
13506:L
13503:(
13493:x
13489:T
13468:X
13462:x
13442:X
13422:)
13419:L
13416:(
13413:K
13393:X
13373:L
13353:)
13350:)
13347:1
13344:(
13341:U
13338:,
13332:(
13294:)
13285:,
13282:l
13276:i
13270:2
13267:(
13255:l
13235:l
13215:)
13212:X
13209:(
13204:0
13185:X
13163:X
13129:/
13106:X
13060:}
13056:Z
13043:,
13040:l
13034::
13018:l
13015:{
13012:=
12980:)
12976:R
12972:,
12969:V
12966:(
12960:R
12928:)
12924:C
12920:,
12917:V
12914:(
12904:C
12892:=
12816:v
12794:)
12790:)
12784:(
12775:v
12771:i
12765:2
12761:(
12751:=
12748:)
12742:(
12693:0
12685:)
12682:X
12679:(
12664:)
12661:X
12658:(
12643:)
12640:X
12637:(
12632:0
12615:1
12587:0
12579:)
12576:X
12573:(
12570:S
12567:N
12557:)
12551:(
12546:P
12534:)
12531:)
12528:1
12525:(
12522:U
12519:,
12513:(
12498:1
12474:)
12471:X
12468:(
12457:)
12451:(
12446:P
12424:L
12404:H
12384:X
12378:L
12358:L
12332:)
12326:,
12323:H
12320:(
12316:a
12295:)
12289:,
12286:H
12283:(
12280:L
12260:)
12257:t
12254:)
12251:v
12248:,
12242:(
12237:)
12231:,
12228:H
12225:(
12221:a
12217:,
12214:t
12211:+
12208:v
12205:(
12202:=
12199:)
12196:t
12193:,
12190:v
12187:(
12160:C
12153:V
12109:/
12104:C
12097:V
12091:)
12085:,
12082:H
12079:(
12076:L
12056:)
12053:v
12050:,
12044:(
12041:a
12038:)
12032:+
12029:v
12026:,
12020:(
12017:a
12014:=
12011:)
12008:v
12005:,
11999:+
11993:(
11990:a
11970:)
11967:)
11961:,
11955:(
11952:H
11947:2
11939:+
11936:)
11930:,
11927:v
11924:(
11921:H
11915:(
11906:)
11900:(
11894:=
11891:)
11888:v
11885:,
11879:(
11876:a
11849:C
11841:V
11832::
11827:)
11821:,
11818:H
11815:(
11811:a
11768:)
11762:,
11759:H
11756:(
11752:a
11731:)
11725:,
11722:H
11719:(
11691:)
11685:,
11682:H
11679:(
11676:L
11656:1
11650:)
11647:X
11644:(
11641:S
11638:N
11632:)
11626:(
11621:P
11613:)
11610:)
11607:1
11604:(
11601:U
11598:,
11592:(
11581:1
11561:)
11558:)
11555:1
11552:(
11549:U
11546:,
11540:(
11515:)
11512:X
11509:(
11506:S
11503:N
11479:)
11476:)
11470:,
11464:(
11459:2
11455:H
11443:i
11440:+
11437:)
11431:,
11425:(
11420:1
11416:H
11404:i
11401:(
11392:)
11386:(
11381:2
11371:1
11363:)
11357:(
11352:2
11342:1
11334:=
11324:)
11321:)
11315:,
11309:(
11304:2
11300:H
11288:i
11285:(
11276:)
11273:)
11267:,
11261:(
11256:1
11252:H
11240:i
11237:(
11228:)
11222:(
11217:2
11209:)
11203:(
11198:2
11190:)
11184:(
11179:1
11171:)
11165:(
11160:1
11152:=
11142:)
11136:+
11130:(
11125:2
11117:)
11111:+
11105:(
11100:1
11092:=
11085:)
11079:+
11073:(
11068:2
11058:1
11027:2
11017:1
10992:)
10987:2
10977:1
10969:,
10964:2
10960:H
10956:+
10951:1
10947:H
10943:(
10940:=
10937:)
10932:2
10924:,
10919:2
10915:H
10911:(
10905:)
10900:1
10892:,
10887:1
10883:H
10879:(
10859:)
10853:(
10848:P
10822:)
10819:H
10816:,
10810:(
10788:g
10785:2
10780:Z
10774:/
10768:g
10765:2
10760:R
10752:)
10749:)
10746:1
10743:(
10740:U
10737:,
10731:(
10687:v
10665:)
10661:)
10655:(
10646:v
10642:i
10636:2
10632:(
10622:=
10619:)
10613:(
10590:)
10587:1
10584:(
10581:U
10574:Z
10569:/
10564:R
10556:R
10529:)
10526:)
10523:1
10520:(
10517:U
10514:,
10508:(
10483:X
10463:0
10443:)
10440:X
10437:(
10432:0
10381:X
10375:X
10368:C
10347:)
10344:X
10341:(
10338:S
10335:N
10315:H
10271:)
10268:)
10262:,
10256:(
10253:H
10242:i
10239:(
10230:)
10224:(
10218:)
10212:(
10206:=
10203:)
10197:+
10191:(
10168:)
10165:1
10162:(
10159:U
10150::
10127:H
10099:Z
10092:a
10069:)
10063:(
10048:w
10043:z
10034:a
10031:=
10028:)
10025:w
10022:,
10019:z
10016:(
10011:a
10007:H
9985:C
9977:C
9969:C
9965::
9960:a
9956:H
9933:a
9929:E
9908:a
9888:)
9885:w
9882:i
9879:,
9876:v
9873:i
9870:(
9867:E
9864:=
9861:)
9858:w
9855:,
9852:v
9849:(
9846:E
9823:)
9817:(
9808:1
9803:a
9800:=
9797:e
9776:Z
9769:)
9763:,
9757:(
9754:E
9730:0
9727:=
9722:)
9714:1
9706:e
9694:2
9686:e
9680:(
9670:)
9662:2
9648:1
9637:(
9627:2
9619:e
9616:=
9611:)
9603:1
9595:e
9583:2
9575:e
9569:(
9559:)
9553:0
9546:1
9540:(
9529:2
9521:e
9515:=
9510:)
9504:e
9494:0
9488:(
9478:)
9470:2
9456:1
9445:(
9437:0
9434:=
9429:)
9423:e
9413:0
9407:(
9397:)
9391:0
9384:1
9378:(
9349:,
9346:1
9320:)
9312:1
9304:e
9292:2
9284:e
9278:(
9273:=
9268:)
9260:2
9246:1
9235:(
9227:E
9219:)
9213:e
9203:0
9197:(
9192:=
9187:)
9181:0
9174:1
9168:(
9160:E
9133:,
9130:1
9108:)
9102:0
9097:e
9087:e
9082:0
9076:(
9071:=
9068:E
9046:1
9042:y
9036:2
9032:z
9028:+
9023:1
9019:x
9013:1
9009:z
9005:=
9000:2
8996:z
8992:i
8989:+
8984:1
8980:z
8976:=
8973:z
8952:C
8929:1
8925:y
8921:,
8916:1
8912:x
8891:)
8887:Z
8883:,
8877:(
8872:2
8859:E
8838:H
8809:)
8798:1
8792:(
8768:E
8737:Z
8730:)
8724:,
8718:(
8715:E
8695:V
8675:E
8655:)
8651:Z
8647:,
8644:X
8641:(
8636:2
8632:H
8625:)
8615:X
8609:O
8603:(
8598:1
8594:H
8590::
8585:1
8581:c
8559:Z
8552:)
8546:,
8540:(
8537:H
8512:V
8492:H
8472:)
8469:X
8466:(
8463:S
8460:N
8433:/
8429:V
8426:=
8423:X
8389:V
8383:w
8380:,
8377:v
8354:)
8351:w
8348:,
8345:v
8342:(
8339:E
8336:i
8333:+
8330:)
8327:w
8324:,
8321:v
8318:i
8315:(
8312:E
8309:=
8306:)
8303:w
8300:,
8297:v
8294:(
8291:H
8270:)
8267:w
8264:,
8261:v
8258:(
8255:E
8252:=
8249:)
8246:w
8243:,
8240:v
8237:(
8234:H
8205:C
8198:V
8192:V
8189::
8186:H
8166:X
8160:L
8140:)
8137:L
8134:(
8129:1
8125:c
8101:V
8095:w
8092:,
8089:v
8069:)
8066:w
8063:,
8060:v
8057:(
8054:E
8051:=
8048:)
8045:w
8042:i
8039:,
8036:v
8033:i
8030:(
8027:E
8005:Z
7998:)
7992:,
7986:(
7983:E
7959:R
7952:V
7946:V
7943::
7940:E
7919:R
7897:R
7876:X
7870:L
7848:L
7844:E
7822:Z
7786:)
7783:v
7780:(
7774:)
7771:v
7768:,
7762:(
7759:f
7756:=
7753:)
7747:+
7744:v
7741:(
7717:C
7710:V
7707::
7684:X
7678:U
7657:}
7649:)
7646:U
7643:(
7638:1
7618:)
7615:v
7612:,
7606:(
7594:)
7591:v
7588:(
7582:)
7579:v
7576:,
7570:(
7567:f
7564:=
7561:)
7555:+
7552:v
7549:(
7531::
7527:C
7520:)
7517:U
7514:(
7509:1
7498::
7491:{
7487:=
7484:)
7481:U
7478:(
7473:L
7449:L
7427:)
7424:X
7421:(
7410:]
7407:L
7404:[
7401:=
7398:]
7395:f
7392:[
7387:1
7362:)
7359:)
7349:V
7343:O
7337:,
7334:V
7331:(
7326:0
7322:H
7318:,
7312:(
7307:1
7303:H
7296:]
7293:f
7290:[
7263:C
7255:V
7246::
7243:f
7223:L
7193:)
7187:0
7180:3
7177:,
7174:4
7170:e
7159:2
7156:,
7153:4
7149:e
7138:1
7135:,
7132:4
7128:e
7115:3
7112:,
7109:4
7105:e
7099:0
7092:3
7089:,
7086:2
7082:e
7071:1
7068:,
7065:3
7061:e
7048:2
7045:,
7042:4
7038:e
7030:2
7027:,
7024:3
7020:e
7014:0
7007:1
7004:,
7001:2
6997:e
6984:1
6981:,
6978:4
6974:e
6966:1
6963:,
6960:3
6956:e
6948:1
6945:,
6942:2
6938:e
6932:0
6926:(
6921:=
6916:L
6912:E
6869:L
6865:E
6842:2
6837:C
6807:2
6802:C
6778:)
6772:1
6767:0
6760:2
6757:,
6754:2
6750:z
6742:1
6739:,
6736:2
6732:z
6724:0
6719:1
6712:2
6709:,
6706:1
6702:z
6694:1
6691:,
6688:1
6684:z
6677:(
6672:=
6641:V
6635:v
6606:,
6583:)
6580:v
6577:,
6571:(
6568:g
6562:)
6556:+
6553:v
6550:,
6544:(
6541:g
6535:)
6532:v
6529:,
6523:(
6520:g
6517:+
6514:)
6508:+
6505:v
6502:,
6496:(
6493:g
6490:=
6487:)
6481:,
6475:(
6470:L
6466:E
6445:)
6442:g
6439:i
6433:2
6430:(
6421:=
6418:f
6398:L
6356:L
6352:E
6330:Z
6309:)
6306:L
6303:(
6298:1
6294:c
6273:)
6269:Z
6265:,
6259:(
6254:2
6250:t
6246:l
6243:A
6203:)
6199:Z
6195:,
6192:X
6189:(
6184:2
6180:H
6155:)
6151:Z
6147:,
6144:X
6141:(
6136:2
6132:H
6125:)
6115:X
6109:O
6103:(
6098:1
6094:H
6090::
6085:1
6081:c
6060:0
6047:X
6041:O
6030:X
6024:O
6014:Z
6007:0
5976:X
5956:)
5953:)
5943:V
5937:O
5931:(
5926:0
5922:H
5918:,
5912:(
5907:1
5903:H
5882:X
5842:)
5839:X
5836:(
5831:1
5806:)
5796:X
5790:O
5784:,
5781:X
5778:(
5773:1
5769:H
5762:)
5759:)
5744:X
5736:O
5730:(
5725:0
5721:H
5717:,
5714:)
5711:X
5708:(
5703:1
5695:(
5690:1
5686:H
5665:0
5659:)
5644:X
5636:O
5630:,
5621:X
5615:(
5610:1
5606:H
5577:)
5574:)
5559:X
5551:O
5545:,
5536:X
5530:(
5525:1
5521:H
5514:)
5504:X
5498:O
5492:,
5489:X
5486:(
5481:1
5477:H
5473:(
5461:)
5458:)
5443:X
5435:O
5429:(
5424:0
5420:H
5416:,
5413:)
5410:X
5407:(
5402:1
5394:(
5389:1
5385:H
5364:)
5354:X
5348:O
5342:,
5339:X
5336:(
5331:1
5327:H
5320:)
5317:)
5302:X
5294:O
5288:(
5283:0
5279:H
5275:,
5272:)
5269:X
5266:(
5261:1
5253:(
5248:1
5244:Z
5223:X
5211:X
5205::
5182:X
5176:L
5173::
5170:p
5150:)
5147:X
5144:(
5139:1
5130:/
5125:C
5112:X
5106:=
5103:L
5083:f
5063:)
5060:t
5054:)
5051:x
5048:,
5045:a
5042:(
5039:f
5036:,
5033:x
5027:a
5024:(
5021:=
5018:)
5015:t
5012:,
5009:x
5006:(
5000:a
4980:)
4977:X
4974:(
4969:1
4938:X
4928:C
4915:X
4892:X
4872:f
4843:C
4830:V
4827::
4824:g
4804:)
4801:g
4795:i
4789:2
4786:(
4777:=
4774:f
4739:C
4729:n
4726:2
4721:Z
4711:n
4706:C
4701::
4698:f
4678:f
4646:f
4622:)
4619:)
4604:X
4596:O
4590:(
4585:0
4581:H
4577:,
4574:)
4571:X
4568:(
4563:1
4555:(
4550:1
4546:Z
4519:X
4510:x
4490:)
4487:X
4484:(
4479:1
4468:b
4465:,
4462:a
4442:)
4439:x
4436:,
4433:b
4430:(
4427:f
4424:)
4421:x
4415:b
4412:,
4409:a
4406:(
4403:f
4400:=
4397:)
4394:x
4391:,
4388:b
4382:a
4379:(
4376:f
4349:C
4335:X
4326:)
4323:X
4320:(
4315:1
4307::
4304:f
4284:)
4281:X
4278:(
4273:1
4248:}
4238:C
4224:X
4218::
4215:f
4212:{
4209:=
4206:)
4191:X
4183:O
4177:(
4172:0
4168:H
4141:X
4118:)
4115:X
4112:(
4107:1
4082:)
4079:)
4064:X
4056:O
4050:(
4045:0
4041:H
4037:,
4034:)
4031:X
4028:(
4023:1
4015:(
4010:1
4006:H
3975:n
3970:C
3956:X
3933:L
3903:)
3900:X
3897:(
3892:1
3857:X
3848:L
3818:X
3812:L
3789:X
3755:2
3751:g
3747:2
3744:=
3739:2
3735:g
3731:2
3723:2
3719:g
3715:4
3695:)
3692:g
3689:(
3683:C
3678:L
3674:G
3670:/
3666:)
3663:g
3660:2
3657:(
3651:R
3646:L
3642:G
3636:)
3633:g
3630:2
3627:(
3621:Z
3616:L
3612:G
3604:g
3598:T
3570:g
3564:T
3541:g
3521:)
3518:g
3515:2
3512:(
3506:Z
3501:L
3497:G
3477:)
3474:g
3471:(
3465:C
3460:L
3456:G
3452:/
3448:)
3445:g
3442:2
3439:(
3433:R
3428:L
3424:G
3402:g
3399:2
3394:R
3361:n
3357:/
3331:g
3328:2
3324:)
3319:Z
3315:n
3311:/
3306:Z
3302:(
3294:n
3290:X
3269:x
3266:n
3260:x
3240:X
3234:X
3231::
3226:X
3222:n
3199:0
3191:Z
3183:n
3155:f
3147:)
3144:0
3141:(
3138:h
3134:t
3130:=
3127:h
3103:X
3096:X
3093::
3090:f
3066:X
3062:,
3059:X
3039:h
3017:0
3013:x
3009:+
3006:x
3000:x
2980:X
2974:X
2971::
2964:0
2960:x
2955:t
2934:X
2926:0
2922:x
2888:g
2884:g
2881:4
2875:m
2854:Q
2847:)
2844:X
2841:(
2816:)
2805:,
2799:(
2793:Z
2779:)
2772:X
2768:,
2765:X
2762:(
2754::
2749:r
2724:)
2717:V
2713:,
2710:V
2707:(
2701:C
2687:)
2680:X
2676:,
2673:X
2670:(
2662::
2657:a
2618::
2609:F
2588:F
2564:V
2557:V
2554::
2551:F
2527:X
2520:X
2517::
2514:f
2490:g
2486:,
2483:g
2454:/
2446:V
2442:=
2435:X
2410:/
2406:V
2403:=
2400:X
2358:2
2353:+
2350:2
2330:)
2327:Z
2324:(
2297:)
2291:1
2286:0
2281:i
2276:2
2271:+
2268:1
2263:i
2260:2
2257:+
2254:1
2247:0
2242:1
2237:i
2231:1
2226:i
2223:+
2220:1
2214:(
2190:)
2184:1
2179:0
2172:2
2169:,
2166:2
2162:z
2154:1
2151:,
2148:2
2144:z
2136:0
2131:1
2124:2
2121:,
2118:1
2114:z
2106:1
2103:,
2100:1
2096:z
2089:(
2055:0
2049:)
2046:)
2043:Z
2040:(
2032:(
2016:)
2008:Z
2000:Z
1997:(
1991:=
1981:)
1973:Z
1966:g
1962:1
1956:g
1952:1
1945:Z
1942:(
1936:)
1931:g
1927:1
1923:(
1917:=
1910:P
1881:)
1873:g
1869:1
1858:Z
1847:g
1843:1
1837:Z
1831:(
1809:P
1789:0
1783:)
1780:Z
1777:(
1749:V
1729:0
1723:)
1720:Z
1717:(
1689:)
1686:g
1683:(
1677:C
1672:t
1668:a
1665:M
1659:Z
1637:g
1633:1
1612:)
1607:g
1603:1
1599:,
1596:Z
1593:(
1553:g
1529:/
1525:V
1522:=
1519:X
1489:)
1483:1
1478:1
1473:i
1465:1
1458:i
1455:2
1450:i
1445:0
1440:1
1434:(
1429:=
1404:)
1396:4
1393:,
1390:2
1378:3
1375:,
1372:2
1360:2
1357:,
1354:2
1342:1
1339:,
1336:2
1322:4
1319:,
1316:1
1304:3
1301:,
1298:1
1286:2
1283:,
1280:1
1268:1
1265:,
1262:1
1251:(
1246:=
1214:R
1191:g
1186:C
1138:)
1112:(
1107:=
1104:P
1037:)
1034:g
1031:2
1028:,
1025:g
1022:2
1019:(
1013:C
1008:t
1004:a
1001:M
995:P
975:)
972:g
969:2
966:,
963:g
960:(
954:C
949:t
945:a
942:M
914:g
911:2
906:Z
897:/
891:g
886:C
881:=
878:X
854:/
850:V
847:=
844:X
822:j
818:e
812:i
809:j
799:j
791:=
786:i
759:)
751:g
748:2
745:,
742:g
725:1
722:,
719:g
692:g
689:2
686:,
683:1
666:1
663:,
660:1
649:(
644:=
621:V
599:g
595:e
591:,
585:,
580:1
576:e
533:g
530:2
522:,
516:,
511:1
466:g
463:2
457:g
447:g
421:/
417:V
397:V
371:V
348:U
338:/
332:g
308:g
280:G
275:0
271:T
267:=
262:g
240:G
208:n
200:n
184:n
157:n
145:n
123:/
119:V
106:C
95:M
87:n
83:n
79:N
72:N
56:M
37:2
33:1
20:)
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.