83:
50:
27:
6298:
113:
3550:
4224:
2976:
2866:
The representation of a permutation as a product of transpositions is not unique; however, the number of transpositions needed to represent a given permutation is either always even or always odd. There are several short proofs of the invariance of this parity of a permutation.
2619:
3857:
7694:
7505:
2451:
2319:
6229:(1915) proved that each permutation can be written as a product of three squares. (Any squared element must belong to the hypothesized subgroup of index 2, hence so must the product of any number of squares.) However it contains the normal subgroup
4450:
3658:
3424:
3327:
4004:
7906:
7812:
8284:
rather than representations. The representation obtained from an irreducible representation defined over the integers by reducing modulo the characteristic will not in general be irreducible. The modules so constructed are called
1983:
which are not solvable by radicals, that is, the solutions cannot be expressed by performing a finite number of operations of addition, subtraction, multiplication, division and root extraction on the polynomial's coefficients.
3044:
3991:
2880:
7997:
8061:
7950:
4581:
2842:
is a permutation which exchanges two elements and keeps all others fixed; for example (1 3) is a transposition. Every permutation can be written as a product of transpositions; for instance, the permutation
5976:
7110:
are generated by elements like (123)(45) that have one cycle of length 3 and another cycle of length 2. This rules out many groups as possible subgroups of symmetric groups of a given size. For example,
6989:
8218:. Unlike the general situation for finite groups, there is in fact a natural way to parametrize irreducible representation by the same set that parametrizes conjugacy classes, namely by partitions of
8277:
is semisimple. In these cases the irreducible representations defined over the integers give the complete set of irreducible representations (after reduction modulo the characteristic if necessary).
4993:, since these symmetries permute the three vertices of the triangle. Cycles of length two correspond to reflections, and cycles of length three are rotations. In Galois theory, the sign map from S
2478:
3737:
6085:
5862:
4861:, the representation theory of the symmetric group on two points is quite simple and is seen as writing a function of two variables as a sum of its symmetric and anti-symmetric parts: Setting
2780:
7586:
7397:
5608:
8291:, and every irreducible does arise inside some such module. There are now fewer irreducibles, and although they can be classified they are very poorly understood. For example, even their
8233:
Each such irreducible representation can be realized over the integers (every permutation acting by a matrix with integer coefficients); it can be explicitly constructed by computing the
4363:
2325:
4300:
2193:
7041:
1677:
1494:
8298:
The determination of the irreducible modules for the symmetric group over an arbitrary field is widely regarded as one of the most important open problems in representation theory.
4682:
6647:
5799:
3729:
1618:
1412:
1329:
5915:
1558:
1587:
1381:
1059:
932:
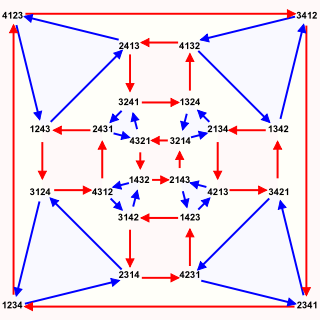
7567:
send an involution to 1 (the trivial map) or to −1 (the sign map). One must also show that the sign map is well-defined, but assuming that, this gives the first homology of S
3545:{\displaystyle \sigma {\begin{pmatrix}a&b&c&\ldots \end{pmatrix}}\sigma ^{-1}={\begin{pmatrix}\sigma (a)&\sigma (b)&\sigma (c)&\ldots \end{pmatrix}}}
4219:{\displaystyle \mathrm {sgn} (\rho _{n})=(-1)^{\lfloor n/2\rfloor }=(-1)^{n(n-1)/2}={\begin{cases}+1&n\equiv 0,1{\pmod {4}}\\-1&n\equiv 2,3{\pmod {4}}\end{cases}}}
3581:
558:
533:
496:
3243:
1645:
1439:
8809:
6018:
7119:), because the only group of order 15 is the cyclic group. The largest possible order of a cyclic subgroup (equivalently, the largest possible order of an element in S
7908:
due to the exceptional 3-fold cover) does not change the homology of the symmetric group; the alternating group phenomena do yield symmetric group phenomena – the map
4360:
can be constructed in "two line notation" by placing the "cycle notations" of the two conjugate permutations on top of one another. Continuing the previous example,
3412:
1927:
1891:
7553:
and all involutions are conjugate, hence map to the same element in the abelianization (since conjugation is trivial in abelian groups). Thus the only possible maps
7098:
are those that are generated by a single permutation. When a permutation is represented in cycle notation, the order of the cyclic subgroup that it generates is the
1957:
8280:
However, the irreducible representations of the symmetric group are not known in arbitrary characteristic. In this context it is more usual to use the language of
4776:
1841:
1815:
7817:
7723:
7704:
5297:
1741:
1462:
1082:
1003:
1981:
1789:
1765:
1715:
1514:
1352:
1279:
1252:
1232:
1212:
1184:
1152:
1023:
980:
956:
2137:
The group operation in a symmetric group is function composition, denoted by the symbol ∘ or simply by just a composition of the permutations. The composition
7138:
5275:
4818:. In this case the alternating group agrees with the symmetric group, rather than being an index 2 subgroup, and the sign map is trivial. In the case of S
4787:
2987:
3564:} are of particular interest (these can be generalized to the symmetric group of any finite totally ordered set, but not to that of an unordered set).
9029:
5269:
fixes a point and thus is not transitive) and, while this map does not make the general quintic solvable, it yields the exotic outer automorphism of S
4455:
2870:
The product of two even permutations is even, the product of two odd permutations is even, and all other products are odd. Thus we can define the
6341:
fall into three classes: the intransitive, the imprimitive, and the primitive. The intransitive maximal subgroups are exactly those of the form
3863:
6266:
860:
4452:
which can be written as the product of cycles as (2 4). This permutation then relates (1 2 3)(4 5) and (1 4 3)(2 5) via conjugation, that is,
2971:{\displaystyle \operatorname {sgn} f={\begin{cases}+1,&{\text{if }}f{\mbox{ is even}}\\-1,&{\text{if }}f{\text{ is odd}}.\end{cases}}}
8162:
8156:
2025:
7955:
8543:
Theo
Douvropoulos; Joel Brewster Lewis; Alejandro H. Morales (2022), "Hurwitz Numbers for Reflection Groups I: Generatingfunctionology",
8022:
7911:
7083:
is isomorphic to a subgroup of some symmetric group. In particular, one may take a subgroup of the symmetric group on the elements of
3365:
by starting at a different point. The order of a cycle is equal to its length. Cycles of length two are transpositions. Two cycles are
1258:. For finite sets, "permutations" and "bijective functions" refer to the same operation, namely rearrangement. The symmetric group of
7052:
5921:
8895:
6934:
3148:
is an application of this fact. The representation of a permutation as a product of adjacent transpositions is also not unique.
8326:
8166:
6416:
2614:{\displaystyle fg=f\circ g=(1\ 2\ 4)(3\ 5)={\begin{pmatrix}1&2&3&4&5\\2&4&5&1&3\end{pmatrix}}.}
418:
5098:
also yields a 2-dimensional irreducible representation, which is an irreducible representation of a symmetric group of degree
9015:
8993:
8868:
8793:
8764:
8738:
8591:
8492:
8461:
6265:
are the only nontrivial proper normal subgroups of the symmetric group on a countably infinite set. This was first proved by
3852:{\displaystyle (1\,n)(2\,n-1)\cdots ,{\text{ or }}\sum _{k=1}^{n-1}k={\frac {n(n-1)}{2}}{\text{ adjacent transpositions: }}}
2016:, the symmetric group acts on the variables of a multi-variate function, and the functions left invariant are the so-called
368:
2021:
1123:
9027:(1911), "Über die Darstellung der symmetrischen und der alternierenden Gruppe durch gebrochene lineare Substitutionen",
7689:{\displaystyle H_{2}(\mathrm {S} _{n},\mathbf {Z} )={\begin{cases}0&n<4\\\mathbf {Z} /2&n\geq 4.\end{cases}}}
7500:{\displaystyle H_{1}(\mathrm {S} _{n},\mathbf {Z} )={\begin{cases}0&n<2\\\mathbf {Z} /2&n\geq 2.\end{cases}}}
3391:
the order of the factors, and the freedom present in representing each individual cycle by choosing its starting point.
6025:
5815:
3668:
2674:
1682:
Symmetric groups on infinite sets behave quite differently from symmetric groups on finite sets, and are discussed in (
853:
363:
9198:
8414:
6090:
where 1 represents the identity permutation. This representation endows the symmetric group with the structure of a
2446:{\displaystyle g=(1\ 2\ 5)(3\ 4)={\begin{pmatrix}1&2&3&4&5\\2&5&4&3&1\end{pmatrix}}.}
2314:{\displaystyle f=(1\ 3)(2)(4\ 5)={\begin{pmatrix}1&2&3&4&5\\3&2&1&5&4\end{pmatrix}}}
2823:
that undoes its action, and thus each element of a symmetric group does have an inverse which is a permutation too.
8169:, for which a concrete and detailed theory can be obtained. This has a large area of potential applications, from
6233:
of permutations that fix all but finitely many elements, which is generated by transpositions. Those elements of
4252:
4837:
This group consists of exactly two elements: the identity and the permutation swapping the two points. It is a
6994:
5516:
in either the identity (and thus themselves be the identity or a 2-element group, which is not normal), or in A
779:
4792:
The low-degree symmetric groups have simpler and exceptional structure, and often must be treated separately.
4354:, (1 2 3)(4 5) and (1 4 3)(2 5) are conjugate; (1 2 3)(4 5) and (1 2)(4 5) are not. A conjugating element of S
9119:
1650:
1467:
846:
5039:
is isomorphic to the group of proper rotations about opposite faces, opposite diagonals and opposite edges,
2464:
maps 1 first to 2 and then 2 to itself; 2 to 5 and then to 4; 3 to 4 and then to 5, and so on. So composing
87:
Some matrices are not arranged symmetrically to the main diagonal – thus the symmetric group is not abelian.
9164:
4633:
2098:
8970:
1111:
theory. For the remainder of this article, "symmetric group" will mean a symmetric group on a finite set.
9188:
9114:
8331:
8321:
6602:
6523:
5753:
4350:
if and only if they consist of the same number of disjoint cycles of the same lengths. For instance, in S
3700:
2833:
1592:
1386:
1284:
463:
277:
5879:
5033:
57:
8730:
8207:
6412:
5393:(or rather, a class of such maps up to inner automorphism) corresponding to the outer automorphism of S
5014:
2086:
1519:
195:
6309:
1563:
1357:
1035:
908:
9109:
6815:
6120:, or more generally any set of transpositions that forms a connected graph, and a set containing any
4986:
4445:{\displaystyle k={\begin{pmatrix}1&2&3&4&5\\1&4&3&2&5\end{pmatrix}},}
3653:{\displaystyle {\begin{pmatrix}1&2&\cdots &n\\n&n-1&\cdots &1\end{pmatrix}}.}
2856:
7634:
7445:
7372:
4124:
3322:{\displaystyle h={\begin{pmatrix}1&2&3&4&5\\4&2&1&3&5\end{pmatrix}}}
2901:
8292:
8253:
7287:
7106:, one cyclic subgroup of order 5 is generated by (13254), whereas the largest cyclic subgroups of S
1930:
1096:
661:
395:
272:
160:
8206:. Therefore, according to the representation theory of a finite group, the number of inequivalent
6411:. The primitive maximal subgroups are more difficult to identify, but with the assistance of the
541:
516:
479:
9073:
8312:
6478:
6250:
1623:
1417:
1091:, this article focuses on the finite symmetric groups: their applications, their elements, their
8507:
J. Irving; A. Rattan (2009), "Minimal factorizations of permutations into star transpositions",
5981:
3671:
in the symmetric group with respect to generating set consisting of the adjacent transpositions
2798:, it is necessary to verify the group axioms of closure, associativity, identity, and inverses.
8387:
8317:
5748:
3118:
811:
601:
65:
6423:) gave a fairly satisfactory description of the maximal subgroups of this type, according to (
76:. Green circle is an odd permutation, white is an even permutation and black is the identity.
35:
8919:
8402:
7283:
7099:
6853:
3397:
2106:
2102:
1906:
1858:
890:
685:
82:
8484:
8453:
8432:
Die
Untergruppenverbände der Gruppen der Ordnungen ̤100 mit Ausnahme der Ordnungen 64 und 96
2802:
The operation of function composition is closed in the set of permutations of the given set
1936:
49:
8878:
8840:
8774:
8281:
8261:
7901:{\displaystyle H_{2}(\mathrm {A} _{6})\cong H_{2}(\mathrm {A} _{7})\cong \mathrm {C} _{6},}
7807:{\displaystyle H_{1}(\mathrm {A} _{3})\cong H_{1}(\mathrm {A} _{4})\cong \mathrm {C} _{3},}
7126:
6257:, it is also a normal subgroup of the full symmetric group of the infinite set. The groups
5132:
4990:
4854:
4249:
is the permutation that splits the set into 2 piles and interleaves them. Its sign is also
3370:
2062:
2050:
1718:
1255:
1108:
1029:
902:
625:
613:
231:
165:
69:
45:
of all four set elements, and (blue) a left circular shift of the first three set elements.
9094:
8959:
4761:
8:
9150:
8245:
8178:
7534:
is generated by involutions (2-cycles, which have order 2), so the only non-trivial maps
7076:
5684:
5239:
2795:
1820:
1794:
1131:
886:
200:
95:
8542:
5242:. The resolvent of a quintic is of degree 6—this corresponds to an exotic inclusion map
1723:
1444:
1064:
985:
73:
9065:
9046:
9007:
8936:
8804:
8749:
8723:
8664:
8552:
8170:
7717:
5591:
5581:
5352:
5231:
5177:
5087:
5079:
5040:
5018:
3157:
3104:
3050:
2625:
2094:
2017:
1966:
1774:
1750:
1700:
1499:
1337:
1264:
1237:
1217:
1197:
1169:
1137:
1104:
1008:
965:
941:
185:
157:
26:
9193:
9147:
9128:
9050:
9011:
8989:
8864:
8828:
8789:
8760:
8734:
8683:
8668:
8587:
8488:
8457:
8410:
8234:
8199:
8174:
7528:< 2 the symmetric group is trivial. This homology is easily computed as follows: S
7044:
6883:
6174:
6145:
5459:
5139:
5048:
4850:
4593:
3063:
2855:
can be written as a product of an odd number of transpositions, it is then called an
2090:
2082:
1155:
882:
590:
433:
327:
9131:
9057:
8476:
6270:
6237:
that are products of an even number of transpositions form a subgroup of index 2 in
4335:
756:
9090:
9082:
9038:
8981:
8966:
8955:
8928:
8904:
8856:
8818:
8698:
8654:
8562:
8524:
8516:
7577:
7065:
6891:
6887:
6329:
6219:
6095:
5293:
5165:
5083:
5059:
5006:
5002:
4858:
4313:
3058:
2820:
2013:
1119:
874:
741:
733:
725:
717:
709:
697:
637:
577:
567:
409:
351:
226:
5308:, these do not correspond to exceptional Schur multipliers of the symmetric group.
3039:{\displaystyle \operatorname {sgn} \colon \mathrm {S} _{n}\rightarrow \{+1,-1\}\ }
8874:
8852:
8836:
8781:
8770:
8756:
8336:
8195:
8071:
7510:
The first homology group is the abelianization, and corresponds to the sign map S
7087:, since every group acts on itself faithfully by (left or right) multiplication.
6497:
6226:
6157:
5211:
fixes a point and thus is not transitive. This yields the outer automorphism of S
5063:
4325:
3074:
3054:
1092:
898:
825:
818:
804:
761:
649:
572:
402:
256:
136:
8985:
9061:
8886:
8520:
8287:
8211:
7388:
7378:
7279:
6913:
6546:
6436:
6408:
6274:
6225:
The symmetric group on an infinite set does not have a subgroup of index 2, as
6218:
where there is one additional such normal subgroup, which is isomorphic to the
5660:
5173:
4967:
4823:
3061:
of this homomorphism, that is, the set of all even permutations, is called the
2070:
2066:
1900:
1163:
832:
768:
458:
438:
375:
340:
261:
251:
236:
221:
175:
152:
42:
20:
9042:
8909:
8893:(1988), "On the O'Nan–Scott theorem for finite primitive permutation groups",
8643:"Teoria delle sostituzioni che operano su una infinità numerabile di elementi"
3387:
can be written as a product of disjoint cycles; this representation is unique
9182:
8832:
8621:
Vitali, G. (1915). "Sostituzioni sopra una infinità numerabile di elementi".
8579:
8238:
8223:
6091:
5235:
5075:
4846:
4842:
4811:
2054:
2036:
2029:
2009:
1852:
1848:
1768:
1127:
1115:
751:
673:
507:
380:
246:
9086:
9066:"Über die Automorphismen der Permutationsgruppe der natürlichen Zahlenfolge"
8980:, Graduate Texts in Mathematics, vol. 148, Springer, pp. 154–216,
8566:
9173:
8702:
8144:
7095:
6101:
Other possible generating sets include the set of transpositions that swap
5469:
5161:
5156:
is one of the three non-solvable groups of order 120, up to isomorphism. S
4838:
4245:
3986:{\displaystyle (n\,n-1)(n-1\,n-2)\cdots (2\,1)(n-1\,n-2)(n-2\,n-3)\cdots ,}
3664:
2074:
1997:
1088:
606:
305:
294:
241:
216:
211:
170:
141:
104:
53:
31:
8917:
Nakaoka, Minoru (March 1961), "Homology of the
Infinite Symmetric Group",
7351:
of cardinality other than 6, every automorphism of the symmetric group on
4985:
is the first nonabelian symmetric group. This group is isomorphic to the
4312:
elements have the same sign; these are important to the classification of
9024:
8947:
8445:
8307:
3145:
2184:
2122:
2058:
1114:
The symmetric group is important to diverse areas of mathematics such as
959:
8529:
6297:
5215:, discussed below, and corresponds to the resolvent sextic of a quintic.
8940:
8860:
8823:
8659:
8642:
8606:
Bray, J.N.; Conder, M.D.E.; Leedham-Green, C.R.; O'Brien, E.A. (2007),
8265:
8133:
6482:
6173:
has at most 2 elements, and so has no nontrivial proper subgroups. The
5351:, the most notable homomorphisms between symmetric groups, in order of
5082:, which allows the quartic to be solved by radicals, as established by
2046:
2001:
1960:
935:
773:
501:
9155:
9136:
7387:
is quite regular and stabilizes: the first homology (concretely, the
5510:, and has no other proper normal subgroups, as they would intersect A
4807:
3394:
Cycles admit the following conjugation property with any permutation
1844:
1744:
1025:
894:
594:
8932:
7992:{\displaystyle \mathrm {S} _{4}\twoheadrightarrow \mathrm {S} _{3},}
2085:
and are widely studied because of their importance in understanding
8890:
8805:"La structure des p-groupes de Sylow des groupes symétriques finis"
8557:
8056:{\displaystyle \mathrm {S} _{4}\twoheadrightarrow \mathrm {S} _{3}}
7945:{\displaystyle \mathrm {A} _{4}\twoheadrightarrow \mathrm {C} _{3}}
6372:. The imprimitive maximal subgroups are exactly those of the form
6141:
2078:
1159:
1100:
131:
8605:
2827:
6440:
4788:
Representation theory of the symmetric group § Special cases
473:
387:
5491:
which is split by taking a transposition of two elements. Thus S
5176:
translates into the non-existence of a general formula to solve
4576:{\displaystyle (2~4)\circ (1~2~3)(4~5)\circ (2~4)=(1~4~3)(2~5).}
112:
9145:
8248:
the situation can become much more complicated. If the field
8067:, and the triple covers do not correspond to homology either.
5410:
as a transitive subgroup, yielding the outer automorphism of S
3116:
Furthermore, every permutation can be written as a product of
3053:({+1, −1} is a group under multiplication, where +1 is e, the
72:. The black arrows indicate disjoint cycles and correspond to
8139:
The homology of the infinite symmetric group is computed in (
7308:, which is abelian, and hence the center is the whole group.
5971:{\displaystyle \sigma _{i}\sigma _{j}=\sigma _{j}\sigma _{i}}
5600:
of order 2 corresponds to conjugation by an odd element. For
3388:
1214:
is the group whose elements are all bijective functions from
6856:, and mentions that it is even covered in textbook form in (
6202:
it is in fact the only nontrivial proper normal subgroup of
9170:
8729:, London Mathematical Society Student Texts, vol. 45,
7682:
7493:
6984:{\displaystyle S_{a_{1}}\times \cdots \times S_{a_{\ell }}}
5131:
is the first non-solvable symmetric group. Along with the
5044:
4212:
3369:
if they have disjoint subsets of elements. Disjoint cycles
2964:
8952:
Substitutionentheorie und ihre
Anwendungen auf die Algebra
6878:
is a subgroup whose action on {1, 2, ,...,
6446:. They are more easily described in special cases first:
8851:, Lecture Notes in Mathematics, Vol. 240, vol. 240,
8684:"Über die Permutationsgruppe der natürlichen Zahlenfolge"
6582:, and every element of the Sylow 3-subgroup has the form
9126:
8608:
Short presentations for alternating and symmetric groups
7115:
has no subgroup of order 15 (a divisor of the order of S
4989:, the group of reflection and rotation symmetries of an
2112:
6852:, p. 26) attributes the result to an 1844 work of
6160:
of the finite symmetric groups are well understood. If
3663:
This is the unique maximal element with respect to the
3560:
Certain elements of the symmetric group of {1, 2, ...,
3353:, leaving 2 and 5 untouched. We denote such a cycle by
8327:
Symmetry in quantum mechanics § Exchange symmetry
4636:
4378:
3590:
3486:
3436:
3258:
3113:
and any subgroup generated by a single transposition.
2924:
2547:
2379:
2250:
8025:
7958:
7914:
7820:
7726:
7589:
7400:
7329:, and the automorphism group is a semidirect product
7139:
Automorphisms of the symmetric and alternating groups
6997:
6937:
6605:
6028:
5984:
5924:
5882:
5818:
5756:
5276:
Automorphisms of the symmetric and alternating groups
4764:
4458:
4366:
4255:
4007:
3866:
3740:
3703:
3584:
3427:
3400:
3246:
2990:
2883:
2677:
2481:
2328:
2196:
1969:
1939:
1909:
1861:
1823:
1797:
1777:
1753:
1726:
1703:
1653:
1626:
1595:
1566:
1522:
1502:
1470:
1447:
1420:
1389:
1360:
1340:
1287:
1267:
1240:
1220:
1200:
1172:
1140:
1067:
1038:
1011:
988:
968:
944:
911:
544:
519:
482:
5253:
as a transitive subgroup (the obvious inclusion map
5086:. The Klein group can be understood in terms of the
2039:, the symmetric group is the Coxeter group of type A
8810:
7720:
low-dimensional homology of the alternating group (
5078:, this map corresponds to the resolving cubic to a
4583:It is clear that such a permutation is not unique.
2812:The trivial bijection that assigns each element of
8884:
8748:
8722:
8506:
8400:
8055:
7991:
7944:
7900:
7806:
7688:
7499:
7035:
6983:
6641:
6439:of the symmetric groups are important examples of
6420:
6079:
6012:
5970:
5909:
5856:
5793:
5446:
4770:
4676:
4575:
4444:
4294:
4218:
3985:
3851:
3723:
3652:
3544:
3406:
3321:
3233:itself would not be moved either. The permutation
3038:
2970:
2774:
2613:
2445:
2313:
1975:
1951:
1921:
1885:
1835:
1809:
1783:
1759:
1735:
1709:
1671:
1639:
1612:
1581:
1552:
1508:
1488:
1456:
1433:
1406:
1375:
1346:
1323:
1273:
1246:
1226:
1206:
1189:
1178:
1146:
1076:
1053:
1017:
997:
974:
950:
926:
552:
527:
490:
7814:corresponding to non-trivial abelianization, and
7051:. These groups may also be characterized as the
6080:{\displaystyle (\sigma _{i}\sigma _{i+1})^{3}=1,}
5857:{\displaystyle \sigma _{1},\ldots ,\sigma _{n-1}}
5369:corresponding to the exceptional normal subgroup
2775:{\displaystyle (1~2~3~4~5~6)^{2}=(1~3~5)(2~4~6).}
9180:
8755:, Graduate Texts in Mathematics, vol. 163,
8132:. This is analogous to the homology of families
7102:of the lengths of its cycles. For example, in S
6927:that is generated by transpositions is called a
5312:
1929:. This is an essential part of the proof of the
9030:Journal für die reine und angewandte Mathematik
8214:, is equal to the number of partitions of
6560:, a Sylow 3-subgroup of Sym(9) is generated by
5721:to all odd permutations, while embedding into A
5192:
5180:by radicals. There is an exotic inclusion map
2828:Transpositions, sign, and the alternating group
2785:
8896:Journal of the Australian Mathematical Society
8358:
8356:
8354:
8352:
6181:is always a normal subgroup, a proper one for
4758:, whose elements are said to be of cycle-type
2816:to itself serves as an identity for the group.
2028:plays a fundamental role through the ideas of
8746:
8475:
7360:
6424:
6282:
5418:There are also a host of other homomorphisms
5300:) and that these extend to triple covers of S
5001:corresponds to the resolving quadratic for a
3415:
2117:The elements of the symmetric group on a set
1687:
905:. In particular, the finite symmetric group
854:
9165:Marcus du Sautoy: Symmetry, reality's riddle
9056:
8681:
8163:representation theory of the symmetric group
8157:Representation theory of the symmetric group
7356:
6709:-subgroups of the symmetric group of degree
6658:-subgroups of the symmetric group of degree
6541:-subgroups of the symmetric group of degree
6453:-subgroups of the symmetric group of degree
5472:, and the induced quotient is the sign map:
4284:
4270:
4066:
4052:
3718:
3704:
3414:, this property is often used to obtain its
3030:
3012:
2065:provide a rich source of problems involving
2026:representation theory of the symmetric group
1547:
1523:
1318:
1294:
1087:Although symmetric groups can be defined on
1032:(number of elements) of the symmetric group
8349:
7318:, it has an outer automorphism of order 2:
6457:are just the cyclic subgroups generated by
5738:
5017:of order 3 in the solution, in the form of
4338:of permutations; that is, two elements of S
4295:{\displaystyle (-1)^{\lfloor n/2\rfloor }.}
2809:Function composition is always associative.
2790:To check that the symmetric group on a set
79:These are the positions of the six matrices
8802:
8545:Enumerative Combinatorics and Applications
8070:The homology "stabilizes" in the sense of
7300:, the automorphism group is trivial, but S
6841:
5238:, this can also be understood in terms of
5068:{(1), (1 2)(3 4), (1 3)(2 4), (1 4)(2 3)},
861:
847:
8908:
8822:
8658:
8556:
8528:
8401:Vasishtha, A.R.; Vasishtha, A.K. (2008).
8143:), with the cohomology algebra forming a
7036:{\displaystyle (a_{1},\ldots ,a_{\ell })}
4857:after extracting only a single root. In
3964:
3939:
3920:
3898:
3873:
3760:
3747:
546:
521:
484:
68:. To the left of the matrices, are their
9174:Entries dealing with the Symmetric Group
8849:Representations of permutation groups. I
8780:
8747:Dixon, John D.; Mortimer, Brian (1996),
8429:
8388:"Symmetric Group is not Abelian/Proof 1"
8374:
8362:
8150:
8128:is an isomorphism for sufficiently high
6672:), and using this notation one has that
4849:, this corresponds to the fact that the
2057:, the symmetric groups, their elements (
48:
25:
8978:An Introduction to the Theory of Groups
8916:
8720:
8409:. Krishna Prakashan Media. p. 49.
8140:
8063:does not change the abelianization of S
7373:Alternating group § Group homology
6863:
5687:, as it lies between the simple group A
5664:
5623:is not the full automorphism group of A
4853:gives a direct solution to the general
4718:of permutations with cycles of lengths
3697:This is an involution, and consists of
3357:, but it could equally well be written
1691:
1672:{\displaystyle \operatorname {Sym} (n)}
1489:{\displaystyle \operatorname {Sym} (X)}
9181:
8965:
8846:
8640:
8620:
8167:representation theory of finite groups
6886:. For example, the Galois group of a (
6849:
6845:
6840:These calculations are attributed to (
6417:classification of finite simple groups
4677:{\textstyle n=\sum _{i=1}^{k}\mu _{i}}
3122:, that is, transpositions of the form
1354:is denoted in various ways, including
419:Classification of finite simple groups
9146:
9127:
9023:
9001:
8946:
8788:, vol. 1 (2nd ed.), Dover,
8578:
8444:
8241:of shape given by the Young diagram.
7700:
7576:The second homology (concretely, the
7304:is not trivial: it is isomorphic to C
7132:
6857:
6278:
6135:
5013:kernel corresponds to the use of the
3215:; it conventionally is required that
3142:= (4 5)(3 4)(4 5)(1 2)(2 3)(3 4)(4 5)
2113:Group properties and special elements
1992:The symmetric group on a set of size
1683:
1254:and whose group operation is that of
6848:, p. 176). Note however that (
6824:{ (1,3)(2,4), (1,2), (3,4), (5,6) }
6292:
6288:
5873:subject to the following relations:
5645:has no outer automorphisms, and for
5226:Unlike all other symmetric groups, S
4781:
4319:
3846: adjacent transpositions:
1560:then the name may be abbreviated to
1194:The symmetric group on a finite set
8237:acting on a space generated by the
7705:double cover of the symmetric group
7090:
7071:
6844:) and described in more detail in (
6818:, and so a Sylow 2-subgroup of the
6642:{\displaystyle 0\leq i,j,k,l\leq 2}
6151:
6128:-cycle of adjacent elements in the
5794:{\displaystyle \sigma _{i}=(i,i+1)}
5580:. Conjugation by even elements are
5562:is the full automorphism group of A
4201:
4194:
4158:
4151:
3724:{\displaystyle \lfloor n/2\rfloor }
3555:
2022:representation theory of Lie groups
1613:{\displaystyle {\mathfrak {S}}_{n}}
1599:
1407:{\displaystyle {\mathfrak {S}}_{X}}
1393:
1324:{\displaystyle X=\{1,2,\ldots ,n\}}
1124:representation theory of Lie groups
1028:) such permutation operations, the
41:using the generators (red) a right
13:
8074:theory: there is an inclusion map
8043:
8028:
7976:
7961:
7932:
7917:
7885:
7867:
7836:
7791:
7773:
7742:
7605:
7416:
6907:
6477:such subgroups simply by counting
6430:
6241:, called the alternating subgroup
5910:{\displaystyle \sigma _{i}^{2}=1,}
4814:are trivial, which corresponds to
4015:
4012:
4009:
3332:is a cycle of length three, since
3171:for which there exists an element
3137:from above can also be written as
2999:
1628:
1569:
1422:
1363:
1281:is the symmetric group on the set
1041:
914:
897:from the set to itself, and whose
64:. The elements are represented as
14:
9210:
9102:
7355:is inner, a result first due to (
6144:of a symmetric group is called a
5066:, namely the even transpositions
4308:elements and perfect shuffle on 2
3133:. For instance, the permutation
2187:for an explanation of notation):
2132:
1553:{\displaystyle \{1,2,\ldots ,n\}}
60:omitted, of the symmetric group S
16:Type of group in abstract algebra
8452:(2 ed.), Springer, p.
7656:
7619:
7520:which is the abelianization for
7467:
7430:
7142:
6421:Liebeck, Praeger & Saxl 1988
6296:
5693:and its group of automorphisms.
1791:is less than or equal to 2. For
1697:The symmetric group on a set of
1582:{\displaystyle \mathrm {S} _{n}}
1376:{\displaystyle \mathrm {S} _{X}}
1054:{\displaystyle \mathrm {S} _{n}}
927:{\displaystyle \mathrm {S} _{n}}
111:
81:
8954:(in German), Leipzig. Teubner,
8682:Schreier, J.; Ulam, S. (1933).
8675:
8633:
8614:
8599:
8572:
8481:Combinatorics of Coxeter groups
8403:"2. Groups S3 Group Definition"
5709:by appending the transposition
5447:Relation with alternating group
5090:of the quartic. The map from S
3379:(4 1 3)(2 5 6) = (2 5 6)(4 1 3)
3211:are the only elements moved by
2081:of symmetric groups are called
2008:and plays an important role in
1987:
1190:Definition and first properties
1162:of the symmetric group on (the
8536:
8500:
8469:
8438:
8423:
8394:
8380:
8368:
8256:equal to zero or greater than
8094:, the induced map on homology
8038:
7971:
7927:
7877:
7862:
7846:
7831:
7783:
7768:
7752:
7737:
7623:
7600:
7434:
7411:
7030:
6998:
6549:of two cyclic groups of order
6277:(1934). For more details see (
6059:
6029:
6000:
5986:
5788:
5770:
5609:exceptional outer automorphism
4567:
4555:
4552:
4534:
4528:
4516:
4510:
4498:
4495:
4477:
4471:
4459:
4266:
4256:
4205:
4195:
4162:
4152:
4103:
4091:
4084:
4074:
4048:
4038:
4032:
4019:
3974:
3952:
3949:
3927:
3924:
3914:
3908:
3886:
3883:
3867:
3835:
3823:
3770:
3754:
3751:
3741:
3731:(non-adjacent) transpositions
3526:
3520:
3512:
3506:
3498:
3492:
3009:
2766:
2748:
2745:
2727:
2715:
2678:
2646:th power, will decompose into
2536:
2524:
2521:
2503:
2368:
2356:
2353:
2335:
2239:
2227:
2224:
2218:
2215:
2203:
1666:
1660:
1483:
1477:
780:Infinite dimensional Lie group
1:
8713:
6894:is a transitive subgroup of S
6770: + ... +
5313:Maps between symmetric groups
2847:from above can be written as
1334:The symmetric group on a set
962:that can be performed on the
8586:, Pearson, Exercise 6.6.16,
8479:; Brenti, Francesco (2005),
8165:is a particular case of the
8007:extend to triple covers of S
6931:. They are all of the form
6822:of degree 7 is generated by
5747:letters is generated by the
5195:; the obvious inclusion map
4966:. This process is known as
4806:The symmetric groups on the
2786:Verification of group axioms
1851:), the symmetric groups are
553:{\displaystyle \mathbb {Z} }
528:{\displaystyle \mathbb {Z} }
491:{\displaystyle \mathbb {Z} }
7:
9115:Encyclopedia of Mathematics
8986:10.1007/978-1-4612-4176-8_7
8971:"Extensions and Cohomology"
8332:Symmetric inverse semigroup
8322:Generalized symmetric group
8301:
8208:irreducible representations
7366:
6524:affine general linear group
5317:Other than the trivial map
3571:order reversing permutation
2834:Transposition (mathematics)
1640:{\displaystyle \Sigma _{n}}
1434:{\displaystyle \Sigma _{X}}
278:List of group theory topics
10:
9215:
8731:Cambridge University Press
8721:Cameron, Peter J. (1999),
8521:10.1016/j.disc.2008.02.018
8295:are not known in general.
8154:
7999:and the triple covers of A
7703:), and corresponds to the
7370:
7136:
6911:
6686:is the wreath product of W
6013:{\displaystyle |i-j|>1}
5497:is the semidirect product
5015:discrete Fourier transform
4785:
2831:
1933:that shows that for every
18:
9043:10.1515/crll.1911.139.155
8910:10.1017/S144678870003216X
8847:Kerber, Adalbert (1971),
8610:, Transactions of the AMS
7361:Dixon & Mortimer 1996
6816:dihedral group of order 8
6425:Dixon & Mortimer 1996
6283:Dixon & Mortimer 1996
6269:(1929) and independently
5652:it has no center, so for
5522:(and thus themselves be A
5234:. Using the language of
4987:dihedral group of order 6
4822:, its only member is the
4752:is a conjugacy class of S
4304:Note that the reverse on
3151:
2851:= (1 2)(2 5)(3 4). Since
2183:)). Concretely, let (see
1688:Dixon & Mortimer 1996
982:symbols. Since there are
9199:Finite reflection groups
8803:Kaloujnine, Léo (1948),
8434:(PhD). Universität Kiel.
8377:, p. 32 Theorem 1.1
8342:
7357:Schreier & Ulam 1936
7288:outer automorphism group
7079:states that every group
6713:are a direct product of
5739:Generators and relations
5549:by conjugation, and for
5109:, which only occurs for
4709:, is associated the set
4316:, which are 8-periodic.
3416:generators and relations
3144:. The sorting algorithm
2863:is an even permutation.
1134:states that every group
958:symbols consists of the
903:composition of functions
396:Elementary abelian group
273:Glossary of group theory
19:Not to be confused with
9151:"Symmetric group graph"
9087:10.4064/fm-28-1-258-260
9074:Fundamenta Mathematicae
8567:10.54550/ECA2022V2S3R20
8313:History of group theory
7064:when it is viewed as a
6662:are sometimes denoted W
6565:= (1 4 7)(2 5 8)(3 6 9)
6403:is a proper divisor of
6251:characteristic subgroup
5749:adjacent transpositions
5743:The symmetric group on
4229:which is 4-periodic in
3407:{\displaystyle \sigma }
3119:adjacent transpositions
2819:Every bijection has an
1922:{\displaystyle n\leq 4}
1886:{\displaystyle 0!=1!=1}
34:of the symmetric group
8703:10.4064/sm-4-1-134-141
8551:(3), Proposition 2.1,
8318:Signed symmetric group
8173:theory to problems of
8057:
7993:
7946:
7902:
7808:
7699:This was computed in (
7690:
7501:
7037:
6985:
6705:In general, the Sylow
6643:
6553:. For instance, when
6081:
6014:
5972:
5911:
5858:
5795:
5702:can be embedded into A
5543:acts on its subgroup A
4772:
4678:
4663:
4586:Conjugacy classes of S
4577:
4446:
4296:
4220:
3987:
3853:
3810:
3725:
3654:
3546:
3408:
3377:there is the equality
3323:
3040:
2981:With this definition,
2972:
2776:
2615:
2447:
2315:
1977:
1953:
1952:{\displaystyle n>4}
1923:
1887:
1837:
1811:
1785:
1761:
1737:
1711:
1673:
1641:
1614:
1583:
1554:
1510:
1490:
1458:
1435:
1408:
1377:
1348:
1325:
1275:
1248:
1228:
1208:
1180:
1148:
1078:
1055:
1019:
999:
976:
952:
928:
812:Linear algebraic group
554:
529:
492:
88:
46:
8920:Annals of Mathematics
8430:Neubüser, J. (1967).
8184:The symmetric group S
8151:Representation theory
8058:
7994:
7947:
7903:
7809:
7691:
7502:
7347:In fact, for any set
7137:Further information:
7100:least common multiple
7038:
6986:
6826:and is isomorphic to
6644:
6407:and "wr" denotes the
6082:
6015:
5973:
5912:
5859:
5796:
5168:, and the fact that S
5043:permutations, of the
4773:
4679:
4643:
4578:
4447:
4297:
4221:
3998:so it thus has sign:
3988:
3854:
3784:
3726:
3655:
3575:is the one given by:
3547:
3409:
3324:
3097:elements. The group S
3041:
2973:
2777:
2616:
2448:
2316:
1978:
1954:
1924:
1888:
1838:
1812:
1786:
1762:
1738:
1712:
1674:
1642:
1615:
1584:
1555:
1511:
1491:
1459:
1436:
1409:
1378:
1349:
1326:
1276:
1249:
1229:
1209:
1181:
1149:
1079:
1056:
1020:
1000:
977:
953:
929:
555:
530:
493:
52:
29:
9002:Scott, W.R. (1987),
8647:Annali di Matematica
8483:, Springer, p.
8023:
8015:– but these are not
7956:
7912:
7818:
7724:
7587:
7398:
6995:
6935:
6864:Transitive subgroups
6603:
6485:therefore has order
6461:-cycles. There are
6026:
5982:
5922:
5880:
5816:
5754:
5292:have an exceptional
5133:special linear group
4991:equilateral triangle
4855:quadratic polynomial
4771:{\displaystyle \mu }
4762:
4634:
4456:
4364:
4253:
4005:
3864:
3738:
3701:
3582:
3425:
3398:
3381:. Every element of S
3244:
2988:
2881:
2675:
2479:
2326:
2194:
2163:", maps any element
2051:general linear group
1967:
1937:
1931:Abel–Ruffini theorem
1907:
1859:
1821:
1795:
1775:
1751:
1724:
1701:
1651:
1624:
1593:
1564:
1520:
1500:
1468:
1445:
1418:
1387:
1358:
1338:
1285:
1265:
1256:function composition
1238:
1218:
1198:
1170:
1138:
1065:
1036:
1009:
986:
966:
942:
909:
542:
517:
480:
8641:Onofri, L. (1929).
8623:Bollettino Mathesis
8450:The Symmetric Group
8179:identical particles
7053:parabolic subgroups
6870:transitive subgroup
6413:O'Nan–Scott theorem
6188:and nontrivial for
5897:
5685:almost simple group
5582:inner automorphisms
5414:as discussed above.
5240:Lagrange resolvents
5193:transitive subgroup
5178:quintic polynomials
5102:of dimension below
5088:Lagrange resolvents
5047:. Beyond the group
5019:Lagrange resolvents
5005:, as discovered by
4600:: to the partition
3373:: for example, in S
2095:automorphism groups
2018:symmetric functions
1836:{\displaystyle n=1}
1810:{\displaystyle n=0}
1105:automorphism groups
1097:finite presentation
186:Group homomorphisms
96:Algebraic structure
9189:Permutation groups
9148:Weisstein, Eric W.
9129:Weisstein, Eric W.
9010:, pp. 45–46,
9008:Dover Publications
8861:10.1007/BFb0067943
8824:10.24033/asens.961
8751:Permutation groups
8725:Permutation Groups
8660:10.1007/BF02409971
8235:Young symmetrizers
8171:symmetric function
8053:
7989:
7942:
7898:
7804:
7686:
7681:
7497:
7492:
7290:are both trivial.
7133:Automorphism group
7033:
6981:
6639:
6496:and is known as a
6308:. You can help by
6136:Subgroup structure
6077:
6010:
5968:
5907:
5883:
5854:
5812:. The collection
5791:
5728:is impossible for
5665:automorphism group
5663:, as discussed in
5592:outer automorphism
5353:relative dimension
5232:outer automorphism
5080:quartic polynomial
4768:
4674:
4594:integer partitions
4573:
4442:
4433:
4344:are conjugate in S
4334:correspond to the
4292:
4216:
4211:
3983:
3849:
3721:
3650:
3641:
3542:
3536:
3459:
3404:
3319:
3313:
3105:semidirect product
3051:group homomorphism
3036:
2968:
2963:
2928:
2874:of a permutation:
2772:
2611:
2602:
2443:
2434:
2311:
2305:
2091:homogeneous spaces
2083:permutation groups
2045:and occurs as the
1973:
1949:
1919:
1883:
1833:
1807:
1781:
1757:
1736:{\displaystyle n!}
1733:
1707:
1669:
1637:
1610:
1579:
1550:
1506:
1486:
1457:{\displaystyle X!}
1454:
1431:
1404:
1373:
1344:
1321:
1271:
1244:
1224:
1204:
1176:
1144:
1077:{\displaystyle n!}
1074:
1051:
1015:
998:{\displaystyle n!}
995:
972:
948:
924:
662:Special orthogonal
550:
525:
488:
369:Lagrange's theorem
89:
47:
9167:(video of a talk)
9132:"Symmetric group"
9110:"Symmetric group"
9017:978-0-486-65377-8
8995:978-1-4612-8686-8
8967:Rotman, Joseph J.
8870:978-3-540-05693-5
8795:978-0-486-47189-1
8766:978-0-387-94599-6
8740:978-0-521-65378-7
8593:978-0-13-004763-2
8494:978-3-540-27596-1
8463:978-0-387-95067-9
8262:Maschke's theorem
8196:conjugacy classes
8175:quantum mechanics
7263:
7262:
7127:Landau's function
7045:integer partition
6567:and the elements
6330:maximal subgroups
6326:
6325:
6289:Maximal subgroups
6175:alternating group
6146:permutation group
5460:alternating group
5338:and the sign map
5284:Note that while A
5140:icosahedral group
4851:quadratic formula
4782:Low degree groups
4563:
4548:
4542:
4524:
4506:
4491:
4485:
4467:
4326:conjugacy classes
4320:Conjugacy classes
4314:Clifford algebras
3847:
3842:
3782:
3167:is a permutation
3064:alternating group
3035:
2956:
2948:
2927:
2918:
2762:
2756:
2741:
2735:
2710:
2704:
2698:
2692:
2686:
2650:cycles of length
2532:
2517:
2511:
2364:
2349:
2343:
2235:
2211:
2107:Higman–Sims graph
2103:Higman–Sims group
2035:In the theory of
1976:{\displaystyle n}
1855:(they have order
1784:{\displaystyle n}
1760:{\displaystyle n}
1710:{\displaystyle n}
1509:{\displaystyle X}
1347:{\displaystyle X}
1274:{\displaystyle n}
1247:{\displaystyle X}
1227:{\displaystyle X}
1207:{\displaystyle X}
1179:{\displaystyle G}
1147:{\displaystyle G}
1093:conjugacy classes
1018:{\displaystyle n}
975:{\displaystyle n}
951:{\displaystyle n}
881:defined over any
871:
870:
446:
445:
328:Alternating group
285:
284:
9206:
9161:
9160:
9142:
9141:
9123:
9097:
9070:
9053:
9037:(139): 155–250,
9020:
8998:
8975:
8962:
8943:
8913:
8912:
8881:
8843:
8826:
8798:
8782:Jacobson, Nathan
8777:
8754:
8743:
8728:
8707:
8706:
8688:
8679:
8673:
8672:
8662:
8637:
8631:
8630:
8618:
8612:
8611:
8603:
8597:
8596:
8576:
8570:
8569:
8560:
8540:
8534:
8533:
8532:
8504:
8498:
8497:
8485:4. Example 1.2.3
8473:
8467:
8466:
8442:
8436:
8435:
8427:
8421:
8420:
8398:
8392:
8391:
8384:
8378:
8372:
8366:
8360:
8222:or equivalently
8177:for a number of
8127:
8090:, and for fixed
8089:
8062:
8060:
8059:
8054:
8052:
8051:
8046:
8037:
8036:
8031:
7998:
7996:
7995:
7990:
7985:
7984:
7979:
7970:
7969:
7964:
7951:
7949:
7948:
7943:
7941:
7940:
7935:
7926:
7925:
7920:
7907:
7905:
7904:
7899:
7894:
7893:
7888:
7876:
7875:
7870:
7861:
7860:
7845:
7844:
7839:
7830:
7829:
7813:
7811:
7810:
7805:
7800:
7799:
7794:
7782:
7781:
7776:
7767:
7766:
7751:
7750:
7745:
7736:
7735:
7695:
7693:
7692:
7687:
7685:
7684:
7664:
7659:
7622:
7614:
7613:
7608:
7599:
7598:
7578:Schur multiplier
7566:
7548:
7506:
7504:
7503:
7498:
7496:
7495:
7475:
7470:
7433:
7425:
7424:
7419:
7410:
7409:
7363:, p. 259).
7359:) according to (
7343:
7328:
7317:
7299:
7271:
7143:
7091:Cyclic subgroups
7077:Cayley's theorem
7072:Cayley's theorem
7066:reflection group
7063:
7050:
7042:
7040:
7039:
7034:
7029:
7028:
7010:
7009:
6990:
6988:
6987:
6982:
6980:
6979:
6978:
6977:
6954:
6953:
6952:
6951:
6926:
6892:Galois extension
6836:
6825:
6813:
6783:
6746:
6685:
6650:
6648:
6646:
6645:
6640:
6596:
6581:
6566:
6559:
6533:
6521:
6515:(especially for
6514:
6495:
6476:
6427:, p. 268).
6402:
6390:
6371:
6359:
6340:
6321:
6318:
6300:
6293:
6281:, Ch. 11.3) or (
6220:Klein four group
6217:
6210:
6201:
6194:
6187:
6166:
6158:normal subgroups
6152:Normal subgroups
6131:
6127:
6123:
6119:
6108:
6104:
6096:reflection group
6086:
6084:
6083:
6078:
6067:
6066:
6057:
6056:
6041:
6040:
6019:
6017:
6016:
6011:
6003:
5989:
5977:
5975:
5974:
5969:
5967:
5966:
5957:
5956:
5944:
5943:
5934:
5933:
5916:
5914:
5913:
5908:
5896:
5891:
5872:
5863:
5861:
5860:
5855:
5853:
5852:
5828:
5827:
5811:
5804:
5800:
5798:
5797:
5792:
5766:
5765:
5746:
5734:
5720:
5676:
5658:
5651:
5638:
5632:Conversely, for
5606:
5555:
5509:
5490:
5457:
5442:
5432:
5409:
5392:
5379:
5368:
5350:
5337:
5294:Schur multiplier
5268:
5252:
5210:
5190:
5166:quintic equation
5151:
5137:
5115:
5108:
5084:Lodovico Ferrari
5069:
5060:Klein four-group
5007:Gerolamo Cardano
5003:cubic polynomial
4965:
4945:, one gets that
4944:
4902:
4859:invariant theory
4817:
4777:
4775:
4774:
4769:
4742:
4708:
4683:
4681:
4680:
4675:
4673:
4672:
4662:
4657:
4629:
4582:
4580:
4579:
4574:
4561:
4546:
4540:
4522:
4504:
4489:
4483:
4465:
4451:
4449:
4448:
4443:
4438:
4437:
4301:
4299:
4298:
4293:
4288:
4287:
4280:
4225:
4223:
4222:
4217:
4215:
4214:
4208:
4165:
4115:
4114:
4110:
4070:
4069:
4062:
4031:
4030:
4018:
3992:
3990:
3989:
3984:
3858:
3856:
3855:
3850:
3848:
3845:
3843:
3838:
3818:
3809:
3798:
3783:
3780:
3730:
3728:
3727:
3722:
3714:
3693:
3681:
3659:
3657:
3656:
3651:
3646:
3645:
3573:
3572:
3556:Special elements
3551:
3549:
3548:
3543:
3541:
3540:
3477:
3476:
3464:
3463:
3413:
3411:
3410:
3405:
3380:
3364:
3360:
3356:
3352:
3345:
3338:
3328:
3326:
3325:
3320:
3318:
3317:
3228:
3221:
3143:
3132:
3096:
3089:
3045:
3043:
3042:
3037:
3033:
3008:
3007:
3002:
2977:
2975:
2974:
2969:
2967:
2966:
2957:
2954:
2949:
2946:
2929:
2925:
2919:
2916:
2821:inverse function
2781:
2779:
2778:
2773:
2760:
2754:
2739:
2733:
2723:
2722:
2708:
2702:
2696:
2690:
2684:
2667:
2660:
2654:: For example, (
2641:
2620:
2618:
2617:
2612:
2607:
2606:
2530:
2515:
2509:
2452:
2450:
2449:
2444:
2439:
2438:
2362:
2347:
2341:
2320:
2318:
2317:
2312:
2310:
2309:
2233:
2209:
2147:of permutations
2146:
2014:invariant theory
1982:
1980:
1979:
1974:
1958:
1956:
1955:
1950:
1928:
1926:
1925:
1920:
1892:
1890:
1889:
1884:
1842:
1840:
1839:
1834:
1816:
1814:
1813:
1808:
1790:
1788:
1787:
1782:
1766:
1764:
1763:
1758:
1742:
1740:
1739:
1734:
1716:
1714:
1713:
1708:
1678:
1676:
1675:
1670:
1646:
1644:
1643:
1638:
1636:
1635:
1619:
1617:
1616:
1611:
1609:
1608:
1603:
1602:
1588:
1586:
1585:
1580:
1578:
1577:
1572:
1559:
1557:
1556:
1551:
1515:
1513:
1512:
1507:
1495:
1493:
1492:
1487:
1463:
1461:
1460:
1455:
1440:
1438:
1437:
1432:
1430:
1429:
1413:
1411:
1410:
1405:
1403:
1402:
1397:
1396:
1382:
1380:
1379:
1374:
1372:
1371:
1366:
1353:
1351:
1350:
1345:
1330:
1328:
1327:
1322:
1280:
1278:
1277:
1272:
1253:
1251:
1250:
1245:
1233:
1231:
1230:
1225:
1213:
1211:
1210:
1205:
1185:
1183:
1182:
1177:
1153:
1151:
1150:
1145:
1132:Cayley's theorem
1120:invariant theory
1083:
1081:
1080:
1075:
1060:
1058:
1057:
1052:
1050:
1049:
1044:
1024:
1022:
1021:
1016:
1004:
1002:
1001:
996:
981:
979:
978:
973:
957:
955:
954:
949:
933:
931:
930:
925:
923:
922:
917:
875:abstract algebra
863:
856:
849:
805:Algebraic groups
578:Hyperbolic group
568:Arithmetic group
559:
557:
556:
551:
549:
534:
532:
531:
526:
524:
497:
495:
494:
489:
487:
410:Schur multiplier
364:Cauchy's theorem
352:Quaternion group
300:
299:
126:
125:
115:
102:
91:
90:
85:
9214:
9213:
9209:
9208:
9207:
9205:
9204:
9203:
9179:
9178:
9108:
9105:
9100:
9068:
9062:Ulam, Stanislaw
9058:Schreier, Józef
9018:
8996:
8973:
8933:10.2307/1970333
8885:Liebeck, M.W.;
8871:
8853:Springer-Verlag
8796:
8767:
8757:Springer-Verlag
8741:
8716:
8711:
8710:
8686:
8680:
8676:
8639:§141, p.124 in
8638:
8634:
8619:
8615:
8604:
8600:
8594:
8577:
8573:
8541:
8537:
8505:
8501:
8495:
8477:Björner, Anders
8474:
8470:
8464:
8446:Sagan, Bruce E.
8443:
8439:
8428:
8424:
8417:
8399:
8395:
8386:
8385:
8381:
8373:
8369:
8361:
8350:
8345:
8337:Symmetric power
8304:
8276:
8212:complex numbers
8198:are labeled by
8189:
8159:
8153:
8125:
8118:
8109:
8103:
8095:
8088:
8081:
8075:
8072:stable homotopy
8066:
8047:
8042:
8041:
8032:
8027:
8026:
8024:
8021:
8020:
8014:
8010:
8006:
8002:
7980:
7975:
7974:
7965:
7960:
7959:
7957:
7954:
7953:
7936:
7931:
7930:
7921:
7916:
7915:
7913:
7910:
7909:
7889:
7884:
7883:
7871:
7866:
7865:
7856:
7852:
7840:
7835:
7834:
7825:
7821:
7819:
7816:
7815:
7795:
7790:
7789:
7777:
7772:
7771:
7762:
7758:
7746:
7741:
7740:
7731:
7727:
7725:
7722:
7721:
7712:
7680:
7679:
7668:
7660:
7655:
7652:
7651:
7640:
7630:
7629:
7618:
7609:
7604:
7603:
7594:
7590:
7588:
7585:
7584:
7572:
7564:
7560:
7554:
7552:
7547:
7541:
7535:
7533:
7519:
7515:
7491:
7490:
7479:
7471:
7466:
7463:
7462:
7451:
7441:
7440:
7429:
7420:
7415:
7414:
7405:
7401:
7399:
7396:
7395:
7386:
7375:
7369:
7342:
7338:
7334:
7330:
7327:
7323:
7319:
7312:
7307:
7303:
7294:
7277:
7266:
7259:
7253:
7247:
7243:
7229:
7223:
7217:
7203:
7197:
7191:
7174:
7165:
7156:
7141:
7135:
7124:
7118:
7114:
7109:
7105:
7093:
7074:
7062:
7056:
7048:
7024:
7020:
7005:
7001:
6996:
6993:
6992:
6973:
6969:
6968:
6964:
6947:
6943:
6942:
6938:
6936:
6933:
6932:
6925:
6919:
6916:
6910:
6908:Young subgroups
6899:
6877:
6866:
6842:Kaloujnine 1948
6835:
6831:
6827:
6823:
6820:symmetric group
6812:
6808:
6804:
6800:
6796:
6782:
6769:
6758:
6748:
6739:
6733:
6727:
6721:
6701:
6691:
6679:
6673:
6667:
6604:
6601:
6600:
6598:
6583:
6568:
6561:
6554:
6527:
6516:
6513:
6500:
6498:Frobenius group
6486:
6462:
6437:Sylow subgroups
6433:
6431:Sylow subgroups
6392:
6389:
6379:
6373:
6361:
6358:
6348:
6342:
6339:
6333:
6322:
6316:
6313:
6306:needs expansion
6291:
6212:
6209:
6203:
6196:
6189:
6182:
6172:
6161:
6154:
6138:
6129:
6125:
6121:
6110:
6106:
6102:
6094:(and so also a
6062:
6058:
6046:
6042:
6036:
6032:
6027:
6024:
6023:
5999:
5985:
5983:
5980:
5979:
5962:
5958:
5952:
5948:
5939:
5935:
5929:
5925:
5923:
5920:
5919:
5892:
5887:
5881:
5878:
5877:
5871:
5865:
5842:
5838:
5823:
5819:
5817:
5814:
5813:
5806:
5802:
5761:
5757:
5755:
5752:
5751:
5744:
5741:
5729:
5727:
5710:
5708:
5701:
5692:
5682:
5671:
5653:
5646:
5644:
5633:
5628:
5622:
5616:
5601:
5599:
5589:
5579:
5573:
5567:
5561:
5550:
5548:
5542:
5533:
5527:
5521:
5515:
5508:
5504:
5498:
5496:
5489:
5485:
5479:
5473:
5467:
5452:
5449:
5434:
5431:
5425:
5419:
5413:
5408:
5404:
5400:
5396:
5391:
5387:
5383:
5378:
5374:
5370:
5367:
5363:
5359:
5349:
5345:
5339:
5336:
5332:
5328:
5324:
5318:
5315:
5307:
5303:
5291:
5287:
5272:
5267:
5260:
5254:
5251:
5247:
5243:
5229:
5223:
5214:
5209:
5202:
5196:
5189:
5185:
5181:
5171:
5164:of the general
5159:
5155:
5150:
5146:
5142:
5135:
5130:
5124:
5110:
5103:
5097:
5093:
5073:
5070:with quotient S
5067:
5064:normal subgroup
5057:
5052:
5037:
5029:
5012:
5000:
4996:
4984:
4978:
4964:
4957:
4946:
4910:
4904:
4868:
4862:
4834:
4821:
4815:
4803:
4799:
4790:
4784:
4763:
4760:
4759:
4757:
4751:
4741:
4732:
4725:
4719:
4717:
4707:
4698:
4691:
4685:
4668:
4664:
4658:
4647:
4635:
4632:
4631:
4627:
4618:
4611:
4601:
4591:
4457:
4454:
4453:
4432:
4431:
4426:
4421:
4416:
4411:
4405:
4404:
4399:
4394:
4389:
4384:
4374:
4373:
4365:
4362:
4361:
4359:
4353:
4349:
4343:
4333:
4322:
4276:
4269:
4265:
4254:
4251:
4250:
4246:perfect shuffle
4242:
4210:
4209:
4193:
4176:
4167:
4166:
4150:
4133:
4120:
4119:
4106:
4087:
4083:
4058:
4051:
4047:
4026:
4022:
4008:
4006:
4003:
4002:
3865:
3862:
3861:
3844:
3819:
3817:
3799:
3788:
3779:
3739:
3736:
3735:
3710:
3702:
3699:
3698:
3683:
3672:
3669:longest element
3640:
3639:
3634:
3629:
3618:
3612:
3611:
3606:
3601:
3596:
3586:
3585:
3583:
3580:
3579:
3570:
3569:
3558:
3535:
3534:
3529:
3515:
3501:
3482:
3481:
3469:
3465:
3458:
3457:
3452:
3447:
3442:
3432:
3431:
3426:
3423:
3422:
3399:
3396:
3395:
3386:
3378:
3376:
3362:
3358:
3354:
3347:
3340:
3333:
3312:
3311:
3306:
3301:
3296:
3291:
3285:
3284:
3279:
3274:
3269:
3264:
3254:
3253:
3245:
3242:
3241:
3223:
3216:
3154:
3138:
3123:
3112:
3102:
3091:
3084:
3082:
3075:normal subgroup
3072:
3055:neutral element
3003:
2998:
2997:
2989:
2986:
2985:
2962:
2961:
2953:
2945:
2943:
2931:
2930:
2923:
2915:
2913:
2897:
2896:
2882:
2879:
2878:
2857:odd permutation
2836:
2830:
2788:
2718:
2714:
2676:
2673:
2672:
2662:
2655:
2642:, taken to the
2629:
2601:
2600:
2595:
2590:
2585:
2580:
2574:
2573:
2568:
2563:
2558:
2553:
2543:
2542:
2480:
2477:
2476:
2433:
2432:
2427:
2422:
2417:
2412:
2406:
2405:
2400:
2395:
2390:
2385:
2375:
2374:
2327:
2324:
2323:
2304:
2303:
2298:
2293:
2288:
2283:
2277:
2276:
2271:
2266:
2261:
2256:
2246:
2245:
2195:
2192:
2191:
2138:
2135:
2115:
2071:plactic monoids
2063:representations
2044:
2000:of the general
1990:
1968:
1965:
1964:
1938:
1935:
1934:
1908:
1905:
1904:
1903:if and only if
1898:
1860:
1857:
1856:
1822:
1819:
1818:
1796:
1793:
1792:
1776:
1773:
1772:
1771:if and only if
1752:
1749:
1748:
1725:
1722:
1721:
1702:
1699:
1698:
1690:, Ch. 8), and (
1652:
1649:
1648:
1631:
1627:
1625:
1622:
1621:
1604:
1598:
1597:
1596:
1594:
1591:
1590:
1573:
1568:
1567:
1565:
1562:
1561:
1521:
1518:
1517:
1501:
1498:
1497:
1469:
1466:
1465:
1446:
1443:
1442:
1425:
1421:
1419:
1416:
1415:
1398:
1392:
1391:
1390:
1388:
1385:
1384:
1367:
1362:
1361:
1359:
1356:
1355:
1339:
1336:
1335:
1286:
1283:
1282:
1266:
1263:
1262:
1239:
1236:
1235:
1219:
1216:
1215:
1199:
1196:
1195:
1192:
1171:
1168:
1167:
1139:
1136:
1135:
1066:
1063:
1062:
1045:
1040:
1039:
1037:
1034:
1033:
1010:
1007:
1006:
987:
984:
983:
967:
964:
963:
943:
940:
939:
934:defined over a
918:
913:
912:
910:
907:
906:
899:group operation
879:symmetric group
867:
838:
837:
826:Abelian variety
819:Reductive group
807:
797:
796:
795:
794:
745:
737:
729:
721:
713:
686:Special unitary
597:
583:
582:
564:
563:
545:
543:
540:
539:
520:
518:
515:
514:
483:
481:
478:
477:
469:
468:
459:Discrete groups
448:
447:
403:Frobenius group
348:
335:
324:
317:Symmetric group
313:
297:
287:
286:
137:Normal subgroup
123:
103:
94:
86:
80:
78:
77:
63:
39:
24:
17:
12:
11:
5:
9212:
9202:
9201:
9196:
9191:
9177:
9176:
9168:
9162:
9143:
9124:
9104:
9103:External links
9101:
9099:
9098:
9054:
9021:
9016:
8999:
8994:
8963:
8944:
8927:(2): 229–257,
8914:
8903:(3): 389–396,
8882:
8869:
8844:
8800:
8794:
8778:
8765:
8744:
8739:
8717:
8715:
8712:
8709:
8708:
8697:(1): 134–141.
8674:
8653:(1): 103–130.
8632:
8613:
8598:
8592:
8580:Artin, Michael
8571:
8535:
8509:Discrete Math.
8499:
8493:
8468:
8462:
8437:
8422:
8415:
8407:Modern Algebra
8393:
8379:
8367:
8347:
8346:
8344:
8341:
8340:
8339:
8334:
8329:
8324:
8315:
8310:
8303:
8300:
8288:Specht modules
8272:
8254:characteristic
8239:Young tableaux
8224:Young diagrams
8185:
8155:Main article:
8152:
8149:
8120:
8114:
8105:
8099:
8083:
8077:
8064:
8050:
8045:
8040:
8035:
8030:
8012:
8008:
8004:
8000:
7988:
7983:
7978:
7973:
7968:
7963:
7939:
7934:
7929:
7924:
7919:
7897:
7892:
7887:
7882:
7879:
7874:
7869:
7864:
7859:
7855:
7851:
7848:
7843:
7838:
7833:
7828:
7824:
7803:
7798:
7793:
7788:
7785:
7780:
7775:
7770:
7765:
7761:
7757:
7754:
7749:
7744:
7739:
7734:
7730:
7716:Note that the
7708:
7697:
7696:
7683:
7678:
7675:
7672:
7669:
7667:
7663:
7658:
7654:
7653:
7650:
7647:
7644:
7641:
7639:
7636:
7635:
7633:
7628:
7625:
7621:
7617:
7612:
7607:
7602:
7597:
7593:
7568:
7562:
7556:
7550:
7543:
7537:
7529:
7517:
7511:
7508:
7507:
7494:
7489:
7486:
7483:
7480:
7478:
7474:
7469:
7465:
7464:
7461:
7458:
7455:
7452:
7450:
7447:
7446:
7444:
7439:
7436:
7432:
7428:
7423:
7418:
7413:
7408:
7404:
7389:abelianization
7382:
7379:group homology
7368:
7365:
7340:
7336:
7332:
7325:
7321:
7305:
7301:
7280:complete group
7273:
7261:
7260:
7257:
7254:
7251:
7248:
7245:
7241:
7238:
7231:
7230:
7227:
7224:
7221:
7218:
7215:
7212:
7205:
7204:
7201:
7198:
7195:
7192:
7187:
7184:
7177:
7176:
7170:
7167:
7161:
7158:
7152:
7149:
7134:
7131:
7125:) is given by
7120:
7116:
7112:
7107:
7103:
7092:
7089:
7073:
7070:
7058:
7032:
7027:
7023:
7019:
7016:
7013:
7008:
7004:
7000:
6976:
6972:
6967:
6963:
6960:
6957:
6950:
6946:
6941:
6929:Young subgroup
6921:
6918:A subgroup of
6914:Young subgroup
6912:Main article:
6909:
6906:
6895:
6873:
6865:
6862:
6833:
6829:
6810:
6806:
6802:
6798:
6795:For instance,
6778:
6767:
6756:
6737:
6723:
6717:
6697:
6687:
6675:
6663:
6638:
6635:
6632:
6629:
6626:
6623:
6620:
6617:
6614:
6611:
6608:
6547:wreath product
6522:), and is the
6504:
6432:
6429:
6409:wreath product
6381:
6375:
6350:
6344:
6335:
6324:
6323:
6317:September 2009
6303:
6301:
6290:
6287:
6211:, except when
6205:
6168:
6153:
6150:
6137:
6134:
6088:
6087:
6076:
6073:
6070:
6065:
6061:
6055:
6052:
6049:
6045:
6039:
6035:
6031:
6021:
6009:
6006:
6002:
5998:
5995:
5992:
5988:
5965:
5961:
5955:
5951:
5947:
5942:
5938:
5932:
5928:
5917:
5906:
5903:
5900:
5895:
5890:
5886:
5867:
5851:
5848:
5845:
5841:
5837:
5834:
5831:
5826:
5822:
5790:
5787:
5784:
5781:
5778:
5775:
5772:
5769:
5764:
5760:
5740:
5737:
5722:
5703:
5697:
5688:
5678:
5661:complete group
5640:
5624:
5618:
5612:
5607:, there is an
5595:
5585:
5575:
5569:
5563:
5557:
5544:
5538:
5529:
5523:
5517:
5511:
5506:
5500:
5492:
5487:
5481:
5475:
5463:
5448:
5445:
5427:
5421:
5416:
5415:
5411:
5406:
5402:
5398:
5394:
5389:
5385:
5381:
5376:
5372:
5365:
5361:
5347:
5341:
5334:
5330:
5326:
5320:
5314:
5311:
5310:
5309:
5305:
5301:
5289:
5285:
5281:
5280:
5270:
5262:
5256:
5249:
5245:
5227:
5224:
5221:
5217:
5216:
5212:
5204:
5198:
5187:
5183:
5174:solvable group
5169:
5157:
5153:
5148:
5144:
5128:
5125:
5122:
5118:
5117:
5095:
5091:
5071:
5062:V as a proper
5055:
5050:
5035:
5030:
5027:
5023:
5022:
5010:
4998:
4994:
4982:
4979:
4976:
4972:
4971:
4968:symmetrization
4962:
4955:
4908:
4866:
4835:
4832:
4828:
4827:
4824:empty function
4819:
4804:
4801:
4797:
4783:
4780:
4767:
4753:
4747:
4737:
4730:
4723:
4713:
4703:
4696:
4689:
4671:
4667:
4661:
4656:
4653:
4650:
4646:
4642:
4639:
4623:
4616:
4609:
4592:correspond to
4587:
4572:
4569:
4566:
4560:
4557:
4554:
4551:
4545:
4539:
4536:
4533:
4530:
4527:
4521:
4518:
4515:
4512:
4509:
4503:
4500:
4497:
4494:
4488:
4482:
4479:
4476:
4473:
4470:
4464:
4461:
4441:
4436:
4430:
4427:
4425:
4422:
4420:
4417:
4415:
4412:
4410:
4407:
4406:
4403:
4400:
4398:
4395:
4393:
4390:
4388:
4385:
4383:
4380:
4379:
4377:
4372:
4369:
4355:
4351:
4345:
4339:
4329:
4321:
4318:
4291:
4286:
4283:
4279:
4275:
4272:
4268:
4264:
4261:
4258:
4237:
4227:
4226:
4213:
4207:
4204:
4200:
4197:
4192:
4189:
4186:
4183:
4180:
4177:
4175:
4172:
4169:
4168:
4164:
4161:
4157:
4154:
4149:
4146:
4143:
4140:
4137:
4134:
4132:
4129:
4126:
4125:
4123:
4118:
4113:
4109:
4105:
4102:
4099:
4096:
4093:
4090:
4086:
4082:
4079:
4076:
4073:
4068:
4065:
4061:
4057:
4054:
4050:
4046:
4043:
4040:
4037:
4034:
4029:
4025:
4021:
4017:
4014:
4011:
3996:
3995:
3994:
3993:
3982:
3979:
3976:
3973:
3970:
3967:
3963:
3960:
3957:
3954:
3951:
3948:
3945:
3942:
3938:
3935:
3932:
3929:
3926:
3923:
3919:
3916:
3913:
3910:
3907:
3904:
3901:
3897:
3894:
3891:
3888:
3885:
3882:
3879:
3876:
3872:
3869:
3841:
3837:
3834:
3831:
3828:
3825:
3822:
3816:
3813:
3808:
3805:
3802:
3797:
3794:
3791:
3787:
3781: or
3778:
3775:
3772:
3769:
3766:
3763:
3759:
3756:
3753:
3750:
3746:
3743:
3720:
3717:
3713:
3709:
3706:
3661:
3660:
3649:
3644:
3638:
3635:
3633:
3630:
3628:
3625:
3622:
3619:
3617:
3614:
3613:
3610:
3607:
3605:
3602:
3600:
3597:
3595:
3592:
3591:
3589:
3557:
3554:
3553:
3552:
3539:
3533:
3530:
3528:
3525:
3522:
3519:
3516:
3514:
3511:
3508:
3505:
3502:
3500:
3497:
3494:
3491:
3488:
3487:
3485:
3480:
3475:
3472:
3468:
3462:
3456:
3453:
3451:
3448:
3446:
3443:
3441:
3438:
3437:
3435:
3430:
3403:
3382:
3374:
3330:
3329:
3316:
3310:
3307:
3305:
3302:
3300:
3297:
3295:
3292:
3290:
3287:
3286:
3283:
3280:
3278:
3275:
3273:
3270:
3268:
3265:
3263:
3260:
3259:
3257:
3252:
3249:
3153:
3150:
3108:
3098:
3078:
3068:
3047:
3046:
3032:
3029:
3026:
3023:
3020:
3017:
3014:
3011:
3006:
3001:
2996:
2993:
2979:
2978:
2965:
2960:
2952:
2944:
2942:
2939:
2936:
2933:
2932:
2922:
2914:
2912:
2909:
2906:
2903:
2902:
2900:
2895:
2892:
2889:
2886:
2832:Main article:
2829:
2826:
2825:
2824:
2817:
2810:
2807:
2787:
2784:
2783:
2782:
2771:
2768:
2765:
2759:
2753:
2750:
2747:
2744:
2738:
2732:
2729:
2726:
2721:
2717:
2713:
2707:
2701:
2695:
2689:
2683:
2680:
2622:
2621:
2610:
2605:
2599:
2596:
2594:
2591:
2589:
2586:
2584:
2581:
2579:
2576:
2575:
2572:
2569:
2567:
2564:
2562:
2559:
2557:
2554:
2552:
2549:
2548:
2546:
2541:
2538:
2535:
2529:
2526:
2523:
2520:
2514:
2508:
2505:
2502:
2499:
2496:
2493:
2490:
2487:
2484:
2454:
2453:
2442:
2437:
2431:
2428:
2426:
2423:
2421:
2418:
2416:
2413:
2411:
2408:
2407:
2404:
2401:
2399:
2396:
2394:
2391:
2389:
2386:
2384:
2381:
2380:
2378:
2373:
2370:
2367:
2361:
2358:
2355:
2352:
2346:
2340:
2337:
2334:
2331:
2321:
2308:
2302:
2299:
2297:
2294:
2292:
2289:
2287:
2284:
2282:
2279:
2278:
2275:
2272:
2270:
2267:
2265:
2262:
2260:
2257:
2255:
2252:
2251:
2249:
2244:
2241:
2238:
2232:
2229:
2226:
2223:
2220:
2217:
2214:
2208:
2205:
2202:
2199:
2155:, pronounced "
2134:
2133:Multiplication
2131:
2114:
2111:
2101:, such as the
2067:Young tableaux
2040:
2037:Coxeter groups
2030:Schur functors
1989:
1986:
1972:
1948:
1945:
1942:
1918:
1915:
1912:
1894:
1893:). The group S
1882:
1879:
1876:
1873:
1870:
1867:
1864:
1832:
1829:
1826:
1806:
1803:
1800:
1780:
1756:
1732:
1729:
1706:
1668:
1665:
1662:
1659:
1656:
1634:
1630:
1607:
1601:
1576:
1571:
1549:
1546:
1543:
1540:
1537:
1534:
1531:
1528:
1525:
1505:
1485:
1482:
1479:
1476:
1473:
1453:
1450:
1428:
1424:
1401:
1395:
1370:
1365:
1343:
1320:
1317:
1314:
1311:
1308:
1305:
1302:
1299:
1296:
1293:
1290:
1270:
1243:
1223:
1203:
1191:
1188:
1175:
1164:underlying set
1143:
1109:representation
1073:
1070:
1048:
1043:
1014:
994:
991:
971:
947:
921:
916:
869:
868:
866:
865:
858:
851:
843:
840:
839:
836:
835:
833:Elliptic curve
829:
828:
822:
821:
815:
814:
808:
803:
802:
799:
798:
793:
792:
789:
786:
782:
778:
777:
776:
771:
769:Diffeomorphism
765:
764:
759:
754:
748:
747:
743:
739:
735:
731:
727:
723:
719:
715:
711:
706:
705:
694:
693:
682:
681:
670:
669:
658:
657:
646:
645:
634:
633:
626:Special linear
622:
621:
614:General linear
610:
609:
604:
598:
589:
588:
585:
584:
581:
580:
575:
570:
562:
561:
548:
536:
523:
510:
508:Modular groups
506:
505:
504:
499:
486:
470:
467:
466:
461:
455:
454:
453:
450:
449:
444:
443:
442:
441:
436:
431:
428:
422:
421:
415:
414:
413:
412:
406:
405:
399:
398:
393:
384:
383:
381:Hall's theorem
378:
376:Sylow theorems
372:
371:
366:
358:
357:
356:
355:
349:
344:
341:Dihedral group
337:
336:
331:
325:
320:
314:
309:
298:
293:
292:
289:
288:
283:
282:
281:
280:
275:
267:
266:
265:
264:
259:
254:
249:
244:
239:
234:
232:multiplicative
229:
224:
219:
214:
206:
205:
204:
203:
198:
190:
189:
181:
180:
179:
178:
176:Wreath product
173:
168:
163:
161:direct product
155:
153:Quotient group
147:
146:
145:
144:
139:
134:
124:
121:
120:
117:
116:
108:
107:
74:cycle notation
61:
43:circular shift
37:
21:Symmetry group
15:
9:
6:
4:
3:
2:
9211:
9200:
9197:
9195:
9192:
9190:
9187:
9186:
9184:
9175:
9172:
9169:
9166:
9163:
9158:
9157:
9152:
9149:
9144:
9139:
9138:
9133:
9130:
9125:
9121:
9117:
9116:
9111:
9107:
9106:
9096:
9092:
9088:
9084:
9080:
9077:(in German),
9076:
9075:
9067:
9063:
9059:
9055:
9052:
9048:
9044:
9040:
9036:
9032:
9031:
9026:
9022:
9019:
9013:
9009:
9005:
9000:
8997:
8991:
8987:
8983:
8979:
8972:
8968:
8964:
8961:
8957:
8953:
8949:
8945:
8942:
8938:
8934:
8930:
8926:
8922:
8921:
8915:
8911:
8906:
8902:
8898:
8897:
8892:
8888:
8887:Praeger, C.E.
8883:
8880:
8876:
8872:
8866:
8862:
8858:
8854:
8850:
8845:
8842:
8838:
8834:
8830:
8825:
8820:
8816:
8812:
8811:
8806:
8801:
8797:
8791:
8787:
8786:Basic algebra
8783:
8779:
8776:
8772:
8768:
8762:
8758:
8753:
8752:
8745:
8742:
8736:
8732:
8727:
8726:
8719:
8718:
8704:
8700:
8696:
8692:
8685:
8678:
8670:
8666:
8661:
8656:
8652:
8648:
8644:
8636:
8628:
8624:
8617:
8609:
8602:
8595:
8589:
8585:
8581:
8575:
8568:
8564:
8559:
8554:
8550:
8546:
8539:
8531:
8526:
8522:
8518:
8515:: 1435–1442,
8514:
8510:
8503:
8496:
8490:
8486:
8482:
8478:
8472:
8465:
8459:
8455:
8451:
8447:
8441:
8433:
8426:
8418:
8416:9788182830561
8412:
8408:
8404:
8397:
8389:
8383:
8376:
8375:Jacobson 2009
8371:
8364:
8363:Jacobson 2009
8359:
8357:
8355:
8353:
8348:
8338:
8335:
8333:
8330:
8328:
8325:
8323:
8319:
8316:
8314:
8311:
8309:
8306:
8305:
8299:
8296:
8294:
8290:
8289:
8283:
8278:
8275:
8270:
8267:
8266:group algebra
8263:
8259:
8255:
8251:
8247:
8242:
8240:
8236:
8231:
8229:
8226:of size
8225:
8221:
8217:
8213:
8209:
8205:
8201:
8197:
8193:
8188:
8182:
8180:
8176:
8172:
8168:
8164:
8158:
8148:
8146:
8142:
8137:
8136:stabilizing.
8135:
8131:
8123:
8117:
8113:
8108:
8102:
8098:
8093:
8086:
8080:
8073:
8068:
8048:
8033:
8018:
7986:
7981:
7966:
7937:
7922:
7895:
7890:
7880:
7872:
7857:
7853:
7849:
7841:
7826:
7822:
7801:
7796:
7786:
7778:
7763:
7759:
7755:
7747:
7732:
7728:
7719:
7714:
7711:
7706:
7702:
7676:
7673:
7670:
7665:
7661:
7648:
7645:
7642:
7637:
7631:
7626:
7615:
7610:
7595:
7591:
7583:
7582:
7581:
7579:
7574:
7571:
7559:
7546:
7540:
7532:
7527:
7523:
7514:
7487:
7484:
7481:
7476:
7472:
7459:
7456:
7453:
7448:
7442:
7437:
7426:
7421:
7406:
7402:
7394:
7393:
7392:
7390:
7385:
7380:
7374:
7364:
7362:
7358:
7354:
7350:
7345:
7315:
7309:
7297:
7291:
7289:
7285:
7281:
7276:
7269:
7255:
7249:
7239:
7236:
7233:
7232:
7225:
7219:
7213:
7210:
7207:
7206:
7199:
7193:
7190:
7185:
7182:
7179:
7178:
7173:
7168:
7164:
7159:
7155:
7150:
7148:
7145:
7144:
7140:
7130:
7128:
7123:
7101:
7097:
7096:Cyclic groups
7088:
7086:
7082:
7078:
7069:
7067:
7061:
7054:
7046:
7025:
7021:
7017:
7014:
7011:
7006:
7002:
6974:
6970:
6965:
6961:
6958:
6955:
6948:
6944:
6939:
6930:
6924:
6915:
6905:
6903:
6898:
6893:
6889:
6885:
6881:
6876:
6871:
6861:
6859:
6855:
6851:
6847:
6843:
6838:
6821:
6817:
6793:
6791:
6788:expansion of
6787:
6781:
6777:
6773:
6766:
6762:
6759: +
6755:
6751:
6744:
6740:
6731:
6726:
6720:
6716:
6712:
6708:
6703:
6700:
6695:
6690:
6683:
6678:
6671:
6666:
6661:
6657:
6652:
6636:
6633:
6630:
6627:
6624:
6621:
6618:
6615:
6612:
6609:
6606:
6595:
6592:
6589:
6586:
6579:
6575:
6571:
6564:
6557:
6552:
6548:
6544:
6540:
6535:
6531:
6525:
6519:
6511:
6507:
6503:
6499:
6493:
6489:
6484:
6480:
6474:
6470:
6466:
6460:
6456:
6452:
6447:
6445:
6443:
6438:
6428:
6426:
6422:
6418:
6414:
6410:
6406:
6400:
6396:
6388:
6384:
6378:
6369:
6365:
6357:
6353:
6347:
6338:
6331:
6320:
6311:
6307:
6304:This section
6302:
6299:
6295:
6294:
6286:
6284:
6280:
6276:
6272:
6268:
6264:
6260:
6256:
6252:
6248:
6244:
6240:
6236:
6232:
6228:
6223:
6221:
6215:
6208:
6199:
6192:
6185:
6180:
6176:
6171:
6164:
6159:
6149:
6147:
6143:
6133:
6124:-cycle and a
6118:
6114:
6099:
6097:
6093:
6092:Coxeter group
6074:
6071:
6068:
6063:
6053:
6050:
6047:
6043:
6037:
6033:
6022:
6007:
6004:
5996:
5993:
5990:
5963:
5959:
5953:
5949:
5945:
5940:
5936:
5930:
5926:
5918:
5904:
5901:
5898:
5893:
5888:
5884:
5876:
5875:
5874:
5870:
5849:
5846:
5843:
5839:
5835:
5832:
5829:
5824:
5820:
5809:
5785:
5782:
5779:
5776:
5773:
5767:
5762:
5758:
5750:
5736:
5732:
5725:
5718:
5714:
5706:
5700:
5694:
5691:
5686:
5681:
5674:
5668:
5666:
5662:
5656:
5649:
5643:
5636:
5630:
5627:
5621:
5615:
5610:
5604:
5598:
5593:
5588:
5583:
5578:
5572:
5566:
5560:
5553:
5547:
5541:
5535:
5532:
5526:
5520:
5514:
5503:
5495:
5484:
5478:
5471:
5466:
5461:
5455:
5444:
5441:
5437:
5430:
5424:
5399:
5382:
5358:
5357:
5356:
5354:
5344:
5323:
5299:
5295:
5283:
5282:
5278:
5277:
5265:
5259:
5241:
5237:
5236:Galois theory
5233:
5225:
5219:
5218:
5207:
5201:
5194:
5179:
5175:
5167:
5163:
5141:
5134:
5126:
5120:
5119:
5113:
5106:
5101:
5089:
5085:
5081:
5077:
5076:Galois theory
5065:
5061:
5053:
5046:
5042:
5038:
5031:
5025:
5024:
5020:
5016:
5009:, while the A
5008:
5004:
4992:
4988:
4980:
4974:
4973:
4969:
4961:
4954:
4950:
4942:
4938:
4934:
4930:
4926:
4922:
4918:
4914:
4907:
4900:
4896:
4892:
4888:
4884:
4880:
4876:
4872:
4865:
4860:
4856:
4852:
4848:
4847:Galois theory
4844:
4840:
4836:
4830:
4829:
4825:
4813:
4812:singleton set
4809:
4805:
4795:
4794:
4793:
4789:
4779:
4765:
4756:
4750:
4746:
4740:
4736:
4729:
4722:
4716:
4712:
4706:
4702:
4695:
4688:
4669:
4665:
4659:
4654:
4651:
4648:
4644:
4640:
4637:
4626:
4622:
4615:
4608:
4604:
4599:
4595:
4590:
4584:
4570:
4564:
4558:
4549:
4543:
4537:
4531:
4525:
4519:
4513:
4507:
4501:
4492:
4486:
4480:
4474:
4468:
4462:
4439:
4434:
4428:
4423:
4418:
4413:
4408:
4401:
4396:
4391:
4386:
4381:
4375:
4370:
4367:
4358:
4348:
4342:
4337:
4332:
4327:
4317:
4315:
4311:
4307:
4302:
4289:
4281:
4277:
4273:
4262:
4259:
4248:
4247:
4241:
4234:
4232:
4202:
4198:
4190:
4187:
4184:
4181:
4178:
4173:
4170:
4159:
4155:
4147:
4144:
4141:
4138:
4135:
4130:
4127:
4121:
4116:
4111:
4107:
4100:
4097:
4094:
4088:
4080:
4077:
4071:
4063:
4059:
4055:
4044:
4041:
4035:
4027:
4023:
4001:
4000:
3999:
3980:
3977:
3971:
3968:
3965:
3961:
3958:
3955:
3946:
3943:
3940:
3936:
3933:
3930:
3921:
3917:
3911:
3905:
3902:
3899:
3895:
3892:
3889:
3880:
3877:
3874:
3870:
3860:
3859:
3839:
3832:
3829:
3826:
3820:
3814:
3811:
3806:
3803:
3800:
3795:
3792:
3789:
3785:
3776:
3773:
3767:
3764:
3761:
3757:
3748:
3744:
3734:
3733:
3732:
3715:
3711:
3707:
3695:
3691:
3687:
3679:
3676:
3670:
3666:
3647:
3642:
3636:
3631:
3626:
3623:
3620:
3615:
3608:
3603:
3598:
3593:
3587:
3578:
3577:
3576:
3574:
3565:
3563:
3537:
3531:
3523:
3517:
3509:
3503:
3495:
3489:
3483:
3478:
3473:
3470:
3466:
3460:
3454:
3449:
3444:
3439:
3433:
3428:
3421:
3420:
3419:
3417:
3401:
3392:
3390:
3385:
3372:
3368:
3350:
3343:
3336:
3314:
3308:
3303:
3298:
3293:
3288:
3281:
3276:
3271:
3266:
3261:
3255:
3250:
3247:
3240:
3239:
3238:
3236:
3232:
3226:
3219:
3214:
3210:
3206:
3202:
3198:
3194:
3190:
3186:
3182:
3178:
3174:
3170:
3166:
3163:
3159:
3149:
3147:
3141:
3136:
3130:
3127:
3121:
3120:
3114:
3111:
3106:
3101:
3094:
3087:
3081:
3076:
3071:
3066:
3065:
3060:
3056:
3052:
3027:
3024:
3021:
3018:
3015:
3004:
2994:
2991:
2984:
2983:
2982:
2958:
2950:
2940:
2937:
2934:
2926: is even
2920:
2910:
2907:
2904:
2898:
2893:
2890:
2887:
2884:
2877:
2876:
2875:
2873:
2868:
2864:
2862:
2858:
2854:
2850:
2846:
2841:
2840:transposition
2835:
2822:
2818:
2815:
2811:
2808:
2805:
2801:
2800:
2799:
2797:
2793:
2769:
2763:
2757:
2751:
2742:
2736:
2730:
2724:
2719:
2711:
2705:
2699:
2693:
2687:
2681:
2671:
2670:
2669:
2665:
2658:
2653:
2649:
2645:
2640:
2636:
2632:
2627:
2608:
2603:
2597:
2592:
2587:
2582:
2577:
2570:
2565:
2560:
2555:
2550:
2544:
2539:
2533:
2527:
2518:
2512:
2506:
2500:
2497:
2494:
2491:
2488:
2485:
2482:
2475:
2474:
2473:
2471:
2467:
2463:
2459:
2440:
2435:
2429:
2424:
2419:
2414:
2409:
2402:
2397:
2392:
2387:
2382:
2376:
2371:
2365:
2359:
2350:
2344:
2338:
2332:
2329:
2322:
2306:
2300:
2295:
2290:
2285:
2280:
2273:
2268:
2263:
2258:
2253:
2247:
2242:
2236:
2230:
2221:
2212:
2206:
2200:
2197:
2190:
2189:
2188:
2186:
2182:
2178:
2174:
2170:
2166:
2162:
2158:
2154:
2150:
2145:
2141:
2130:
2128:
2124:
2120:
2110:
2108:
2104:
2100:
2096:
2092:
2088:
2087:group actions
2084:
2080:
2076:
2072:
2068:
2064:
2061:), and their
2060:
2056:
2055:combinatorics
2052:
2048:
2043:
2038:
2033:
2031:
2027:
2023:
2019:
2015:
2011:
2010:Galois theory
2007:
2003:
1999:
1995:
1985:
1970:
1962:
1946:
1943:
1940:
1932:
1916:
1913:
1910:
1902:
1897:
1880:
1877:
1874:
1871:
1868:
1865:
1862:
1854:
1850:
1849:singleton set
1846:
1830:
1827:
1824:
1804:
1801:
1798:
1778:
1770:
1754:
1746:
1730:
1727:
1720:
1717:elements has
1704:
1695:
1693:
1689:
1685:
1680:
1663:
1657:
1654:
1632:
1605:
1574:
1544:
1541:
1538:
1535:
1532:
1529:
1526:
1503:
1480:
1474:
1471:
1451:
1448:
1426:
1399:
1368:
1341:
1332:
1315:
1312:
1309:
1306:
1303:
1300:
1297:
1291:
1288:
1268:
1261:
1257:
1241:
1221:
1201:
1187:
1173:
1165:
1161:
1157:
1141:
1133:
1129:
1128:combinatorics
1125:
1121:
1117:
1116:Galois theory
1112:
1110:
1106:
1102:
1098:
1094:
1090:
1089:infinite sets
1085:
1071:
1068:
1046:
1031:
1027:
1012:
992:
989:
969:
961:
945:
937:
919:
904:
900:
896:
892:
888:
884:
880:
876:
864:
859:
857:
852:
850:
845:
844:
842:
841:
834:
831:
830:
827:
824:
823:
820:
817:
816:
813:
810:
809:
806:
801:
800:
790:
787:
784:
783:
781:
775:
772:
770:
767:
766:
763:
760:
758:
755:
753:
750:
749:
746:
740:
738:
732:
730:
724:
722:
716:
714:
708:
707:
703:
699:
696:
695:
691:
687:
684:
683:
679:
675:
672:
671:
667:
663:
660:
659:
655:
651:
648:
647:
643:
639:
636:
635:
631:
627:
624:
623:
619:
615:
612:
611:
608:
605:
603:
600:
599:
596:
592:
587:
586:
579:
576:
574:
571:
569:
566:
565:
537:
512:
511:
509:
503:
500:
475:
472:
471:
465:
462:
460:
457:
456:
452:
451:
440:
437:
435:
432:
429:
426:
425:
424:
423:
420:
417:
416:
411:
408:
407:
404:
401:
400:
397:
394:
392:
390:
386:
385:
382:
379:
377:
374:
373:
370:
367:
365:
362:
361:
360:
359:
353:
350:
347:
342:
339:
338:
334:
329:
326:
323:
318:
315:
312:
307:
304:
303:
302:
301:
296:
295:Finite groups
291:
290:
279:
276:
274:
271:
270:
269:
268:
263:
260:
258:
255:
253:
250:
248:
245:
243:
240:
238:
235:
233:
230:
228:
225:
223:
220:
218:
215:
213:
210:
209:
208:
207:
202:
199:
197:
194:
193:
192:
191:
188:
187:
183:
182:
177:
174:
172:
169:
167:
164:
162:
159:
156:
154:
151:
150:
149:
148:
143:
140:
138:
135:
133:
130:
129:
128:
127:
122:Basic notions
119:
118:
114:
110:
109:
106:
101:
97:
93:
92:
84:
75:
71:
70:two-line form
67:
59:
55:
51:
44:
40:
33:
28:
22:
9154:
9135:
9113:
9078:
9072:
9034:
9028:
9025:Schur, Issai
9004:Group Theory
9003:
8977:
8951:
8948:Netto, Eugen
8924:
8918:
8900:
8894:
8848:
8814:
8808:
8785:
8750:
8724:
8694:
8690:
8677:
8650:
8646:
8635:
8626:
8622:
8616:
8607:
8601:
8583:
8574:
8548:
8544:
8538:
8530:1721.1/96203
8512:
8508:
8502:
8480:
8471:
8449:
8440:
8431:
8425:
8406:
8396:
8382:
8370:
8365:, p. 31
8297:
8286:
8279:
8273:
8268:
8257:
8249:
8243:
8232:
8227:
8219:
8215:
8203:
8191:
8186:
8183:
8160:
8145:Hopf algebra
8141:Nakaoka 1961
8138:
8129:
8121:
8115:
8111:
8106:
8100:
8096:
8091:
8084:
8078:
8069:
8016:
7715:
7709:
7698:
7575:
7569:
7557:
7544:
7538:
7530:
7525:
7521:
7512:
7509:
7383:
7376:
7352:
7348:
7346:
7313:
7310:
7295:
7292:
7274:
7267:
7264:
7234:
7208:
7188:
7180:
7171:
7162:
7153:
7146:
7121:
7094:
7084:
7080:
7075:
7059:
6928:
6922:
6917:
6901:
6896:
6879:
6874:
6869:
6867:
6839:
6819:
6809:(2) = D
6801:(1) = C
6794:
6789:
6785:
6779:
6775:
6771:
6764:
6760:
6753:
6749:
6742:
6735:
6729:
6724:
6718:
6714:
6710:
6706:
6704:
6698:
6693:
6688:
6681:
6676:
6669:
6664:
6659:
6655:
6653:
6593:
6590:
6587:
6584:
6577:
6573:
6569:
6562:
6555:
6550:
6542:
6538:
6536:
6529:
6517:
6509:
6505:
6501:
6491:
6487:
6472:
6468:
6464:
6458:
6454:
6450:
6448:
6441:
6434:
6404:
6398:
6394:
6386:
6382:
6376:
6367:
6363:
6355:
6351:
6345:
6336:
6327:
6314:
6310:adding to it
6305:
6285:, Ch. 8.1).
6262:
6258:
6254:
6246:
6242:
6238:
6234:
6230:
6224:
6213:
6206:
6197:
6190:
6183:
6178:
6169:
6162:
6155:
6139:
6116:
6112:
6100:
6089:
5868:
5807:
5742:
5730:
5723:
5716:
5712:
5704:
5698:
5695:
5689:
5679:
5672:
5669:
5654:
5647:
5641:
5634:
5631:
5625:
5619:
5613:
5602:
5596:
5586:
5576:
5570:
5564:
5558:
5551:
5545:
5539:
5536:
5530:
5524:
5518:
5512:
5501:
5493:
5482:
5476:
5464:
5453:
5450:
5439:
5435:
5428:
5422:
5417:
5342:
5321:
5316:
5298:triple cover
5279:for details.
5274:
5263:
5257:
5205:
5199:
5162:Galois group
5111:
5104:
5099:
4959:
4952:
4948:
4940:
4936:
4932:
4928:
4924:
4920:
4916:
4912:
4905:
4898:
4894:
4890:
4886:
4882:
4878:
4874:
4870:
4863:
4841:and is thus
4839:cyclic group
4791:
4754:
4748:
4744:
4738:
4734:
4727:
4720:
4714:
4710:
4704:
4700:
4693:
4686:
4624:
4620:
4613:
4606:
4602:
4597:
4588:
4585:
4356:
4346:
4340:
4330:
4323:
4309:
4305:
4303:
4244:
4239:
4235:
4230:
4228:
3997:
3696:
3689:
3685:
3677:
3674:
3665:Bruhat order
3662:
3568:
3566:
3561:
3559:
3393:
3383:
3366:
3348:
3341:
3334:
3331:
3234:
3230:
3229:the element
3224:
3217:
3212:
3208:
3204:
3200:
3196:
3192:
3188:
3184:
3180:
3179:} such that
3176:
3175:in {1, ...,
3172:
3168:
3164:
3161:
3155:
3139:
3134:
3128:
3125:
3117:
3115:
3109:
3099:
3092:
3085:
3079:
3069:
3062:
3048:
2980:
2955: is odd
2871:
2869:
2865:
2860:
2852:
2848:
2844:
2839:
2837:
2813:
2803:
2794:is indeed a
2791:
2789:
2663:
2656:
2651:
2647:
2643:
2638:
2634:
2630:
2623:
2469:
2465:
2461:
2457:
2455:
2180:
2176:
2172:
2168:
2164:
2160:
2156:
2152:
2148:
2143:
2139:
2136:
2126:
2123:permutations
2118:
2116:
2075:Bruhat order
2059:permutations
2041:
2034:
2005:
1998:Galois group
1993:
1991:
1988:Applications
1895:
1696:
1692:Cameron 1999
1686:, Ch. 11), (
1681:
1333:
1259:
1193:
1113:
1107:, and their
1086:
960:permutations
893:are all the
878:
872:
701:
689:
677:
665:
653:
641:
629:
617:
388:
345:
332:
321:
316:
310:
306:Cyclic group
184:
171:Free product
142:Group action
105:Group theory
100:Group theory
99:
54:Cayley table
32:Cayley graph
9081:: 258–260,
8817:: 239–276,
8813:, Série 3,
8691:Studia Math
8308:Braid group
8244:Over other
8210:, over the
8017:homological
7952:extends to
7718:exceptional
6900:, for some
6860:, §39–40).
6850:Kerber 1971
6846:Rotman 1995
6722:copies of W
6576:= (4 5 6),
6572:= (1 2 3),
4816:0! = 1! = 1
4336:cycle types
3237:defined by
3222:since with
3146:bubble sort
2185:permutation
1961:polynomials
1516:is the set
591:Topological
430:alternating
9183:Categories
9095:0016.20301
8960:14.0090.01
8714:References
8558:2112.03427
8293:dimensions
8200:partitions
8190:has order
8134:Lie groups
8019:– the map
7701:Schur 1911
7371:See also:
6884:transitive
6858:Netto 1882
6784:(the base
6654:The Sylow
6537:The Sylow
6483:normalizer
6479:generators
6449:The Sylow
6279:Scott 1987
6249:is even a
6177:of degree
5864:generates
5801:that swap
5590:while the
5041:9, 8 and 6
5032:The group
4786:See also:
3083:, and for
3073:. It is a
2859:, whereas
2628:of length
2073:, and the
2047:Weyl group
2020:. In the
2004:of degree
2002:polynomial
1963:of degree
1959:there are
1684:Scott 1987
1156:isomorphic
936:finite set
895:bijections
698:Symplectic
638:Orthogonal
595:Lie groups
502:Free group
227:continuous
166:Direct sum
9156:MathWorld
9137:MathWorld
9120:EMS Press
9051:122809608
8833:0012-9593
8669:186219904
8039:↠
7972:↠
7928:↠
7881:≅
7850:≅
7787:≅
7756:≅
7674:≥
7524:≥ 2; for
7485:≥
7026:ℓ
7015:…
6975:ℓ
6962:×
6959:⋯
6956:×
6732:), where
6634:≤
6610:≤
6580:= (7 8 9)
6044:σ
6034:σ
5994:−
5960:σ
5950:σ
5937:σ
5927:σ
5885:σ
5847:−
5840:σ
5833:…
5821:σ
5759:σ
5667:, below.
5230:, has an
5172:is not a
4808:empty set
4766:μ
4666:μ
4645:∑
4514:∘
4475:∘
4285:⌋
4271:⌊
4260:−
4182:≡
4171:−
4139:≡
4098:−
4078:−
4067:⌋
4053:⌊
4042:−
4024:ρ
3978:⋯
3969:−
3959:−
3944:−
3934:−
3912:⋯
3903:−
3893:−
3878:−
3830:−
3804:−
3786:∑
3774:⋯
3765:−
3719:⌋
3705:⌊
3632:⋯
3624:−
3604:⋯
3532:…
3518:σ
3504:σ
3490:σ
3471:−
3467:σ
3455:…
3429:σ
3402:σ
3025:−
3010:→
2995::
2935:−
2888:
2495:∘
2456:Applying
2079:Subgroups
1914:≤
1845:empty set
1767:). It is
1745:factorial
1658:
1629:Σ
1539:…
1475:
1423:Σ
1310:…
1101:subgroups
1026:factorial
762:Conformal
650:Euclidean
257:nilpotent
9194:Symmetry
9064:(1936),
8969:(1995),
8950:(1882),
8891:Saxl, J.
8784:(2009),
8629:: 29–31.
8582:(1991),
8448:(2001),
8302:See also
8260:then by
8202:of
7549:are to S
7367:Homology
6545:are the
6471:− 1) = (
6415:and the
6391:, where
6271:Schreier
6245:. Since
6142:subgroup
6132:-cycle.
5659:it is a
5371:V < A
5138:and the
5136:SL(2, 5)
4810:and the
4699:≥ ... ≥
3667:and the
3367:disjoint
3199:), ...,
2947:if
2917:if
2121:are the
2105:and the
1901:solvable
1847:and the
1160:subgroup
1103:, their
1099:, their
891:elements
757:Poincaré
602:Solenoid
474:Integers
464:Lattices
439:sporadic
434:Lie type
262:solvable
252:dihedral
237:additive
222:infinite
132:Subgroup
66:matrices
9122:, 2001
8941:1970333
8879:0325752
8841:0028834
8775:1409812
8584:Algebra
8282:modules
8194:!. Its
7707:, 2 · S
7565:≅ {±1}
7183:≠ 2, 6
6696:) and W
6649:
6599:
6528:AGL(1,
6481:. The
6467:− 1)!/(
6444:-groups
5568:: Aut(A
5355:, are:
5160:is the
4843:abelian
4743:. Then
4733:, ...,
4619:, ...,
3371:commute
3363:(3 1 4)
3359:(4 3 1)
3355:(1 4 3)
3351:(3) = 1
3344:(4) = 3
3337:(1) = 4
3103:is the
3090:it has
3057:). The
2049:of the
1996:is the
1853:trivial
1769:abelian
901:is the
885:is the
752:Lorentz
674:Unitary
573:Lattice
513:PSL(2,
247:abelian
158:(Semi-)
56:, with
9093:
9049:
9014:
8992:
8958:
8939:
8877:
8867:
8839:
8831:
8792:
8773:
8763:
8737:
8667:
8590:
8491:
8460:
8413:
8246:fields
7580:) is:
7391:) is:
7284:center
7282:: its
7270:≠ 2, 6
7043:is an
6991:where
6888:finite
6854:Cauchy
6814:, the
6267:Onofri
6227:Vitali
6195:; for
5733:> 1
5683:is an
5657:≠ 2, 6
5470:simple
5458:, the
5433:where
5375:< S
5058:has a
4903:, and
4845:. In
4562:
4547:
4541:
4523:
4505:
4490:
4484:
4466:
4243:, the
3162:length
3152:Cycles
3059:kernel
3034:
2761:
2755:
2740:
2734:
2709:
2703:
2697:
2691:
2685:
2531:
2516:
2510:
2472:gives
2460:after
2363:
2348:
2342:
2234:
2210:
2099:graphs
2093:, and
2053:. In
2024:, the
2012:. In
1496:. If
1464:, and
1260:degree
1126:, and
1122:, the
889:whose
877:, the
607:Circle
538:SL(2,
427:cyclic
391:-group
242:cyclic
217:finite
212:simple
196:kernel
58:header
9069:(PDF)
9047:S2CID
8974:(PDF)
8937:JSTOR
8923:, 2,
8687:(PDF)
8665:S2CID
8553:arXiv
8343:Notes
8011:and S
8003:and A
7335:) = S
7331:Aut(S
7324:) = C
7320:Out(S
7278:is a
7160:Out(S
7151:Aut(S
6882:} is
6805:and W
6702:(1).
6475:− 2)!
6366:<
6020:, and
5715:+ 1,
5574:) ≅ S
5438:<
5304:and S
5288:and A
5273:—see
5191:as a
5074:. In
4800:and S
4630:with
3389:up to
3158:cycle
3049:is a
2796:group
2626:cycle
1843:(the
1743:(the
1719:order
1647:, or
1158:to a
1030:order
887:group
791:Sp(∞)
788:SU(∞)
201:image
9171:OEIS
9035:1911
9012:ISBN
8990:ISBN
8865:ISBN
8829:ISSN
8790:ISBN
8761:ISBN
8735:ISBN
8588:ISBN
8489:ISBN
8458:ISBN
8411:ISBN
8320:and
8264:the
8252:has
8161:The
8110:) →
7646:<
7457:<
7381:of S
7377:The
7311:For
7293:For
7286:and
7265:For
7237:= 6
7211:= 2
6872:of S
6747:and
6734:0 ≤
6684:+ 1)
6597:for
6494:− 1)
6435:The
6393:2 ≤
6380:wr S
6362:1 ≤
6360:for
6328:The
6275:Ulam
6261:and
6156:The
6111:2 ≤
6109:for
6105:and
6005:>
5978:for
5805:and
5719:+ 2)
5670:For
5617:so S
5611:of A
5594:of A
5584:of A
5528:or S
5451:For
5094:to S
5045:cube
4997:to S
4931:) −
4919:) =
4889:) +
4877:) =
4684:and
4328:of S
4324:The
4236:In S
3684:1 ≤
3567:The
3346:and
3207:) =
3107:of A
3077:of S
2872:sign
2468:and
2151:and
2032:.
1944:>
1817:and
1166:of)
1095:, a
785:O(∞)
774:Loop
593:and
9091:Zbl
9083:doi
9039:doi
8982:doi
8956:JFM
8929:doi
8905:doi
8857:doi
8819:doi
8699:doi
8655:doi
8563:doi
8525:hdl
8517:doi
8513:309
8082:→ S
7561:→ S
7542:→ C
7516:→ S
7339:⋊ C
7316:= 6
7298:= 2
7272:, S
7244:⋊ C
7169:Z(S
7055:of
7047:of
6832:× C
6792:).
6745:− 1
6558:= 3
6520:= 5
6512:−1)
6419:, (
6349:× S
6332:of
6312:.
6253:of
6216:= 4
6200:≥ 3
6193:≥ 3
6186:≥ 2
6167:, S
6165:≤ 2
6098:).
5810:+ 1
5677:, S
5675:≥ 5
5650:≠ 2
5639:, S
5637:≠ 6
5605:= 6
5556:, S
5554:≠ 6
5534:).
5505:⋊ S
5486:→ S
5480:→ S
5468:is
5456:≥ 5
5426:→ S
5405:→ S
5388:→ S
5364:→ S
5346:→ S
5333:≅ S
5329:≅ S
5325:→ C
5296:(a
5261:→ S
5248:→ S
5203:→ S
5186:→ S
5152:, S
5147:× S
5114:= 4
5107:− 1
5054:, S
4605:= (
4596:of
4199:mod
4156:mod
3692:− 1
3680:+1)
3361:or
3227:= 1
3220:≥ 2
3191:),
3160:of
3131:+1)
3095:!/2
3088:≥ 2
2992:sgn
2885:sgn
2668:),
2666:= 3
2659:= 2
2171:to
2167:of
2159:of
2125:of
2097:of
2077:.
1899:is
1747:of
1694:).
1655:Sym
1472:Sym
1234:to
1154:is
1061:is
938:of
883:set
873:In
700:Sp(
688:SU(
664:SO(
628:SL(
616:GL(
9185::
9153:.
9134:.
9118:,
9112:,
9089:,
9079:28
9071:,
9060:;
9045:,
9033:,
9006:,
8988:,
8976:,
8935:,
8925:73
8901:44
8899:,
8889:;
8875:MR
8873:,
8863:,
8855:,
8837:MR
8835:,
8827:,
8815:65
8807:,
8771:MR
8769:,
8759:,
8733:,
8693:.
8689:.
8663:.
8649:.
8645:.
8625:.
8561:,
8547:,
8523:,
8511:,
8487:,
8456:,
8405:.
8351:^
8230:.
8181:.
8147:.
8124:+1
8119:(S
8104:(S
8087:+1
7713:.
7677:4.
7573:.
7488:2.
7344:.
7175:)
7166:)
7157:)
7129:.
7068:.
6904:.
6890:)
6868:A
6837:.
6752:=
6741:≤
6651:.
6534:.
6526:,
6490:⋅(
6401:/2
6397:≤
6370:/2
6222:.
6148:.
6140:A
6115:≤
5735:.
5726:+1
5707:+2
5629:.
5443:.
5266:+1
5208:+1
4958:+
4951:=
4947:2⋅
4939:,
4927:,
4915:,
4897:,
4885:,
4873:,
4778:.
4726:,
4692:≥
4612:,
4233:.
3694:.
3688:≤
3682:,
3418:.
3339:,
3183:,
3156:A
2838:A
2661:,
2637:·
2633:=
2624:A
2142:∘
2129:.
2109:.
2089:,
2069:,
1679:.
1620:,
1589:,
1441:,
1414:,
1383:,
1331:.
1186:.
1130:.
1118:,
1084:.
676:U(
652:E(
640:O(
98:→
30:A
9159:.
9140:.
9085::
9041::
8984::
8931::
8907::
8859::
8821::
8799:.
8705:.
8701::
8695:4
8671:.
8657::
8651:7
8627:7
8565::
8555::
8549:2
8527::
8519::
8454:4
8419:.
8390:.
8274:n
8271:S
8269:K
8258:n
8250:K
8228:n
8220:n
8216:n
8204:n
8192:n
8187:n
8130:n
8126:)
8122:n
8116:k
8112:H
8107:n
8101:k
8097:H
8092:k
8085:n
8079:n
8076:S
8065:4
8049:3
8044:S
8034:4
8029:S
8013:7
8009:6
8005:7
8001:6
7987:,
7982:3
7977:S
7967:4
7962:S
7938:3
7933:C
7923:4
7918:A
7896:,
7891:6
7886:C
7878:)
7873:7
7868:A
7863:(
7858:2
7854:H
7847:)
7842:6
7837:A
7832:(
7827:2
7823:H
7802:,
7797:3
7792:C
7784:)
7779:4
7774:A
7769:(
7764:1
7760:H
7753:)
7748:3
7743:A
7738:(
7733:1
7729:H
7710:n
7671:n
7666:2
7662:/
7657:Z
7649:4
7643:n
7638:0
7632:{
7627:=
7624:)
7620:Z
7616:,
7611:n
7606:S
7601:(
7596:2
7592:H
7570:n
7563:2
7558:n
7555:S
7551:2
7545:p
7539:n
7536:S
7531:n
7526:n
7522:n
7518:2
7513:n
7482:n
7477:2
7473:/
7468:Z
7460:2
7454:n
7449:0
7443:{
7438:=
7435:)
7431:Z
7427:,
7422:n
7417:S
7412:(
7407:1
7403:H
7384:n
7353:X
7349:X
7341:2
7337:6
7333:6
7326:2
7322:6
7314:n
7306:2
7302:2
7296:n
7275:n
7268:n
7258:1
7256:C
7252:2
7250:C
7246:2
7242:6
7240:S
7235:n
7228:2
7226:S
7222:1
7220:C
7216:1
7214:C
7209:n
7202:1
7200:C
7196:1
7194:C
7189:n
7186:S
7181:n
7172:n
7163:n
7154:n
7147:n
7122:n
7117:5
7113:5
7111:S
7108:5
7104:5
7085:G
7081:G
7060:n
7057:S
7049:n
7031:)
7022:a
7018:,
7012:,
7007:1
7003:a
6999:(
6971:a
6966:S
6949:1
6945:a
6940:S
6923:n
6920:S
6902:n
6897:n
6880:n
6875:n
6834:2
6830:8
6828:D
6811:8
6807:2
6803:2
6799:2
6797:W
6790:n
6786:p
6780:k
6776:a
6774:⋅
6772:p
6768:1
6765:a
6763:⋅
6761:p
6757:0
6754:a
6750:n
6743:p
6738:i
6736:a
6730:i
6728:(
6725:p
6719:i
6715:a
6711:n
6707:p
6699:p
6694:n
6692:(
6689:p
6682:n
6680:(
6677:p
6674:W
6670:n
6668:(
6665:p
6660:p
6656:p
6637:2
6631:l
6628:,
6625:k
6622:,
6619:j
6616:,
6613:i
6607:0
6594:z
6591:y
6588:x
6585:a
6578:z
6574:y
6570:x
6563:a
6556:p
6551:p
6543:p
6539:p
6532:)
6530:p
6518:p
6510:p
6508:(
6506:p
6502:F
6492:p
6488:p
6473:p
6469:p
6465:p
6463:(
6459:p
6455:p
6451:p
6442:p
6405:n
6399:n
6395:k
6387:k
6385:/
6383:n
6377:k
6374:S
6368:n
6364:k
6356:k
6354:–
6352:n
6346:k
6343:S
6337:n
6334:S
6319:)
6315:(
6273:–
6263:S
6259:A
6255:S
6247:A
6243:A
6239:S
6235:S
6231:S
6214:n
6207:n
6204:S
6198:n
6191:n
6184:n
6179:n
6170:n
6163:n
6130:n
6126:2
6122:n
6117:n
6113:i
6107:i
6103:1
6075:,
6072:1
6069:=
6064:3
6060:)
6054:1
6051:+
6048:i
6038:i
6030:(
6008:1
6001:|
5997:j
5991:i
5987:|
5964:i
5954:j
5946:=
5941:j
5931:i
5905:,
5902:1
5899:=
5894:2
5889:i
5869:n
5866:S
5850:1
5844:n
5836:,
5830:,
5825:1
5808:i
5803:i
5789:)
5786:1
5783:+
5780:i
5777:,
5774:i
5771:(
5768:=
5763:i
5745:n
5731:n
5724:n
5717:n
5713:n
5711:(
5705:n
5699:n
5696:S
5690:n
5680:n
5673:n
5655:n
5648:n
5642:n
5635:n
5626:n
5620:n
5614:n
5603:n
5597:n
5587:n
5577:n
5571:n
5565:n
5559:n
5552:n
5546:n
5540:n
5537:S
5531:n
5525:n
5519:n
5513:n
5507:2
5502:n
5499:A
5494:n
5488:2
5483:n
5477:n
5474:A
5465:n
5462:A
5454:n
5440:n
5436:m
5429:n
5423:m
5420:S
5412:6
5407:6
5403:5
5401:S
5397:.
5395:6
5390:6
5386:6
5384:S
5380:;
5377:4
5373:4
5366:3
5362:4
5360:S
5348:2
5343:n
5340:S
5335:1
5331:0
5327:1
5322:n
5319:S
5306:7
5302:6
5290:7
5286:6
5271:6
5264:n
5258:n
5255:S
5250:6
5246:5
5244:S
5228:6
5222:6
5220:S
5213:6
5206:n
5200:n
5197:S
5188:6
5184:5
5182:S
5170:5
5158:5
5154:5
5149:2
5145:5
5143:A
5129:5
5127:S
5123:5
5121:S
5116:.
5112:n
5105:n
5100:n
5096:3
5092:4
5072:3
5056:4
5051:4
5049:A
5036:4
5034:S
5028:4
5026:S
5021:.
5011:3
4999:2
4995:3
4983:3
4981:S
4977:3
4975:S
4970:.
4963:a
4960:f
4956:s
4953:f
4949:f
4943:)
4941:x
4937:y
4935:(
4933:f
4929:y
4925:x
4923:(
4921:f
4917:y
4913:x
4911:(
4909:a
4906:f
4901:)
4899:x
4895:y
4893:(
4891:f
4887:y
4883:x
4881:(
4879:f
4875:y
4871:x
4869:(
4867:s
4864:f
4833:2
4831:S
4826:.
4820:0
4802:1
4798:0
4796:S
4755:n
4749:μ
4745:C
4739:k
4735:μ
4731:2
4728:μ
4724:1
4721:μ
4715:μ
4711:C
4705:k
4701:μ
4697:2
4694:μ
4690:1
4687:μ
4670:i
4660:k
4655:1
4652:=
4649:i
4641:=
4638:n
4628:)
4625:k
4621:μ
4617:2
4614:μ
4610:1
4607:μ
4603:μ
4598:n
4589:n
4571:.
4568:)
4565:5
4559:2
4556:(
4553:)
4550:3
4544:4
4538:1
4535:(
4532:=
4529:)
4526:4
4520:2
4517:(
4511:)
4508:5
4502:4
4499:(
4496:)
4493:3
4487:2
4481:1
4478:(
4472:)
4469:4
4463:2
4460:(
4440:,
4435:)
4429:5
4424:2
4419:3
4414:4
4409:1
4402:5
4397:4
4392:3
4387:2
4382:1
4376:(
4371:=
4368:k
4357:n
4352:5
4347:n
4341:n
4331:n
4310:n
4306:n
4290:.
4282:2
4278:/
4274:n
4267:)
4263:1
4257:(
4240:n
4238:2
4231:n
4206:)
4203:4
4196:(
4191:3
4188:,
4185:2
4179:n
4174:1
4163:)
4160:4
4153:(
4148:1
4145:,
4142:0
4136:n
4131:1
4128:+
4122:{
4117:=
4112:2
4108:/
4104:)
4101:1
4095:n
4092:(
4089:n
4085:)
4081:1
4075:(
4072:=
4064:2
4060:/
4056:n
4049:)
4045:1
4039:(
4036:=
4033:)
4028:n
4020:(
4016:n
4013:g
4010:s
3981:,
3975:)
3972:3
3966:n
3962:2
3956:n
3953:(
3950:)
3947:2
3941:n
3937:1
3931:n
3928:(
3925:)
3922:1
3918:2
3915:(
3909:)
3906:2
3900:n
3896:1
3890:n
3887:(
3884:)
3881:1
3875:n
3871:n
3868:(
3840:2
3836:)
3833:1
3827:n
3824:(
3821:n
3815:=
3812:k
3807:1
3801:n
3796:1
3793:=
3790:k
3777:,
3771:)
3768:1
3762:n
3758:2
3755:(
3752:)
3749:n
3745:1
3742:(
3716:2
3712:/
3708:n
3690:n
3686:i
3678:i
3675:i
3673:(
3648:.
3643:)
3637:1
3627:1
3621:n
3616:n
3609:n
3599:2
3594:1
3588:(
3562:n
3538:)
3527:)
3524:c
3521:(
3513:)
3510:b
3507:(
3499:)
3496:a
3493:(
3484:(
3479:=
3474:1
3461:)
3450:c
3445:b
3440:a
3434:(
3384:n
3375:6
3349:h
3342:h
3335:h
3315:)
3309:5
3304:3
3299:1
3294:2
3289:4
3282:5
3277:4
3272:3
3267:2
3262:1
3256:(
3251:=
3248:h
3235:h
3231:x
3225:k
3218:k
3213:f
3209:x
3205:x
3203:(
3201:f
3197:x
3195:(
3193:f
3189:x
3187:(
3185:f
3181:x
3177:n
3173:x
3169:f
3165:k
3140:g
3135:g
3129:a
3126:a
3124:(
3110:n
3100:n
3093:n
3086:n
3080:n
3070:n
3067:A
3031:}
3028:1
3022:,
3019:1
3016:+
3013:{
3005:n
3000:S
2959:.
2951:f
2941:,
2938:1
2921:f
2911:,
2908:1
2905:+
2899:{
2894:=
2891:f
2861:f
2853:g
2849:g
2845:g
2814:X
2806:.
2804:X
2792:X
2770:.
2767:)
2764:6
2758:4
2752:2
2749:(
2746:)
2743:5
2737:3
2731:1
2728:(
2725:=
2720:2
2716:)
2712:6
2706:5
2700:4
2694:3
2688:2
2682:1
2679:(
2664:m
2657:k
2652:m
2648:k
2644:k
2639:m
2635:k
2631:L
2609:.
2604:)
2598:3
2593:1
2588:5
2583:4
2578:2
2571:5
2566:4
2561:3
2556:2
2551:1
2545:(
2540:=
2537:)
2534:5
2528:3
2525:(
2522:)
2519:4
2513:2
2507:1
2504:(
2501:=
2498:g
2492:f
2489:=
2486:g
2483:f
2470:g
2466:f
2462:g
2458:f
2441:.
2436:)
2430:1
2425:3
2420:4
2415:5
2410:2
2403:5
2398:4
2393:3
2388:2
2383:1
2377:(
2372:=
2369:)
2366:4
2360:3
2357:(
2354:)
2351:5
2345:2
2339:1
2336:(
2333:=
2330:g
2307:)
2301:4
2296:5
2291:1
2286:2
2281:3
2274:5
2269:4
2264:3
2259:2
2254:1
2248:(
2243:=
2240:)
2237:5
2231:4
2228:(
2225:)
2222:2
2219:(
2216:)
2213:3
2207:1
2204:(
2201:=
2198:f
2181:x
2179:(
2177:g
2175:(
2173:f
2169:X
2165:x
2161:g
2157:f
2153:g
2149:f
2144:g
2140:f
2127:X
2119:X
2042:n
2006:n
1994:n
1971:n
1947:4
1941:n
1917:4
1911:n
1896:n
1881:1
1878:=
1875:!
1872:1
1869:=
1866:!
1863:0
1831:1
1828:=
1825:n
1805:0
1802:=
1799:n
1779:n
1755:n
1731:!
1728:n
1705:n
1667:)
1664:n
1661:(
1633:n
1606:n
1600:S
1575:n
1570:S
1548:}
1545:n
1542:,
1536:,
1533:2
1530:,
1527:1
1524:{
1504:X
1484:)
1481:X
1478:(
1452:!
1449:X
1427:X
1400:X
1394:S
1369:X
1364:S
1342:X
1319:}
1316:n
1313:,
1307:,
1304:2
1301:,
1298:1
1295:{
1292:=
1289:X
1269:n
1242:X
1222:X
1202:X
1174:G
1142:G
1072:!
1069:n
1047:n
1042:S
1013:n
1005:(
993:!
990:n
970:n
946:n
920:n
915:S
862:e
855:t
848:v
744:8
742:E
736:7
734:E
728:6
726:E
720:4
718:F
712:2
710:G
704:)
702:n
692:)
690:n
680:)
678:n
668:)
666:n
656:)
654:n
644:)
642:n
632:)
630:n
620:)
618:n
560:)
547:Z
535:)
522:Z
498:)
485:Z
476:(
389:p
354:Q
346:n
343:D
333:n
330:A
322:n
319:S
311:n
308:Z
62:3
38:4
36:S
23:.
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.