4369:
1761:
9944:
3681:
1867:
4536:
8845:
1877:
1935:
29:
9930:
1768:
10827:
2203:
4364:{\displaystyle {\begin{aligned}1-{\sqrt {1-4x}}&=-\sum _{n=1}^{\infty }{\binom {1/2}{n}}(-4x)^{n}=-\sum _{n=1}^{\infty }{\frac {(-1)^{n-1}(2n-3)!!}{2^{n}n!}}(-4x)^{n}\\&=-\sum _{n=0}^{\infty }{\frac {(-1)^{n}(2n-1)!!}{2^{n+1}(n+1)!}}(-4x)^{n+1}=\sum _{n=0}^{\infty }{\frac {2^{n+1}(2n-1)!!}{(n+1)!}}x^{n+1}\\&=\sum _{n=0}^{\infty }{\frac {2(2n)!}{(n+1)!n!}}x^{n+1}=\sum _{n=0}^{\infty }{\frac {2}{n+1}}{\binom {2n}{n}}x^{n+1}\,.\end{aligned}}}
12757:
1550:
9361:
9925:{\displaystyle \sum _{i_{1}+\cdots +i_{m}=n \atop i_{1},\ldots ,i_{m}\geq 0}C_{i_{1}}\cdots C_{i_{m}}={\begin{cases}{\dfrac {m(n+1)(n+2)\cdots (n+m/2-1)}{2(n+m/2+2)(n+m/2+3)\cdots (n+m)}}C_{n+m/2},&m{\text{ even,}}\\{\dfrac {m(n+1)(n+2)\cdots (n+(m-1)/2)}{(n+(m+3)/2)(n+(m+3)/2+1)\cdots (n+m)}}C_{n+(m-1)/2},&m{\text{ odd.}}\end{cases}}}
5342:. Each of the 20 possible monotonic paths appears somewhere in the table. The first column shows all paths of exceedance three, which lie entirely above the diagonal. The columns to the right show the result of successive applications of the algorithm, with the exceedance decreasing one unit at a time. There are five rows, that is
1851:
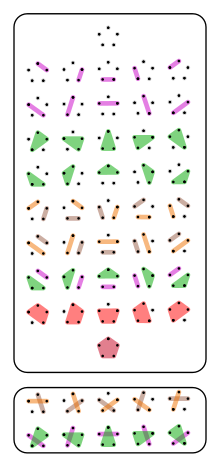
square cells, which do not pass above the diagonal. A monotonic path is one which starts in the lower left corner, finishes in the upper right corner, and consists entirely of edges pointing rightwards or upwards. Counting such paths is equivalent to counting Dyck words: X stands for "move right" and
5127:
for any path that we feed it, because the first vertical step starting on the diagonal (at the point marked with a black dot) is the only vertical edge that changes from being above the diagonal to being below it when we apply the algorithm - all the other vertical edges stay on the same side of the
8831:
1372:
This has a simple probabilistic interpretation. Consider a random walk on the integer line, starting at 0. Let -1 be a "trap" state, such that if the walker arrives at -1, it will remain there. The walker can arrive at the trap state at times 1, 3, 5, 7..., and the number of ways the walker can
1296:
4645:
The part of the path after the higher diagonal is then flipped about that diagonal, as illustrated with the red dotted line. This swaps all the right steps to up steps and vice versa. In the section of the path that is not reflected, there is one more up step than right steps, so therefore the
8470:
281:
8299:
8896:, which was completed by his student Chen Jixin in 1774 but published sixty years later. Peter J. Larcombe (1999) sketched some of the features of the work of Mingantu, including the stimulus of Pierre Jartoux, who brought three infinite series to China early in the 1700s.
7027:
4520:
3221:
6323:
943:
815:
8702:
5104:, and we place the last lattice point of the red portion in the top-right corner, and the first lattice point of the green portion in the bottom-left corner, and place X accordingly, to make a new path, shown in the second diagram.
5005:
2948:
8710:
1119:
8349:
103:
4853:
8610:
8178:
6790:
565:
1021:
8060:
5331:
1504:
6031:
1367:
5781:
7297:
678:
5048:
4374:
6801:
4706:
grid meets the higher diagonal, and because the reflection process is reversible, the reflection is therefore a bijection between bad paths in the original grid and monotonic paths in the new grid.
8545:
7375:
5108:
6596:
5132:
3358:
426:
5906:
3071:
3686:
3596:
3528:
9137:
7198:
7116:
6441:
7899:
5399:
3667:
5251:
5697:
3104:
6206:
826:
699:
7726:
7690:
7654:
7618:
7582:
4636:
3427:
8967:
8932:
8618:
6171:
9326:. The classical recurrence relation generalizes: the Catalan number of a Coxeter diagram is equal to the sum of the Catalan numbers of all its maximal proper sub-diagrams.
8999:
5586:
sides and a (different) triangulation, again mark one of its sides as the base. Mark one of the sides other than the base side (and not an inner triangle edge). There are
5475:
6504:
456:
6067:
7464:
4646:
remaining section of the bad path has one more right step than up steps. When this portion of the path is reflected, it will have one more up step than right steps.
9324:
9297:
9236:
9038:
7847:
6198:
6106:
5426:
2740:
1427:
7974:
6470:
2984:
2413:
2341:
2303:
2265:
1400:
9204:
9178:
8086:
7925:
7795:
7546:
7520:
7490:
5501:
2850:
6622:
4858:
and the number of
Catalan paths (i.e. good paths) is obtained by removing the number of bad paths from the total number of monotonic paths of the original grid,
1063:
9256:
8106:
7945:
7815:
7769:
7749:
7438:
7418:
6662:
6642:
6382:
6362:
2824:
2804:
2784:
2764:
5018:
Y's and interchange all X's and Y's after the first Y that violates the Dyck condition. After this Y, note that there is exactly one more Y than there are Xs.
1526:
contains a set of exercises which describe 66 different interpretations of the
Catalan numbers. Following are some examples, with illustrations of the cases
10859:
8826:{\displaystyle \det {\begin{bmatrix}2&5&14&42\\5&14&42&132\\14&42&132&429\\42&132&429&1430\end{bmatrix}}=5}
4864:
2855:
2087: 123 (or, alternatively, any of the other patterns of length 3); that is, the number of permutations with no three-term increasing subsequence. For
1291:{\displaystyle C_{n}={\frac {1}{2\pi }}\int _{0}^{4}x^{n}{\sqrt {\frac {4-x}{x}}}\,dx\,={\frac {2}{\pi }}4^{n}\int _{-1}^{1}t^{2n}{\sqrt {1-t^{2}}}\,dt.}
951:
10691:
10382:
8465:{\displaystyle \det {\begin{bmatrix}1&2&5&14\\2&5&14&42\\5&14&42&132\\14&42&132&429\end{bmatrix}}=1.}
276:{\displaystyle C_{n}={\frac {1}{n+1}}{2n \choose n}={\frac {(2n)!}{(n+1)!\,n!}}=\prod \limits _{k=2}^{n}{\frac {n+k}{k}}\qquad {\text{for }}n\geq 0.}
7400:
if, reading from left to right, the number of X's is always strictly greater than the number of Y's. The cycle lemma states that any sequence of
8294:{\displaystyle \det {\begin{bmatrix}1&1&2&5\\1&2&5&14\\2&5&14&42\\5&14&42&132\end{bmatrix}}=1.}
2191:
rectangles. Cutting across the anti-diagonal and looking at only the edges gives full binary trees. The following figure illustrates the case
4715:
8553:
6670:
1880:
The dark triangle is the root node, the light triangles correspond to internal nodes of the binary trees, and the green bars are the leaves.
464:
2228:
downstrokes that all stay above a horizontal line. The mountain range interpretation is that the mountains will never go below the horizon.
10399:
5063:
edges above the diagonal. For example, in Figure 2, the edges above the diagonal are marked in red, so the exceedance of this path is 5.
7979:
5256:
1432:
12796:
10814:
307:
5610:
whose side is marked (in two ways, and subtract the two that cannot collapse the base), or, in reverse, expand the oriented edge in
10852:
5917:
1606:
Y's such that no initial segment of the string has more Y's than X's. For example, the following are the Dyck words up to length 6:
1304:
5706:
5066:
Given a monotonic path whose exceedance is not zero, we apply the following algorithm to construct a new path whose exceedance is
1066:
7206:
7022:{\displaystyle B_{n+1}-C_{n+1}=2\sum _{i=0}^{n}B_{i}C_{n-i}-\sum _{i=0}^{n}C_{i}\,C_{n-i}=\sum _{i=0}^{n}(2B_{i}-C_{i})C_{n-i}.}
595:
12786:
10831:
10727:
10674:
10607:
9993:
1035:
8505:
7305:
6512:
3287:
336:
5791:
3001:
11659:
10845:
3533:
3465:
1703:((ab)c)d (a(bc))d (ab)(cd) a((bc)d) a(b(cd))
12791:
11654:
8860:, who was interested in the number of different ways of dividing a polygon into triangles. The sequence is named after
7548:
X's. Each of these X's was the start of a dominating circular shift before anything was removed. For example, consider
2704:
rectangle so that each row and each column is increasing. As such, the formula can be derived as a special case of the
9057:
11669:
10654:
10038:
7124:
7042:
6387:
3436:. On the one hand, the recurrence relation uniquely determines the Catalan numbers; on the other hand, interpreting
11649:
10821:
10493:
Choi, Hayoung; Yeh, Yeong-Nan; Yoo, Seonguk (2020), "Catalan-like number sequences and
Hausdorff moment sequences",
10105:
Choi, Hayoung; Yeh, Yeong-Nan; Yoo, Seonguk (2020), "Catalan-like number sequences and
Hausdorff moment sequences",
10023:
9148:
12362:
11942:
10468:
and Nathan
Reading, "Root systems and generalized associahedra", Geometric combinatorics, IAS/Park City Math. Ser.
9206:, the numbers have an easy combinatorial description. However, other combinatorial descriptions are only known for
4530:
9272:, it is the number of anti-chains (or order ideals) in the poset of positive roots. The classical Catalan number
7852:
5358:
4515:{\displaystyle c(x)={\frac {1-{\sqrt {1-4x}}}{2x}}=\sum _{n=0}^{\infty }{\frac {1}{n+1}}{\binom {2n}{n}}x^{n}\,.}
1650:((())) (()()) (())() ()(()) ()()()
4539:
Figure 1. The invalid portion of the path (dotted red) is flipped (solid red). Bad paths (after the flip) reach
3608:
1806:
11664:
5210:
3216:{\displaystyle C_{0}=1\quad {\text{and}}\quad C_{n+1}=\sum _{i=0}^{n}C_{i}\,C_{n-i}\quad {\text{for }}n\geq 0.}
6318:{\displaystyle C_{0}=1\quad {\text{and}}\quad C_{n+1}=\sum _{i=0}^{n}C_{i}\,C_{n-i}\quad {\text{for }}n\geq 0}
5623:
1621:
XXXYYY XYXXYY XYXYXY XXYYXY XXYXYY
12448:
10473:
5606:
There is a simple bijection between these two marked triangulations: We can either collapse the triangle in
10252:
10232:
A. de Segner, Enumeratio modorum, quibus figurae planae rectilineae per diagonales dividuntur in triangula.
5100:
In Figure 3, the black dot indicates the point where the path first crosses the diagonal. The black edge is
938:{\displaystyle C_{0}=1\quad {\text{and}}\quad C_{n}={\frac {2(2n-1)}{n+1}}C_{n-1}\quad {\text{for }}n>0.}
12114:
11764:
11433:
11226:
9963:
9493:
9010:
8876:
2705:
810:{\displaystyle C_{0}=1\quad {\text{and}}\quad C_{n}=\sum _{i=1}^{n}C_{i-1}C_{n-i}\quad {\text{for }}n>0}
94:
12290:
12149:
11980:
11794:
11784:
11438:
11418:
10664:
9978:
1688:
1039:
12119:
10158:
Feng, Qi; Bai-Ni, Guo (2017), "Integral
Representations of the Catalan Numbers and Their Applications",
3084:; they involve literally counting a collection of some kind of object to arrive at the correct formula.
582:, which is not immediately obvious from the first formula given. This expression forms the basis for a
12781:
12239:
11862:
11704:
11619:
11428:
11410:
11304:
11294:
11284:
11120:
10719:
10043:
8697:{\displaystyle \det {\begin{bmatrix}2&5&14\\5&14&42\\14&42&132\end{bmatrix}}=4}
7695:
7659:
7623:
7587:
7551:
4595:
3369:
3363:
The recurrence relation given above can then be summarized in generating function form by the relation
1973:
12144:
5167:
on the diagonal. Alternatively, reverse the original algorithm to look for the first edge that passes
12367:
11912:
11533:
11319:
11314:
11309:
11299:
11276:
9264:
and Nathan
Reading have given a generalized Catalan number associated to any finite crystallographic
1595:
1429:. Since the 1D random walk is recurrent, the probability that the walker eventually arrives at -1 is
12124:
10445:
Gheorghiciuc, Irina; Orelowitz, Gidon (2020). "Super-Catalan
Numbers of the Third and Fourth Kind".
8937:
8902:
8861:
6127:
1795:
79:
11789:
11699:
11352:
9330:
8972:
3459:, the generating function relation can be algebraically solved to yield two solution possibilities
7390:
12478:
12443:
12229:
12139:
12013:
11988:
11897:
11887:
11609:
11499:
11481:
11401:
10745:
9988:
5435:
2101:, they are 1432, 2143, 2413, 2431, 3142, 3214, 3241, 3412, 3421, 4132, 4213, 4231, 4312 and 4321.
10638:
10632:
10360:
Stanley, Richard P. (2021). "Enumerative and
Algebraic Combinatorics in the 1960's and 1970's".
10286:
5511:
This proof uses the triangulation definition of
Catalan numbers to establish a relation between
3432:
in other words, this equation follows from the recurrence relation by expanding both sides into
431:
12738:
12008:
11882:
11513:
11289:
11069:
10996:
10403:
4642:
path crosses the main diagonal and touches the next higher diagonal (red in the illustration).
1953:
1709:
10820:"Equivalence of Three Catalan Number Interpretations" from The Wolfram Demonstrations Project
5135:
Figure 4. All monotonic paths in a 3×3 grid, illustrating the exceedance-decreasing algorithm.
12702:
12342:
11993:
11847:
11774:
10929:
10699:
10064:
9973:
6039:
3093:
2115:
1905:
40:
20:
10682:
Koshy, Thomas & Zhenguang Gao (2011) "Some divisibility properties of Catalan numbers",
7443:
12635:
12529:
12493:
12234:
11957:
11937:
11754:
11423:
11211:
10737:
10683:
10642:
10524:
10136:
10013:
9302:
9275:
9268:, namely the number of fully commutative elements of the group; in terms of the associated
9209:
9016:
8864:, who discovered the connection to parenthesized expressions during his exploration of the
7820:
6475:
6176:
6084:
5404:
2718:
1405:
11714:
11183:
8869:
7950:
6446:
2969:
2389:
2317:
2279:
2241:
1376:
8:
12357:
12221:
12216:
12184:
11947:
11922:
11917:
11892:
11822:
11818:
11749:
11639:
11471:
11267:
11236:
9183:
9157:
8065:
7904:
7774:
7525:
7499:
7469:
5548:
sides and a triangulation, mark one of its sides as the base, and also orient one of its
5480:
5074:
Starting from the bottom left, follow the path until it first travels above the diagonal.
5044:. A generalized version of this proof can be found in a paper of Rukavicka Josef (2011).
3278:
3096:
3077:
2829:
2127:
2084:
1742:
691:
10646:
6604:
5159:, which originally was the first horizontal step ending on the diagonal, has become the
1045:
12760:
12514:
12509:
12423:
12397:
12295:
12274:
12046:
11927:
11877:
11799:
11769:
11709:
11476:
11456:
11387:
11100:
10762:
10578:
10558:
10528:
10502:
10477:
10446:
10425:
10361:
10186:
10140:
10114:
10087:
9968:
9949:
9241:
8091:
7930:
7800:
7754:
7734:
7423:
7403:
6647:
6627:
6367:
6347:
3448:
2963:
2809:
2789:
2769:
2749:
1760:
1523:
47:
11644:
10346:
10018:
2686:
1698:, for example, we have the following five different parenthesizations of four factors:
12756:
12654:
12599:
12453:
12428:
12402:
11857:
11852:
11779:
11759:
11744:
11466:
11448:
11367:
11357:
11342:
11105:
10796:
10723:
10670:
10650:
10603:
10532:
10277:
10144:
9943:
7771:
Y's if and only if prepending an X to the Dyck word gives a dominating sequence with
5000:{\displaystyle C_{n}={2n \choose n}-{2n \choose n+1}={\frac {1}{n+1}}{2n \choose n}.}
3456:
2943:{\displaystyle 1234,1233,1232,1231,1223,1222,1221,1212,1211,1123,1122,1121,1112,1111}
12179:
10582:
7817:
Y's, so we can count the former by instead counting the latter. In particular, when
5047:
3602:
From the two possibilities, the second must be chosen because only the second gives
12690:
12483:
12069:
12041:
12031:
12023:
11907:
11872:
11867:
11834:
11528:
11491:
11382:
11377:
11372:
11362:
11334:
11221:
11168:
11125:
11064:
10799:
10568:
10512:
10342:
10309:
10281:
10273:
10208:
10167:
10124:
10079:
2962:. Record the values at only the X's. Compared to the similar representation of the
11173:
10313:
5107:
1866:
12666:
12555:
12488:
12414:
12337:
12311:
12129:
11842:
11634:
11604:
11594:
11589:
11255:
11163:
11110:
10954:
10894:
10733:
10520:
10424:
Chen, Xin; Wang, Jane (2012). "The super Catalan numbers S(m, m + s) for s ≤ 4".
10327:
10132:
10008:
9983:
8868:
puzzle. The reflection counting trick (second proof) for Dyck words was found by
8865:
5131:
4535:
3674:
3081:
1684:
12671:
12539:
12524:
12388:
12352:
12327:
12203:
12174:
12159:
12036:
11932:
11902:
11629:
11461:
11059:
11054:
11049:
11021:
11006:
10919:
10904:
10882:
10869:
10783:
10628:
10617:
10613:
10516:
10128:
10033:
10003:
8857:
8844:
7493:
5203:
equally sized classes, corresponding to the possible exceedances between 0 and
1886:
67:
10573:
10546:
5432:
that brings an initial subsequence to equality, and configure the sequence as
1708:
Successive applications of a binary operator can be represented in terms of a
12775:
12594:
12578:
12519:
12473:
12169:
12154:
12064:
11347:
11216:
11178:
11135:
11016:
11001:
10991:
10949:
10939:
10914:
10837:
9958:
9265:
8899:
For instance, Ming used the Catalan sequence to express series expansions of
8126:
8108:
Y's that are dominating, each of which corresponds to exactly one Dyck word.
7386:
6078:
3092:
We first observe that all of the combinatorial problems listed above satisfy
3076:
solves the combinatorial problems listed above. The first proof below uses a
1772:
1677:
1627:
1515:
55:
9258:, and it is an open problem to find a general combinatorial interpretation.
8882:
In 1988, it came to light that the Catalan number sequence had been used in
12630:
12619:
12534:
12372:
12347:
12264:
12164:
12134:
12109:
12093:
11998:
11965:
11688:
11599:
11538:
11115:
11011:
10944:
10924:
10899:
10465:
9998:
9261:
3433:
1934:
1901:
1876:
1838:
1824:
for a sentence (assuming binary branching), in natural language processing.
28:
10264:
Dershowitz, Nachum; Zaks, Shmuel (1980), "Enumerations of ordered trees",
8475:
Taken together, these two conditions uniquely define the Catalan numbers.
1873:
This can be represented by listing the Catalan elements by column height:
12589:
12464:
12269:
11733:
11624:
11579:
11574:
11324:
11231:
11130:
10959:
10934:
10909:
10300:
Dvoretzky, Aryeh; Motzkin, Theodore (1947), "A problem of arrangements",
10209:"An efficient representation for solving Catalan number related problems"
9269:
8158:
2148:
1957:
684:
71:
44:
10826:
10172:
10091:
8850:
The Quick Method for Obtaining the Precise Ratio of Division of a Circle
1767:
12726:
12707:
12003:
11614:
10547:"Counting symmetry: classes of dissections of a convex regular polygon"
9144:
5192:, and so on, down to zero. In other words, we have split up the set of
4848:{\displaystyle {n-1+n+1 \choose n-1}={2n \choose n-1}={2n \choose n+1}}
4567:
We count the number of paths which start and end on the diagonal of an
2202:
1821:
78:
defined objects. They are named after the French-Belgian mathematician
8605:{\displaystyle \det {\begin{bmatrix}2&5\\5&14\end{bmatrix}}=3}
6785:{\displaystyle B_{n+1}-C_{n+1}=\sum _{i=0}^{n}{2i+1 \choose i}C_{n-i}}
5352:, and the last column displays all paths no higher than the diagonal.
1027:-th Catalan number and the expression on the right tends towards 1 as
560:{\displaystyle {\tbinom {2n}{n+1}}={\tfrac {n}{n+1}}{\tbinom {2n}{n}}}
12332:
12259:
12251:
12056:
11970:
11088:
10804:
10713:
10481:
10083:
10028:
7522:
X's and Y's in a circle. Repeatedly removing XY pairs leaves exactly
1584:
75:
6112:
pairs of brackets. We denote a (possibly empty) correct string with
12433:
10767:
10507:
10451:
10366:
10119:
8887:
3230:
of length ≥ 2 can be written in a unique way in the form
1897:
1016:{\displaystyle C_{n}\sim {\frac {4^{n}}{n^{3/2}{\sqrt {\pi }}}}\,,}
83:
63:
10563:
10430:
8055:{\displaystyle \textstyle {\frac {1}{2n+1}}{2n+1 \choose n}=C_{n}}
1549:
12438:
12097:
12091:
5026:
This bijective proof provides a natural explanation for the term
3673:
The square root term can be expanded as a power series using the
1673:
579:
9009:
The Catalan numbers can be interpreted as a special case of the
8478:
Another feature unique to the Catalan–Hankel matrix is that the
6443:. A balanced string can also be uniquely decomposed into either
5326:{\displaystyle \textstyle C_{n}={\frac {1}{n+1}}{2n \choose n}.}
2693:
rectangle. In other words, it is the number of ways the numbers
1499:{\displaystyle \sum _{n=0}^{\infty }{\frac {C_{n}}{2^{2n+1}}}=1}
10400:"Ming Antu, the First Inventor of Catalan Numbers in the World"
9180:, this is just two times the ordinary Catalan numbers, and for
7584:. This sequence is dominating, but none of its circular shifts
5010:
In terms of Dyck words, we start with a (non-Dyck) sequence of
11153:
10250:
On Generalized Dyck Paths, Electronic Journal of Combinatorics
5155:
when the algorithm is applied to it. Indeed, the (black) edge
5123:. In fact, the algorithm causes the exceedance to decrease by
1912:
and the number of different ways that this can be achieved is
8883:
6026:{\displaystyle {\frac {(2n)!}{n!}}=2^{n}(2n-1)!!=(4n-2)!!!!.}
1362:{\displaystyle \sum _{n=0}^{\infty }{\frac {C_{n}}{4^{n}}}=2}
93:-th Catalan number can be expressed directly in terms of the
9139:
is a generalization of the Catalan numbers. These are named
8332:
then the determinant is still 1, regardless of the value of
7849:, there is exactly one dominating circular shift. There are
5776:{\displaystyle \textstyle {\frac {4n-2}{n+1}}C_{n-1}=C_{n}.}
10718:, Cambridge Studies in Advanced Mathematics, vol. 62,
10692:"The 18th century Chinese discovery of the Catalan numbers"
10383:"The 18th century Chinese discovery of the Catalan numbers"
9918:
1090:; all others are even. The only prime Catalan numbers are
302:
298:
1, 1, 2, 5, 14, 42, 132, 429, 1430, 4862, 16796, 58786, ...
8856:
The Catalan sequence was described in the 18th century by
7292:{\displaystyle C_{i}=2{\binom {2i}{i}}-{\binom {2i+1}{i}}}
2183:
is the number of ways to tile a stairstep shape of height
2094:, these permutations are 132, 213, 231, 312 and 321. For
673:{\displaystyle C_{n}={\frac {1}{2n+1}}{2n+1 \choose n}\,,}
461:
which is equivalent to the expression given above because
9151:, which sometimes are also called super-Catalan numbers.
7713:
7710:
7707:
7704:
7677:
7674:
7671:
7668:
7641:
7638:
7635:
7632:
7605:
7602:
7599:
7596:
7569:
7566:
7563:
7560:
5111:
Figure 3. The green and red portions are being exchanged.
1518:
whose solution is given by the Catalan numbers. The book
82:, though they were previously discovered in the 1730s by
6601:
Any incorrect (non-Catalan) balanced string starts with
2712:
123 124 125 134 135 456 356 346 256 246
2162:
is also the number of noncrossing partitions of the set
10794:
10785:
Catalan addendum to Enumerative Combinatorics, Volume 2
9329:
The Catalan numbers are a solution of a version of the
8722:
8630:
8565:
8540:{\displaystyle \det {\begin{bmatrix}2\end{bmatrix}}=2}
8517:
8361:
8190:
7983:
7856:
7370:{\displaystyle C_{i}={\frac {1}{i+1}}{\binom {2i}{i}}}
6391:
5710:
5362:
5260:
5214:
5185:, which is equal to the number of paths of exceedance
4600:
4531:
Method of images § Mathematics for discrete cases
2220:
is the number of ways to form a "mountain range" with
1113:
The Catalan numbers have the integral representations
1036:
asymptotic growth of the central binomial coefficients
529:
509:
469:
11817:
10756:
10444:
10234:
Novi commentarii academiae scientiarum Petropolitanae
10216:
International Journal of Pure and Applied Mathematics
9692:
9497:
9364:
9305:
9278:
9244:
9212:
9186:
9160:
9060:
9019:
8975:
8940:
8905:
8713:
8621:
8556:
8508:
8352:
8181:
8094:
8068:
7982:
7953:
7933:
7907:
7855:
7823:
7803:
7777:
7757:
7737:
7698:
7662:
7626:
7590:
7554:
7528:
7502:
7472:
7446:
7426:
7406:
7308:
7209:
7127:
7045:
6804:
6673:
6650:
6630:
6607:
6591:{\displaystyle B_{n+1}=2\sum _{i=0}^{n}B_{i}C_{n-i}.}
6515:
6478:
6449:
6390:
6370:
6350:
6209:
6179:
6130:
6087:
6042:
5920:
5794:
5709:
5626:
5483:
5438:
5407:
5361:
5259:
5213:
5033:
appearing in the denominator of the formula for
4867:
4718:
4598:
4377:
3684:
3611:
3536:
3468:
3372:
3353:{\displaystyle c(x)=\sum _{n=0}^{\infty }C_{n}x^{n}.}
3290:
3107:
3004:
2995:
There are several ways of explaining why the formula
2972:
2858:
2832:
2812:
2792:
2772:
2752:
2721:
2392:
2320:
2282:
2244:
1435:
1408:
1379:
1307:
1122:
1048:
954:
829:
702:
598:
467:
434:
421:{\displaystyle C_{n}={2n \choose n}-{2n \choose n+1}}
339:
106:
12202:
9939:
9054:
The two-parameter sequence of non-negative integers
7976:
circular shifts is dominating. Therefore there are
5901:{\displaystyle (2n)!=(2n)!!(2n-1)!!=2^{n}n!(2n-1)!!}
5174:
This implies that the number of paths of exceedance
3066:{\displaystyle C_{n}={\frac {1}{n+1}}{2n \choose n}}
8890:by 1730. That is when he started to write his book
8304:Moreover, if the indexing is "shifted" so that the
7389:interpretation of the Catalan numbers and uses the
3591:{\displaystyle c(x)={\frac {1-{\sqrt {1-4x}}}{2x}}}
3523:{\displaystyle c(x)={\frac {1+{\sqrt {1-4x}}}{2x}}}
10759:Super-Catalan Numbers of the Third and Fourth Kind
9924:
9318:
9291:
9250:
9230:
9198:
9172:
9131:
9032:
8993:
8961:
8926:
8825:
8696:
8604:
8539:
8464:
8293:
8100:
8080:
8054:
7968:
7939:
7919:
7893:
7841:
7809:
7789:
7763:
7743:
7720:
7684:
7648:
7612:
7576:
7540:
7514:
7484:
7458:
7432:
7412:
7369:
7291:
7192:
7110:
7021:
6784:
6656:
6636:
6616:
6590:
6498:
6464:
6435:
6376:
6356:
6317:
6192:
6165:
6100:
6061:
6025:
5900:
5775:
5691:
5495:
5469:
5420:
5393:
5325:
5245:
4999:
4847:
4630:
4514:
4363:
3661:
3590:
3522:
3421:
3352:
3215:
3065:
2978:
2942:
2844:
2818:
2798:
2778:
2758:
2734:
2407:
2335:
2297:
2259:
1498:
1421:
1394:
1361:
1290:
1057:
1015:
937:
809:
683:which can be directly interpreted in terms of the
672:
559:
450:
420:
275:
11201:
10326:Dershowitz, Nachum; Zaks, Shmuel (January 1990).
7361:
7343:
7283:
7259:
7247:
7229:
7184:
7160:
7102:
7078:
6760:
6736:
4988:
4970:
4940:
4914:
4902:
4884:
4839:
4813:
4801:
4775:
4763:
4722:
4492:
4474:
4331:
4313:
3768:
3745:
3057:
3039:
1645:pairs of parentheses which are correctly matched:
660:
636:
412:
386:
374:
356:
159:
141:
12773:
10634:Time Travel and Other Mathematical Bewilderments
10299:
9132:{\displaystyle {\frac {(2m)!(2n)!}{(m+n)!m!n!}}}
8714:
8622:
8557:
8509:
8353:
8182:
3626:
1509:
11087:
10637:, New York: W.H. Freeman and Company, pp.
10206:
7193:{\displaystyle C_{i}=2B_{i}-{\binom {2i+1}{i}}}
7111:{\displaystyle 2B_{i}-C_{i}={\binom {2i+1}{i}}}
6436:{\displaystyle \textstyle B_{n}={2n \choose n}}
5253:monotonic paths, we obtain the desired formula
43:of a 5-element set (below, the other 10 of the
10881:
10867:
10757:Gheorghiciuc, Irina; Orelowitz, Gidon (2020),
10325:
10263:
5178:is equal to the number of paths of exceedance
5088:Swap the portion of the path occurring before
10853:
10062:
9336:
9040:is the number of ways for a candidate A with
8032:
8008:
7884:
7860:
7496:. To see this, arrange the given sequence of
6426:
6408:
5603:such marked triangulations for a given base.
5572:such marked triangulations for a given base.
5384:
5366:
5355:Using Dyck words, start with a sequence from
5313:
5295:
5236:
5218:
4621:
4603:
2062:being the identity for one-element sequences.
1923:. The following hexagons illustrate the case
550:
532:
498:
472:
12689:
11039:
5335:Figure 4 illustrates the situation for
1641:counts the number of expressions containing
948:Asymptotically, the Catalan numbers grow as
10544:
8875:The name “Catalan numbers” originated from
7894:{\displaystyle \textstyle {2n+1 \choose n}}
6200:immediately gives the recursive definition
5394:{\displaystyle \textstyle {\binom {2n}{n}}}
5059:of the path is defined to be the number of
4585:up steps. Since we can choose which of the
1553:Lattice of the 14 Dyck words of length 8 –
11154:Possessing a specific set of other numbers
10977:
10860:
10846:
10492:
10157:
10104:
10063:Koshy, Thomas; Salmassi, Mohammad (2006).
6081:interpretation of the Catalan numbers, so
4592:steps are up or right, there are in total
3662:{\displaystyle C_{0}=\lim _{x\to 0}c(x)=1}
1752:leaves, or, equivalently, with a total of
12617:
11564:
10766:
10743:
10572:
10562:
10506:
10450:
10429:
10365:
10285:
10171:
10118:
10065:"Parity and primality of Catalan numbers"
7947:Y's. For each of these, only one of the
6927:
6283:
6108:is the number of ways to correctly match
5246:{\displaystyle \textstyle {2n \choose n}}
5151:, there is exactly one path which yields
4508:
4353:
3181:
2806:, or decrease by any number (to at least
2002:is defined recursively as follows: write
1900:by connecting vertices with non-crossing
1278:
1203:
1196:
1009:
666:
444:
206:
10689:
10624:. New York: Copernicus, pp. 96–106.
10423:
10417:
8843:
8316:entry is filled with the Catalan number
6624:, and the remaining string has one more
5692:{\displaystyle (4n+2)C_{n}=(n+2)C_{n+1}}
5130:
5106:
5046:
4682:, all bad paths after reflection end at
4534:
1875:
1766:
1626:Re-interpreting the symbol X as an open
1548:
27:
10781:
10747:A Catalan–Hankel Determinant Evaluation
10711:
10627:
10359:
10328:"The Cycle Lemma and Some Applications"
10207:Črepinšek, Matej; Mernik, Luka (2009).
9299:corresponds to the root system of type
6173:, summing over the possible lengths of
2990:
2950:. From a Dyck path, start a counter at
584:proof of the correctness of the formula
12774:
12725:
9147:. These should not confused with the
4709:The number of bad paths is therefore:
4694:. Because every monotonic path in the
4670:right steps. So, instead of reaching
3281:for the Catalan numbers is defined by
1807:encoding general trees as binary trees
1023:in the sense that the quotient of the
12724:
12688:
12652:
12616:
12576:
12201:
12090:
11816:
11731:
11686:
11563:
11253:
11200:
11152:
11086:
11038:
10976:
10880:
10841:
10795:
10662:
9994:List of factorial and binomial topics
6795:Also, from the definitions, we have:
5614:to a triangle and mark its new side.
5077:Continue to follow the path until it
1908:). The number of triangles formed is
1856:The following diagrams show the case
11254:
10380:
5139:It can be seen that this process is
5085:the first such edge that is reached.
1514:There are many counting problems in
12653:
10476:, Providence, RI, 2007, pp 63–131.
8848:Catalan numbers in Mingantu's book
7032:Therefore, as this is true for all
5070:less than the one we started with.
5051:Figure 2. A path with exceedance 5.
3080:. The other proofs are examples of
1779:=14 full binary trees with 5 leaves
1520:Enumerative Combinatorics: Volume 2
220:
13:
12577:
10832:Partition related number triangles
9370:
9004:
8012:
7864:
7701:
7665:
7629:
7593:
7557:
7396:We call a sequence of X's and Y's
7347:
7263:
7233:
7164:
7082:
6740:
6412:
5370:
5299:
5222:
4974:
4918:
4888:
4817:
4779:
4726:
4607:
4478:
4448:
4317:
4287:
4197:
4084:
3947:
3818:
3749:
3737:
3322:
3043:
2201:
2170:in which every block is of size 2.
1933:
1865:
1759:
1452:
1324:
640:
589:Another alternative expression is
536:
476:
390:
360:
145:
14:
12808:
10775:
10715:Enumerative combinatorics. Vol. 2
10666:Catalan Numbers with Applications
10335:European Journal of Combinatorics
7721:{\displaystyle {\mathit {YXXYX}}}
7685:{\displaystyle {\mathit {XYXXY}}}
7649:{\displaystyle {\mathit {YXYXX}}}
7613:{\displaystyle {\mathit {XYXYX}}}
7577:{\displaystyle {\mathit {XXYXY}}}
5092:with the portion occurring after
4631:{\displaystyle {\tbinom {2n}{n}}}
3422:{\displaystyle c(x)=1+xc(x)^{2};}
3256:with (possibly empty) Dyck words
2075:is the number of permutations of
1373:arrive at the trap state at time
1083:that are odd are those for which
583:
12797:Eponymous numbers in mathematics
12755:
12363:Perfect digit-to-digit invariant
11732:
10825:
10545:Bowman, D.; Regev, Alon (2014).
9942:
8111:
6124:can be uniquely decomposed into
5115:The exceedance has dropped from
4638:monotonic paths of this type. A
2954:. An X increases the counter by
1794:is the number of non-isomorphic
1665:is the number of different ways
1034:This can be proved by using the
690:The Catalan numbers satisfy the
10538:
10486:
10459:
10438:
10392:
10374:
10353:
10319:
10293:
10072:The College Mathematics Journal
9047:votes to lead candidate B with
8886:by the Mongolian mathematician
6333:be a balanced string of length
6300:
6232:
6226:
5506:
4524:
3198:
3130:
3124:
2028:are shorter sequences, and set
1841:along the edges of a grid with
920:
852:
846:
792:
725:
719:
258:
10830:Learning materials related to
10602:. Cambridge University Press,
10287:2027/uiuo.ark:/13960/t3kw6z60d
10257:
10242:
10226:
10200:
10191:
10180:
10151:
10098:
10056:
9889:
9877:
9859:
9847:
9841:
9824:
9812:
9803:
9800:
9789:
9777:
9768:
9763:
9752:
9740:
9731:
9725:
9713:
9710:
9698:
9643:
9631:
9625:
9599:
9596:
9570:
9562:
9536:
9530:
9518:
9515:
9503:
9108:
9096:
9088:
9079:
9073:
9064:
8988:
8982:
8962:{\displaystyle \sin(4\alpha )}
8956:
8947:
8927:{\displaystyle \sin(2\alpha )}
8921:
8912:
8161:1, regardless of the value of
7380:
6997:
6968:
6651:
6631:
6611:
6490:
6479:
6456:
6450:
6371:
6351:
6166:{\displaystyle c=(c_{1})c_{2}}
6150:
6137:
6072:
6005:
5990:
5978:
5963:
5933:
5924:
5889:
5874:
5846:
5831:
5822:
5813:
5804:
5795:
5670:
5658:
5642:
5627:
5464:
5458:
5445:
5439:
5147:whose exceedance is less than
5081:the diagonal again. Denote by
5021:
4387:
4381:
4237:
4225:
4217:
4208:
4146:
4134:
4123:
4108:
4050:
4037:
4028:
4016:
3989:
3974:
3965:
3955:
3909:
3896:
3866:
3851:
3836:
3826:
3787:
3774:
3650:
3644:
3633:
3546:
3540:
3478:
3472:
3407:
3400:
3382:
3376:
3300:
3294:
3087:
1630:and Y as a close parenthesis,
887:
872:
567:. This expression shows that
319:An alternative expression for
286:The first Catalan numbers for
200:
188:
180:
171:
1:
12787:Factorial and binomial topics
11202:Expressible via specific sums
10592:
10474:American Mathematical Society
10347:10.1016/S0195-6698(13)80053-4
10314:10.1215/s0012-7094-47-01423-3
10039:Wedderburn–Etherington number
8994:{\displaystyle \sin(\alpha )}
3226:For example, every Dyck word
2766:, and can increase by either
2231:
1741:is the number of full binary
1510:Applications in combinatorics
314:
95:central binomial coefficients
10782:Stanley, Richard P. (1998),
10712:Stanley, Richard P. (1999),
10598:Stanley, Richard P. (2015),
10278:10.1016/0012-365x(80)90168-5
8141:entry is the Catalan number
6344:contains an equal number of
6036:Applying the recursion with
5055:Given a monotonic path, the
1616:XXYY XYXY
7:
12291:Multiplicative digital root
10669:, Oxford University Press,
9935:
9149:Schröder–Hipparchus numbers
7731:A string is a Dyck word of
7385:This proof is based on the
6077:This proof is based on the
5470:{\displaystyle (F)X_{d}(L)}
2746:sequences that start with
1837:is the number of monotonic
1689:matrix chain multiplication
10:
12813:
11687:
10720:Cambridge University Press
10517:10.1016/j.disc.2019.111808
10129:10.1016/j.disc.2019.111808
10024:Schröder–Hipparchus number
9337:Catalan k-fold convolution
8839:
7393:of Dvoretzky and Motzkin.
4577:grid. All such paths have
4528:
2700:can be arranged in a 2-by-
2016:is the largest element in
1820:is the number of possible
1676:(or the number of ways of
1672:factors can be completely
451:{\displaystyle n\geq 0\,,}
18:
16:Recursive integer sequence
12792:Enumerative combinatorics
12751:
12734:
12720:
12698:
12684:
12662:
12648:
12626:
12612:
12585:
12572:
12548:
12502:
12462:
12413:
12387:
12368:Perfect digital invariant
12320:
12304:
12283:
12250:
12215:
12211:
12197:
12105:
12086:
12055:
12022:
11979:
11956:
11943:Superior highly composite
11833:
11829:
11812:
11740:
11727:
11695:
11682:
11570:
11559:
11521:
11512:
11490:
11447:
11409:
11400:
11333:
11275:
11266:
11262:
11249:
11207:
11196:
11159:
11148:
11096:
11082:
11045:
11034:
10987:
10972:
10890:
10876:
10574:10.1016/j.aam.2014.01.004
10302:Duke Mathematical Journal
10197:Stanley p.221 example (e)
9964:Bertrand's ballot theorem
9345:-fold convolution, where
9011:Bertrand's ballot theorem
1301:which immediately yields
1072:The only Catalan numbers
56:combinatorial mathematics
11981:Euler's totient function
11765:Euler–Jacobi pseudoprime
11040:Other polynomial numbers
10744:Egecioglu, Omer (2009),
10248:Rukavicka Josef (2011),
10050:
9331:Hausdorff moment problem
2958:and a Y decreases it by
2742:is the number of length
2689:whose diagram is a 2-by-
1796:ordered (or plane) trees
1712:, by labeling each leaf
1067:via generating functions
1040:Stirling's approximation
19:Not to be confused with
11795:Somer–Lucas pseudoprime
11785:Lucas–Carmichael number
11620:Lazy caterer's sequence
10690:Larcombe, P.J. (1999).
10044:Wigner's semicircle law
9979:Catalan–Mersenne number
7901:sequences with exactly
6062:{\displaystyle C_{0}=1}
5556:total edges. There are
2687:standard Young tableaux
1852:Y stands for "move up".
11670:Wedderburn–Etherington
11070:Lucky numbers of Euler
10817:. Still more examples.
10663:Koshy, Thomas (2008),
9926:
9320:
9293:
9252:
9232:
9200:
9174:
9133:
9034:
8995:
8963:
8928:
8862:Eugène Charles Catalan
8853:
8827:
8698:
8606:
8541:
8488:submatrix starting at
8466:
8295:
8102:
8082:
8062:distinct sequences of
8056:
7970:
7941:
7921:
7895:
7843:
7811:
7791:
7765:
7745:
7722:
7686:
7650:
7614:
7578:
7542:
7516:
7486:
7460:
7459:{\displaystyle m>n}
7434:
7414:
7371:
7293:
7194:
7112:
7023:
6967:
6916:
6866:
6786:
6732:
6658:
6638:
6618:
6592:
6558:
6500:
6466:
6437:
6378:
6358:
6319:
6272:
6194:
6167:
6102:
6063:
6027:
5902:
5777:
5693:
5497:
5477:. The new sequence is
5471:
5422:
5395:
5327:
5247:
5136:
5112:
5052:
5001:
4849:
4649:Since there are still
4632:
4564:
4516:
4452:
4365:
4291:
4201:
4088:
3951:
3822:
3741:
3663:
3592:
3524:
3423:
3354:
3326:
3217:
3170:
3067:
2980:
2944:
2846:
2820:
2800:
2780:
2760:
2736:
2409:
2337:
2299:
2261:
2206:
2116:noncrossing partitions
1938:
1896:sides can be cut into
1881:
1870:
1780:
1764:
1569:
1500:
1456:
1423:
1396:
1363:
1328:
1292:
1059:
1017:
939:
811:
759:
674:
561:
452:
422:
277:
239:
80:Eugène Charles Catalan
70:that occur in various
51:
41:noncrossing partitions
11958:Prime omega functions
11775:Frobenius pseudoprime
11565:Combinatorial numbers
11434:Centered dodecahedral
11227:Primary pseudoperfect
10700:Mathematical Spectrum
9927:
9321:
9319:{\displaystyle A_{n}}
9294:
9292:{\displaystyle C_{n}}
9253:
9233:
9231:{\displaystyle m=2,3}
9201:
9175:
9141:super-Catalan numbers
9134:
9035:
9033:{\displaystyle C_{n}}
8996:
8964:
8929:
8847:
8828:
8699:
8607:
8542:
8467:
8296:
8103:
8083:
8057:
7971:
7942:
7922:
7896:
7844:
7842:{\displaystyle m=n+1}
7812:
7792:
7766:
7746:
7723:
7687:
7651:
7615:
7579:
7543:
7517:
7487:
7461:
7435:
7415:
7372:
7294:
7195:
7113:
7024:
6947:
6896:
6846:
6787:
6712:
6659:
6639:
6619:
6593:
6538:
6501:
6499:{\displaystyle )c'(b}
6467:
6438:
6379:
6359:
6320:
6252:
6195:
6193:{\displaystyle c_{1}}
6168:
6116:and its inverse with
6103:
6101:{\displaystyle C_{n}}
6064:
6028:
5903:
5778:
5694:
5498:
5472:
5423:
5421:{\displaystyle X_{d}}
5396:
5328:
5248:
5196:monotonic paths into
5134:
5110:
5050:
5002:
4850:
4656:steps, there are now
4633:
4538:
4517:
4432:
4366:
4271:
4181:
4068:
3931:
3802:
3721:
3664:
3593:
3525:
3424:
3355:
3306:
3218:
3150:
3068:
2981:
2945:
2847:
2821:
2801:
2781:
2761:
2737:
2735:{\displaystyle C_{n}}
2410:
2338:
2300:
2262:
2205:
1937:
1906:polygon triangulation
1879:
1869:
1775:of order 4 with the C
1770:
1763:
1552:
1501:
1436:
1424:
1422:{\displaystyle C_{k}}
1397:
1364:
1308:
1293:
1060:
1031:approaches infinity.
1018:
940:
812:
739:
675:
562:
453:
423:
278:
219:
31:
12417:-composition related
12217:Arithmetic functions
11819:Arithmetic functions
11755:Elliptic pseudoprime
11439:Centered icosahedral
11419:Centered tetrahedral
10684:Mathematical Gazette
10495:Discrete Mathematics
10266:Discrete Mathematics
10107:Discrete Mathematics
10014:Narayana polynomials
9362:
9303:
9276:
9242:
9210:
9184:
9158:
9058:
9017:
8973:
8938:
8903:
8892:Ge Yuan Mi Lu Jie Fa
8711:
8619:
8554:
8506:
8350:
8179:
8092:
8066:
7980:
7969:{\displaystyle 2n+1}
7951:
7931:
7905:
7853:
7821:
7801:
7775:
7755:
7735:
7696:
7660:
7624:
7588:
7552:
7526:
7500:
7470:
7444:
7424:
7404:
7306:
7207:
7125:
7043:
6802:
6671:
6648:
6628:
6605:
6513:
6476:
6465:{\displaystyle (c)b}
6447:
6388:
6368:
6348:
6207:
6177:
6128:
6085:
6040:
5918:
5792:
5707:
5624:
5481:
5436:
5405:
5359:
5257:
5211:
4865:
4716:
4596:
4375:
3682:
3609:
3534:
3466:
3370:
3288:
3105:
3002:
2991:Proof of the formula
2979:{\displaystyle 1213}
2970:
2856:
2830:
2810:
2790:
2770:
2750:
2719:
2408:{\displaystyle n=3:}
2390:
2336:{\displaystyle n=2:}
2318:
2298:{\displaystyle n=1:}
2280:
2260:{\displaystyle n=0:}
2242:
1522:by combinatorialist
1433:
1406:
1395:{\displaystyle 2k+1}
1377:
1305:
1120:
1046:
952:
827:
700:
692:recurrence relations
596:
465:
432:
337:
104:
12343:Kaprekar's constant
11863:Colossally abundant
11750:Catalan pseudoprime
11650:Schröder–Hipparchus
11429:Centered octahedral
11305:Centered heptagonal
11295:Centered pentagonal
11285:Centered triangular
10885:and related numbers
10647:1988ttom.book.....G
10622:The Book of Numbers
10381:Larcombe, Peter J.
10173:10.3390/math5030040
9989:Fuss–Catalan number
9199:{\displaystyle m=n}
9173:{\displaystyle m=1}
8336:. For example, for
8165:. For example, for
8081:{\displaystyle n+1}
7920:{\displaystyle n+1}
7790:{\displaystyle n+1}
7541:{\displaystyle m-n}
7515:{\displaystyle m+n}
7485:{\displaystyle m-n}
5496:{\displaystyle LXF}
3279:generating function
3097:recurrence relation
3078:generating function
2845:{\displaystyle n=4}
2706:hook-length formula
2235:
2085:permutation pattern
1594:. A Dyck word is a
1244:
1165:
12761:Mathematics portal
12703:Aronson's sequence
12449:Smarandache–Wellin
12206:-dependent numbers
11913:Primitive abundant
11800:Strong pseudoprime
11790:Perrin pseudoprime
11770:Fermat pseudoprime
11710:Wolstenholme prime
11534:Squared triangular
11320:Centered decagonal
11315:Centered nonagonal
11310:Centered octagonal
11300:Centered hexagonal
10797:Weisstein, Eric W.
10239:(1758/59) 203–209.
9974:Catalan's triangle
9969:Binomial transform
9950:Mathematics portal
9922:
9917:
9864:
9648:
9447:
9316:
9289:
9248:
9228:
9196:
9170:
9129:
9030:
8991:
8959:
8924:
8854:
8823:
8811:
8694:
8682:
8602:
8590:
8537:
8525:
8462:
8450:
8291:
8279:
8098:
8078:
8052:
8051:
7966:
7937:
7917:
7891:
7890:
7839:
7807:
7787:
7761:
7741:
7718:
7682:
7646:
7610:
7574:
7538:
7512:
7482:
7456:
7430:
7410:
7367:
7289:
7190:
7108:
7019:
6782:
6654:
6634:
6617:{\displaystyle c)}
6614:
6588:
6496:
6462:
6433:
6432:
6374:
6354:
6315:
6190:
6163:
6098:
6069:gives the result.
6059:
6023:
5898:
5773:
5772:
5689:
5493:
5467:
5418:
5391:
5390:
5323:
5322:
5243:
5242:
5207:. Since there are
5137:
5113:
5053:
4997:
4845:
4628:
4626:
4565:
4512:
4361:
4359:
3659:
3640:
3588:
3520:
3449:quadratic equation
3419:
3350:
3213:
3063:
2976:
2940:
2842:
2816:
2796:
2776:
2756:
2732:
2405:
2333:
2295:
2257:
2233:
2207:
2143:never exceeds the
1939:
1882:
1871:
1781:
1765:
1730:. It follows that
1683:applications of a
1570:
1524:Richard P. Stanley
1496:
1419:
1392:
1359:
1288:
1227:
1151:
1058:{\displaystyle n!}
1055:
1013:
935:
807:
670:
557:
555:
526:
503:
448:
418:
273:
74:, often involving
52:
21:Catalan's constant
12782:Integer sequences
12769:
12768:
12747:
12746:
12716:
12715:
12680:
12679:
12644:
12643:
12608:
12607:
12568:
12567:
12564:
12563:
12383:
12382:
12193:
12192:
12082:
12081:
12078:
12077:
12024:Aliquot sequences
11835:Divisor functions
11808:
11807:
11780:Lucas pseudoprime
11760:Euler pseudoprime
11745:Carmichael number
11723:
11722:
11678:
11677:
11555:
11554:
11551:
11550:
11547:
11546:
11508:
11507:
11396:
11395:
11353:Square triangular
11245:
11244:
11192:
11191:
11144:
11143:
11078:
11077:
11030:
11029:
10968:
10967:
10729:978-0-521-56069-6
10676:978-0-19-533454-8
10608:978-1-107-42774-7
10501:(5): 111808, 11,
10113:(5): 111808, 11,
9913:
9863:
9685:
9647:
9445:
9365:
9251:{\displaystyle 4}
9127:
8101:{\displaystyle n}
8030:
8003:
7940:{\displaystyle n}
7882:
7810:{\displaystyle n}
7764:{\displaystyle n}
7744:{\displaystyle n}
7433:{\displaystyle n}
7413:{\displaystyle m}
7359:
7338:
7281:
7245:
7182:
7100:
6758:
6657:{\displaystyle )}
6637:{\displaystyle (}
6424:
6377:{\displaystyle )}
6357:{\displaystyle (}
6304:
6230:
5948:
5738:
5382:
5311:
5290:
5234:
5143:: given any path
4986:
4965:
4938:
4900:
4837:
4799:
4761:
4619:
4490:
4469:
4427:
4416:
4329:
4308:
4250:
4153:
4035:
3894:
3766:
3709:
3625:
3586:
3575:
3518:
3507:
3457:quadratic formula
3202:
3128:
3055:
3034:
2819:{\displaystyle 1}
2799:{\displaystyle 1}
2779:{\displaystyle 0}
2759:{\displaystyle 1}
2685:is the number of
2671:
2670:
2114:is the number of
1952:is the number of
1583:is the number of
1488:
1351:
1276:
1215:
1194:
1193:
1149:
1007:
1004:
924:
902:
850:
796:
723:
658:
631:
548:
525:
496:
410:
372:
291:= 0, 1, 2, 3, ...
262:
256:
214:
157:
136:
72:counting problems
12804:
12759:
12722:
12721:
12691:Natural language
12686:
12685:
12650:
12649:
12618:Generated via a
12614:
12613:
12574:
12573:
12479:Digit-reassembly
12444:Self-descriptive
12248:
12247:
12213:
12212:
12199:
12198:
12150:Lucas–Carmichael
12140:Harmonic divisor
12088:
12087:
12014:Sparsely totient
11989:Highly cototient
11898:Multiply perfect
11888:Highly composite
11831:
11830:
11814:
11813:
11729:
11728:
11684:
11683:
11665:Telephone number
11561:
11560:
11519:
11518:
11500:Square pyramidal
11482:Stella octangula
11407:
11406:
11273:
11272:
11264:
11263:
11256:Figurate numbers
11251:
11250:
11198:
11197:
11150:
11149:
11084:
11083:
11036:
11035:
10974:
10973:
10878:
10877:
10862:
10855:
10848:
10839:
10838:
10829:
10810:
10809:
10800:"Catalan Number"
10791:
10790:
10771:
10770:
10753:
10752:
10740:
10708:
10696:
10679:
10659:
10639:253–266 (Ch. 20)
10587:
10586:
10576:
10566:
10542:
10536:
10535:
10510:
10490:
10484:
10463:
10457:
10456:
10454:
10442:
10436:
10435:
10433:
10421:
10415:
10414:
10412:
10411:
10402:. Archived from
10396:
10390:
10389:
10387:
10378:
10372:
10371:
10369:
10357:
10351:
10350:
10332:
10323:
10317:
10316:
10297:
10291:
10290:
10289:
10261:
10255:
10246:
10240:
10230:
10224:
10223:
10213:
10204:
10198:
10195:
10189:
10184:
10178:
10176:
10175:
10155:
10149:
10147:
10122:
10102:
10096:
10095:
10084:10.2307/27646275
10069:
10060:
9952:
9947:
9946:
9931:
9929:
9928:
9923:
9921:
9920:
9914:
9911:
9901:
9900:
9896:
9865:
9862:
9831:
9796:
9766:
9759:
9693:
9686:
9683:
9673:
9672:
9668:
9649:
9646:
9615:
9586:
9565:
9552:
9498:
9484:
9483:
9482:
9481:
9464:
9463:
9462:
9461:
9446:
9444:
9437:
9436:
9418:
9417:
9407:
9400:
9399:
9381:
9380:
9354:
9344:
9325:
9323:
9322:
9317:
9315:
9314:
9298:
9296:
9295:
9290:
9288:
9287:
9257:
9255:
9254:
9249:
9237:
9235:
9234:
9229:
9205:
9203:
9202:
9197:
9179:
9177:
9176:
9171:
9138:
9136:
9135:
9130:
9128:
9126:
9094:
9062:
9050:
9046:
9039:
9037:
9036:
9031:
9029:
9028:
9013:. Specifically,
9000:
8998:
8997:
8992:
8968:
8966:
8965:
8960:
8933:
8931:
8930:
8925:
8832:
8830:
8829:
8824:
8816:
8815:
8703:
8701:
8700:
8695:
8687:
8686:
8611:
8609:
8608:
8603:
8595:
8594:
8546:
8544:
8543:
8538:
8530:
8529:
8498:
8492:has determinant
8491:
8487:
8471:
8469:
8468:
8463:
8455:
8454:
8342:
8335:
8331:
8315:
8300:
8298:
8297:
8292:
8284:
8283:
8171:
8164:
8156:
8140:
8125:
8107:
8105:
8104:
8099:
8087:
8085:
8084:
8079:
8061:
8059:
8058:
8053:
8050:
8049:
8037:
8036:
8035:
8026:
8011:
8004:
8002:
7985:
7975:
7973:
7972:
7967:
7946:
7944:
7943:
7938:
7926:
7924:
7923:
7918:
7900:
7898:
7897:
7892:
7889:
7888:
7887:
7878:
7863:
7848:
7846:
7845:
7840:
7816:
7814:
7813:
7808:
7796:
7794:
7793:
7788:
7770:
7768:
7767:
7762:
7750:
7748:
7747:
7742:
7727:
7725:
7724:
7719:
7717:
7716:
7691:
7689:
7688:
7683:
7681:
7680:
7655:
7653:
7652:
7647:
7645:
7644:
7619:
7617:
7616:
7611:
7609:
7608:
7583:
7581:
7580:
7575:
7573:
7572:
7547:
7545:
7544:
7539:
7521:
7519:
7518:
7513:
7491:
7489:
7488:
7483:
7466:, has precisely
7465:
7463:
7462:
7457:
7439:
7437:
7436:
7431:
7419:
7417:
7416:
7411:
7376:
7374:
7373:
7368:
7366:
7365:
7364:
7355:
7346:
7339:
7337:
7323:
7318:
7317:
7298:
7296:
7295:
7290:
7288:
7287:
7286:
7277:
7262:
7252:
7251:
7250:
7241:
7232:
7219:
7218:
7199:
7197:
7196:
7191:
7189:
7188:
7187:
7178:
7163:
7153:
7152:
7137:
7136:
7117:
7115:
7114:
7109:
7107:
7106:
7105:
7096:
7081:
7071:
7070:
7058:
7057:
7035:
7028:
7026:
7025:
7020:
7015:
7014:
6996:
6995:
6983:
6982:
6966:
6961:
6943:
6942:
6926:
6925:
6915:
6910:
6892:
6891:
6876:
6875:
6865:
6860:
6839:
6838:
6820:
6819:
6791:
6789:
6788:
6783:
6781:
6780:
6765:
6764:
6763:
6754:
6739:
6731:
6726:
6708:
6707:
6689:
6688:
6663:
6661:
6660:
6655:
6643:
6641:
6640:
6635:
6623:
6621:
6620:
6615:
6597:
6595:
6594:
6589:
6584:
6583:
6568:
6567:
6557:
6552:
6531:
6530:
6505:
6503:
6502:
6497:
6489:
6471:
6469:
6468:
6463:
6442:
6440:
6439:
6434:
6431:
6430:
6429:
6420:
6411:
6401:
6400:
6383:
6381:
6380:
6375:
6363:
6361:
6360:
6355:
6343:
6339:
6332:
6324:
6322:
6321:
6316:
6305:
6302:
6299:
6298:
6282:
6281:
6271:
6266:
6248:
6247:
6231:
6228:
6219:
6218:
6199:
6197:
6196:
6191:
6189:
6188:
6172:
6170:
6169:
6164:
6162:
6161:
6149:
6148:
6123:
6119:
6115:
6111:
6107:
6105:
6104:
6099:
6097:
6096:
6068:
6066:
6065:
6060:
6052:
6051:
6032:
6030:
6029:
6024:
5962:
5961:
5949:
5947:
5939:
5922:
5907:
5905:
5904:
5899:
5867:
5866:
5782:
5780:
5779:
5774:
5768:
5767:
5755:
5754:
5739:
5737:
5726:
5712:
5698:
5696:
5695:
5690:
5688:
5687:
5654:
5653:
5613:
5609:
5602:
5585:
5578:
5575:Given a polygon
5571:
5555:
5547:
5540:
5537:Given a polygon
5533:
5521:
5502:
5500:
5499:
5494:
5476:
5474:
5473:
5468:
5457:
5456:
5431:
5427:
5425:
5424:
5419:
5417:
5416:
5400:
5398:
5397:
5392:
5389:
5388:
5387:
5378:
5369:
5351:
5341:
5332:
5330:
5329:
5324:
5318:
5317:
5316:
5307:
5298:
5291:
5289:
5275:
5270:
5269:
5252:
5250:
5249:
5244:
5241:
5240:
5239:
5230:
5221:
5206:
5202:
5191:
5184:
5177:
5163:horizontal step
5158:
5154:
5150:
5146:
5126:
5122:
5118:
5103:
5095:
5091:
5084:
5069:
5043:
5032:
5017:
5013:
5006:
5004:
5003:
4998:
4993:
4992:
4991:
4982:
4973:
4966:
4964:
4950:
4945:
4944:
4943:
4937:
4926:
4917:
4907:
4906:
4905:
4896:
4887:
4877:
4876:
4854:
4852:
4851:
4846:
4844:
4843:
4842:
4836:
4825:
4816:
4806:
4805:
4804:
4798:
4787:
4778:
4768:
4767:
4766:
4760:
4749:
4725:
4705:
4693:
4681:
4669:
4662:
4655:
4637:
4635:
4634:
4629:
4627:
4625:
4624:
4615:
4606:
4591:
4584:
4580:
4576:
4562:
4550:
4521:
4519:
4518:
4513:
4507:
4506:
4497:
4496:
4495:
4486:
4477:
4470:
4468:
4454:
4451:
4446:
4428:
4426:
4418:
4417:
4403:
4394:
4370:
4368:
4367:
4362:
4360:
4352:
4351:
4336:
4335:
4334:
4325:
4316:
4309:
4307:
4293:
4290:
4285:
4267:
4266:
4251:
4249:
4223:
4203:
4200:
4195:
4174:
4170:
4169:
4154:
4152:
4132:
4107:
4106:
4090:
4087:
4082:
4064:
4063:
4036:
4034:
4015:
4014:
3998:
3973:
3972:
3953:
3950:
3945:
3921:
3917:
3916:
3895:
3893:
3886:
3885:
3875:
3850:
3849:
3824:
3821:
3816:
3795:
3794:
3773:
3772:
3771:
3762:
3758:
3748:
3740:
3735:
3710:
3696:
3668:
3666:
3665:
3660:
3639:
3621:
3620:
3597:
3595:
3594:
3589:
3587:
3585:
3577:
3576:
3562:
3553:
3529:
3527:
3526:
3521:
3519:
3517:
3509:
3508:
3494:
3485:
3454:
3446:
3428:
3426:
3425:
3420:
3415:
3414:
3359:
3357:
3356:
3351:
3346:
3345:
3336:
3335:
3325:
3320:
3273:
3264:
3252:
3229:
3222:
3220:
3219:
3214:
3203:
3200:
3197:
3196:
3180:
3179:
3169:
3164:
3146:
3145:
3129:
3126:
3117:
3116:
3082:bijective proofs
3072:
3070:
3069:
3064:
3062:
3061:
3060:
3051:
3042:
3035:
3033:
3019:
3014:
3013:
2985:
2983:
2982:
2977:
2961:
2957:
2953:
2949:
2947:
2946:
2941:
2851:
2849:
2848:
2843:
2825:
2823:
2822:
2817:
2805:
2803:
2802:
2797:
2785:
2783:
2782:
2777:
2765:
2763:
2762:
2757:
2745:
2741:
2739:
2738:
2733:
2731:
2730:
2703:
2699:
2692:
2684:
2663:
2660:
2657:
2654:
2650:
2646:
2643:
2640:
2637:
2633:
2629:
2626:
2622:
2618:
2615:
2611:
2605:
2602:
2598:
2595:
2592:
2589:
2585:
2582:
2579:
2576:
2573:
2570:
2566:
2563:
2560:
2557:
2553:
2550:
2547:
2544:
2541:
2538:
2535:
2532:
2529:
2526:
2523:
2518:
2515:
2512:
2509:
2506:
2503:
2500:
2497:
2494:
2491:
2488:
2485:
2482:
2479:
2476:
2473:
2470:
2467:
2464:
2461:
2458:
2455:
2452:
2449:
2446:
2443:
2440:
2437:
2434:
2431:
2428:
2425:
2422:
2419:
2414:
2412:
2411:
2406:
2378:
2375:
2371:
2365:
2362:
2359:
2356:
2353:
2350:
2347:
2342:
2340:
2339:
2334:
2304:
2302:
2301:
2296:
2266:
2264:
2263:
2258:
2236:
2234:Mountain Ranges
2232:
2227:
2223:
2219:
2197:
2190:
2186:
2182:
2169:
2161:
2146:
2142:
2125:
2113:
2100:
2093:
2082:
2074:
2061:
2057:
2027:
2023:
2019:
2015:
2011:
2001:
1990:
1971:
1968:. A permutation
1967:
1951:
1929:
1922:
1911:
1895:
1862:
1850:
1836:
1819:
1804:
1793:
1755:
1751:
1740:
1729:
1710:full binary tree
1697:
1682:
1671:
1664:
1644:
1640:
1605:
1601:
1593:
1582:
1560:
1556:
1545:
1535:
1505:
1503:
1502:
1497:
1489:
1487:
1486:
1468:
1467:
1458:
1455:
1450:
1428:
1426:
1425:
1420:
1418:
1417:
1401:
1399:
1398:
1393:
1368:
1366:
1365:
1360:
1352:
1350:
1349:
1340:
1339:
1330:
1327:
1322:
1297:
1295:
1294:
1289:
1277:
1275:
1274:
1259:
1257:
1256:
1243:
1238:
1226:
1225:
1216:
1208:
1195:
1189:
1178:
1177:
1175:
1174:
1164:
1159:
1150:
1148:
1137:
1132:
1131:
1109:
1099:
1089:
1082:
1064:
1062:
1061:
1056:
1030:
1026:
1022:
1020:
1019:
1014:
1008:
1006:
1005:
1000:
998:
997:
993:
979:
978:
969:
964:
963:
944:
942:
941:
936:
925:
922:
919:
918:
903:
901:
890:
867:
862:
861:
851:
848:
839:
838:
816:
814:
813:
808:
797:
794:
791:
790:
775:
774:
758:
753:
735:
734:
724:
721:
712:
711:
679:
677:
676:
671:
665:
664:
663:
654:
639:
632:
630:
613:
608:
607:
577:
566:
564:
563:
558:
556:
554:
553:
544:
535:
527:
524:
510:
504:
502:
501:
495:
484:
475:
457:
455:
454:
449:
427:
425:
424:
419:
417:
416:
415:
409:
398:
389:
379:
378:
377:
368:
359:
349:
348:
329:
305:
299:
292:
282:
280:
279:
274:
263:
260:
257:
252:
241:
238:
233:
215:
213:
186:
169:
164:
163:
162:
153:
144:
137:
135:
121:
116:
115:
92:
39:
12812:
12811:
12807:
12806:
12805:
12803:
12802:
12801:
12772:
12771:
12770:
12765:
12743:
12739:Strobogrammatic
12730:
12712:
12694:
12676:
12658:
12640:
12622:
12604:
12581:
12560:
12544:
12503:Divisor-related
12498:
12458:
12409:
12379:
12316:
12300:
12279:
12246:
12219:
12207:
12189:
12101:
12100:related numbers
12074:
12051:
12018:
12009:Perfect totient
11975:
11952:
11883:Highly abundant
11825:
11804:
11736:
11719:
11691:
11674:
11660:Stirling second
11566:
11543:
11504:
11486:
11443:
11392:
11329:
11290:Centered square
11258:
11241:
11203:
11188:
11155:
11140:
11092:
11091:defined numbers
11074:
11041:
11026:
10997:Double Mersenne
10983:
10964:
10886:
10872:
10870:natural numbers
10866:
10815:Catalan numbers
10788:
10778:
10750:
10730:
10694:
10677:
10657:
10629:Gardner, Martin
10600:Catalan numbers
10595:
10590:
10551:Adv. Appl. Math
10543:
10539:
10491:
10487:
10464:
10460:
10443:
10439:
10422:
10418:
10409:
10407:
10398:
10397:
10393:
10385:
10379:
10375:
10358:
10354:
10330:
10324:
10320:
10298:
10294:
10262:
10258:
10247:
10243:
10231:
10227:
10211:
10205:
10201:
10196:
10192:
10185:
10181:
10156:
10152:
10103:
10099:
10067:
10061:
10057:
10053:
10048:
10019:Schröder number
10009:Narayana number
9984:Delannoy number
9948:
9941:
9938:
9916:
9915:
9910:
9905:
9892:
9870:
9866:
9827:
9792:
9767:
9755:
9694:
9691:
9688:
9687:
9682:
9677:
9664:
9654:
9650:
9611:
9582:
9566:
9548:
9499:
9496:
9489:
9488:
9477:
9473:
9472:
9468:
9457:
9453:
9452:
9448:
9432:
9428:
9413:
9409:
9408:
9395:
9391:
9376:
9372:
9371:
9369:
9363:
9360:
9359:
9346:
9342:
9339:
9310:
9306:
9304:
9301:
9300:
9283:
9279:
9277:
9274:
9273:
9243:
9240:
9239:
9211:
9208:
9207:
9185:
9182:
9181:
9159:
9156:
9155:
9095:
9063:
9061:
9059:
9056:
9055:
9048:
9041:
9024:
9020:
9018:
9015:
9014:
9007:
9005:Generalizations
8974:
8971:
8970:
8939:
8936:
8935:
8904:
8901:
8900:
8866:Towers of Hanoi
8842:
8810:
8809:
8804:
8799:
8794:
8788:
8787:
8782:
8777:
8772:
8766:
8765:
8760:
8755:
8750:
8744:
8743:
8738:
8733:
8728:
8718:
8717:
8712:
8709:
8708:
8681:
8680:
8675:
8670:
8664:
8663:
8658:
8653:
8647:
8646:
8641:
8636:
8626:
8625:
8620:
8617:
8616:
8589:
8588:
8583:
8577:
8576:
8571:
8561:
8560:
8555:
8552:
8551:
8524:
8523:
8513:
8512:
8507:
8504:
8503:
8493:
8489:
8479:
8449:
8448:
8443:
8438:
8433:
8427:
8426:
8421:
8416:
8411:
8405:
8404:
8399:
8394:
8389:
8383:
8382:
8377:
8372:
8367:
8357:
8356:
8351:
8348:
8347:
8337:
8333:
8330:
8317:
8305:
8278:
8277:
8272:
8267:
8262:
8256:
8255:
8250:
8245:
8240:
8234:
8233:
8228:
8223:
8218:
8212:
8211:
8206:
8201:
8196:
8186:
8185:
8180:
8177:
8176:
8166:
8162:
8155:
8142:
8130:
8117:
8114:
8093:
8090:
8089:
8067:
8064:
8063:
8045:
8041:
8031:
8013:
8007:
8006:
8005:
7989:
7984:
7981:
7978:
7977:
7952:
7949:
7948:
7932:
7929:
7928:
7906:
7903:
7902:
7883:
7865:
7859:
7858:
7857:
7854:
7851:
7850:
7822:
7819:
7818:
7802:
7799:
7798:
7776:
7773:
7772:
7756:
7753:
7752:
7736:
7733:
7732:
7700:
7699:
7697:
7694:
7693:
7664:
7663:
7661:
7658:
7657:
7628:
7627:
7625:
7622:
7621:
7592:
7591:
7589:
7586:
7585:
7556:
7555:
7553:
7550:
7549:
7527:
7524:
7523:
7501:
7498:
7497:
7494:circular shifts
7471:
7468:
7467:
7445:
7442:
7441:
7425:
7422:
7421:
7405:
7402:
7401:
7383:
7360:
7348:
7342:
7341:
7340:
7327:
7322:
7313:
7309:
7307:
7304:
7303:
7282:
7264:
7258:
7257:
7256:
7246:
7234:
7228:
7227:
7226:
7214:
7210:
7208:
7205:
7204:
7183:
7165:
7159:
7158:
7157:
7148:
7144:
7132:
7128:
7126:
7123:
7122:
7101:
7083:
7077:
7076:
7075:
7066:
7062:
7053:
7049:
7044:
7041:
7040:
7033:
7004:
7000:
6991:
6987:
6978:
6974:
6962:
6951:
6932:
6928:
6921:
6917:
6911:
6900:
6881:
6877:
6871:
6867:
6861:
6850:
6828:
6824:
6809:
6805:
6803:
6800:
6799:
6770:
6766:
6759:
6741:
6735:
6734:
6733:
6727:
6716:
6697:
6693:
6678:
6674:
6672:
6669:
6668:
6649:
6646:
6645:
6629:
6626:
6625:
6606:
6603:
6602:
6573:
6569:
6563:
6559:
6553:
6542:
6520:
6516:
6514:
6511:
6510:
6482:
6477:
6474:
6473:
6448:
6445:
6444:
6425:
6413:
6407:
6406:
6405:
6396:
6392:
6389:
6386:
6385:
6369:
6366:
6365:
6349:
6346:
6345:
6341:
6334:
6330:
6301:
6288:
6284:
6277:
6273:
6267:
6256:
6237:
6233:
6227:
6214:
6210:
6208:
6205:
6204:
6184:
6180:
6178:
6175:
6174:
6157:
6153:
6144:
6140:
6129:
6126:
6125:
6121:
6117:
6113:
6109:
6092:
6088:
6086:
6083:
6082:
6075:
6047:
6043:
6041:
6038:
6037:
5957:
5953:
5940:
5923:
5921:
5919:
5916:
5915:
5862:
5858:
5793:
5790:
5789:
5763:
5759:
5744:
5740:
5727:
5713:
5711:
5708:
5705:
5704:
5677:
5673:
5649:
5645:
5625:
5622:
5621:
5611:
5607:
5601:
5587:
5580:
5576:
5570:
5557:
5549:
5542:
5538:
5532:
5523:
5520:
5512:
5509:
5482:
5479:
5478:
5452:
5448:
5437:
5434:
5433:
5429:
5412:
5408:
5406:
5403:
5402:
5383:
5371:
5365:
5364:
5363:
5360:
5357:
5356:
5349:
5343:
5336:
5312:
5300:
5294:
5293:
5292:
5279:
5274:
5265:
5261:
5258:
5255:
5254:
5235:
5223:
5217:
5216:
5215:
5212:
5209:
5208:
5204:
5197:
5186:
5179:
5175:
5156:
5152:
5148:
5144:
5124:
5120:
5116:
5101:
5093:
5089:
5082:
5067:
5042:
5034:
5027:
5024:
5015:
5011:
4987:
4975:
4969:
4968:
4967:
4954:
4949:
4939:
4927:
4919:
4913:
4912:
4911:
4901:
4889:
4883:
4882:
4881:
4872:
4868:
4866:
4863:
4862:
4838:
4826:
4818:
4812:
4811:
4810:
4800:
4788:
4780:
4774:
4773:
4772:
4762:
4750:
4727:
4721:
4720:
4719:
4717:
4714:
4713:
4695:
4683:
4671:
4664:
4657:
4650:
4620:
4608:
4602:
4601:
4599:
4597:
4594:
4593:
4586:
4582:
4578:
4568:
4552:
4540:
4533:
4527:
4502:
4498:
4491:
4479:
4473:
4472:
4471:
4458:
4453:
4447:
4436:
4419:
4402:
4395:
4393:
4376:
4373:
4372:
4358:
4357:
4341:
4337:
4330:
4318:
4312:
4311:
4310:
4297:
4292:
4286:
4275:
4256:
4252:
4224:
4204:
4202:
4196:
4185:
4172:
4171:
4159:
4155:
4133:
4096:
4092:
4091:
4089:
4083:
4072:
4053:
4049:
4004:
4000:
3999:
3968:
3964:
3954:
3952:
3946:
3935:
3919:
3918:
3912:
3908:
3881:
3877:
3876:
3839:
3835:
3825:
3823:
3817:
3806:
3790:
3786:
3767:
3754:
3750:
3744:
3743:
3742:
3736:
3725:
3711:
3695:
3685:
3683:
3680:
3679:
3675:binomial series
3629:
3616:
3612:
3610:
3607:
3606:
3578:
3561:
3554:
3552:
3535:
3532:
3531:
3530: or
3510:
3493:
3486:
3484:
3467:
3464:
3463:
3452:
3437:
3410:
3406:
3371:
3368:
3367:
3341:
3337:
3331:
3327:
3321:
3310:
3289:
3286:
3285:
3272:
3266:
3263:
3257:
3251:
3244:
3234:
3227:
3199:
3186:
3182:
3175:
3171:
3165:
3154:
3135:
3131:
3125:
3112:
3108:
3106:
3103:
3102:
3090:
3056:
3044:
3038:
3037:
3036:
3023:
3018:
3009:
3005:
3003:
3000:
2999:
2993:
2971:
2968:
2967:
2959:
2955:
2951:
2857:
2854:
2853:
2831:
2828:
2827:
2811:
2808:
2807:
2791:
2788:
2787:
2771:
2768:
2767:
2751:
2748:
2747:
2743:
2726:
2722:
2720:
2717:
2716:
2713:
2701:
2694:
2690:
2683:
2675:
2672:
2661:
2658:
2655:
2652:
2648:
2644:
2641:
2638:
2635:
2631:
2627:
2624:
2620:
2616:
2613:
2609:
2607:
2603:
2600:
2596:
2593:
2590:
2587:
2583:
2580:
2577:
2574:
2571:
2568:
2564:
2561:
2558:
2555:
2551:
2548:
2545:
2542:
2539:
2536:
2533:
2530:
2527:
2524:
2521:
2520:
2516:
2513:
2510:
2507:
2504:
2501:
2498:
2495:
2492:
2489:
2486:
2483:
2480:
2477:
2474:
2471:
2468:
2465:
2462:
2459:
2456:
2453:
2450:
2447:
2444:
2441:
2438:
2435:
2432:
2429:
2426:
2423:
2420:
2417:
2391:
2388:
2387:
2376:
2373:
2369:
2367:
2363:
2360:
2357:
2354:
2351:
2348:
2345:
2319:
2316:
2315:
2281:
2278:
2277:
2243:
2240:
2239:
2225:
2221:
2218:
2210:
2192:
2188:
2184:
2181:
2173:
2163:
2160:
2152:
2144:
2141:
2133:
2119:
2112:
2104:
2095:
2088:
2083:that avoid the
2076:
2073:
2065:
2059:
2029:
2025:
2021:
2017:
2013:
2003:
1992:
1977:
1969:
1961:
1950:
1942:
1924:
1921:
1913:
1909:
1890:
1857:
1842:
1835:
1827:
1818:
1810:
1809:. For example,
1799:
1792:
1784:
1778:
1756:internal nodes:
1753:
1746:
1739:
1731:
1713:
1705:
1704:
1692:
1685:binary operator
1680:
1666:
1663:
1655:
1652:
1651:
1642:
1639:
1631:
1623:
1622:
1618:
1617:
1613:
1612:
1603:
1599:
1588:
1581:
1573:
1561:interpreted as
1558:
1554:
1543:
1537:
1533:
1527:
1512:
1473:
1469:
1463:
1459:
1457:
1451:
1440:
1434:
1431:
1430:
1413:
1409:
1407:
1404:
1403:
1378:
1375:
1374:
1345:
1341:
1335:
1331:
1329:
1323:
1312:
1306:
1303:
1302:
1270:
1266:
1258:
1249:
1245:
1239:
1231:
1221:
1217:
1207:
1179:
1176:
1170:
1166:
1160:
1155:
1141:
1136:
1127:
1123:
1121:
1118:
1117:
1107:
1101:
1097:
1091:
1084:
1081:
1073:
1047:
1044:
1043:
1028:
1024:
999:
989:
985:
981:
980:
974:
970:
968:
959:
955:
953:
950:
949:
921:
908:
904:
891:
868:
866:
857:
853:
847:
834:
830:
828:
825:
824:
793:
780:
776:
764:
760:
754:
743:
730:
726:
720:
707:
703:
701:
698:
697:
659:
641:
635:
634:
633:
617:
612:
603:
599:
597:
594:
593:
576:
568:
549:
537:
531:
530:
528:
514:
508:
497:
485:
477:
471:
470:
468:
466:
463:
462:
433:
430:
429:
411:
399:
391:
385:
384:
383:
373:
361:
355:
354:
353:
344:
340:
338:
335:
334:
328:
320:
317:
301:
297:
287:
259:
242:
240:
234:
223:
187:
170:
168:
158:
146:
140:
139:
138:
125:
120:
111:
107:
105:
102:
101:
90:
68:natural numbers
60:Catalan numbers
37:
33:
24:
17:
12:
11:
5:
12810:
12800:
12799:
12794:
12789:
12784:
12767:
12766:
12764:
12763:
12752:
12749:
12748:
12745:
12744:
12742:
12741:
12735:
12732:
12731:
12718:
12717:
12714:
12713:
12711:
12710:
12705:
12699:
12696:
12695:
12682:
12681:
12678:
12677:
12675:
12674:
12672:Sorting number
12669:
12667:Pancake number
12663:
12660:
12659:
12646:
12645:
12642:
12641:
12639:
12638:
12633:
12627:
12624:
12623:
12610:
12609:
12606:
12605:
12603:
12602:
12597:
12592:
12586:
12583:
12582:
12579:Binary numbers
12570:
12569:
12566:
12565:
12562:
12561:
12559:
12558:
12552:
12550:
12546:
12545:
12543:
12542:
12537:
12532:
12527:
12522:
12517:
12512:
12506:
12504:
12500:
12499:
12497:
12496:
12491:
12486:
12481:
12476:
12470:
12468:
12460:
12459:
12457:
12456:
12451:
12446:
12441:
12436:
12431:
12426:
12420:
12418:
12411:
12410:
12408:
12407:
12406:
12405:
12394:
12392:
12389:P-adic numbers
12385:
12384:
12381:
12380:
12378:
12377:
12376:
12375:
12365:
12360:
12355:
12350:
12345:
12340:
12335:
12330:
12324:
12322:
12318:
12317:
12315:
12314:
12308:
12306:
12305:Coding-related
12302:
12301:
12299:
12298:
12293:
12287:
12285:
12281:
12280:
12278:
12277:
12272:
12267:
12262:
12256:
12254:
12245:
12244:
12243:
12242:
12240:Multiplicative
12237:
12226:
12224:
12209:
12208:
12204:Numeral system
12195:
12194:
12191:
12190:
12188:
12187:
12182:
12177:
12172:
12167:
12162:
12157:
12152:
12147:
12142:
12137:
12132:
12127:
12122:
12117:
12112:
12106:
12103:
12102:
12084:
12083:
12080:
12079:
12076:
12075:
12073:
12072:
12067:
12061:
12059:
12053:
12052:
12050:
12049:
12044:
12039:
12034:
12028:
12026:
12020:
12019:
12017:
12016:
12011:
12006:
12001:
11996:
11994:Highly totient
11991:
11985:
11983:
11977:
11976:
11974:
11973:
11968:
11962:
11960:
11954:
11953:
11951:
11950:
11945:
11940:
11935:
11930:
11925:
11920:
11915:
11910:
11905:
11900:
11895:
11890:
11885:
11880:
11875:
11870:
11865:
11860:
11855:
11850:
11848:Almost perfect
11845:
11839:
11837:
11827:
11826:
11810:
11809:
11806:
11805:
11803:
11802:
11797:
11792:
11787:
11782:
11777:
11772:
11767:
11762:
11757:
11752:
11747:
11741:
11738:
11737:
11725:
11724:
11721:
11720:
11718:
11717:
11712:
11707:
11702:
11696:
11693:
11692:
11680:
11679:
11676:
11675:
11673:
11672:
11667:
11662:
11657:
11655:Stirling first
11652:
11647:
11642:
11637:
11632:
11627:
11622:
11617:
11612:
11607:
11602:
11597:
11592:
11587:
11582:
11577:
11571:
11568:
11567:
11557:
11556:
11553:
11552:
11549:
11548:
11545:
11544:
11542:
11541:
11536:
11531:
11525:
11523:
11516:
11510:
11509:
11506:
11505:
11503:
11502:
11496:
11494:
11488:
11487:
11485:
11484:
11479:
11474:
11469:
11464:
11459:
11453:
11451:
11445:
11444:
11442:
11441:
11436:
11431:
11426:
11421:
11415:
11413:
11404:
11398:
11397:
11394:
11393:
11391:
11390:
11385:
11380:
11375:
11370:
11365:
11360:
11355:
11350:
11345:
11339:
11337:
11331:
11330:
11328:
11327:
11322:
11317:
11312:
11307:
11302:
11297:
11292:
11287:
11281:
11279:
11270:
11260:
11259:
11247:
11246:
11243:
11242:
11240:
11239:
11234:
11229:
11224:
11219:
11214:
11208:
11205:
11204:
11194:
11193:
11190:
11189:
11187:
11186:
11181:
11176:
11171:
11166:
11160:
11157:
11156:
11146:
11145:
11142:
11141:
11139:
11138:
11133:
11128:
11123:
11118:
11113:
11108:
11103:
11097:
11094:
11093:
11080:
11079:
11076:
11075:
11073:
11072:
11067:
11062:
11057:
11052:
11046:
11043:
11042:
11032:
11031:
11028:
11027:
11025:
11024:
11019:
11014:
11009:
11004:
10999:
10994:
10988:
10985:
10984:
10970:
10969:
10966:
10965:
10963:
10962:
10957:
10952:
10947:
10942:
10937:
10932:
10927:
10922:
10917:
10912:
10907:
10902:
10897:
10891:
10888:
10887:
10874:
10873:
10865:
10864:
10857:
10850:
10842:
10836:
10835:
10834:at Wikiversity
10823:
10818:
10811:
10792:
10777:
10776:External links
10774:
10773:
10772:
10754:
10741:
10728:
10709:
10687:
10680:
10675:
10660:
10655:
10625:
10611:
10594:
10591:
10589:
10588:
10537:
10485:
10458:
10437:
10416:
10391:
10373:
10352:
10318:
10308:(2): 305–313,
10292:
10256:
10241:
10225:
10199:
10190:
10179:
10150:
10097:
10054:
10052:
10049:
10047:
10046:
10041:
10036:
10034:Tamari lattice
10031:
10026:
10021:
10016:
10011:
10006:
10004:Motzkin number
10001:
9996:
9991:
9986:
9981:
9976:
9971:
9966:
9961:
9955:
9954:
9953:
9937:
9934:
9933:
9932:
9919:
9909:
9906:
9904:
9899:
9895:
9891:
9888:
9885:
9882:
9879:
9876:
9873:
9869:
9861:
9858:
9855:
9852:
9849:
9846:
9843:
9840:
9837:
9834:
9830:
9826:
9823:
9820:
9817:
9814:
9811:
9808:
9805:
9802:
9799:
9795:
9791:
9788:
9785:
9782:
9779:
9776:
9773:
9770:
9765:
9762:
9758:
9754:
9751:
9748:
9745:
9742:
9739:
9736:
9733:
9730:
9727:
9724:
9721:
9718:
9715:
9712:
9709:
9706:
9703:
9700:
9697:
9690:
9689:
9681:
9678:
9676:
9671:
9667:
9663:
9660:
9657:
9653:
9645:
9642:
9639:
9636:
9633:
9630:
9627:
9624:
9621:
9618:
9614:
9610:
9607:
9604:
9601:
9598:
9595:
9592:
9589:
9585:
9581:
9578:
9575:
9572:
9569:
9564:
9561:
9558:
9555:
9551:
9547:
9544:
9541:
9538:
9535:
9532:
9529:
9526:
9523:
9520:
9517:
9514:
9511:
9508:
9505:
9502:
9495:
9494:
9492:
9487:
9480:
9476:
9471:
9467:
9460:
9456:
9451:
9443:
9440:
9435:
9431:
9427:
9424:
9421:
9416:
9412:
9406:
9403:
9398:
9394:
9390:
9387:
9384:
9379:
9375:
9368:
9338:
9335:
9313:
9309:
9286:
9282:
9247:
9227:
9224:
9221:
9218:
9215:
9195:
9192:
9189:
9169:
9166:
9163:
9125:
9122:
9119:
9116:
9113:
9110:
9107:
9104:
9101:
9098:
9093:
9090:
9087:
9084:
9081:
9078:
9075:
9072:
9069:
9066:
9027:
9023:
9006:
9003:
8990:
8987:
8984:
8981:
8978:
8958:
8955:
8952:
8949:
8946:
8943:
8923:
8920:
8917:
8914:
8911:
8908:
8858:Leonhard Euler
8841:
8838:
8834:
8833:
8822:
8819:
8814:
8808:
8805:
8803:
8800:
8798:
8795:
8793:
8790:
8789:
8786:
8783:
8781:
8778:
8776:
8773:
8771:
8768:
8767:
8764:
8761:
8759:
8756:
8754:
8751:
8749:
8746:
8745:
8742:
8739:
8737:
8734:
8732:
8729:
8727:
8724:
8723:
8721:
8716:
8705:
8704:
8693:
8690:
8685:
8679:
8676:
8674:
8671:
8669:
8666:
8665:
8662:
8659:
8657:
8654:
8652:
8649:
8648:
8645:
8642:
8640:
8637:
8635:
8632:
8631:
8629:
8624:
8613:
8612:
8601:
8598:
8593:
8587:
8584:
8582:
8579:
8578:
8575:
8572:
8570:
8567:
8566:
8564:
8559:
8548:
8547:
8536:
8533:
8528:
8522:
8519:
8518:
8516:
8511:
8473:
8472:
8461:
8458:
8453:
8447:
8444:
8442:
8439:
8437:
8434:
8432:
8429:
8428:
8425:
8422:
8420:
8417:
8415:
8412:
8410:
8407:
8406:
8403:
8400:
8398:
8395:
8393:
8390:
8388:
8385:
8384:
8381:
8378:
8376:
8373:
8371:
8368:
8366:
8363:
8362:
8360:
8355:
8321:
8302:
8301:
8290:
8287:
8282:
8276:
8273:
8271:
8268:
8266:
8263:
8261:
8258:
8257:
8254:
8251:
8249:
8246:
8244:
8241:
8239:
8236:
8235:
8232:
8229:
8227:
8224:
8222:
8219:
8217:
8214:
8213:
8210:
8207:
8205:
8202:
8200:
8197:
8195:
8192:
8191:
8189:
8184:
8146:
8113:
8110:
8097:
8077:
8074:
8071:
8048:
8044:
8040:
8034:
8029:
8025:
8022:
8019:
8016:
8010:
8001:
7998:
7995:
7992:
7988:
7965:
7962:
7959:
7956:
7936:
7916:
7913:
7910:
7886:
7881:
7877:
7874:
7871:
7868:
7862:
7838:
7835:
7832:
7829:
7826:
7806:
7786:
7783:
7780:
7760:
7740:
7715:
7712:
7709:
7706:
7703:
7679:
7676:
7673:
7670:
7667:
7643:
7640:
7637:
7634:
7631:
7607:
7604:
7601:
7598:
7595:
7571:
7568:
7565:
7562:
7559:
7537:
7534:
7531:
7511:
7508:
7505:
7481:
7478:
7475:
7455:
7452:
7449:
7429:
7409:
7382:
7379:
7378:
7377:
7363:
7358:
7354:
7351:
7345:
7336:
7333:
7330:
7326:
7321:
7316:
7312:
7300:
7299:
7285:
7280:
7276:
7273:
7270:
7267:
7261:
7255:
7249:
7244:
7240:
7237:
7231:
7225:
7222:
7217:
7213:
7201:
7200:
7186:
7181:
7177:
7174:
7171:
7168:
7162:
7156:
7151:
7147:
7143:
7140:
7135:
7131:
7119:
7118:
7104:
7099:
7095:
7092:
7089:
7086:
7080:
7074:
7069:
7065:
7061:
7056:
7052:
7048:
7030:
7029:
7018:
7013:
7010:
7007:
7003:
6999:
6994:
6990:
6986:
6981:
6977:
6973:
6970:
6965:
6960:
6957:
6954:
6950:
6946:
6941:
6938:
6935:
6931:
6924:
6920:
6914:
6909:
6906:
6903:
6899:
6895:
6890:
6887:
6884:
6880:
6874:
6870:
6864:
6859:
6856:
6853:
6849:
6845:
6842:
6837:
6834:
6831:
6827:
6823:
6818:
6815:
6812:
6808:
6793:
6792:
6779:
6776:
6773:
6769:
6762:
6757:
6753:
6750:
6747:
6744:
6738:
6730:
6725:
6722:
6719:
6715:
6711:
6706:
6703:
6700:
6696:
6692:
6687:
6684:
6681:
6677:
6653:
6633:
6613:
6610:
6599:
6598:
6587:
6582:
6579:
6576:
6572:
6566:
6562:
6556:
6551:
6548:
6545:
6541:
6537:
6534:
6529:
6526:
6523:
6519:
6495:
6492:
6488:
6485:
6481:
6461:
6458:
6455:
6452:
6428:
6423:
6419:
6416:
6410:
6404:
6399:
6395:
6373:
6353:
6327:
6326:
6314:
6311:
6308:
6297:
6294:
6291:
6287:
6280:
6276:
6270:
6265:
6262:
6259:
6255:
6251:
6246:
6243:
6240:
6236:
6225:
6222:
6217:
6213:
6187:
6183:
6160:
6156:
6152:
6147:
6143:
6139:
6136:
6133:
6095:
6091:
6074:
6071:
6058:
6055:
6050:
6046:
6034:
6033:
6022:
6019:
6016:
6013:
6010:
6007:
6004:
6001:
5998:
5995:
5992:
5989:
5986:
5983:
5980:
5977:
5974:
5971:
5968:
5965:
5960:
5956:
5952:
5946:
5943:
5938:
5935:
5932:
5929:
5926:
5909:
5908:
5897:
5894:
5891:
5888:
5885:
5882:
5879:
5876:
5873:
5870:
5865:
5861:
5857:
5854:
5851:
5848:
5845:
5842:
5839:
5836:
5833:
5830:
5827:
5824:
5821:
5818:
5815:
5812:
5809:
5806:
5803:
5800:
5797:
5771:
5766:
5762:
5758:
5753:
5750:
5747:
5743:
5736:
5733:
5730:
5725:
5722:
5719:
5716:
5701:
5700:
5686:
5683:
5680:
5676:
5672:
5669:
5666:
5663:
5660:
5657:
5652:
5648:
5644:
5641:
5638:
5635:
5632:
5629:
5596:
5566:
5527:
5516:
5508:
5505:
5492:
5489:
5486:
5466:
5463:
5460:
5455:
5451:
5447:
5444:
5441:
5415:
5411:
5386:
5381:
5377:
5374:
5368:
5347:
5321:
5315:
5310:
5306:
5303:
5297:
5288:
5285:
5282:
5278:
5273:
5268:
5264:
5238:
5233:
5229:
5226:
5220:
5171:the diagonal.
5098:
5097:
5086:
5075:
5038:
5023:
5020:
5008:
5007:
4996:
4990:
4985:
4981:
4978:
4972:
4963:
4960:
4957:
4953:
4948:
4942:
4936:
4933:
4930:
4925:
4922:
4916:
4910:
4904:
4899:
4895:
4892:
4886:
4880:
4875:
4871:
4856:
4855:
4841:
4835:
4832:
4829:
4824:
4821:
4815:
4809:
4803:
4797:
4794:
4791:
4786:
4783:
4777:
4771:
4765:
4759:
4756:
4753:
4748:
4745:
4742:
4739:
4736:
4733:
4730:
4724:
4623:
4618:
4614:
4611:
4605:
4526:
4523:
4511:
4505:
4501:
4494:
4489:
4485:
4482:
4476:
4467:
4464:
4461:
4457:
4450:
4445:
4442:
4439:
4435:
4431:
4425:
4422:
4415:
4412:
4409:
4406:
4401:
4398:
4392:
4389:
4386:
4383:
4380:
4356:
4350:
4347:
4344:
4340:
4333:
4328:
4324:
4321:
4315:
4306:
4303:
4300:
4296:
4289:
4284:
4281:
4278:
4274:
4270:
4265:
4262:
4259:
4255:
4248:
4245:
4242:
4239:
4236:
4233:
4230:
4227:
4222:
4219:
4216:
4213:
4210:
4207:
4199:
4194:
4191:
4188:
4184:
4180:
4177:
4175:
4173:
4168:
4165:
4162:
4158:
4151:
4148:
4145:
4142:
4139:
4136:
4131:
4128:
4125:
4122:
4119:
4116:
4113:
4110:
4105:
4102:
4099:
4095:
4086:
4081:
4078:
4075:
4071:
4067:
4062:
4059:
4056:
4052:
4048:
4045:
4042:
4039:
4033:
4030:
4027:
4024:
4021:
4018:
4013:
4010:
4007:
4003:
3997:
3994:
3991:
3988:
3985:
3982:
3979:
3976:
3971:
3967:
3963:
3960:
3957:
3949:
3944:
3941:
3938:
3934:
3930:
3927:
3924:
3922:
3920:
3915:
3911:
3907:
3904:
3901:
3898:
3892:
3889:
3884:
3880:
3874:
3871:
3868:
3865:
3862:
3859:
3856:
3853:
3848:
3845:
3842:
3838:
3834:
3831:
3828:
3820:
3815:
3812:
3809:
3805:
3801:
3798:
3793:
3789:
3785:
3782:
3779:
3776:
3770:
3765:
3761:
3757:
3753:
3747:
3739:
3734:
3731:
3728:
3724:
3720:
3717:
3714:
3712:
3708:
3705:
3702:
3699:
3694:
3691:
3688:
3687:
3671:
3670:
3658:
3655:
3652:
3649:
3646:
3643:
3638:
3635:
3632:
3628:
3624:
3619:
3615:
3600:
3599:
3584:
3581:
3574:
3571:
3568:
3565:
3560:
3557:
3551:
3548:
3545:
3542:
3539:
3516:
3513:
3506:
3503:
3500:
3497:
3492:
3489:
3483:
3480:
3477:
3474:
3471:
3455:and using the
3430:
3429:
3418:
3413:
3409:
3405:
3402:
3399:
3396:
3393:
3390:
3387:
3384:
3381:
3378:
3375:
3361:
3360:
3349:
3344:
3340:
3334:
3330:
3324:
3319:
3316:
3313:
3309:
3305:
3302:
3299:
3296:
3293:
3270:
3261:
3254:
3253:
3249:
3242:
3224:
3223:
3212:
3209:
3206:
3195:
3192:
3189:
3185:
3178:
3174:
3168:
3163:
3160:
3157:
3153:
3149:
3144:
3141:
3138:
3134:
3123:
3120:
3115:
3111:
3089:
3086:
3074:
3073:
3059:
3054:
3050:
3047:
3041:
3032:
3029:
3026:
3022:
3017:
3012:
3008:
2992:
2989:
2988:
2987:
2975:
2939:
2936:
2933:
2930:
2927:
2924:
2921:
2918:
2915:
2912:
2909:
2906:
2903:
2900:
2897:
2894:
2891:
2888:
2885:
2882:
2879:
2876:
2873:
2870:
2867:
2864:
2861:
2841:
2838:
2835:
2815:
2795:
2775:
2755:
2729:
2725:
2711:
2710:
2709:
2679:
2669:
2668:
2665:
2415:
2404:
2401:
2398:
2395:
2384:
2383:
2380:
2343:
2332:
2329:
2326:
2323:
2312:
2311:
2308:
2305:
2294:
2291:
2288:
2285:
2274:
2273:
2270:
2267:
2256:
2253:
2250:
2247:
2230:
2229:
2224:upstrokes and
2214:
2200:
2199:
2177:
2171:
2156:
2137:
2108:
2102:
2069:
2063:
1974:stack-sortable
1946:
1932:
1931:
1917:
1887:convex polygon
1854:
1853:
1831:
1825:
1814:
1805:vertices. See
1788:
1776:
1758:
1757:
1735:
1702:
1701:
1700:
1699:
1691:problem). For
1659:
1649:
1648:
1647:
1646:
1635:
1620:
1619:
1615:
1614:
1610:
1609:
1608:
1607:
1598:consisting of
1577:
1541:
1531:
1511:
1508:
1495:
1492:
1485:
1482:
1479:
1476:
1472:
1466:
1462:
1454:
1449:
1446:
1443:
1439:
1416:
1412:
1391:
1388:
1385:
1382:
1358:
1355:
1348:
1344:
1338:
1334:
1326:
1321:
1318:
1315:
1311:
1299:
1298:
1287:
1284:
1281:
1273:
1269:
1265:
1262:
1255:
1252:
1248:
1242:
1237:
1234:
1230:
1224:
1220:
1214:
1211:
1206:
1202:
1199:
1192:
1188:
1185:
1182:
1173:
1169:
1163:
1158:
1154:
1147:
1144:
1140:
1135:
1130:
1126:
1105:
1095:
1077:
1054:
1051:
1012:
1003:
996:
992:
988:
984:
977:
973:
967:
962:
958:
946:
945:
934:
931:
928:
917:
914:
911:
907:
900:
897:
894:
889:
886:
883:
880:
877:
874:
871:
865:
860:
856:
845:
842:
837:
833:
818:
817:
806:
803:
800:
789:
786:
783:
779:
773:
770:
767:
763:
757:
752:
749:
746:
742:
738:
733:
729:
718:
715:
710:
706:
681:
680:
669:
662:
657:
653:
650:
647:
644:
638:
629:
626:
623:
620:
616:
611:
606:
602:
572:
552:
547:
543:
540:
534:
523:
520:
517:
513:
507:
500:
494:
491:
488:
483:
480:
474:
459:
458:
447:
443:
440:
437:
414:
408:
405:
402:
397:
394:
388:
382:
376:
371:
367:
364:
358:
352:
347:
343:
324:
316:
313:
312:
311:
284:
283:
272:
269:
266:
255:
251:
248:
245:
237:
232:
229:
226:
222:
218:
212:
209:
205:
202:
199:
196:
193:
190:
185:
182:
179:
176:
173:
167:
161:
156:
152:
149:
143:
134:
131:
128:
124:
119:
114:
110:
35:
15:
9:
6:
4:
3:
2:
12809:
12798:
12795:
12793:
12790:
12788:
12785:
12783:
12780:
12779:
12777:
12762:
12758:
12754:
12753:
12750:
12740:
12737:
12736:
12733:
12728:
12723:
12719:
12709:
12706:
12704:
12701:
12700:
12697:
12692:
12687:
12683:
12673:
12670:
12668:
12665:
12664:
12661:
12656:
12651:
12647:
12637:
12634:
12632:
12629:
12628:
12625:
12621:
12615:
12611:
12601:
12598:
12596:
12593:
12591:
12588:
12587:
12584:
12580:
12575:
12571:
12557:
12554:
12553:
12551:
12547:
12541:
12538:
12536:
12533:
12531:
12530:Polydivisible
12528:
12526:
12523:
12521:
12518:
12516:
12513:
12511:
12508:
12507:
12505:
12501:
12495:
12492:
12490:
12487:
12485:
12482:
12480:
12477:
12475:
12472:
12471:
12469:
12466:
12461:
12455:
12452:
12450:
12447:
12445:
12442:
12440:
12437:
12435:
12432:
12430:
12427:
12425:
12422:
12421:
12419:
12416:
12412:
12404:
12401:
12400:
12399:
12396:
12395:
12393:
12390:
12386:
12374:
12371:
12370:
12369:
12366:
12364:
12361:
12359:
12356:
12354:
12351:
12349:
12346:
12344:
12341:
12339:
12336:
12334:
12331:
12329:
12326:
12325:
12323:
12319:
12313:
12310:
12309:
12307:
12303:
12297:
12294:
12292:
12289:
12288:
12286:
12284:Digit product
12282:
12276:
12273:
12271:
12268:
12266:
12263:
12261:
12258:
12257:
12255:
12253:
12249:
12241:
12238:
12236:
12233:
12232:
12231:
12228:
12227:
12225:
12223:
12218:
12214:
12210:
12205:
12200:
12196:
12186:
12183:
12181:
12178:
12176:
12173:
12171:
12168:
12166:
12163:
12161:
12158:
12156:
12153:
12151:
12148:
12146:
12143:
12141:
12138:
12136:
12133:
12131:
12128:
12126:
12123:
12121:
12120:Erdős–Nicolas
12118:
12116:
12113:
12111:
12108:
12107:
12104:
12099:
12095:
12089:
12085:
12071:
12068:
12066:
12063:
12062:
12060:
12058:
12054:
12048:
12045:
12043:
12040:
12038:
12035:
12033:
12030:
12029:
12027:
12025:
12021:
12015:
12012:
12010:
12007:
12005:
12002:
12000:
11997:
11995:
11992:
11990:
11987:
11986:
11984:
11982:
11978:
11972:
11969:
11967:
11964:
11963:
11961:
11959:
11955:
11949:
11946:
11944:
11941:
11939:
11938:Superabundant
11936:
11934:
11931:
11929:
11926:
11924:
11921:
11919:
11916:
11914:
11911:
11909:
11906:
11904:
11901:
11899:
11896:
11894:
11891:
11889:
11886:
11884:
11881:
11879:
11876:
11874:
11871:
11869:
11866:
11864:
11861:
11859:
11856:
11854:
11851:
11849:
11846:
11844:
11841:
11840:
11838:
11836:
11832:
11828:
11824:
11820:
11815:
11811:
11801:
11798:
11796:
11793:
11791:
11788:
11786:
11783:
11781:
11778:
11776:
11773:
11771:
11768:
11766:
11763:
11761:
11758:
11756:
11753:
11751:
11748:
11746:
11743:
11742:
11739:
11735:
11730:
11726:
11716:
11713:
11711:
11708:
11706:
11703:
11701:
11698:
11697:
11694:
11690:
11685:
11681:
11671:
11668:
11666:
11663:
11661:
11658:
11656:
11653:
11651:
11648:
11646:
11643:
11641:
11638:
11636:
11633:
11631:
11628:
11626:
11623:
11621:
11618:
11616:
11613:
11611:
11608:
11606:
11603:
11601:
11598:
11596:
11593:
11591:
11588:
11586:
11583:
11581:
11578:
11576:
11573:
11572:
11569:
11562:
11558:
11540:
11537:
11535:
11532:
11530:
11527:
11526:
11524:
11520:
11517:
11515:
11514:4-dimensional
11511:
11501:
11498:
11497:
11495:
11493:
11489:
11483:
11480:
11478:
11475:
11473:
11470:
11468:
11465:
11463:
11460:
11458:
11455:
11454:
11452:
11450:
11446:
11440:
11437:
11435:
11432:
11430:
11427:
11425:
11424:Centered cube
11422:
11420:
11417:
11416:
11414:
11412:
11408:
11405:
11403:
11402:3-dimensional
11399:
11389:
11386:
11384:
11381:
11379:
11376:
11374:
11371:
11369:
11366:
11364:
11361:
11359:
11356:
11354:
11351:
11349:
11346:
11344:
11341:
11340:
11338:
11336:
11332:
11326:
11323:
11321:
11318:
11316:
11313:
11311:
11308:
11306:
11303:
11301:
11298:
11296:
11293:
11291:
11288:
11286:
11283:
11282:
11280:
11278:
11274:
11271:
11269:
11268:2-dimensional
11265:
11261:
11257:
11252:
11248:
11238:
11235:
11233:
11230:
11228:
11225:
11223:
11220:
11218:
11215:
11213:
11212:Nonhypotenuse
11210:
11209:
11206:
11199:
11195:
11185:
11182:
11180:
11177:
11175:
11172:
11170:
11167:
11165:
11162:
11161:
11158:
11151:
11147:
11137:
11134:
11132:
11129:
11127:
11124:
11122:
11119:
11117:
11114:
11112:
11109:
11107:
11104:
11102:
11099:
11098:
11095:
11090:
11085:
11081:
11071:
11068:
11066:
11063:
11061:
11058:
11056:
11053:
11051:
11048:
11047:
11044:
11037:
11033:
11023:
11020:
11018:
11015:
11013:
11010:
11008:
11005:
11003:
11000:
10998:
10995:
10993:
10990:
10989:
10986:
10981:
10975:
10971:
10961:
10958:
10956:
10953:
10951:
10950:Perfect power
10948:
10946:
10943:
10941:
10940:Seventh power
10938:
10936:
10933:
10931:
10928:
10926:
10923:
10921:
10918:
10916:
10913:
10911:
10908:
10906:
10903:
10901:
10898:
10896:
10893:
10892:
10889:
10884:
10879:
10875:
10871:
10863:
10858:
10856:
10851:
10849:
10844:
10843:
10840:
10833:
10828:
10824:
10822:
10819:
10816:
10812:
10807:
10806:
10801:
10798:
10793:
10787:
10786:
10780:
10779:
10769:
10764:
10760:
10755:
10749:
10748:
10742:
10739:
10735:
10731:
10725:
10721:
10717:
10716:
10710:
10706:
10702:
10701:
10693:
10688:
10685:
10681:
10678:
10672:
10668:
10667:
10661:
10658:
10656:0-7167-1924-X
10652:
10648:
10644:
10640:
10636:
10635:
10630:
10626:
10623:
10619:
10615:
10612:
10609:
10605:
10601:
10597:
10596:
10584:
10580:
10575:
10570:
10565:
10560:
10556:
10552:
10548:
10541:
10534:
10530:
10526:
10522:
10518:
10514:
10509:
10504:
10500:
10496:
10489:
10483:
10479:
10475:
10471:
10467:
10462:
10453:
10448:
10441:
10432:
10427:
10420:
10406:on 2020-01-31
10405:
10401:
10395:
10384:
10377:
10368:
10363:
10356:
10348:
10344:
10340:
10336:
10329:
10322:
10315:
10311:
10307:
10303:
10296:
10288:
10283:
10279:
10275:
10271:
10267:
10260:
10254:
10251:
10245:
10238:
10235:
10229:
10222:(4): 589–604.
10221:
10217:
10210:
10203:
10194:
10188:
10183:
10174:
10169:
10165:
10161:
10154:
10148:, Example 3.1
10146:
10142:
10138:
10134:
10130:
10126:
10121:
10116:
10112:
10108:
10101:
10093:
10089:
10085:
10081:
10077:
10073:
10066:
10059:
10055:
10045:
10042:
10040:
10037:
10035:
10032:
10030:
10027:
10025:
10022:
10020:
10017:
10015:
10012:
10010:
10007:
10005:
10002:
10000:
9997:
9995:
9992:
9990:
9987:
9985:
9982:
9980:
9977:
9975:
9972:
9970:
9967:
9965:
9962:
9960:
9959:Associahedron
9957:
9956:
9951:
9945:
9940:
9907:
9902:
9897:
9893:
9886:
9883:
9880:
9874:
9871:
9867:
9856:
9853:
9850:
9844:
9838:
9835:
9832:
9828:
9821:
9818:
9815:
9809:
9806:
9797:
9793:
9786:
9783:
9780:
9774:
9771:
9760:
9756:
9749:
9746:
9743:
9737:
9734:
9728:
9722:
9719:
9716:
9707:
9704:
9701:
9695:
9679:
9674:
9669:
9665:
9661:
9658:
9655:
9651:
9640:
9637:
9634:
9628:
9622:
9619:
9616:
9612:
9608:
9605:
9602:
9593:
9590:
9587:
9583:
9579:
9576:
9573:
9567:
9559:
9556:
9553:
9549:
9545:
9542:
9539:
9533:
9527:
9524:
9521:
9512:
9509:
9506:
9500:
9490:
9485:
9478:
9474:
9469:
9465:
9458:
9454:
9449:
9441:
9438:
9433:
9429:
9425:
9422:
9419:
9414:
9410:
9404:
9401:
9396:
9392:
9388:
9385:
9382:
9377:
9373:
9366:
9358:
9357:
9356:
9353:
9349:
9334:
9332:
9327:
9311:
9307:
9284:
9280:
9271:
9267:
9266:Coxeter group
9263:
9259:
9245:
9225:
9222:
9219:
9216:
9213:
9193:
9190:
9187:
9167:
9164:
9161:
9152:
9150:
9146:
9142:
9123:
9120:
9117:
9114:
9111:
9105:
9102:
9099:
9091:
9085:
9082:
9076:
9070:
9067:
9052:
9044:
9025:
9021:
9012:
9002:
8985:
8979:
8976:
8953:
8950:
8944:
8941:
8918:
8915:
8909:
8906:
8897:
8895:
8893:
8889:
8885:
8880:
8878:
8873:
8871:
8867:
8863:
8859:
8851:
8846:
8837:
8820:
8817:
8812:
8806:
8801:
8796:
8791:
8784:
8779:
8774:
8769:
8762:
8757:
8752:
8747:
8740:
8735:
8730:
8725:
8719:
8707:
8706:
8691:
8688:
8683:
8677:
8672:
8667:
8660:
8655:
8650:
8643:
8638:
8633:
8627:
8615:
8614:
8599:
8596:
8591:
8585:
8580:
8573:
8568:
8562:
8550:
8549:
8534:
8531:
8526:
8520:
8514:
8502:
8501:
8500:
8496:
8486:
8482:
8476:
8459:
8456:
8451:
8445:
8440:
8435:
8430:
8423:
8418:
8413:
8408:
8401:
8396:
8391:
8386:
8379:
8374:
8369:
8364:
8358:
8346:
8345:
8344:
8340:
8328:
8324:
8320:
8313:
8309:
8288:
8285:
8280:
8274:
8269:
8264:
8259:
8252:
8247:
8242:
8237:
8230:
8225:
8220:
8215:
8208:
8203:
8198:
8193:
8187:
8175:
8174:
8173:
8169:
8160:
8153:
8149:
8145:
8138:
8134:
8128:
8127:Hankel matrix
8124:
8120:
8112:Hankel matrix
8109:
8095:
8075:
8072:
8069:
8046:
8042:
8038:
8027:
8023:
8020:
8017:
8014:
7999:
7996:
7993:
7990:
7986:
7963:
7960:
7957:
7954:
7934:
7914:
7911:
7908:
7879:
7875:
7872:
7869:
7866:
7836:
7833:
7830:
7827:
7824:
7804:
7784:
7781:
7778:
7758:
7738:
7729:
7535:
7532:
7529:
7509:
7506:
7503:
7495:
7479:
7476:
7473:
7453:
7450:
7447:
7427:
7407:
7399:
7394:
7392:
7388:
7356:
7352:
7349:
7334:
7331:
7328:
7324:
7319:
7314:
7310:
7302:
7301:
7278:
7274:
7271:
7268:
7265:
7253:
7242:
7238:
7235:
7223:
7220:
7215:
7211:
7203:
7202:
7179:
7175:
7172:
7169:
7166:
7154:
7149:
7145:
7141:
7138:
7133:
7129:
7121:
7120:
7097:
7093:
7090:
7087:
7084:
7072:
7067:
7063:
7059:
7054:
7050:
7046:
7039:
7038:
7037:
7016:
7011:
7008:
7005:
7001:
6992:
6988:
6984:
6979:
6975:
6971:
6963:
6958:
6955:
6952:
6948:
6944:
6939:
6936:
6933:
6929:
6922:
6918:
6912:
6907:
6904:
6901:
6897:
6893:
6888:
6885:
6882:
6878:
6872:
6868:
6862:
6857:
6854:
6851:
6847:
6843:
6840:
6835:
6832:
6829:
6825:
6821:
6816:
6813:
6810:
6806:
6798:
6797:
6796:
6777:
6774:
6771:
6767:
6755:
6751:
6748:
6745:
6742:
6728:
6723:
6720:
6717:
6713:
6709:
6704:
6701:
6698:
6694:
6690:
6685:
6682:
6679:
6675:
6667:
6666:
6665:
6608:
6585:
6580:
6577:
6574:
6570:
6564:
6560:
6554:
6549:
6546:
6543:
6539:
6535:
6532:
6527:
6524:
6521:
6517:
6509:
6508:
6507:
6493:
6486:
6483:
6459:
6453:
6421:
6417:
6414:
6402:
6397:
6393:
6338:
6312:
6309:
6306:
6295:
6292:
6289:
6285:
6278:
6274:
6268:
6263:
6260:
6257:
6253:
6249:
6244:
6241:
6238:
6234:
6223:
6220:
6215:
6211:
6203:
6202:
6201:
6185:
6181:
6158:
6154:
6145:
6141:
6134:
6131:
6093:
6089:
6080:
6070:
6056:
6053:
6048:
6044:
6020:
6017:
6014:
6011:
6008:
6002:
5999:
5996:
5993:
5987:
5984:
5981:
5975:
5972:
5969:
5966:
5958:
5954:
5950:
5944:
5941:
5936:
5930:
5927:
5914:
5913:
5912:
5895:
5892:
5886:
5883:
5880:
5877:
5871:
5868:
5863:
5859:
5855:
5852:
5849:
5843:
5840:
5837:
5834:
5828:
5825:
5819:
5816:
5810:
5807:
5801:
5798:
5788:
5787:
5786:
5783:
5769:
5764:
5760:
5756:
5751:
5748:
5745:
5741:
5734:
5731:
5728:
5723:
5720:
5717:
5714:
5684:
5681:
5678:
5674:
5667:
5664:
5661:
5655:
5650:
5646:
5639:
5636:
5633:
5630:
5620:
5619:
5618:
5615:
5604:
5599:
5595:
5591:
5583:
5573:
5569:
5565:
5561:
5553:
5545:
5535:
5530:
5526:
5519:
5515:
5504:
5490:
5487:
5484:
5461:
5453:
5449:
5442:
5428:be the first
5413:
5409:
5379:
5375:
5372:
5353:
5346:
5339:
5333:
5319:
5308:
5304:
5301:
5286:
5283:
5280:
5276:
5271:
5266:
5262:
5231:
5227:
5224:
5200:
5195:
5189:
5182:
5172:
5170:
5166:
5162:
5142:
5133:
5129:
5109:
5105:
5087:
5080:
5076:
5073:
5072:
5071:
5064:
5062:
5058:
5049:
5045:
5041:
5037:
5030:
5019:
4994:
4983:
4979:
4976:
4961:
4958:
4955:
4951:
4946:
4934:
4931:
4928:
4923:
4920:
4908:
4897:
4893:
4890:
4878:
4873:
4869:
4861:
4860:
4859:
4833:
4830:
4827:
4822:
4819:
4807:
4795:
4792:
4789:
4784:
4781:
4769:
4757:
4754:
4751:
4746:
4743:
4740:
4737:
4734:
4731:
4728:
4712:
4711:
4710:
4707:
4703:
4699:
4691:
4687:
4679:
4675:
4667:
4663:up steps and
4660:
4654:
4647:
4643:
4641:
4616:
4612:
4609:
4590:
4575:
4571:
4560:
4556:
4548:
4544:
4537:
4532:
4522:
4509:
4503:
4499:
4487:
4483:
4480:
4465:
4462:
4459:
4455:
4443:
4440:
4437:
4433:
4429:
4423:
4420:
4413:
4410:
4407:
4404:
4399:
4396:
4390:
4384:
4378:
4354:
4348:
4345:
4342:
4338:
4326:
4322:
4319:
4304:
4301:
4298:
4294:
4282:
4279:
4276:
4272:
4268:
4263:
4260:
4257:
4253:
4246:
4243:
4240:
4234:
4231:
4228:
4220:
4214:
4211:
4205:
4192:
4189:
4186:
4182:
4178:
4176:
4166:
4163:
4160:
4156:
4149:
4143:
4140:
4137:
4129:
4126:
4120:
4117:
4114:
4111:
4103:
4100:
4097:
4093:
4079:
4076:
4073:
4069:
4065:
4060:
4057:
4054:
4046:
4043:
4040:
4031:
4025:
4022:
4019:
4011:
4008:
4005:
4001:
3995:
3992:
3986:
3983:
3980:
3977:
3969:
3961:
3958:
3942:
3939:
3936:
3932:
3928:
3925:
3923:
3913:
3905:
3902:
3899:
3890:
3887:
3882:
3878:
3872:
3869:
3863:
3860:
3857:
3854:
3846:
3843:
3840:
3832:
3829:
3813:
3810:
3807:
3803:
3799:
3796:
3791:
3783:
3780:
3777:
3763:
3759:
3755:
3751:
3732:
3729:
3726:
3722:
3718:
3715:
3713:
3706:
3703:
3700:
3697:
3692:
3689:
3677:
3676:
3656:
3653:
3647:
3641:
3636:
3630:
3622:
3617:
3613:
3605:
3604:
3603:
3582:
3579:
3572:
3569:
3566:
3563:
3558:
3555:
3549:
3543:
3537:
3514:
3511:
3504:
3501:
3498:
3495:
3490:
3487:
3481:
3475:
3469:
3462:
3461:
3460:
3458:
3450:
3444:
3440:
3435:
3416:
3411:
3403:
3397:
3394:
3391:
3388:
3385:
3379:
3373:
3366:
3365:
3364:
3347:
3342:
3338:
3332:
3328:
3317:
3314:
3311:
3307:
3303:
3297:
3291:
3284:
3283:
3282:
3280:
3275:
3269:
3260:
3248:
3241:
3237:
3233:
3232:
3231:
3210:
3207:
3204:
3193:
3190:
3187:
3183:
3176:
3172:
3166:
3161:
3158:
3155:
3151:
3147:
3142:
3139:
3136:
3132:
3121:
3118:
3113:
3109:
3101:
3100:
3099:
3098:
3095:
3085:
3083:
3079:
3052:
3048:
3045:
3030:
3027:
3024:
3020:
3015:
3010:
3006:
2998:
2997:
2996:
2973:
2965:
2937:
2934:
2931:
2928:
2925:
2922:
2919:
2916:
2913:
2910:
2907:
2904:
2901:
2898:
2895:
2892:
2889:
2886:
2883:
2880:
2877:
2874:
2871:
2868:
2865:
2862:
2859:
2839:
2836:
2833:
2813:
2793:
2773:
2753:
2727:
2723:
2715:
2714:
2707:
2698:
2688:
2682:
2678:
2674:
2673:
2666:
2416:
2402:
2399:
2396:
2393:
2386:
2385:
2381:
2344:
2330:
2327:
2324:
2321:
2314:
2313:
2309:
2306:
2292:
2289:
2286:
2283:
2276:
2275:
2271:
2268:
2254:
2251:
2248:
2245:
2238:
2237:
2217:
2213:
2209:
2208:
2204:
2195:
2180:
2176:
2172:
2167:
2159:
2155:
2150:
2140:
2136:
2131:
2130:
2123:
2117:
2111:
2107:
2103:
2098:
2091:
2086:
2080:
2072:
2068:
2064:
2056:
2052:
2048:
2044:
2040:
2036:
2032:
2010:
2006:
1999:
1995:
1988:
1985:) = (1, ...,
1984:
1980:
1975:
1965:
1959:
1955:
1949:
1945:
1941:
1940:
1936:
1927:
1920:
1916:
1907:
1903:
1902:line segments
1899:
1893:
1888:
1884:
1883:
1878:
1874:
1868:
1864:
1860:
1849:
1845:
1840:
1839:lattice paths
1834:
1830:
1826:
1823:
1817:
1813:
1808:
1802:
1797:
1791:
1787:
1783:
1782:
1774:
1773:associahedron
1769:
1762:
1749:
1744:
1738:
1734:
1728:
1724:
1720:
1716:
1711:
1707:
1706:
1695:
1690:
1686:
1679:
1675:
1674:parenthesized
1669:
1662:
1658:
1654:
1653:
1638:
1634:
1629:
1625:
1624:
1597:
1592:
1586:
1580:
1576:
1572:
1571:
1568:
1564:
1551:
1547:
1540:
1530:
1525:
1521:
1517:
1516:combinatorics
1507:
1493:
1490:
1483:
1480:
1477:
1474:
1470:
1464:
1460:
1447:
1444:
1441:
1437:
1414:
1410:
1389:
1386:
1383:
1380:
1370:
1356:
1353:
1346:
1342:
1336:
1332:
1319:
1316:
1313:
1309:
1285:
1282:
1279:
1271:
1267:
1263:
1260:
1253:
1250:
1246:
1240:
1235:
1232:
1228:
1222:
1218:
1212:
1209:
1204:
1200:
1197:
1190:
1186:
1183:
1180:
1171:
1167:
1161:
1156:
1152:
1145:
1142:
1138:
1133:
1128:
1124:
1116:
1115:
1114:
1111:
1104:
1094:
1087:
1080:
1076:
1070:
1068:
1052:
1049:
1041:
1037:
1032:
1010:
1001:
994:
990:
986:
982:
975:
971:
965:
960:
956:
932:
929:
926:
915:
912:
909:
905:
898:
895:
892:
884:
881:
878:
875:
869:
863:
858:
854:
843:
840:
835:
831:
823:
822:
821:
804:
801:
798:
787:
784:
781:
777:
771:
768:
765:
761:
755:
750:
747:
744:
740:
736:
731:
727:
716:
713:
708:
704:
696:
695:
694:
693:
688:
687:; see below.
686:
667:
655:
651:
648:
645:
642:
627:
624:
621:
618:
614:
609:
604:
600:
592:
591:
590:
587:
585:
581:
575:
571:
545:
541:
538:
521:
518:
515:
511:
505:
492:
489:
486:
481:
478:
445:
441:
438:
435:
406:
403:
400:
395:
392:
380:
369:
365:
362:
350:
345:
341:
333:
332:
331:
327:
323:
309:
304:
296:
295:
294:
290:
270:
267:
264:
253:
249:
246:
243:
235:
230:
227:
224:
216:
210:
207:
203:
197:
194:
191:
183:
177:
174:
165:
154:
150:
147:
132:
129:
126:
122:
117:
112:
108:
100:
99:
98:
96:
87:
85:
81:
77:
73:
69:
65:
61:
57:
49:
46:
42:
30:
26:
22:
12494:Transposable
12358:Narcissistic
12265:Digital root
12185:Super-Poulet
12145:Jordan–Pólya
12094:prime factor
11999:Noncototient
11966:Almost prime
11948:Superperfect
11923:Refactorable
11918:Quasiperfect
11893:Hyperperfect
11734:Pseudoprimes
11705:Wall–Sun–Sun
11640:Ordered Bell
11610:Fuss–Catalan
11584:
11522:non-centered
11472:Dodecahedral
11449:non-centered
11335:non-centered
11237:Wolstenholme
10982:× 2 ± 1
10979:
10978:Of the form
10945:Eighth power
10925:Fourth power
10813:Davis, Tom:
10803:
10784:
10758:
10746:
10714:
10704:
10698:
10665:
10633:
10621:
10599:
10554:
10550:
10540:
10498:
10494:
10488:
10482:math/0505518
10469:
10466:Sergey Fomin
10461:
10440:
10419:
10408:. Retrieved
10404:the original
10394:
10376:
10355:
10341:(1): 35–40.
10338:
10334:
10321:
10305:
10301:
10295:
10269:
10265:
10259:
10249:
10244:
10236:
10233:
10228:
10219:
10215:
10202:
10193:
10182:
10163:
10159:
10153:
10110:
10106:
10100:
10078:(1): 52–53.
10075:
10071:
10058:
9999:Lobb numbers
9351:
9347:
9341:The Catalan
9340:
9328:
9262:Sergey Fomin
9260:
9153:
9140:
9053:
9042:
9008:
8969:in terms of
8898:
8894:
8891:
8881:
8877:John Riordan
8874:
8870:Désiré André
8855:
8849:
8835:
8494:
8484:
8480:
8477:
8474:
8338:
8326:
8322:
8318:
8311:
8307:
8303:
8167:
8151:
8147:
8143:
8136:
8132:
8122:
8118:
8115:
7730:
7397:
7395:
7384:
7031:
6794:
6600:
6336:
6328:
6120:. Since any
6076:
6035:
5910:
5784:
5702:
5616:
5605:
5597:
5593:
5589:
5581:
5574:
5567:
5563:
5559:
5551:
5543:
5536:
5528:
5524:
5517:
5513:
5510:
5507:Fourth proof
5354:
5344:
5337:
5334:
5198:
5193:
5187:
5180:
5173:
5168:
5164:
5160:
5140:
5138:
5114:
5099:
5078:
5065:
5060:
5056:
5054:
5039:
5035:
5028:
5025:
5009:
4857:
4708:
4701:
4697:
4689:
4685:
4677:
4673:
4665:
4658:
4652:
4648:
4644:
4639:
4588:
4573:
4569:
4566:
4558:
4554:
4546:
4542:
4525:Second proof
3678:
3672:
3601:
3442:
3438:
3434:power series
3431:
3362:
3276:
3267:
3258:
3255:
3246:
3239:
3235:
3225:
3091:
3075:
2994:
2964:Bell numbers
2696:
2695:1, 2, ..., 2
2680:
2676:
2215:
2211:
2193:
2178:
2174:
2165:
2157:
2153:
2138:
2134:
2128:
2121:
2109:
2105:
2096:
2089:
2078:
2070:
2066:
2054:
2050:
2046:
2042:
2038:
2034:
2030:
2008:
2004:
1997:
1993:
1986:
1982:
1978:
1963:
1958:permutations
1947:
1943:
1925:
1918:
1914:
1891:
1872:
1858:
1855:
1847:
1843:
1832:
1828:
1815:
1811:
1800:
1789:
1785:
1747:
1736:
1732:
1726:
1722:
1718:
1714:
1693:
1687:, as in the
1667:
1660:
1656:
1636:
1632:
1590:
1578:
1574:
1566:
1562:
1538:
1528:
1519:
1513:
1371:
1300:
1112:
1102:
1092:
1085:
1078:
1074:
1071:
1033:
947:
819:
689:
682:
588:
573:
569:
460:
325:
321:
318:
288:
285:
88:
59:
53:
25:
12515:Extravagant
12510:Equidigital
12465:permutation
12424:Palindromic
12398:Automorphic
12296:Sum-product
12275:Sum-product
12230:Persistence
12125:Erdős–Woods
12047:Untouchable
11928:Semiperfect
11878:Hemiperfect
11539:Tesseractic
11477:Icosahedral
11457:Tetrahedral
11388:Dodecagonal
11089:Recursively
10960:Prime power
10935:Sixth power
10930:Fifth power
10910:Power of 10
10868:Classes of
10160:Mathematics
9684: even,
9270:root system
8836:et cetera.
8159:determinant
7492:dominating
7440:Y's, where
7391:cycle lemma
7381:Sixth proof
6073:Fifth proof
5022:Third proof
4551:instead of
3088:First proof
2986:is missing.
2149:Bell number
2118:of the set
1904:(a form of
1822:parse trees
1678:associating
1628:parenthesis
685:cycle lemma
76:recursively
12776:Categories
12727:Graphemics
12600:Pernicious
12454:Undulating
12429:Pandigital
12403:Trimorphic
12004:Nontotient
11853:Arithmetic
11467:Octahedral
11368:Heptagonal
11358:Pentagonal
11343:Triangular
11184:Sierpiński
11106:Jacobsthal
10905:Power of 3
10900:Power of 2
10768:2008.00133
10686:95:96–102.
10593:References
10508:1809.07523
10452:2008.00133
10410:2014-06-24
10367:2105.07884
10187:Dyck paths
10177:,Theorem 1
10120:1809.07523
9912: odd.
9145:Ira Gessel
8852:volume III
7398:dominating
7387:Dyck words
6079:Dyck words
5141:reversible
5128:diagonal.
5057:exceedance
4581:right and
4529:See also:
2852:these are
2164:{1, ..., 2
2129:A fortiori
1972:is called
1956:-sortable
1587:of length
1585:Dyck words
315:Properties
300:(sequence
48:partitions
12484:Parasitic
12333:Factorion
12260:Digit sum
12252:Digit sum
12070:Fortunate
12057:Primorial
11971:Semiprime
11908:Practical
11873:Descartes
11868:Deficient
11858:Betrothed
11700:Wieferich
11529:Pentatope
11492:pyramidal
11383:Decagonal
11378:Nonagonal
11373:Octagonal
11363:Hexagonal
11222:Practical
11169:Congruent
11101:Fibonacci
11065:Loeschian
10805:MathWorld
10564:1209.6270
10557:: 35–55.
10533:214165563
10431:1208.4196
10166:(3): 40,
10145:214165563
10029:Semiorder
9884:−
9845:⋯
9747:−
9729:⋯
9629:⋯
9557:−
9534:⋯
9466:⋯
9439:≥
9423:…
9386:⋯
9367:∑
8986:α
8980:
8954:α
8945:
8919:α
8910:
8872:in 1887.
7533:−
7477:−
7254:−
7155:−
7060:−
7009:−
6985:−
6949:∑
6937:−
6898:∑
6894:−
6886:−
6848:∑
6822:−
6775:−
6714:∑
6691:−
6578:−
6540:∑
6310:≥
6303:for
6293:−
6254:∑
6000:−
5973:−
5884:−
5841:−
5749:−
5721:−
4909:−
4793:−
4755:−
4732:−
4449:∞
4434:∑
4408:−
4400:−
4288:∞
4273:∑
4198:∞
4183:∑
4118:−
4085:∞
4070:∑
4041:−
3984:−
3959:−
3948:∞
3933:∑
3929:−
3900:−
3861:−
3844:−
3830:−
3819:∞
3804:∑
3800:−
3778:−
3738:∞
3723:∑
3719:−
3701:−
3693:−
3634:→
3567:−
3559:−
3499:−
3323:∞
3308:∑
3208:≥
3201:for
3191:−
3152:∑
2120:{1, ...,
2077:{1, ...,
1962:{1, ...,
1898:triangles
1453:∞
1438:∑
1325:∞
1310:∑
1264:−
1233:−
1229:∫
1213:π
1184:−
1153:∫
1146:π
1002:π
966:∼
923:for
913:−
882:−
795:for
785:−
769:−
741:∑
439:≥
381:−
268:≥
261:for
221:∏
12556:Friedman
12489:Primeval
12434:Repdigit
12391:-related
12338:Kaprekar
12312:Meertens
12235:Additive
12222:dynamics
12130:Friendly
12042:Sociable
12032:Amicable
11843:Abundant
11823:dynamics
11645:Schröder
11635:Narayana
11605:Eulerian
11595:Delannoy
11590:Dedekind
11411:centered
11277:centered
11164:Amenable
11121:Narayana
11111:Leonardo
11007:Mersenne
10955:Powerful
10895:Achilles
10631:(1988),
10583:15430707
10272:: 9–28,
10092:27646275
9936:See also
8888:Mingantu
8343:we have
8172:we have
8088:X's and
7927:X's and
7797:X's and
7751:X's and
7420:X's and
6487:′
5911:we have
5785:Because
5165:starting
5061:vertical
5014:X's and
4700:− 1) × (
3094:Segner's
1991:, where
1602:X's and
84:Minggatu
64:sequence
12729:related
12693:related
12657:related
12655:Sorting
12540:Vampire
12525:Harshad
12467:related
12439:Repunit
12353:Lychrel
12328:Dudeney
12180:Størmer
12175:Sphenic
12160:Regular
12098:divisor
12037:Perfect
11933:Sublime
11903:Perfect
11630:Motzkin
11585:Catalan
11126:Padovan
11060:Leyland
11055:Idoneal
11050:Hilbert
11022:Woodall
10738:1676282
10643:Bibcode
10620:(1996)
10525:4052255
10137:4052255
9051:votes.
8840:History
6340:, i.e.
5079:touches
3445:+ 1 = 0
2966:, only
2826:). For
2667:5 ways
2608:/\/\/\,
2382:2 ways
2058:, with
1088:= 2 − 1
580:integer
306:in the
303:A000108
12595:Odious
12520:Frugal
12474:Cyclic
12463:Digit-
12170:Smooth
12155:Pronic
12115:Cyclic
12092:Other
12065:Euclid
11715:Wilson
11689:Primes
11348:Square
11217:Polite
11179:Riesel
11174:Knödel
11136:Perrin
11017:Thabit
11002:Fermat
10992:Cullen
10915:Square
10883:Powers
10736:
10726:
10707:: 5–7.
10673:
10653:
10614:Conway
10606:
10581:
10531:
10523:
10253:online
10143:
10135:
10090:
9355:, is:
9143:, per
8129:whose
5703:Write
5401:. Let
4371:Thus,
2310:1 way
2272:1 way
2012:where
1596:string
578:is an
62:are a
58:, the
12636:Prime
12631:Lucky
12620:sieve
12549:Other
12535:Smith
12415:Digit
12373:Happy
12348:Keith
12321:Other
12165:Rough
12135:Giuga
11600:Euler
11462:Cubic
11116:Lucas
11012:Proth
10789:(PDF)
10763:arXiv
10751:(PDF)
10695:(PDF)
10579:S2CID
10559:arXiv
10529:S2CID
10503:arXiv
10478:arXiv
10447:arXiv
10426:arXiv
10386:(PDF)
10362:arXiv
10331:(PDF)
10212:(PDF)
10141:S2CID
10115:arXiv
10088:JSTOR
10068:(PDF)
10051:Notes
8884:China
7728:are.
6664:, so
6644:than
6506:, so
6384:, so
5617:Thus
5579:with
5541:with
5169:below
4688:− 1,
4545:– 1,
3447:as a
2368:/\/\,
2187:with
1954:stack
1889:with
1798:with
1745:with
1743:trees
1065:, or
1038:, by
12590:Evil
12270:Self
12220:and
12110:Blum
11821:and
11625:Lobb
11580:Cake
11575:Bell
11325:Star
11232:Ulam
11131:Pell
10920:Cube
10724:ISBN
10671:ISBN
10651:ISBN
10616:and
10604:ISBN
9238:and
9154:For
8934:and
8807:1430
8157:has
8116:The
7692:and
7451:>
6364:and
6329:Let
5592:+ 2)
5562:+ 2)
5522:and
5161:last
4704:+ 1)
4692:+ 1)
4549:+ 1)
3277:The
3265:and
2974:1213
2938:1111
2932:1112
2926:1121
2920:1122
2914:1123
2908:1211
2902:1212
2896:1221
2890:1222
2884:1223
2878:1231
2872:1232
2866:1233
2860:1234
2630:\/\,
2586:/\/\
2147:-th
2037:) =
2024:and
2020:and
1771:The
1567:down
1565:and
1557:and
1544:= 14
1536:and
1100:and
1042:for
930:>
820:and
802:>
428:for
308:OEIS
293:are
89:The
38:= 42
32:The
12708:Ban
12096:or
11615:Lah
10618:Guy
10569:doi
10513:doi
10499:343
10343:doi
10310:doi
10282:hdl
10274:doi
10168:doi
10125:doi
10111:343
10080:doi
9045:+ 1
8977:sin
8942:sin
8907:sin
8802:429
8797:132
8785:429
8780:132
8763:132
8715:det
8678:132
8623:det
8558:det
8510:det
8497:+ 1
8446:429
8441:132
8424:132
8354:det
8341:= 4
8275:132
8183:det
8170:= 4
6472:or
6229:and
5600:+ 1
5584:+ 3
5554:+ 1
5546:+ 2
5350:= 5
5340:= 3
5201:+ 1
5194:all
5190:− 2
5183:− 1
5119:to
5031:+ 1
4668:− 1
4661:+ 1
4640:bad
3627:lim
3451:of
3238:= X
3127:and
2786:or
2612:/\/
2307:/\
2196:= 4
2151:.
2099:= 4
2092:= 3
2009:unv
1976:if
1960:of
1928:= 4
1894:+ 2
1861:= 4
1803:+ 1
1750:+ 1
1696:= 3
1670:+ 1
1534:= 5
1402:is
1108:= 5
1098:= 2
849:and
722:and
330:is
97:by
66:of
54:In
12778::
10802:.
10761:,
10734:MR
10732:,
10722:,
10705:32
10703:.
10697:.
10649:,
10641:,
10577:.
10567:.
10555:56
10553:.
10549:.
10527:,
10521:MR
10519:,
10511:,
10497:,
10472:,
10470:13
10339:11
10337:.
10333:.
10306:14
10304:,
10280:,
10270:31
10268:,
10220:56
10218:.
10214:.
10162:,
10139:,
10133:MR
10131:,
10123:,
10109:,
10086:.
10076:37
10074:.
10070:.
9350:=
9333:.
9001:.
8879:.
8792:42
8775:42
8770:14
8758:42
8753:14
8741:42
8736:14
8673:42
8668:14
8661:42
8656:14
8644:14
8586:14
8499:.
8483:×
8460:1.
8436:42
8431:14
8419:42
8414:14
8402:42
8397:14
8380:14
8329:−1
8310:,
8289:1.
8270:42
8265:14
8253:42
8248:14
8231:14
8154:−2
8135:,
8121:×
7656:,
7620:,
7036:,
6118:c'
5558:(4
5534:.
5531:+1
5503:.
4676:,
4572:×
4557:,
3441:−
3439:xc
3274:.
3211:0.
2664:\
2647:\,
2619:\,
2567:/\
2554:/\
2519:/\
2379:\
2366:/\
2269:*
2132:,
2126:.
2007:=
1885:A
1863::
1846:×
1725:,
1721:,
1717:,
1611:XY
1563:up
1546:.
1506:.
1369:.
1110:.
1069:.
933:0.
586:.
310:).
271:0.
86:.
45:52
10980:a
10861:e
10854:t
10847:v
10808:.
10765::
10645::
10610:.
10585:.
10571::
10561::
10515::
10505::
10480::
10455:.
10449::
10434:.
10428::
10413:.
10388:.
10370:.
10364::
10349:.
10345::
10312::
10284::
10276::
10237:7
10170::
10164:5
10127::
10117::
10094:.
10082::
9908:m
9903:,
9898:2
9894:/
9890:)
9887:1
9881:m
9878:(
9875:+
9872:n
9868:C
9860:)
9857:m
9854:+
9851:n
9848:(
9842:)
9839:1
9836:+
9833:2
9829:/
9825:)
9822:3
9819:+
9816:m
9813:(
9810:+
9807:n
9804:(
9801:)
9798:2
9794:/
9790:)
9787:3
9784:+
9781:m
9778:(
9775:+
9772:n
9769:(
9764:)
9761:2
9757:/
9753:)
9750:1
9744:m
9741:(
9738:+
9735:n
9732:(
9726:)
9723:2
9720:+
9717:n
9714:(
9711:)
9708:1
9705:+
9702:n
9699:(
9696:m
9680:m
9675:,
9670:2
9666:/
9662:m
9659:+
9656:n
9652:C
9644:)
9641:m
9638:+
9635:n
9632:(
9626:)
9623:3
9620:+
9617:2
9613:/
9609:m
9606:+
9603:n
9600:(
9597:)
9594:2
9591:+
9588:2
9584:/
9580:m
9577:+
9574:n
9571:(
9568:2
9563:)
9560:1
9554:2
9550:/
9546:m
9543:+
9540:n
9537:(
9531:)
9528:2
9525:+
9522:n
9519:(
9516:)
9513:1
9510:+
9507:n
9504:(
9501:m
9491:{
9486:=
9479:m
9475:i
9470:C
9459:1
9455:i
9450:C
9442:0
9434:m
9430:i
9426:,
9420:,
9415:1
9411:i
9405:n
9402:=
9397:m
9393:i
9389:+
9383:+
9378:1
9374:i
9352:m
9348:k
9343:k
9312:n
9308:A
9285:n
9281:C
9246:4
9226:3
9223:,
9220:2
9217:=
9214:m
9194:n
9191:=
9188:m
9168:1
9165:=
9162:m
9124:!
9121:n
9118:!
9115:m
9112:!
9109:)
9106:n
9103:+
9100:m
9097:(
9092:!
9089:)
9086:n
9083:2
9080:(
9077:!
9074:)
9071:m
9068:2
9065:(
9049:n
9043:n
9026:n
9022:C
8989:)
8983:(
8957:)
8951:4
8948:(
8922:)
8916:2
8913:(
8821:5
8818:=
8813:]
8748:5
8731:5
8726:2
8720:[
8692:4
8689:=
8684:]
8651:5
8639:5
8634:2
8628:[
8600:3
8597:=
8592:]
8581:5
8574:5
8569:2
8563:[
8535:2
8532:=
8527:]
8521:2
8515:[
8495:n
8490:2
8485:n
8481:n
8457:=
8452:]
8409:5
8392:5
8387:2
8375:5
8370:2
8365:1
8359:[
8339:n
8334:n
8327:j
8325:+
8323:i
8319:C
8314:)
8312:j
8308:i
8306:(
8286:=
8281:]
8260:5
8243:5
8238:2
8226:5
8221:2
8216:1
8209:5
8204:2
8199:1
8194:1
8188:[
8168:n
8163:n
8152:j
8150:+
8148:i
8144:C
8139:)
8137:j
8133:i
8131:(
8123:n
8119:n
8096:n
8076:1
8073:+
8070:n
8047:n
8043:C
8039:=
8033:)
8028:n
8024:1
8021:+
8018:n
8015:2
8009:(
8000:1
7997:+
7994:n
7991:2
7987:1
7964:1
7961:+
7958:n
7955:2
7935:n
7915:1
7912:+
7909:n
7885:)
7880:n
7876:1
7873:+
7870:n
7867:2
7861:(
7837:1
7834:+
7831:n
7828:=
7825:m
7805:n
7785:1
7782:+
7779:n
7759:n
7739:n
7714:X
7711:Y
7708:X
7705:X
7702:Y
7678:Y
7675:X
7672:X
7669:Y
7666:X
7642:X
7639:X
7636:Y
7633:X
7630:Y
7606:X
7603:Y
7600:X
7597:Y
7594:X
7570:Y
7567:X
7564:Y
7561:X
7558:X
7536:n
7530:m
7510:n
7507:+
7504:m
7480:n
7474:m
7454:n
7448:m
7428:n
7408:m
7362:)
7357:i
7353:i
7350:2
7344:(
7335:1
7332:+
7329:i
7325:1
7320:=
7315:i
7311:C
7284:)
7279:i
7275:1
7272:+
7269:i
7266:2
7260:(
7248:)
7243:i
7239:i
7236:2
7230:(
7224:2
7221:=
7216:i
7212:C
7185:)
7180:i
7176:1
7173:+
7170:i
7167:2
7161:(
7150:i
7146:B
7142:2
7139:=
7134:i
7130:C
7103:)
7098:i
7094:1
7091:+
7088:i
7085:2
7079:(
7073:=
7068:i
7064:C
7055:i
7051:B
7047:2
7034:n
7017:.
7012:i
7006:n
7002:C
6998:)
6993:i
6989:C
6980:i
6976:B
6972:2
6969:(
6964:n
6959:0
6956:=
6953:i
6945:=
6940:i
6934:n
6930:C
6923:i
6919:C
6913:n
6908:0
6905:=
6902:i
6889:i
6883:n
6879:C
6873:i
6869:B
6863:n
6858:0
6855:=
6852:i
6844:2
6841:=
6836:1
6833:+
6830:n
6826:C
6817:1
6814:+
6811:n
6807:B
6778:i
6772:n
6768:C
6761:)
6756:i
6752:1
6749:+
6746:i
6743:2
6737:(
6729:n
6724:0
6721:=
6718:i
6710:=
6705:1
6702:+
6699:n
6695:C
6686:1
6683:+
6680:n
6676:B
6652:)
6632:(
6612:)
6609:c
6586:.
6581:i
6575:n
6571:C
6565:i
6561:B
6555:n
6550:0
6547:=
6544:i
6536:2
6533:=
6528:1
6525:+
6522:n
6518:B
6494:b
6491:(
6484:c
6480:)
6460:b
6457:)
6454:c
6451:(
6427:)
6422:n
6418:n
6415:2
6409:(
6403:=
6398:n
6394:B
6372:)
6352:(
6342:b
6337:n
6335:2
6331:b
6325:.
6313:0
6307:n
6296:i
6290:n
6286:C
6279:i
6275:C
6269:n
6264:0
6261:=
6258:i
6250:=
6245:1
6242:+
6239:n
6235:C
6224:1
6221:=
6216:0
6212:C
6186:1
6182:c
6159:2
6155:c
6151:)
6146:1
6142:c
6138:(
6135:=
6132:c
6122:c
6114:c
6110:n
6094:n
6090:C
6057:1
6054:=
6049:0
6045:C
6021:.
6018:!
6015:!
6012:!
6009:!
6006:)
6003:2
5997:n
5994:4
5991:(
5988:=
5985:!
5982:!
5979:)
5976:1
5970:n
5967:2
5964:(
5959:n
5955:2
5951:=
5945:!
5942:n
5937:!
5934:)
5931:n
5928:2
5925:(
5896:!
5893:!
5890:)
5887:1
5881:n
5878:2
5875:(
5872:!
5869:n
5864:n
5860:2
5856:=
5853:!
5850:!
5847:)
5844:1
5838:n
5835:2
5832:(
5829:!
5826:!
5823:)
5820:n
5817:2
5814:(
5811:=
5808:!
5805:)
5802:n
5799:2
5796:(
5770:.
5765:n
5761:C
5757:=
5752:1
5746:n
5742:C
5735:1
5732:+
5729:n
5724:2
5718:n
5715:4
5699:.
5685:1
5682:+
5679:n
5675:C
5671:)
5668:2
5665:+
5662:n
5659:(
5656:=
5651:n
5647:C
5643:)
5640:2
5637:+
5634:n
5631:4
5628:(
5612:P
5608:Q
5598:n
5594:C
5590:n
5588:(
5582:n
5577:Q
5568:n
5564:C
5560:n
5552:n
5550:2
5544:n
5539:P
5529:n
5525:C
5518:n
5514:C
5491:F
5488:X
5485:L
5465:)
5462:L
5459:(
5454:d
5450:X
5446:)
5443:F
5440:(
5430:X
5414:d
5410:X
5385:)
5380:n
5376:n
5373:2
5367:(
5348:3
5345:C
5338:n
5320:.
5314:)
5309:n
5305:n
5302:2
5296:(
5287:1
5284:+
5281:n
5277:1
5272:=
5267:n
5263:C
5237:)
5232:n
5228:n
5225:2
5219:(
5205:n
5199:n
5188:n
5181:n
5176:n
5157:X
5153:P
5149:n
5145:P
5125:1
5121:2
5117:3
5102:X
5096:.
5094:X
5090:X
5083:X
5068:1
5040:n
5036:C
5029:n
5016:n
5012:n
4995:.
4989:)
4984:n
4980:n
4977:2
4971:(
4962:1
4959:+
4956:n
4952:1
4947:=
4941:)
4935:1
4932:+
4929:n
4924:n
4921:2
4915:(
4903:)
4898:n
4894:n
4891:2
4885:(
4879:=
4874:n
4870:C
4840:)
4834:1
4831:+
4828:n
4823:n
4820:2
4814:(
4808:=
4802:)
4796:1
4790:n
4785:n
4782:2
4776:(
4770:=
4764:)
4758:1
4752:n
4747:1
4744:+
4741:n
4738:+
4735:1
4729:n
4723:(
4702:n
4698:n
4696:(
4690:n
4686:n
4684:(
4680:)
4678:n
4674:n
4672:(
4666:n
4659:n
4653:n
4651:2
4622:)
4617:n
4613:n
4610:2
4604:(
4589:n
4587:2
4583:n
4579:n
4574:n
4570:n
4563:.
4561:)
4559:n
4555:n
4553:(
4547:n
4543:n
4541:(
4510:.
4504:n
4500:x
4493:)
4488:n
4484:n
4481:2
4475:(
4466:1
4463:+
4460:n
4456:1
4444:0
4441:=
4438:n
4430:=
4424:x
4421:2
4414:x
4411:4
4405:1
4397:1
4391:=
4388:)
4385:x
4382:(
4379:c
4355:.
4349:1
4346:+
4343:n
4339:x
4332:)
4327:n
4323:n
4320:2
4314:(
4305:1
4302:+
4299:n
4295:2
4283:0
4280:=
4277:n
4269:=
4264:1
4261:+
4258:n
4254:x
4247:!
4244:n
4241:!
4238:)
4235:1
4232:+
4229:n
4226:(
4221:!
4218:)
4215:n
4212:2
4209:(
4206:2
4193:0
4190:=
4187:n
4179:=
4167:1
4164:+
4161:n
4157:x
4150:!
4147:)
4144:1
4141:+
4138:n
4135:(
4130:!
4127:!
4124:)
4121:1
4115:n
4112:2
4109:(
4104:1
4101:+
4098:n
4094:2
4080:0
4077:=
4074:n
4066:=
4061:1
4058:+
4055:n
4051:)
4047:x
4044:4
4038:(
4032:!
4029:)
4026:1
4023:+
4020:n
4017:(
4012:1
4009:+
4006:n
4002:2
3996:!
3993:!
3990:)
3987:1
3981:n
3978:2
3975:(
3970:n
3966:)
3962:1
3956:(
3943:0
3940:=
3937:n
3926:=
3914:n
3910:)
3906:x
3903:4
3897:(
3891:!
3888:n
3883:n
3879:2
3873:!
3870:!
3867:)
3864:3
3858:n
3855:2
3852:(
3847:1
3841:n
3837:)
3833:1
3827:(
3814:1
3811:=
3808:n
3797:=
3792:n
3788:)
3784:x
3781:4
3775:(
3769:)
3764:n
3760:2
3756:/
3752:1
3746:(
3733:1
3730:=
3727:n
3716:=
3707:x
3704:4
3698:1
3690:1
3669:.
3657:1
3654:=
3651:)
3648:x
3645:(
3642:c
3637:0
3631:x
3623:=
3618:0
3614:C
3598:.
3583:x
3580:2
3573:x
3570:4
3564:1
3556:1
3550:=
3547:)
3544:x
3541:(
3538:c
3515:x
3512:2
3505:x
3502:4
3496:1
3491:+
3488:1
3482:=
3479:)
3476:x
3473:(
3470:c
3453:c
3443:c
3417:;
3412:2
3408:)
3404:x
3401:(
3398:c
3395:x
3392:+
3389:1
3386:=
3383:)
3380:x
3377:(
3374:c
3348:.
3343:n
3339:x
3333:n
3329:C
3318:0
3315:=
3312:n
3304:=
3301:)
3298:x
3295:(
3292:c
3271:2
3268:w
3262:1
3259:w
3250:2
3247:w
3245:Y
3243:1
3240:w
3236:w
3228:w
3205:n
3194:i
3188:n
3184:C
3177:i
3173:C
3167:n
3162:0
3159:=
3156:i
3148:=
3143:1
3140:+
3137:n
3133:C
3122:1
3119:=
3114:0
3110:C
3058:)
3053:n
3049:n
3046:2
3040:(
3031:1
3028:+
3025:n
3021:1
3016:=
3011:n
3007:C
2960:1
2956:1
2952:0
2935:,
2929:,
2923:,
2917:,
2911:,
2905:,
2899:,
2893:,
2887:,
2881:,
2875:,
2869:,
2863:,
2840:4
2837:=
2834:n
2814:1
2794:1
2774:0
2754:1
2744:n
2728:n
2724:C
2708:.
2702:n
2697:n
2691:n
2681:n
2677:C
2662:0
2659:0
2656:0
2653:0
2651:/
2649:0
2645:0
2642:0
2639:0
2636:0
2634:/
2632:0
2628:0
2625:0
2623:/
2621:0
2617:0
2614:0
2610:0
2606:\
2604:0
2601:0
2599:/
2597:0
2594:0
2591:0
2588:0
2584:0
2581:0
2578:0
2575:0
2572:0
2569:0
2565:0
2562:0
2559:0
2556:0
2552:0
2549:0
2546:0
2543:0
2540:0
2537:0
2534:0
2531:0
2528:0
2525:0
2522:0
2517:0
2514:0
2511:0
2508:0
2505:0
2502:0
2499:0
2496:0
2493:0
2490:0
2487:0
2484:0
2481:0
2478:0
2475:0
2472:0
2469:0
2466:0
2463:0
2460:0
2457:0
2454:0
2451:0
2448:0
2445:0
2442:0
2439:0
2436:0
2433:0
2430:0
2427:0
2424:0
2421:0
2418:0
2403::
2400:3
2397:=
2394:n
2377:0
2374:0
2372:/
2370:0
2364:0
2361:0
2358:0
2355:0
2352:0
2349:0
2346:0
2331::
2328:2
2325:=
2322:n
2293::
2290:1
2287:=
2284:n
2255::
2252:0
2249:=
2246:n
2226:n
2222:n
2216:n
2212:C
2198::
2194:n
2189:n
2185:n
2179:n
2175:C
2168:}
2166:n
2158:n
2154:C
2145:n
2139:n
2135:C
2124:}
2122:n
2110:n
2106:C
2097:n
2090:n
2081:}
2079:n
2071:n
2067:C
2060:S
2055:n
2053:)
2051:v
2049:(
2047:S
2045:)
2043:u
2041:(
2039:S
2035:w
2033:(
2031:S
2026:v
2022:u
2018:w
2014:n
2005:w
2000:)
1998:w
1996:(
1994:S
1989:)
1987:n
1983:w
1981:(
1979:S
1970:w
1966:}
1964:n
1948:n
1944:C
1930::
1926:n
1919:n
1915:C
1910:n
1892:n
1859:n
1848:n
1844:n
1833:n
1829:C
1816:n
1812:C
1801:n
1790:n
1786:C
1777:4
1754:n
1748:n
1737:n
1733:C
1727:d
1723:c
1719:b
1715:a
1694:n
1681:n
1668:n
1661:n
1657:C
1643:n
1637:n
1633:C
1604:n
1600:n
1591:n
1589:2
1579:n
1575:C
1559:)
1555:(
1542:4
1539:C
1532:3
1529:C
1494:1
1491:=
1484:1
1481:+
1478:n
1475:2
1471:2
1465:n
1461:C
1448:0
1445:=
1442:n
1415:k
1411:C
1390:1
1387:+
1384:k
1381:2
1357:2
1354:=
1347:n
1343:4
1337:n
1333:C
1320:0
1317:=
1314:n
1286:.
1283:t
1280:d
1272:2
1268:t
1261:1
1254:n
1251:2
1247:t
1241:1
1236:1
1223:n
1219:4
1210:2
1205:=
1201:x
1198:d
1191:x
1187:x
1181:4
1172:n
1168:x
1162:4
1157:0
1143:2
1139:1
1134:=
1129:n
1125:C
1106:3
1103:C
1096:2
1093:C
1086:n
1079:n
1075:C
1053:!
1050:n
1029:n
1025:n
1011:,
995:2
991:/
987:3
983:n
976:n
972:4
961:n
957:C
927:n
916:1
910:n
906:C
899:1
896:+
893:n
888:)
885:1
879:n
876:2
873:(
870:2
864:=
859:n
855:C
844:1
841:=
836:0
832:C
805:0
799:n
788:i
782:n
778:C
772:1
766:i
762:C
756:n
751:1
748:=
745:i
737:=
732:n
728:C
717:1
714:=
709:0
705:C
668:,
661:)
656:n
652:1
649:+
646:n
643:2
637:(
628:1
625:+
622:n
619:2
615:1
610:=
605:n
601:C
574:n
570:C
551:)
546:n
542:n
539:2
533:(
522:1
519:+
516:n
512:n
506:=
499:)
493:1
490:+
487:n
482:n
479:2
473:(
446:,
442:0
436:n
413:)
407:1
404:+
401:n
396:n
393:2
387:(
375:)
370:n
366:n
363:2
357:(
351:=
346:n
342:C
326:n
322:C
289:n
265:n
254:k
250:k
247:+
244:n
236:n
231:2
228:=
225:k
217:=
211:!
208:n
204:!
201:)
198:1
195:+
192:n
189:(
184:!
181:)
178:n
175:2
172:(
166:=
160:)
155:n
151:n
148:2
142:(
133:1
130:+
127:n
123:1
118:=
113:n
109:C
91:n
50:)
36:5
34:C
23:.
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.