Riemann integral - Knowledge

20: 36: 28: 8934: 8282: 2234: 3138: 6532:

The Lebesgue–Vitali theorem does not imply that all type of discontinuities have the same weight on the obstruction that a real-valued bounded function be Riemann integrable on . In fact, certain discontinuities have absolutely no role on the Riemann integrability of the function—a consequence of the

7893:

is a generalisation of the Lebesgue integral that is at the same time closer to the Riemann integral. These more general theories allow for the integration of more "jagged" or "highly oscillating" functions whose Riemann integral does not exist; but the theories give the same value as the Riemann

7943:

The Riemann integral was introduced in Bernhard Riemann's paper "Über die Darstellbarkeit einer Function durch eine trigonometrische Reihe" (On the representability of a function by a trigonometric series; i.e., when can a function be represented by a trigonometric series). This paper was submitted

6494:

zero, and thus a bounded function (on a compact interval) with only finitely or countably many discontinuities is Riemann integrable. Another sufficient criterion to Riemann integrability over , but which does not involve the concept of measure, is the existence of a right-hand (or left-hand) limit

5127:

However, combining these restrictions, so that one uses only left-hand or right-hand Riemann sums on regularly divided intervals, is dangerous. If a function is known in advance to be Riemann integrable, then this technique will give the correct value of the integral. But under these conditions the

2277:

One important requirement is that the mesh of the partitions must become smaller and smaller, so that it has the limit zero. If this were not so, then we would not be getting a good approximation to the function on certain subintervals. In fact, this is enough to define an integral. To be specific,

7770:

This demonstrates that for integrals on unbounded intervals, uniform convergence of a function is not strong enough to allow passing a limit through an integral sign. This makes the Riemann integral unworkable in applications (even though the Riemann integral assigns both sides the correct value),

3710: 5160:

will appear to be integrable on with integral equal to one: Every endpoint of every subinterval will be a rational number, so the function will always be evaluated at rational numbers, and hence it will appear to always equal one. The problem with this definition becomes apparent when we try to

5334: 7778:. The definition of the Lebesgue integral is not obviously a generalization of the Riemann integral, but it is not hard to prove that every Riemann-integrable function is Lebesgue-integrable and that the values of the two integrals agree whenever they are both defined. Moreover, a function 2482:

Unfortunately, this definition is very difficult to use. It would help to develop an equivalent definition of the Riemann integral which is easier to work with. We develop this definition now, with a proof of equivalence following. Our new definition says that the Riemann integral of

4066: 7340:

In general, this improper Riemann integral is undefined. Even standardizing a way for the interval to approach the real line does not work because it leads to disturbingly counterintuitive results. If we agree (for instance) that the improper integral should always be

6723: 5562: 1910: 7015: 7336: 7766: 2957: 2738: 2477: 3503: 4245:

takes the value 1 on rational numbers and 0 on irrational numbers. This function does not have a Riemann integral. To prove this, we will show how to construct tagged partitions whose Riemann sums get arbitrarily close to both zero and one.

5164: 5338:

If we use regular subdivisions and left-hand or right-hand Riemann sums, then the two terms on the left are equal to zero, since every endpoint except 0 and 1 will be irrational, but as we have seen the term on the right will equal 1.

7433:

Unfortunately, the improper Riemann integral is not powerful enough. The most severe problem is that there are no widely applicable theorems for commuting improper Riemann integrals with limits of functions. In applications such as

7567: 7956:.) For Riemann's definition of his integral, see section 4, "Über den Begriff eines bestimmten Integrals und den Umfang seiner Gültigkeit" (On the concept of a definite integral and the extent of its validity), pages 101–103. 4932:

Some calculus books do not use general tagged partitions, but limit themselves to specific types of tagged partitions. If the type of partition is limited too much, some non-integrable functions may appear to be integrable.

1387: 7901:

offers a simpler definition that is easier to work with; it can be used to introduce the Riemann integral. The Darboux integral is defined whenever the Riemann integral is, and always gives the same result. Conversely, the

5647:

definition of integrability (formally, the Riemann condition for integrability) – a function is Riemann integrable if and only if the upper and lower sums can be made arbitrarily close by choosing an appropriate partition.

6870: 3916: 3892: 1163: 3283:

on . If all intervals had this property, then this would conclude the proof, because each term in the Riemann sum would be bounded by a corresponding term in the Darboux sums, and we chose the Darboux sums to be near

4823:

is equivalent (that is, equal almost everywhere) to a Riemann integrable function, but there are non-Riemann integrable bounded functions which are not equivalent to any Riemann integrable function. For example, let

7425:

is −2, so this definition is not invariant under shifts, a highly undesirable property. In fact, not only does this function not have an improper Riemann integral, its Lebesgue integral is also undefined (it equals

7200:. But there are many ways for the interval of integration to expand to fill the real line, and other ways can produce different results; in other words, the multivariate limit does not always exist. We can compute 6577: 1855: 6731:(on a monotone pointwise limit) does not hold for Riemann integrals. Thus, in Riemann integration, taking limits under the integral sign is far more difficult to logically justify than in Lebesgue integration. 3423: 1494: 7412: 7093: 5406: 2242:

is continuous, then the lower and upper Darboux sums for an untagged partition are equal to the Riemann sum for that partition, where the tags are chosen to be the minimum or maximum (respectively) of

6892: 7651: 7203: 6521:

on the interval it is Riemann integrable, since its set of discontinuities is at most countable, and therefore of Lebesgue measure zero. If a real-valued function on is Riemann integrable, it is

7771:

because there is no other general criterion for exchanging a limit and a Riemann integral, and without such a criterion it is difficult to approximate integrals by approximating their integrands.

142: 4757: 7669: 7208: 1915: 1859:

Each term in the sum is the product of the value of the function at a given point and the length of an interval. Consequently, each term represents the (signed) area of a rectangle with height

4210: 7863: 3791: 3263: 2610: 2349: 7906:

is a simple but more powerful generalization of the Riemann integral and has led some educators to advocate that it should replace the Riemann integral in introductory calculus courses.

5120: 7438:

it is important to be able to approximate the integral of a function using integrals of approximations to the function. For proper Riemann integrals, a standard theorem states that if

7194: 2921: 1223: 6881:

The Riemann integral is only defined on bounded intervals, and it does not extend well to unbounded intervals. The simplest possible extension is to define such an integral as a

5124:

Again, alone this restriction does not impose a problem, but the reasoning required to see this fact is more difficult than in the case of left-hand and right-hand Riemann sums.

4144: 5372: 7817:

in the definition of a Riemann sum by something else; roughly speaking, this gives the interval of integration a different notion of length. This is the approach taken by the

6766: 5158: 4821: 4243: 2229:{\displaystyle {\begin{aligned}L(f,P)&=\sum _{i=0}^{n-1}\inf _{t\in }f(t)(x_{i+1}-x_{i}),\\U(f,P)&=\sum _{i=0}^{n-1}\sup _{t\in }f(t)(x_{i+1}-x_{i}).\end{aligned}}} 31:

A sequence of Riemann sums over a regular partition of an interval. The number on top is the total area of the rectangles, which converges to the integral of the function.

7458: 4779:

Since we started from an arbitrary partition and ended up as close as we wanted to either zero or one, it is false to say that we are eventually trapped near some number

6263:

of the interval, and since the interval is compact there is a finite subcover of them. This subcover is a finite collection of open intervals, which are subintervals of

1289: 6529:(meaning more difficult to satisfy) condition than Lebesgue-integrability. The converse does not hold; not all Lebesgue-integrable functions are Riemann integrable. 4612: 6798: 3803: 3133:{\displaystyle \delta <\min \left\{{\frac {\varepsilon }{2r(m-1)}},\left(y_{1}-y_{0}\right),\left(y_{2}-y_{1}\right),\cdots ,\left(y_{m}-y_{m-1}\right)\right\}} 1068: 4979:. Alone this restriction does not impose a problem: we can refine any partition in a way that makes it a left-hand or right-hand sum by subdividing it at each 4772:. The second way is to always choose an irrational point, so that the Riemann sum is as small as possible. This will make the value of the Riemann sum at most 5764:

less a finite number of points (as a finite number of points can always be covered by a finite collection of intervals with arbitrarily small total length).

3705:{\displaystyle f\left(t_{i}\right)\left(x_{i+1}-x_{i}\right)=f\left(t_{i}\right)\left(x_{i+1}-y_{j+1}\right)+f\left(t_{i}\right)\left(y_{j+1}-x_{i}\right).} 1755: 5329:{\displaystyle \int _{0}^{{\sqrt {2}}-1}I_{\mathbb {Q} }(x)\,dx+\int _{{\sqrt {2}}-1}^{1}I_{\mathbb {Q} }(x)\,dx=\int _{0}^{1}I_{\mathbb {Q} }(x)\,dx.} 3328: 7877:

The Riemann integral is unsuitable for many theoretical purposes. Some of the technical deficiencies in Riemann integration can be remedied with the

4929:. This is because the Darboux integral is technically simpler and because a function is Riemann-integrable if and only if it is Darboux-integrable. 1688:. That is, a tagged partition breaks up some of the sub-intervals and adds sample points where necessary, "refining" the accuracy of the partition. 8960: 1406: 7344: 7025: 1025:

in 1854, but not published in a journal until 1868. For many functions and practical applications, the Riemann integral can be evaluated by the

8894: 7598: 3305:. In this case, it is possible that one of the is not contained in any . Instead, it may stretch across two of the intervals determined by 4640: 238: 39:

The partition does not need to be regular, as shown here. The approximation works as long as the width of each subdivision tends to zero.

4761:

We still have to choose tags for the other subintervals. We will choose them in two different ways. The first way is to always choose a

8714: 4061:{\displaystyle \left|f\left(t_{i}\right)-f\left(t_{i}^{*}\right)\right|\left(x_{i+1}-y_{j+1}\right)<{\frac {\varepsilon }{2(m-1)}}.} 8749: 3427:(We may assume that all the inequalities are strict because otherwise we are in the previous case by our assumption on the length of 5795:, possibly up to a finite number of points (which may fall on interval edges). Thus these intervals have a total length of at least 8597: 8502: 3731: 3206: 8754: 5000: 8185: 8103:

updated April 2010, William F. Trench, 3.5 "A More Advanced Look at the Existence of the Proper Riemann Integral", pp. 171–177

7139: 3441:

To handle this case, we will estimate the difference between the Riemann sum and the Darboux sum by subdividing the partition

2936:

is the zero function, which is clearly both Darboux and Riemann integrable with integral zero. Therefore, we will assume that

6728: 6718:{\displaystyle \int _{a}^{b}f\,dx=\int _{a}^{b}{\lim _{n\to \infty }{f_{n}}\,dx}=\lim _{n\to \infty }\int _{a}^{b}f_{n}\,dx.} 2847: 1174: 8507: 8482: 504: 479: 2250:

is discontinuous on a subinterval, there may not be a tag that achieves the infimum or supremum on that subinterval.) The

8580: 8332: 2809:. First, one shows that the second definition is equivalent to the definition of the Darboux integral; for this see the 8869: 8859: 8844: 8764: 8412: 8124: 4160: 980: 543: 8085:

Basic real analysis, by Houshang H. Sohrab, section 7.3, Sets of Measure Zero and Lebesgue’s Integrability Condition,

7831: 61: 8904: 8575: 8497: 8286: 8255: 8015: 7977: 6882: 6534: 6005:– a finite collections of open intervals in with arbitrarily small total length that together contain all points in 5598: 4829: 1695:

by saying that one tagged partition is greater than or equal to another if the former is a refinement of the latter.

499: 217: 8919: 8512: 8477: 5557:{\displaystyle \int _{a}^{b}(\alpha f(x)+\beta g(x))\,dx=\alpha \int _{a}^{b}f(x)\,dx+\beta \int _{a}^{b}g(x)\,dx.} 1516:

of an interval is a partition together with a choice of a sample point within each sub-interval: that is, numbers

484: 7019:

This definition carries with it some subtleties, such as the fact that it is not always equivalent to compute the

8492: 7010:{\displaystyle \int _{-\infty }^{\infty }f(x)\,dx=\lim _{a\to -\infty \atop b\to \infty }\int _{a}^{b}f(x)\,dx.} 2751:. Since this is true no matter how close we demand the sums be trapped, we say that the Riemann sums converge to 1026: 820: 494: 469: 151: 2274:). The Riemann sum can be made as close as desired to the Riemann integral by making the partition fine enough. 8914: 8909: 8884: 8739: 8707: 8427: 8387: 7878: 7818: 7790: 7666:

converges uniformly to the zero function, and clearly the integral of the zero function is zero. Consequently,

7331:{\displaystyle {\begin{aligned}\int _{-a}^{2a}f(x)\,dx&=a,\\\int _{-2a}^{a}f(x)\,dx&=-a.\end{aligned}}} 4986:. In more formal language, the set of all left-hand Riemann sums and the set of all right-hand Riemann sums is 8487: 8303: 7782:

defined on a bounded interval is Riemann-integrable if and only if it is bounded and the set of points where

4936:

One popular restriction is the use of "left-hand" and "right-hand" Riemann sums. In a left-hand Riemann sum,

2254:, which is similar to the Riemann integral but based on Darboux sums, is equivalent to the Riemann integral. 602: 549: 430: 7761:{\displaystyle \int _{-\infty }^{\infty }f\,dx\neq \lim _{n\to \infty }\int _{-\infty }^{\infty }f_{n}\,dx.} 5752:. In particular this is also true for every such finite collection of intervals. This remains true also for 4538: 8849: 8734: 8098: 4342:. Then, by carefully choosing the new tags, we can make the value of the Riemann sum turn out to be within 256: 228: 8666: 339: 8874: 8651: 8450: 8298: 853: 461: 299: 271: 7952:(Proceedings of the Royal Philosophical Society at Göttingen), vol. 13, pages 87-132. (Available online 4765:, so that the Riemann sum is as large as possible. This will make the value of the Riemann sum at least 4103: 1231:. By taking better and better approximations, we can say that "in the limit" we get exactly the area of 8899: 8879: 8620: 8587: 8455: 5910:

as well. This is because every neighborhood of the limit point is also a neighborhood of some point in

5345: 3715: 724: 688: 465: 344: 233: 223: 8293: 6742: 5134: 4797: 4219: 1022: 8965: 8938: 8700: 5651: 2733:{\displaystyle \left|\left(\sum _{i=0}^{n-1}f(t_{i})(x_{i+1}-x_{i})\right)-s\right|<\varepsilon .} 2472:{\displaystyle \left|\left(\sum _{i=0}^{n-1}f(t_{i})(x_{i+1}-x_{i})\right)-s\right|<\varepsilon .} 488: 8112: 2770:

works in the second definition. To show that the first definition implies the second, start with an

324: 8889: 8779: 8744: 8465: 6118: 6055:

of the union of these intervals is itself a union of a finite number of intervals, which we denote

5987: 5768: 2305: 1283: 623: 183: 8799: 8325: 7953: 6739:

It is easy to extend the Riemann integral to functions with values in the Euclidean vector space

6052: 2813:

article. Now we will show that a Darboux integrable function satisfies the first definition. Fix

1034: 937: 729: 618: 6110: 4993:

Another popular restriction is the use of regular subdivisions of an interval. For example, the

8814: 7825: 7020: 5617:(of characterization of the Riemann integrable functions). It has been proven independently by 2266:

of a function as the partitions get finer. If the limit exists then the function is said to be

1018: 1014: 973: 902: 863: 747: 683: 607: 8086: 8043:

Brown, A. B. (September 1936). "A Proof of the Lebesgue Condition for Riemann Integrability".

3325:

is assumed to be smaller than the length of any one interval.) In symbols, it may happen that

8839: 8625: 8527: 6282:(except for those that include an edge point, for which we only take their intersection with 4987: 1030: 947: 613: 384: 329: 290: 196: 8005: 1227:

The basic idea of the Riemann integral is to use very simple approximations for the area of

8472: 8362: 7925: 6475:

In total, the difference between the upper and lower sums of the partition is smaller than

6177: 5811: 5706: 5632:

The integrability condition can be proven in various ways, one of which is sketched below.

1900: 1062: 952: 932: 858: 527: 446: 420: 334: 5566:

Because the Riemann integral of a function is a number, this makes the Riemann integral a

1061:

and above the interval . See the figure on the top right. This region can be expressed in

8: 8864: 8774: 8759: 8607: 8522: 8517: 8407: 8223: 7448: 6552: 5677:

is discontinuous has a positive oscillation and vice versa, the set of points in , where

5591: 927: 897: 887: 774: 628: 425: 281: 164: 159: 6204:} with arbitrarily small total length, we choose them to have total length smaller than 8784: 8630: 8567: 8460: 8432: 8397: 8318: 8205: 8166: 8068: 7886: 6503: 5129: 4213: 892: 795: 779: 719: 714: 709: 673: 554: 473: 379: 374: 178: 173: 8854: 8794: 8679: 8656: 8592: 8562: 8554: 8532: 8402: 8251: 8158: 8060: 8011: 8001: 7983: 7973: 7882: 7866: 7775: 6886: 6522: 6518: 5949: 5594: 4788: 4784: 2833:

such that the lower and upper Darboux sums with respect to this partition are within

2756: 966: 800: 578: 456: 409: 266: 261: 7789:

An integral which is in fact a direct generalization of the Riemann integral is the

7562:{\displaystyle \lim _{n\to \infty }\int _{a}^{b}f_{n}(x)\,dx=\int _{a}^{b}f(x)\,dx.} 5342:

As defined above, the Riemann integral avoids this problem by refusing to integrate

8769: 8723: 8674: 8537: 8422: 8382: 8377: 8372: 8367: 8197: 8150: 8052: 7898: 6491: 5968: 5734: 5702: 5644: 5606: 5583: 4926: 3203:

To see this, choose an interval . If this interval is contained within some , then

2810: 2806: 2251: 1006: 810: 704: 678: 539: 451: 2778:

that satisfies the condition. Choose any tagged partition whose mesh is less than

8834: 8809: 8661: 8544: 8417: 7571:

On non-compact intervals such as the real line, this is false. For example, take

5618: 4884:, must be zero on a dense set, so as in the previous example, any Riemann sum of 1403:

of a partition is defined to be the length of the longest sub-interval, that is,

1382:{\displaystyle a=x_{0}<x_{1}<x_{2}<\dots <x_{i}<\dots <x_{n}=b} 942: 815: 769: 764: 651: 564: 509: 19: 8615: 5971:. Therefore, there is a countable collections of open intervals in which is an 8824: 8819: 7920: 7903: 7890: 7435: 6511: 6507: 5622: 5374:

The Lebesgue integral is defined in such a way that all these integrals are 0.

4846: 4762: 2805:

To show that the second definition implies the first, it is easiest to use the

1549:. The mesh of a tagged partition is the same as that of an ordinary partition. 825: 633: 400: 6865:{\displaystyle \int \mathbf {f} =\left(\int f_{1},\,\dots ,\int f_{n}\right).} 3887:{\displaystyle x_{i+1}-y_{j+1}<\delta <{\frac {\varepsilon }{2r(m-1)}},} 8954: 8646: 8162: 8064: 7098: 6487: 5873: 5587: 2755:. These definitions are actually a special case of a more general concept, a 998: 805: 569: 319: 276: 35: 7987: 2762:

As we stated earlier, these two definitions are equivalent. In other words,

1158:{\displaystyle S=\left\{(x,y)\,:\,a\leq x\leq b\,,\,0<y<f(x)\right\}.} 8804: 8392: 7796:

Another way of generalizing the Riemann integral is to replace the factors

6875: 6325:, including the edge points of the intervals themselves, as our partition. 5626: 5602: 5571: 4146:

be the function which takes the value 1 at every point. Any Riemann sum of

1692: 559: 304: 27: 5161:

split the integral into two pieces. The following equation ought to hold:

4328:

at those points. But if we cut the partition into tiny pieces around each

8829: 8262: 7967: 7950:

Abhandlungen der Königlichen Gesellschaft der Wissenschaften zu Göttingen

5567: 4787:. In the Lebesgue sense its integral is zero, since the function is zero 4077:

times, the distance between the Riemann sum and a Darboux sum is at most

3793:

so this term is bounded by the corresponding term in the Darboux sum for

2263: 1709: 1050: 994: 922: 6117:, from which Riemann integrability follows. To this end, we construct a 1171:. Once we have measured it, we will denote the area in the usual way by 8209: 8170: 8138: 8072: 6260: 6250:, so each point in it has a neighborhood with oscillation smaller than 5972: 4791:. But this is a fact that is beyond the reach of the Riemann integral. 668: 592: 314: 309: 213: 1899:. These are similar to Riemann sums, but the tags are replaced by the 7948:(qualification to become an instructor). It was published in 1868 in 5730: 2790:, and any refinement of this partition will also have mesh less than 597: 587: 8201: 8154: 8056: 6471:

to the difference between the upper and lower sums of the partition.

6401:

to the difference between the upper and lower sums of the partition.

5771:, consider the set of intervals whose interiors include points from 1850:{\displaystyle \sum _{i=0}^{n-1}f(t_{i})\left(x_{i+1}-x_{i}\right).} 8692: 8341: 8115:, John Armstrong, December 15, 2009, The Unapologetic Mathematician 6002: 5629:, but makes use of neither Lebesgue's general measure or integral. 1010: 663: 405: 362: 51: 8127:, John Armstrong, December 9, 2009, The Unapologetic Mathematician 6506:

of a bounded set is Riemann-integrable if and only if the set is

5986:, such that the sum over all their lengths is arbitrarily small. 5725:

does not have a zero measure. Thus there is some positive number

7872: 4364:. If we confine each of them to an interval of length less than 3418:{\displaystyle y_{j}<x_{i}<y_{j+1}<x_{i+1}<y_{j+2}.} 8281: 7885:, though the latter does not have a satisfactory treatment of 6332:

Intervals of the latter kind (themselves subintervals of some

4528:. If one of these leaves the interval , then we leave it out. 1892:. The Riemann sum is the (signed) area of all the rectangles. 6772:. The integral is defined component-wise; in other words, if 5387:

The Riemann integral is a linear transformation; that is, if

4783:, so this function is not Riemann integrable. However, it is 4150:

on will have the value 1, therefore the Riemann integral of

8310: 1489:{\displaystyle \max \left(x_{i+1}-x_{i}\right),\quad i\in .} 7915: 7407:{\displaystyle \lim _{a\to \infty }\int _{-a}^{a}f(x)\,dx,} 7088:{\displaystyle \lim _{a\to \infty }\int _{-a}^{a}f(x)\,dx.} 6878:, this allows the integration of complex valued functions. 4324:

have already been chosen, and we can't change the value of

2794:, so the Riemann sum of the refinement will also be within 2262:

Loosely speaking, the Riemann integral is the limit of the

1057:

be the region of the plane under the graph of the function

7774:

A better route is to abandon the Riemann integral for the

7972:. Boca Raton, Fla.: Chapman & Hall/CRC. p. 173. 1707:

be a real-valued function defined on the interval . The

6328:

Thus the partition divides to two kinds of intervals:

2743:

Both of these mean that eventually, the Riemann sum of

7646:{\displaystyle \int _{-\infty }^{\infty }f_{n}\,dx=1.} 6302:. We take the edge points of the subintervals for all 5669:

be the set of points in with oscillation of at least

4084:. Therefore, the distance between the Riemann sum and 8248:

Integral, Measure, and Derivative: A Unified Approach

7834: 7672: 7601: 7461: 7347: 7206: 7142: 7028: 6895: 6801: 6745: 6580: 6364:. Since the total length of these is not larger than 5409: 5348: 5167: 5137: 5003: 4997:

th regular subdivision of consists of the intervals

4800: 4643: 4541: 4403:. This makes the total sum at least zero and at most 4349:

Our first step is to cut up the partition. There are

4222: 4163: 4106: 3919: 3806: 3734: 3506: 3331: 3209: 2960: 2850: 2613: 2352: 1913: 1758: 1409: 1292: 1177: 1071: 64: 7944:

to the University of Göttingen in 1854 as Riemann's

6874:

In particular, since the complex numbers are a real

6514:

as the integral with respect to the Jordan measure.

5783:. These interiors consist of a finite open cover of 4925:

It is popular to define the Riemann integral as the

4900:

exists, then it must equal the Lebesgue integral of

4752:{\displaystyle \left,\quad {\text{and}}\quad \left.} 2747:

with respect to any partition gets trapped close to

1691:

We can turn the set of all tagged partitions into a

8250:, Richard A. Silverman, trans. Dover Publications. 5846:We now prove the converse direction using the sets 23:

The integral as the area of a region under a curve.

7857: 7760: 7645: 7561: 7406: 7330: 7188: 7087: 7009: 6864: 6760: 6717: 5556: 5366: 5328: 5152: 5114: 4815: 4751: 4606: 4237: 4204: 4138: 4060: 3886: 3785: 3704: 3417: 3279:are respectively, the infimum and the supremum of 3257: 3132: 2915: 2732: 2471: 2228: 1849: 1582:are both partitions of the interval . We say that 1488: 1381: 1249:can take negative values, the integral equals the 1217: 1157: 136: 4858:is not Riemann integrable. Moreover, no function 4535:will be the tag corresponding to the subinterval 4360:, and we want their total effect to be less than 4205:{\displaystyle I_{\mathbb {Q} }:\to \mathbb {R} } 8952: 7858:{\displaystyle \mathbb {R} ^{n}\to \mathbb {R} } 7705: 7463: 7349: 7030: 6937: 6665: 6626: 2967: 2861: 2125: 1971: 1410: 137:{\displaystyle \int _{a}^{b}f'(t)\,dt=f(b)-f(a)} 3153:to be less than one. Choose a tagged partition 5818:in each of these intervals differ by at least 4493:Now we add two cuts to the partition for each 3192:. We must show that the Riemann sum is within 8708: 8326: 8224:"An Open Letter to Authors of Calculus Books" 7873:Comparison with other theories of integration 5654:definition of continuity: For every positive 3786:{\displaystyle m_{j}\leq f(t_{i})\leq M_{j},} 2766:works in the first definition if and only if 974: 8750:Grothendieck–Hirzebruch–Riemann–Roch theorem 8010:. American Mathematical Society. p. 1. 7786:is discontinuous has Lebesgue measure zero. 5681:is discontinuous is equal to the union over 4472:smaller. Since there are only finitely many 3258:{\displaystyle m_{j}\leq f(t_{i})\leq M_{j}} 1286:is a finite sequence of numbers of the form 1277: 7828:, the Riemann integrals for functions from 5590:is Riemann integrable if and only if it is 1167:We are interested in measuring the area of 1053:-valued function on the interval , and let 1009:, was the first rigorous definition of the 8715: 8701: 8333: 8319: 8246:Shilov, G. E., and Gurevich, B. L., 1978. 8183: 6510:. The Riemann integral can be interpreted 6444:. Thus together they contribute less than 5839:. Since this is true for every partition, 5709:of the measure there is at least one such 5115:{\displaystyle \left,\left,\ldots ,\left.} 3500:in the Riemann sum splits into two terms: 3321:. (It cannot meet three intervals because 1021:. It was presented to the faculty at the 981: 967: 8895:Riemann–Roch theorem for smooth manifolds 8024: 7851: 7837: 7748: 7694: 7630: 7549: 7512: 7394: 7301: 7244: 7173: 7075: 6997: 6926: 6834: 6748: 6705: 6653: 6599: 5544: 5504: 5464: 5355: 5316: 5301: 5270: 5255: 5214: 5199: 5144: 4807: 4229: 4198: 4170: 4132: 3295:, so the proof is finished in that case. 1205: 1122: 1118: 1102: 1098: 97: 8598:Common integrals in quantum field theory 7189:{\displaystyle \int _{-a}^{a}f(x)\,dx=0} 6421:}. These have total length smaller than 5899:that is converging in , its limit is in 34: 26: 18: 8961:Definitions of mathematical integration 8508:Differentiation under the integral sign 8261: 8186:"Taking limits under the integral sign" 8136: 8030: 6486:In particular, any set that is at most 3896:It follows that, for some (indeed any) 3800:. To bound the other term, notice that 2916:{\displaystyle r=2\sup _{x\in }|f(x)|.} 505:Differentiating under the integral sign 8953: 8125:Jordan Content Integrability Condition 8000: 7965: 6537:of the discontinuities of a function. 6525:. That is, Riemann-integrability is a 5650:One direction can be proven using the 4216:of the rational numbers in ; that is, 1218:{\displaystyle \int _{a}^{b}f(x)\,dx.} 8696: 8314: 8042: 7414:then the integral of the translation 6729:Lebesgue monotone convergence theorem 4990:in the set of all tagged partitions. 1717:with respect to the tagged partition 16:Basic integral in elementary calculus 8722: 6559:, then Riemann integrability of all 6259:. These neighborhoods consist of an 4381:to the Riemann sum will be at least 4335:, we can minimize the effect of the 2278:we say that the Riemann integral of 6434:oscillates on them by no more than 6374:, they together contribute at most 6184:on . Since we may choose intervals 5929:on it. Hence the limit point is in 5825:. Thus the upper and lower sums of 4956:, and in a right-hand Riemann sum, 4920: 4841:be its indicator function. Because 2537:such that for any tagged partition 2257: 1261:-axis: that is, the area above the 13: 8860:Riemannian connection on a surface 8765:Measurable Riemann mapping theorem 8184:Cunningham, Frederick Jr. (1967). 7733: 7728: 7715: 7686: 7681: 7615: 7610: 7473: 7359: 7040: 6963: 6952: 6941: 6909: 6904: 6734: 6675: 6636: 4892:of 0 for any positive number 4139:{\displaystyle f:\to \mathbb {R} } 2502:, there exists a tagged partition 2491:if the following condition holds: 2286:if the following condition holds: 46:Part of a series of articles about 14: 8977: 8274: 8143:The American Mathematical Monthly 8045:The American Mathematical Monthly 6570:implies Riemann integrability of 6236:} has an empty intersection with 5574:of Riemann-integrable functions. 5367:{\displaystyle I_{\mathbb {Q} }.} 4896:. But if the Riemann integral of 4888:has a refinement which is within 4623:is directly on top of one of the 4436:smaller. If it happens that some 1895:Closely related concepts are the 8933: 8932: 8280: 7591:on and zero elsewhere. For all 7447:is a sequence of functions that 6806: 6761:{\displaystyle \mathbb {R} ^{n}} 5625:in 1907, and uses the notion of 5577: 5153:{\displaystyle I_{\mathbb {Q} }} 4816:{\displaystyle I_{\mathbb {Q} }} 4421:. If it happens that two of the 4374:, then the contribution of each 4238:{\displaystyle I_{\mathbb {Q} }} 8845:Riemann's differential equation 8755:Hirzebruch–Riemann–Roch theorem 8216: 8177: 8130: 5925:has an oscillation of at least 5748:has a total length of at least 5701:If this set does not have zero 5643:The proof is easiest using the 5395:are Riemann-integrable on and 4794:There are even worse examples. 4699: 4693: 4637:be the tag for both intervals: 4411:be a positive number less than 1455: 1265:-axis minus the area below the 1027:fundamental theorem of calculus 8870:Riemann–Hilbert correspondence 8740:Generalized Riemann hypothesis 8118: 8106: 8091: 8079: 8036: 8007:Measure Theory and Integration 7994: 7959: 7937: 7881:, and most disappear with the 7847: 7712: 7546: 7540: 7509: 7503: 7470: 7391: 7385: 7356: 7298: 7292: 7241: 7235: 7170: 7164: 7072: 7066: 7037: 6994: 6988: 6960: 6946: 6923: 6917: 6672: 6633: 6113:whose difference is less than 5888:For every series of points in 5541: 5535: 5501: 5495: 5461: 5458: 5452: 5440: 5434: 5425: 5313: 5307: 5267: 5261: 5211: 5205: 4194: 4191: 4179: 4128: 4125: 4113: 4049: 4037: 3875: 3863: 3764: 3751: 3298:Therefore, we may assume that 3239: 3226: 2999: 2987: 2906: 2902: 2896: 2889: 2883: 2871: 2702: 2670: 2667: 2654: 2441: 2409: 2406: 2393: 2216: 2184: 2181: 2175: 2167: 2135: 2087: 2075: 2062: 2030: 2027: 2021: 2013: 1981: 1933: 1921: 1802: 1789: 1698: 1480: 1462: 1202: 1196: 1144: 1138: 1095: 1083: 131: 125: 116: 110: 94: 88: 1: 8905:Riemann–Siegel theta function 8340: 8240: 8099:Introduction to Real Analysis 7969:Real Analysis and Foundations 7897:In educational settings, the 7894:integral when it does exist. 6517:If a real-valued function is 5733:collection of open intervals 5377: 4500:. One of the cuts will be at 2844:of the Darboux integral. Let 1272: 431:Integral of inverse functions 8920:Riemann–von Mangoldt formula 6016:. We denote these intervals 5803:has oscillation of at least 5634: 5382: 4284:be a tagged partition (each 2782:. Its Riemann sum is within 1897:lower and upper Darboux sums 1552:Suppose that two partitions 7: 8413:Lebesgue–Stieltjes integral 8299:Encyclopedia of Mathematics 7909: 6102:We now show that for every 5843:is not Riemann integrable. 4917:is not Riemann integrable. 4514:, and the other will be at 4095: 4070:Since this happens at most 3431:.) This can happen at most 2246:on each subinterval. 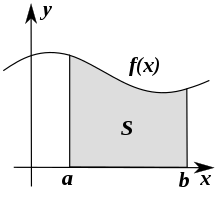(When 1040: 854:Calculus on Euclidean space 272:Logarithmic differentiation 10: 8982: 8915:Riemann–Stieltjes integral 8910:Riemann–Silberstein vector 8885:Riemann–Liouville integral 8428:Riemann–Stieltjes integral 8388:Henstock–Kurzweil integral 8139:"On Riemann Integrability" 7966:Krantz, Steven G. (2005). 7879:Riemann–Stieltjes integral 7819:Riemann–Stieltjes integral 7791:Henstock–Kurzweil integral 7097:For example, consider the 5694:} for all natural numbers 5673:. Since every point where 3716:without loss of generality 1627:, there exists an integer 8928: 8850:Riemann's minimal surface 8730: 8667:Proof that 22/7 exceeds π 8639: 8606: 8553: 8441: 8348: 5857:defined above. For every 4830:Smith–Volterra–Cantor set 2817:, and choose a partition 2572:which is a refinement of 1278:Partitions of an interval 588:Summand limit (term test) 8875:Riemann–Hilbert problems 8780:Riemann curvature tensor 8745:Grand Riemann hypothesis 8735:Cauchy–Riemann equations 7931: 7455:on a compact set , then 6885:, in other words, as an 6555:sequence on with limit 6355:oscillates by less than 5799:. Since in these points 5613:Lebesgue-Vitali theorem 3288:. This is the case when 2342:whose mesh is less than 1284:partition of an interval 267:Implicit differentiation 257:Differentiation notation 184:Inverse function theorem 8800:Riemann mapping theorem 8652:Euler–Maclaurin formula 8137:Metzler, R. C. (1971). 5876:, as it is bounded (by 5599:points of discontinuity 4871:is Riemann integrable: 4486:, we can always choose 4346:of either zero or one. 3188:with mesh smaller than 1035:Monte Carlo integration 1023:University of Göttingen 730:Helmholtz decomposition 8900:Riemann–Siegel formula 8880:Riemann–Lebesgue lemma 8815:Riemann series theorem 8621:Russo–Vallois integral 8588:Bose–Einstein integral 8503:Parametric derivatives 7859: 7826:multivariable calculus 7762: 7647: 7563: 7408: 7332: 7196:always, regardless of 7190: 7089: 7021:Cauchy principal value 7011: 6866: 6762: 6719: 6216:Each of the intervals 5558: 5368: 5330: 5154: 5116: 4817: 4753: 4608: 4607:{\displaystyle \left.} 4432:of each other, choose 4239: 4206: 4140: 4062: 3888: 3787: 3706: 3419: 3259: 3134: 2917: 2741: 2734: 2650: 2480: 2473: 2389: 2270:(or more specifically 2230: 2123: 1969: 1907:on each sub-interval: 1851: 1785: 1490: 1395:of the partition. The 1383: 1219: 1159: 864:Limit of distributions 684:Directional derivative 340:Faà di Bruno's formula 138: 40: 32: 24: 8840:Riemann zeta function 8626:Stratonovich integral 8572:Fermi–Dirac integral 8528:Numerical integration 8267:Mathematical Analysis 7860: 7763: 7648: 7564: 7409: 7333: 7191: 7090: 7012: 6867: 6763: 6720: 6512:measure-theoretically 6351:). In each of these, 5559: 5369: 5331: 5155: 5117: 4818: 4754: 4609: 4240: 4207: 4141: 4063: 3889: 3788: 3707: 3420: 3260: 3135: 2918: 2735: 2624: 2493: 2474: 2363: 2288: 2231: 2097: 1943: 1852: 1759: 1491: 1384: 1253:between the graph of 1220: 1160: 1033:, or simulated using 1031:numerical integration 948:Mathematical analysis 859:Generalized functions 544:arithmetico-geometric 385:Leibniz integral rule 139: 38: 30: 22: 8890:Riemann–Roch theorem 8608:Stochastic integrals 8289:at Wikimedia Commons 8190:Mathematics Magazine 8113:Lebesgue’s Condition 7946:Habilitationsschrift 7926:Lebesgue integration 7832: 7670: 7599: 7459: 7345: 7204: 7140: 7026: 6893: 6799: 6743: 6578: 6553:uniformly convergent 6178:infimum and supremum 6111:upper and lower sums 6001:, there is a finite 5812:infimum and supremum 5707:countable additivity 5407: 5403:are constants, then 5346: 5165: 5135: 5001: 4798: 4641: 4539: 4490:sufficiently small. 4220: 4161: 4104: 3917: 3804: 3732: 3504: 3329: 3207: 2958: 2848: 2611: 2350: 1911: 1901:infimum and supremum 1756: 1616:if for each integer 1407: 1290: 1175: 1069: 1063:set-builder notation 953:Nonstandard analysis 421:Lebesgue integration 291:Rules and identities 62: 8865:Riemannian geometry 8775:Riemann Xi function 8760:Local zeta function 8518:Contour integration 8408:Kolmogorov integral 7737: 7690: 7619: 7536: 7492: 7381: 7288: 7231: 7160: 7062: 6984: 6913: 6694: 6623: 6595: 6523:Lebesgue integrable 6044:, for some natural 5829:differ by at least 5531: 5491: 5424: 5294: 5248: 5192: 4785:Lebesgue integrable 3970: 1192: 1029:or approximated by 624:Cauchy condensation 426:Contour integration 152:Fundamental theorem 79: 8785:Riemann hypothesis 8631:Skorokhod integral 8568:Dirichlet integral 8555:Improper integrals 8498:Reduction formulas 8433:Regulated integral 8398:Hellinger integral 8294:"Riemann integral" 8033:, pp. 169–172 8002:Taylor, Michael E. 7887:improper integrals 7867:multiple integrals 7855: 7758: 7720: 7719: 7673: 7643: 7602: 7559: 7522: 7478: 7477: 7449:converge uniformly 7404: 7364: 7363: 7328: 7326: 7268: 7211: 7186: 7143: 7085: 7045: 7044: 7007: 6970: 6969: 6896: 6862: 6758: 6715: 6680: 6679: 6640: 6609: 6581: 6504:indicator function 5944:Now, suppose that 5605:, in the sense of 5554: 5517: 5477: 5410: 5364: 5326: 5280: 5224: 5168: 5150: 5130:indicator function 5112: 4813: 4749: 4604: 4235: 4214:indicator function 4202: 4136: 4058: 3956: 3884: 3783: 3702: 3415: 3255: 3130: 2913: 2887: 2730: 2487:exists and equals 2469: 2304:such that for any 2282:exists and equals 2272:Riemann-integrable 2226: 2224: 2171: 2017: 1903:(respectively) of 1847: 1486: 1391:Each is called a 1379: 1215: 1178: 1155: 1049:be a non-negative 796:Partial derivative 725:generalized Stokes 619:Alternating series 500:Reduction formulae 489:Heaviside's method 470:tangent half-angle 457:Cylindrical shells 380:Integral transform 375:Lists of integrals 179:Mean value theorem 134: 65: 41: 33: 25: 8948: 8947: 8855:Riemannian circle 8795:Riemann invariant 8690: 8689: 8593:Frullani integral 8563:Gaussian integral 8513:Laplace transform 8488:Inverse functions 8478:Partial fractions 8403:Khinchin integral 8363:Lebesgue integral 8285:Media related to 8149:(10): 1129–1131. 7883:Lebesgue integral 7776:Lebesgue integral 7704: 7462: 7348: 7029: 6967: 6936: 6887:improper integral 6664: 6625: 6508:Jordan measurable 6484: 6483: 6084:and possibly for 5952:. Then for every 5950:almost everywhere 5595:almost everywhere 5568:linear functional 5234: 5183: 5096: 5059: 5046: 5023: 4847:Jordan measurable 4789:almost everywhere 4739: 4697: 4670: 4594: 4568: 4053: 3879: 3149:, then we choose 3003: 2950:, then we choose 2860: 2124: 1970: 1235:under the curve. 993:In the branch of 991: 990: 871: 870: 833: 832: 801:Multiple integral 737: 736: 641: 640: 608:Direct comparison 579:Convergence tests 517: 516: 485:Partial fractions 352: 351: 262:Second derivative 8973: 8966:Bernhard Riemann 8936: 8935: 8790:Riemann integral 8770:Riemann (crater) 8724:Bernhard Riemann 8717: 8710: 8703: 8694: 8693: 8538:Trapezoidal rule 8523:Laplace's method 8423:Pfeffer integral 8383:Darboux integral 8378:Daniell integral 8373:Bochner integral 8368:Burkill integral 8358:Riemann integral 8335: 8328: 8321: 8312: 8311: 8307: 8287:Riemann integral 8284: 8270: 8269:, Addison-Wesley 8235: 8234: 8232: 8230: 8220: 8214: 8213: 8181: 8175: 8174: 8134: 8128: 8122: 8116: 8110: 8104: 8095: 8089: 8083: 8077: 8076: 8040: 8034: 8028: 8022: 8021: 7998: 7992: 7991: 7963: 7957: 7941: 7899:Darboux integral 7864: 7862: 7861: 7856: 7854: 7846: 7845: 7840: 7816: 7785: 7781: 7767: 7765: 7764: 7759: 7747: 7746: 7736: 7731: 7718: 7689: 7684: 7665: 7652: 7650: 7649: 7644: 7629: 7628: 7618: 7613: 7594: 7590: 7584: 7568: 7566: 7565: 7560: 7535: 7530: 7502: 7501: 7491: 7486: 7476: 7454: 7446: 7429: 7424: 7413: 7411: 7410: 7405: 7380: 7375: 7362: 7337: 7335: 7334: 7329: 7327: 7287: 7282: 7230: 7222: 7199: 7195: 7193: 7192: 7187: 7159: 7154: 7135: 7128: 7121: 7114: 7094: 7092: 7091: 7086: 7061: 7056: 7043: 7016: 7014: 7013: 7008: 6983: 6978: 6968: 6966: 6955: 6912: 6907: 6871: 6869: 6868: 6863: 6858: 6854: 6853: 6852: 6830: 6829: 6809: 6794: 6771: 6767: 6765: 6764: 6759: 6757: 6756: 6751: 6724: 6722: 6721: 6716: 6704: 6703: 6693: 6688: 6678: 6660: 6652: 6651: 6650: 6639: 6622: 6617: 6594: 6589: 6573: 6569: 6558: 6550: 6492:Lebesgue measure 6478: 6470: 6456: 6455: 6443: 6433: 6429: 6420: 6400: 6386: 6385: 6373: 6363: 6354: 6350: 6324: 6301: 6281: 6258: 6249: 6235: 6212: 6203: 6183: 6175: 6171: 6167: 6145: 6116: 6108: 6098: 6083: 6071: 6047: 6043: 6032: 6015: 5998: 5985: 5969:Lebesgue measure 5966: 5955: 5947: 5939: 5928: 5924: 5920: 5909: 5898: 5883: 5879: 5871: 5860: 5856: 5842: 5838: 5828: 5824: 5817: 5809: 5802: 5798: 5794: 5782: 5763: 5751: 5747: 5729:such that every 5728: 5724: 5712: 5703:Lebesgue measure 5697: 5693: 5680: 5676: 5672: 5668: 5657: 5645:Darboux integral 5635: 5615: 5614: 5609:). This is the 5607:Lebesgue measure 5597:(the set of its 5588:compact interval 5584:bounded function 5563: 5561: 5560: 5555: 5530: 5525: 5490: 5485: 5423: 5418: 5402: 5398: 5394: 5390: 5373: 5371: 5370: 5365: 5360: 5359: 5358: 5335: 5333: 5332: 5327: 5306: 5305: 5304: 5293: 5288: 5260: 5259: 5258: 5247: 5242: 5235: 5230: 5204: 5203: 5202: 5191: 5184: 5179: 5176: 5159: 5157: 5156: 5151: 5149: 5148: 5147: 5121: 5119: 5118: 5113: 5108: 5104: 5097: 5092: 5081: 5065: 5061: 5060: 5052: 5047: 5039: 5029: 5025: 5024: 5016: 4996: 4985: 4978: 4974: 4955: 4951: 4927:Darboux integral 4921:Similar concepts 4916: 4912: 4908: 4899: 4895: 4891: 4887: 4883: 4874: 4870: 4861: 4857: 4844: 4840: 4827: 4822: 4820: 4819: 4814: 4812: 4811: 4810: 4782: 4775: 4771: 4758: 4756: 4755: 4750: 4745: 4741: 4740: 4732: 4727: 4726: 4714: 4713: 4698: 4695: 4689: 4685: 4684: 4683: 4671: 4663: 4658: 4657: 4636: 4629: 4622: 4613: 4611: 4610: 4605: 4600: 4596: 4595: 4587: 4582: 4581: 4569: 4561: 4556: 4555: 4534: 4527: 4513: 4499: 4489: 4485: 4478: 4471: 4467: 4461:is not equal to 4460: 4453: 4446: 4442: 4435: 4431: 4427: 4420: 4410: 4406: 4402: 4391: 4380: 4373: 4363: 4359: 4352: 4345: 4341: 4334: 4327: 4323: 4316: 4309: 4297: 4290: 4283: 4264: 4244: 4242: 4241: 4236: 4234: 4233: 4232: 4211: 4209: 4208: 4203: 4201: 4175: 4174: 4173: 4153: 4149: 4145: 4143: 4142: 4137: 4135: 4091: 4088:is at most 4087: 4083: 4076: 4067: 4065: 4064: 4059: 4054: 4052: 4029: 4024: 4020: 4019: 4018: 4000: 3999: 3979: 3975: 3974: 3969: 3964: 3945: 3941: 3940: 3912: 3910: 3909: 3893: 3891: 3890: 3885: 3880: 3878: 3852: 3841: 3840: 3822: 3821: 3799: 3792: 3790: 3789: 3784: 3779: 3778: 3763: 3762: 3744: 3743: 3727: 3711: 3709: 3708: 3703: 3698: 3694: 3693: 3692: 3680: 3679: 3659: 3655: 3654: 3635: 3631: 3630: 3629: 3611: 3610: 3590: 3586: 3585: 3566: 3562: 3561: 3560: 3548: 3547: 3527: 3523: 3522: 3499: 3468: 3456: 3437: 3430: 3424: 3422: 3421: 3416: 3411: 3410: 3392: 3391: 3373: 3372: 3354: 3353: 3341: 3340: 3324: 3320: 3304: 3294: 3287: 3278: 3271: 3264: 3262: 3261: 3256: 3254: 3253: 3238: 3237: 3219: 3218: 3199: 3195: 3191: 3187: 3168: 3152: 3148: 3139: 3137: 3136: 3131: 3129: 3125: 3124: 3120: 3119: 3118: 3100: 3099: 3076: 3072: 3071: 3070: 3058: 3057: 3040: 3036: 3035: 3034: 3022: 3021: 3004: 3002: 2976: 2953: 2949: 2942: 2935: 2931: 2922: 2920: 2919: 2914: 2909: 2892: 2886: 2843: 2839: 2832: 2816: 2811:Darboux integral 2807:Darboux integral 2801: 2797: 2793: 2789: 2785: 2781: 2777: 2773: 2769: 2765: 2754: 2750: 2746: 2739: 2737: 2736: 2731: 2720: 2716: 2709: 2705: 2701: 2700: 2688: 2687: 2666: 2665: 2649: 2638: 2606: 2587: 2571: 2552: 2536: 2517: 2501: 2490: 2486: 2478: 2476: 2475: 2470: 2459: 2455: 2448: 2444: 2440: 2439: 2427: 2426: 2405: 2404: 2388: 2377: 2345: 2341: 2322: 2306:tagged partition 2303: 2296: 2285: 2281: 2258:Riemann integral 2252:Darboux integral 2249: 2245: 2241: 2235: 2233: 2232: 2227: 2225: 2215: 2214: 2202: 2201: 2170: 2166: 2165: 2147: 2146: 2122: 2111: 2061: 2060: 2048: 2047: 2016: 2012: 2011: 1993: 1992: 1968: 1957: 1906: 1891: 1872: 1856: 1854: 1853: 1848: 1843: 1839: 1838: 1837: 1825: 1824: 1801: 1800: 1784: 1773: 1751: 1732: 1716: 1706: 1687: 1680: 1676: 1660: 1637: 1626: 1619: 1615: 1596: 1581: 1566: 1548: 1544: 1534: 1515: 1500:tagged partition 1495: 1493: 1492: 1487: 1451: 1447: 1446: 1445: 1433: 1432: 1388: 1386: 1385: 1380: 1372: 1371: 1353: 1352: 1334: 1333: 1321: 1320: 1308: 1307: 1268: 1264: 1260: 1256: 1248: 1234: 1230: 1224: 1222: 1221: 1216: 1191: 1186: 1170: 1164: 1162: 1161: 1156: 1151: 1147: 1060: 1056: 1048: 1007:Bernhard Riemann 1003:Riemann integral 983: 976: 969: 917: 882: 848: 847: 844: 811:Surface integral 754: 753: 750: 658: 657: 654: 614:Limit comparison 534: 533: 530: 416:Riemann integral 369: 368: 365: 325:L'Hôpital's rule 282:Taylor's theorem 203: 202: 199: 143: 141: 140: 135: 87: 78: 73: 43: 42: 8981: 8980: 8976: 8975: 8974: 8972: 8971: 8970: 8951: 8950: 8949: 8944: 8924: 8835:Riemann surface 8810:Riemann problem 8726: 8721: 8691: 8686: 8662:Integration Bee 8635: 8602: 8549: 8545:Risch algorithm 8483:Euler's formula 8443: 8437: 8418:Pettis integral 8350: 8344: 8339: 8292: 8277: 8243: 8238: 8228: 8226: 8222: 8221: 8217: 8202:10.2307/2688673 8182: 8178: 8155:10.2307/2316325 8135: 8131: 8123: 8119: 8111: 8107: 8096: 8092: 8084: 8080: 8057:10.2307/2301737 8041: 8037: 8029: 8025: 8018: 7999: 7995: 7980: 7964: 7960: 7942: 7938: 7934: 7912: 7875: 7850: 7841: 7836: 7835: 7833: 7830: 7829: 7815: 7806: 7797: 7783: 7779: 7742: 7738: 7732: 7724: 7708: 7685: 7677: 7671: 7668: 7667: 7662: 7656: 7624: 7620: 7614: 7606: 7600: 7597: 7596: 7592: 7586: 7577: 7572: 7531: 7526: 7497: 7493: 7487: 7482: 7466: 7460: 7457: 7456: 7452: 7444: 7439: 7427: 7415: 7376: 7368: 7352: 7346: 7343: 7342: 7325: 7324: 7308: 7283: 7272: 7265: 7264: 7251: 7223: 7215: 7207: 7205: 7202: 7201: 7197: 7155: 7147: 7141: 7138: 7137: 7136:. By symmetry, 7130: 7123: 7116: 7101: 7057: 7049: 7033: 7027: 7024: 7023: 6979: 6974: 6956: 6942: 6940: 6908: 6900: 6894: 6891: 6890: 6848: 6844: 6825: 6821: 6817: 6813: 6805: 6800: 6797: 6796: 6792: 6783: 6773: 6769: 6752: 6747: 6746: 6744: 6741: 6740: 6737: 6735:Generalizations 6699: 6695: 6689: 6684: 6668: 6646: 6642: 6641: 6629: 6624: 6618: 6613: 6590: 6585: 6579: 6576: 6575: 6571: 6568: 6560: 6556: 6549: 6541: 6479:, as required. 6476: 6454: 6451: 6450: 6449: 6445: 6435: 6431: 6428: 6422: 6419: 6405: 6384: 6381: 6380: 6379: 6375: 6365: 6362: 6356: 6352: 6349: 6343: 6333: 6319: 6313: 6303: 6299: 6293: 6283: 6280: 6274: 6264: 6257: 6251: 6248: 6247: 6237: 6234: 6228: 6217: 6211: 6205: 6202: 6196: 6185: 6181: 6173: 6169: 6153: 6147: 6131: 6125: 6114: 6103: 6085: 6073: 6070: 6056: 6045: 6034: 6031: 6017: 6014: 6006: 5997: 5989: 5984: 5976: 5965: 5957: 5953: 5945: 5938: 5930: 5926: 5922: 5919: 5911: 5908: 5900: 5897: 5889: 5881: 5877: 5870: 5862: 5858: 5855: 5847: 5840: 5830: 5826: 5819: 5815: 5804: 5800: 5796: 5793: 5784: 5781: 5772: 5762: 5753: 5749: 5746: 5737: 5726: 5723: 5714: 5710: 5695: 5692: 5682: 5678: 5674: 5670: 5667: 5659: 5655: 5619:Giuseppe Vitali 5612: 5611: 5580: 5526: 5521: 5486: 5481: 5419: 5414: 5408: 5405: 5404: 5400: 5396: 5392: 5388: 5385: 5380: 5354: 5353: 5349: 5347: 5344: 5343: 5300: 5299: 5295: 5289: 5284: 5254: 5253: 5249: 5243: 5229: 5228: 5198: 5197: 5193: 5178: 5177: 5172: 5166: 5163: 5162: 5143: 5142: 5138: 5136: 5133: 5132: 5082: 5080: 5079: 5075: 5051: 5038: 5037: 5033: 5015: 5008: 5004: 5002: 4999: 4998: 4994: 4984: 4980: 4976: 4973: 4962: 4957: 4953: 4949: 4942: 4937: 4923: 4914: 4910: 4906: 4901: 4897: 4893: 4889: 4885: 4881: 4876: 4872: 4868: 4863: 4859: 4855: 4850: 4842: 4838: 4833: 4825: 4806: 4805: 4801: 4799: 4796: 4795: 4780: 4773: 4766: 4731: 4722: 4718: 4709: 4705: 4704: 4700: 4694: 4679: 4675: 4662: 4653: 4649: 4648: 4644: 4642: 4639: 4638: 4635: 4631: 4628: 4624: 4621: 4617: 4586: 4577: 4573: 4560: 4551: 4547: 4546: 4542: 4540: 4537: 4536: 4533: 4529: 4520: 4515: 4506: 4501: 4498: 4494: 4487: 4484: 4480: 4477: 4473: 4469: 4466: 4462: 4459: 4455: 4452: 4448: 4444: 4441: 4437: 4433: 4429: 4426: 4422: 4412: 4408: 4404: 4393: 4382: 4379: 4375: 4365: 4361: 4358: 4354: 4350: 4343: 4340: 4336: 4333: 4329: 4325: 4322: 4318: 4311: 4308: 4299: 4296: 4292: 4289: 4285: 4282: 4272: 4266: 4262: 4256: 4250: 4228: 4227: 4223: 4221: 4218: 4217: 4197: 4169: 4168: 4164: 4162: 4159: 4158: 4151: 4147: 4131: 4105: 4102: 4101: 4098: 4089: 4085: 4078: 4071: 4033: 4028: 4008: 4004: 3989: 3985: 3984: 3980: 3965: 3960: 3952: 3936: 3932: 3928: 3924: 3920: 3918: 3915: 3914: 3908: 3903: 3902: 3901: 3897: 3856: 3851: 3830: 3826: 3811: 3807: 3805: 3802: 3801: 3798: 3794: 3774: 3770: 3758: 3754: 3739: 3735: 3733: 3730: 3729: 3724: 3719: 3688: 3684: 3669: 3665: 3664: 3660: 3650: 3646: 3642: 3619: 3615: 3600: 3596: 3595: 3591: 3581: 3577: 3573: 3556: 3552: 3537: 3533: 3532: 3528: 3518: 3514: 3510: 3505: 3502: 3501: 3496: 3490: 3479: 3470: 3467: 3458: 3454: 3448: 3442: 3432: 3428: 3400: 3396: 3381: 3377: 3362: 3358: 3349: 3345: 3336: 3332: 3330: 3327: 3326: 3322: 3318: 3312: 3306: 3299: 3289: 3285: 3277: 3273: 3270: 3266: 3249: 3245: 3233: 3229: 3214: 3210: 3208: 3205: 3204: 3197: 3193: 3189: 3186: 3176: 3170: 3166: 3160: 3154: 3150: 3143: 3108: 3104: 3095: 3091: 3090: 3086: 3066: 3062: 3053: 3049: 3048: 3044: 3030: 3026: 3017: 3013: 3012: 3008: 2980: 2975: 2974: 2970: 2959: 2956: 2955: 2951: 2944: 2937: 2933: 2926: 2905: 2888: 2864: 2849: 2846: 2845: 2841: 2834: 2830: 2824: 2818: 2814: 2799: 2795: 2791: 2787: 2783: 2779: 2775: 2774:, and choose a 2771: 2767: 2763: 2752: 2748: 2744: 2696: 2692: 2677: 2673: 2661: 2657: 2639: 2628: 2623: 2619: 2618: 2614: 2612: 2609: 2608: 2605: 2595: 2589: 2585: 2579: 2573: 2570: 2560: 2554: 2550: 2544: 2538: 2535: 2525: 2519: 2515: 2509: 2503: 2496: 2488: 2484: 2435: 2431: 2416: 2412: 2400: 2396: 2378: 2367: 2362: 2358: 2357: 2353: 2351: 2348: 2347: 2343: 2340: 2330: 2324: 2320: 2314: 2308: 2298: 2297:, there exists 2291: 2283: 2279: 2260: 2247: 2243: 2239: 2223: 2222: 2210: 2206: 2191: 2187: 2155: 2151: 2142: 2138: 2128: 2112: 2101: 2090: 2069: 2068: 2056: 2052: 2037: 2033: 2001: 1997: 1988: 1984: 1974: 1958: 1947: 1936: 1914: 1912: 1909: 1908: 1904: 1889: 1883: 1874: 1869: 1860: 1833: 1829: 1814: 1810: 1809: 1805: 1796: 1792: 1774: 1763: 1757: 1754: 1753: 1750: 1740: 1734: 1730: 1724: 1718: 1714: 1704: 1701: 1682: 1678: 1674: 1667: 1662: 1659: 1644: 1639: 1628: 1621: 1617: 1602: 1583: 1568: 1553: 1546: 1541: 1536: 1533: 1523: 1517: 1502: 1441: 1437: 1422: 1418: 1417: 1413: 1408: 1405: 1404: 1367: 1363: 1348: 1344: 1329: 1325: 1316: 1312: 1303: 1299: 1291: 1288: 1287: 1280: 1275: 1266: 1262: 1258: 1254: 1239: 1232: 1228: 1187: 1182: 1176: 1173: 1172: 1168: 1082: 1078: 1070: 1067: 1066: 1058: 1054: 1046: 1043: 987: 958: 957: 943:Integration Bee 918: 915: 908: 907: 883: 880: 873: 872: 845: 842: 835: 834: 816:Volume integral 751: 746: 739: 738: 655: 650: 643: 642: 612: 531: 526: 519: 518: 510:Risch algorithm 480:Euler's formula 366: 361: 354: 353: 335:General Leibniz 218:generalizations 200: 195: 188: 174:Rolle's theorem 169: 144: 80: 74: 69: 63: 60: 59: 17: 12: 11: 5: 8979: 8969: 8968: 8963: 8946: 8945: 8943: 8942: 8929: 8926: 8925: 8923: 8922: 8917: 8912: 8907: 8902: 8897: 8892: 8887: 8882: 8877: 8872: 8867: 8862: 8857: 8852: 8847: 8842: 8837: 8832: 8827: 8825:Riemann sphere 8822: 8820:Riemann solver 8817: 8812: 8807: 8802: 8797: 8792: 8787: 8782: 8777: 8772: 8767: 8762: 8757: 8752: 8747: 8742: 8737: 8731: 8728: 8727: 8720: 8719: 8712: 8705: 8697: 8688: 8687: 8685: 8684: 8683: 8682: 8677: 8669: 8664: 8659: 8657:Gabriel's horn 8654: 8649: 8643: 8641: 8637: 8636: 8634: 8633: 8628: 8623: 8618: 8612: 8610: 8604: 8603: 8601: 8600: 8595: 8590: 8585: 8584: 8583: 8578: 8570: 8565: 8559: 8557: 8551: 8550: 8548: 8547: 8542: 8541: 8540: 8535: 8533:Simpson's rule 8525: 8520: 8515: 8510: 8505: 8500: 8495: 8493:Changing order 8490: 8485: 8480: 8475: 8470: 8469: 8468: 8463: 8458: 8447: 8445: 8439: 8438: 8436: 8435: 8430: 8425: 8420: 8415: 8410: 8405: 8400: 8395: 8390: 8385: 8380: 8375: 8370: 8365: 8360: 8354: 8352: 8346: 8345: 8338: 8337: 8330: 8323: 8315: 8309: 8308: 8290: 8276: 8275:External links 8273: 8272: 8271: 8259: 8242: 8239: 8237: 8236: 8215: 8196:(4): 179–186. 8176: 8129: 8117: 8105: 8090: 8078: 8051:(7): 396–398. 8035: 8023: 8016: 7993: 7978: 7958: 7935: 7933: 7930: 7929: 7928: 7923: 7921:Antiderivative 7918: 7911: 7908: 7904:gauge integral 7891:gauge integral 7874: 7871: 7853: 7849: 7844: 7839: 7811: 7801: 7757: 7754: 7751: 7745: 7741: 7735: 7730: 7727: 7723: 7717: 7714: 7711: 7707: 7703: 7700: 7697: 7693: 7688: 7683: 7680: 7676: 7660: 7642: 7639: 7636: 7633: 7627: 7623: 7617: 7612: 7609: 7605: 7575: 7558: 7555: 7552: 7548: 7545: 7542: 7539: 7534: 7529: 7525: 7521: 7518: 7515: 7511: 7508: 7505: 7500: 7496: 7490: 7485: 7481: 7475: 7472: 7469: 7465: 7442: 7436:Fourier series 7403: 7400: 7397: 7393: 7390: 7387: 7384: 7379: 7374: 7371: 7367: 7361: 7358: 7355: 7351: 7323: 7320: 7317: 7314: 7311: 7309: 7307: 7304: 7300: 7297: 7294: 7291: 7286: 7281: 7278: 7275: 7271: 7267: 7266: 7263: 7260: 7257: 7254: 7252: 7250: 7247: 7243: 7240: 7237: 7234: 7229: 7226: 7221: 7218: 7214: 7210: 7209: 7185: 7182: 7179: 7176: 7172: 7169: 7166: 7163: 7158: 7153: 7150: 7146: 7115:which is 0 at 7084: 7081: 7078: 7074: 7071: 7068: 7065: 7060: 7055: 7052: 7048: 7042: 7039: 7036: 7032: 7006: 7003: 7000: 6996: 6993: 6990: 6987: 6982: 6977: 6973: 6965: 6962: 6959: 6954: 6951: 6948: 6945: 6939: 6935: 6932: 6929: 6925: 6922: 6919: 6916: 6911: 6906: 6903: 6899: 6861: 6857: 6851: 6847: 6843: 6840: 6837: 6833: 6828: 6824: 6820: 6816: 6812: 6808: 6804: 6788: 6781: 6755: 6750: 6736: 6733: 6714: 6711: 6708: 6702: 6698: 6692: 6687: 6683: 6677: 6674: 6671: 6667: 6663: 6659: 6656: 6649: 6645: 6638: 6635: 6632: 6628: 6621: 6616: 6612: 6608: 6605: 6602: 6598: 6593: 6588: 6584: 6564: 6545: 6535:classification 6482: 6481: 6473: 6472: 6452: 6426: 6415: 6404:The intervals 6402: 6382: 6360: 6345: 6341: 6315: 6311: 6295: 6291: 6276: 6272: 6255: 6245: 6241: 6230: 6226: 6209: 6198: 6194: 6151: 6129: 6066: 6027: 6010: 5993: 5980: 5961: 5948:is continuous 5942: 5941: 5934: 5915: 5904: 5893: 5884:) and closed: 5866: 5851: 5788: 5776: 5757: 5741: 5718: 5687: 5663: 5640: 5639: 5623:Henri Lebesgue 5579: 5576: 5553: 5550: 5547: 5543: 5540: 5537: 5534: 5529: 5524: 5520: 5516: 5513: 5510: 5507: 5503: 5500: 5497: 5494: 5489: 5484: 5480: 5476: 5473: 5470: 5467: 5463: 5460: 5457: 5454: 5451: 5448: 5445: 5442: 5439: 5436: 5433: 5430: 5427: 5422: 5417: 5413: 5384: 5381: 5379: 5376: 5363: 5357: 5352: 5325: 5322: 5319: 5315: 5312: 5309: 5303: 5298: 5292: 5287: 5283: 5279: 5276: 5273: 5269: 5266: 5263: 5257: 5252: 5246: 5241: 5238: 5233: 5227: 5223: 5220: 5217: 5213: 5210: 5207: 5201: 5196: 5190: 5187: 5182: 5175: 5171: 5146: 5141: 5111: 5107: 5103: 5100: 5095: 5091: 5088: 5085: 5078: 5074: 5071: 5068: 5064: 5058: 5055: 5050: 5045: 5042: 5036: 5032: 5028: 5022: 5019: 5014: 5011: 5007: 4982: 4968: 4960: 4947: 4940: 4922: 4919: 4904: 4879: 4866: 4862:equivalent to 4853: 4836: 4809: 4804: 4763:rational point 4748: 4744: 4738: 4735: 4730: 4725: 4721: 4717: 4712: 4708: 4703: 4692: 4688: 4682: 4678: 4674: 4669: 4666: 4661: 4656: 4652: 4647: 4633: 4630:, then we let 4626: 4619: 4603: 4599: 4593: 4590: 4585: 4580: 4576: 4572: 4567: 4564: 4559: 4554: 4550: 4545: 4531: 4518: 4504: 4496: 4482: 4475: 4464: 4457: 4450: 4439: 4424: 4377: 4356: 4338: 4331: 4320: 4303: 4294: 4287: 4277: 4270: 4260: 4254: 4249:To start, let 4231: 4226: 4200: 4196: 4193: 4190: 4187: 4184: 4181: 4178: 4172: 4167: 4134: 4130: 4127: 4124: 4121: 4118: 4115: 4112: 4109: 4097: 4094: 4057: 4051: 4048: 4045: 4042: 4039: 4036: 4032: 4027: 4023: 4017: 4014: 4011: 4007: 4003: 3998: 3995: 3992: 3988: 3983: 3978: 3973: 3968: 3963: 3959: 3955: 3951: 3948: 3944: 3939: 3935: 3931: 3927: 3923: 3904: 3883: 3877: 3874: 3871: 3868: 3865: 3862: 3859: 3855: 3850: 3847: 3844: 3839: 3836: 3833: 3829: 3825: 3820: 3817: 3814: 3810: 3796: 3782: 3777: 3773: 3769: 3766: 3761: 3757: 3753: 3750: 3747: 3742: 3738: 3722: 3701: 3697: 3691: 3687: 3683: 3678: 3675: 3672: 3668: 3663: 3658: 3653: 3649: 3645: 3641: 3638: 3634: 3628: 3625: 3622: 3618: 3614: 3609: 3606: 3603: 3599: 3594: 3589: 3584: 3580: 3576: 3572: 3569: 3565: 3559: 3555: 3551: 3546: 3543: 3540: 3536: 3531: 3526: 3521: 3517: 3513: 3509: 3494: 3485: 3477: 3462: 3452: 3446: 3414: 3409: 3406: 3403: 3399: 3395: 3390: 3387: 3384: 3380: 3376: 3371: 3368: 3365: 3361: 3357: 3352: 3348: 3344: 3339: 3335: 3316: 3310: 3275: 3268: 3252: 3248: 3244: 3241: 3236: 3232: 3228: 3225: 3222: 3217: 3213: 3181: 3174: 3164: 3158: 3128: 3123: 3117: 3114: 3111: 3107: 3103: 3098: 3094: 3089: 3085: 3082: 3079: 3075: 3069: 3065: 3061: 3056: 3052: 3047: 3043: 3039: 3033: 3029: 3025: 3020: 3016: 3011: 3007: 3001: 2998: 2995: 2992: 2989: 2986: 2983: 2979: 2973: 2969: 2966: 2963: 2912: 2908: 2904: 2901: 2898: 2895: 2891: 2885: 2882: 2879: 2876: 2873: 2870: 2867: 2863: 2859: 2856: 2853: 2828: 2822: 2729: 2726: 2723: 2719: 2715: 2712: 2708: 2704: 2699: 2695: 2691: 2686: 2683: 2680: 2676: 2672: 2669: 2664: 2660: 2656: 2653: 2648: 2645: 2642: 2637: 2634: 2631: 2627: 2622: 2617: 2600: 2593: 2583: 2577: 2565: 2558: 2548: 2542: 2530: 2523: 2513: 2507: 2468: 2465: 2462: 2458: 2454: 2451: 2447: 2443: 2438: 2434: 2430: 2425: 2422: 2419: 2415: 2411: 2408: 2403: 2399: 2395: 2392: 2387: 2384: 2381: 2376: 2373: 2370: 2366: 2361: 2356: 2335: 2328: 2318: 2312: 2259: 2256: 2221: 2218: 2213: 2209: 2205: 2200: 2197: 2194: 2190: 2186: 2183: 2180: 2177: 2174: 2169: 2164: 2161: 2158: 2154: 2150: 2145: 2141: 2137: 2134: 2131: 2127: 2121: 2118: 2115: 2110: 2107: 2104: 2100: 2096: 2093: 2091: 2089: 2086: 2083: 2080: 2077: 2074: 2071: 2070: 2067: 2064: 2059: 2055: 2051: 2046: 2043: 2040: 2036: 2032: 2029: 2026: 2023: 2020: 2015: 2010: 2007: 2004: 2000: 1996: 1991: 1987: 1983: 1980: 1977: 1973: 1967: 1964: 1961: 1956: 1953: 1950: 1946: 1942: 1939: 1937: 1935: 1932: 1929: 1926: 1923: 1920: 1917: 1916: 1887: 1878: 1867: 1846: 1842: 1836: 1832: 1828: 1823: 1820: 1817: 1813: 1808: 1804: 1799: 1795: 1791: 1788: 1783: 1780: 1777: 1772: 1769: 1766: 1762: 1745: 1738: 1733:together with 1728: 1722: 1700: 1697: 1672: 1665: 1661:and such that 1650: 1642: 1539: 1528: 1521: 1485: 1482: 1479: 1476: 1473: 1470: 1467: 1464: 1461: 1458: 1454: 1450: 1444: 1440: 1436: 1431: 1428: 1425: 1421: 1416: 1412: 1378: 1375: 1370: 1366: 1362: 1359: 1356: 1351: 1347: 1343: 1340: 1337: 1332: 1328: 1324: 1319: 1315: 1311: 1306: 1302: 1298: 1295: 1279: 1276: 1274: 1271: 1214: 1211: 1208: 1204: 1201: 1198: 1195: 1190: 1185: 1181: 1154: 1150: 1146: 1143: 1140: 1137: 1134: 1131: 1128: 1125: 1121: 1117: 1114: 1111: 1108: 1105: 1101: 1097: 1094: 1091: 1088: 1085: 1081: 1077: 1074: 1042: 1039: 989: 988: 986: 985: 978: 971: 963: 960: 959: 956: 955: 950: 945: 940: 938:List of topics 935: 930: 925: 919: 914: 913: 910: 909: 906: 905: 900: 895: 890: 884: 879: 878: 875: 874: 869: 868: 867: 866: 861: 856: 846: 841: 840: 837: 836: 831: 830: 829: 828: 823: 818: 813: 808: 803: 798: 790: 789: 785: 784: 783: 782: 777: 772: 767: 759: 758: 752: 745: 744: 741: 740: 735: 734: 733: 732: 727: 722: 717: 712: 707: 699: 698: 694: 693: 692: 691: 686: 681: 676: 671: 666: 656: 649: 648: 645: 644: 639: 638: 637: 636: 631: 626: 621: 616: 610: 605: 600: 595: 590: 582: 581: 575: 574: 573: 572: 567: 562: 557: 552: 547: 532: 525: 524: 521: 520: 515: 514: 513: 512: 507: 502: 497: 495:Changing order 492: 482: 477: 459: 454: 449: 441: 440: 439:Integration by 436: 435: 434: 433: 428: 423: 418: 413: 403: 401:Antiderivative 395: 394: 390: 389: 388: 387: 382: 377: 367: 360: 359: 356: 355: 350: 349: 348: 347: 342: 337: 332: 327: 322: 317: 312: 307: 302: 294: 293: 287: 286: 285: 284: 279: 274: 269: 264: 259: 251: 250: 246: 245: 244: 243: 242: 241: 236: 231: 221: 208: 207: 201: 194: 193: 190: 189: 187: 186: 181: 176: 170: 168: 167: 162: 156: 155: 154: 146: 145: 133: 130: 127: 124: 121: 118: 115: 112: 109: 106: 103: 100: 96: 93: 90: 86: 83: 77: 72: 68: 58: 55: 54: 48: 47: 15: 9: 6: 4: 3: 2: 8978: 8967: 8964: 8962: 8959: 8958: 8956: 8941: 8940: 8931: 8930: 8927: 8921: 8918: 8916: 8913: 8911: 8908: 8906: 8903: 8901: 8898: 8896: 8893: 8891: 8888: 8886: 8883: 8881: 8878: 8876: 8873: 8871: 8868: 8866: 8863: 8861: 8858: 8856: 8853: 8851: 8848: 8846: 8843: 8841: 8838: 8836: 8833: 8831: 8828: 8826: 8823: 8821: 8818: 8816: 8813: 8811: 8808: 8806: 8803: 8801: 8798: 8796: 8793: 8791: 8788: 8786: 8783: 8781: 8778: 8776: 8773: 8771: 8768: 8766: 8763: 8761: 8758: 8756: 8753: 8751: 8748: 8746: 8743: 8741: 8738: 8736: 8733: 8732: 8729: 8725: 8718: 8713: 8711: 8706: 8704: 8699: 8698: 8695: 8681: 8678: 8676: 8673: 8672: 8670: 8668: 8665: 8663: 8660: 8658: 8655: 8653: 8650: 8648: 8647:Basel problem 8645: 8644: 8642: 8640:Miscellaneous 8638: 8632: 8629: 8627: 8624: 8622: 8619: 8617: 8614: 8613: 8611: 8609: 8605: 8599: 8596: 8594: 8591: 8589: 8586: 8582: 8579: 8577: 8574: 8573: 8571: 8569: 8566: 8564: 8561: 8560: 8558: 8556: 8552: 8546: 8543: 8539: 8536: 8534: 8531: 8530: 8529: 8526: 8524: 8521: 8519: 8516: 8514: 8511: 8509: 8506: 8504: 8501: 8499: 8496: 8494: 8491: 8489: 8486: 8484: 8481: 8479: 8476: 8474: 8471: 8467: 8464: 8462: 8459: 8457: 8456:Trigonometric 8454: 8453: 8452: 8449: 8448: 8446: 8440: 8434: 8431: 8429: 8426: 8424: 8421: 8419: 8416: 8414: 8411: 8409: 8406: 8404: 8401: 8399: 8396: 8394: 8393:Haar integral 8391: 8389: 8386: 8384: 8381: 8379: 8376: 8374: 8371: 8369: 8366: 8364: 8361: 8359: 8356: 8355: 8353: 8347: 8343: 8336: 8331: 8329: 8324: 8322: 8317: 8316: 8313: 8305: 8301: 8300: 8295: 8291: 8288: 8283: 8279: 8278: 8268: 8264: 8260: 8257: 8256:0-486-63519-8 8253: 8249: 8245: 8244: 8225: 8219: 8211: 8207: 8203: 8199: 8195: 8191: 8187: 8180: 8172: 8168: 8164: 8160: 8156: 8152: 8148: 8144: 8140: 8133: 8126: 8121: 8114: 8109: 8102: 8100: 8094: 8088: 8082: 8074: 8070: 8066: 8062: 8058: 8054: 8050: 8046: 8039: 8032: 8027: 8019: 8017:9780821872468 8013: 8009: 8008: 8003: 7997: 7989: 7985: 7981: 7979:1-58488-483-5 7975: 7971: 7970: 7962: 7955: 7951: 7947: 7940: 7936: 7927: 7924: 7922: 7919: 7917: 7914: 7913: 7907: 7905: 7900: 7895: 7892: 7888: 7884: 7880: 7870: 7868: 7842: 7827: 7822: 7820: 7814: 7810: 7804: 7800: 7794: 7792: 7787: 7777: 7772: 7768: 7755: 7752: 7749: 7743: 7739: 7725: 7721: 7709: 7701: 7698: 7695: 7691: 7678: 7674: 7663: 7655:The sequence 7653: 7640: 7637: 7634: 7631: 7625: 7621: 7607: 7603: 7589: 7582: 7578: 7569: 7556: 7553: 7550: 7543: 7537: 7532: 7527: 7523: 7519: 7516: 7513: 7506: 7498: 7494: 7488: 7483: 7479: 7467: 7450: 7445: 7437: 7431: 7422: 7418: 7401: 7398: 7395: 7388: 7382: 7377: 7372: 7369: 7365: 7353: 7338: 7321: 7318: 7315: 7312: 7310: 7305: 7302: 7295: 7289: 7284: 7279: 7276: 7273: 7269: 7261: 7258: 7255: 7253: 7248: 7245: 7238: 7232: 7227: 7224: 7219: 7216: 7212: 7183: 7180: 7177: 7174: 7167: 7161: 7156: 7151: 7148: 7144: 7133: 7129:, and −1 for 7126: 7119: 7112: 7108: 7104: 7100: 7099:sign function 7095: 7082: 7079: 7076: 7069: 7063: 7058: 7053: 7050: 7046: 7034: 7022: 7017: 7004: 7001: 6998: 6991: 6985: 6980: 6975: 6971: 6957: 6949: 6943: 6933: 6930: 6927: 6920: 6914: 6901: 6897: 6888: 6884: 6879: 6877: 6872: 6859: 6855: 6849: 6845: 6841: 6838: 6835: 6831: 6826: 6822: 6818: 6814: 6810: 6802: 6791: 6787: 6780: 6776: 6753: 6732: 6730: 6727:However, the 6725: 6712: 6709: 6706: 6700: 6696: 6690: 6685: 6681: 6669: 6661: 6657: 6654: 6647: 6643: 6630: 6619: 6614: 6610: 6606: 6603: 6600: 6596: 6591: 6586: 6582: 6567: 6563: 6554: 6548: 6544: 6538: 6536: 6530: 6528: 6524: 6520: 6515: 6513: 6509: 6505: 6500: 6498: 6493: 6489: 6480: 6468: 6464: 6460: 6448: 6442: 6438: 6425: 6418: 6413: 6409: 6403: 6398: 6394: 6390: 6378: 6372: 6368: 6359: 6348: 6340: 6336: 6331: 6330: 6329: 6326: 6323: 6318: 6310: 6306: 6298: 6290: 6286: 6279: 6271: 6267: 6262: 6254: 6244: 6240: 6233: 6225: 6221: 6214: 6208: 6201: 6193: 6189: 6179: 6165: 6161: 6157: 6150: 6143: 6139: 6135: 6128: 6122: 6120: 6119:partition of 6112: 6106: 6100: 6096: 6092: 6088: 6081: 6077: 6069: 6064: 6060: 6054: 6049: 6042: 6038: 6030: 6025: 6021: 6013: 6009: 6004: 6000: 5996: 5992: 5983: 5979: 5974: 5970: 5964: 5960: 5951: 5937: 5933: 5918: 5914: 5907: 5903: 5896: 5892: 5887: 5886: 5885: 5875: 5869: 5865: 5854: 5850: 5844: 5837: 5833: 5823: 5813: 5808: 5792: 5787: 5780: 5775: 5770: 5769:partition of 5765: 5761: 5756: 5745: 5740: 5736: 5732: 5722: 5717: 5708: 5704: 5699: 5691: 5686: 5666: 5662: 5653: 5646: 5642: 5641: 5637: 5636: 5633: 5630: 5628: 5624: 5620: 5616: 5608: 5604: 5600: 5596: 5593: 5589: 5585: 5578:Integrability 5575: 5573: 5569: 5564: 5551: 5548: 5545: 5538: 5532: 5527: 5522: 5518: 5514: 5511: 5508: 5505: 5498: 5492: 5487: 5482: 5478: 5474: 5471: 5468: 5465: 5455: 5449: 5446: 5443: 5437: 5431: 5428: 5420: 5415: 5411: 5375: 5361: 5350: 5340: 5336: 5323: 5320: 5317: 5310: 5296: 5290: 5285: 5281: 5277: 5274: 5271: 5264: 5250: 5244: 5239: 5236: 5231: 5225: 5221: 5218: 5215: 5208: 5194: 5188: 5185: 5180: 5173: 5169: 5139: 5131: 5125: 5122: 5109: 5105: 5101: 5098: 5093: 5089: 5086: 5083: 5076: 5072: 5069: 5066: 5062: 5056: 5053: 5048: 5043: 5040: 5034: 5030: 5026: 5020: 5017: 5012: 5009: 5005: 4991: 4989: 4971: 4967: 4963: 4950: 4943: 4934: 4930: 4928: 4918: 4913:. Therefore, 4907: 4882: 4869: 4856: 4848: 4839: 4831: 4802: 4792: 4790: 4786: 4777: 4770: 4764: 4759: 4746: 4742: 4736: 4733: 4728: 4723: 4719: 4715: 4710: 4706: 4701: 4690: 4686: 4680: 4676: 4672: 4667: 4664: 4659: 4654: 4650: 4645: 4614: 4601: 4597: 4591: 4588: 4583: 4578: 4574: 4570: 4565: 4562: 4557: 4552: 4548: 4543: 4525: 4521: 4511: 4507: 4491: 4419: 4415: 4401: 4397: 4390: 4386: 4372: 4368: 4347: 4314: 4306: 4302: 4280: 4276: 4269: 4263: 4253: 4247: 4224: 4215: 4188: 4185: 4182: 4176: 4165: 4155: 4122: 4119: 4116: 4110: 4107: 4093: 4081: 4074: 4068: 4055: 4046: 4043: 4040: 4034: 4030: 4025: 4021: 4015: 4012: 4009: 4005: 4001: 3996: 3993: 3990: 3986: 3981: 3976: 3971: 3966: 3961: 3957: 3953: 3949: 3946: 3942: 3937: 3933: 3929: 3925: 3921: 3907: 3900: 3894: 3881: 3872: 3869: 3866: 3860: 3857: 3853: 3848: 3845: 3842: 3837: 3834: 3831: 3827: 3823: 3818: 3815: 3812: 3808: 3780: 3775: 3771: 3767: 3759: 3755: 3748: 3745: 3740: 3736: 3725: 3717: 3712: 3699: 3695: 3689: 3685: 3681: 3676: 3673: 3670: 3666: 3661: 3656: 3651: 3647: 3643: 3639: 3636: 3632: 3626: 3623: 3620: 3616: 3612: 3607: 3604: 3601: 3597: 3592: 3587: 3582: 3578: 3574: 3570: 3567: 3563: 3557: 3553: 3549: 3544: 3541: 3538: 3534: 3529: 3524: 3519: 3515: 3511: 3507: 3497: 3488: 3484: 3480: 3473: 3465: 3461: 3455: 3445: 3439: 3435: 3425: 3412: 3407: 3404: 3401: 3397: 3393: 3388: 3385: 3382: 3378: 3374: 3369: 3366: 3363: 3359: 3355: 3350: 3346: 3342: 3337: 3333: 3319: 3309: 3302: 3296: 3292: 3282: 3250: 3246: 3242: 3234: 3230: 3223: 3220: 3215: 3211: 3201: 3184: 3180: 3173: 3167: 3157: 3146: 3140: 3126: 3121: 3115: 3112: 3109: 3105: 3101: 3096: 3092: 3087: 3083: 3080: 3077: 3073: 3067: 3063: 3059: 3054: 3050: 3045: 3041: 3037: 3031: 3027: 3023: 3018: 3014: 3009: 3005: 2996: 2993: 2990: 2984: 2981: 2977: 2971: 2964: 2961: 2947: 2940: 2929: 2923: 2910: 2899: 2893: 2880: 2877: 2874: 2868: 2865: 2857: 2854: 2851: 2840:of the value 2837: 2831: 2821: 2812: 2808: 2803: 2760: 2758: 2740: 2727: 2724: 2721: 2717: 2713: 2710: 2706: 2697: 2693: 2689: 2684: 2681: 2678: 2674: 2662: 2658: 2651: 2646: 2643: 2640: 2635: 2632: 2629: 2625: 2620: 2615: 2603: 2599: 2592: 2586: 2576: 2568: 2564: 2557: 2551: 2541: 2533: 2529: 2522: 2516: 2506: 2499: 2492: 2479: 2466: 2463: 2460: 2456: 2452: 2449: 2445: 2436: 2432: 2428: 2423: 2420: 2417: 2413: 2401: 2397: 2390: 2385: 2382: 2379: 2374: 2371: 2368: 2364: 2359: 2354: 2338: 2334: 2327: 2321: 2311: 2307: 2301: 2294: 2287: 2275: 2273: 2269: 2265: 2255: 2253: 2236: 2219: 2211: 2207: 2203: 2198: 2195: 2192: 2188: 2178: 2172: 2162: 2159: 2156: 2152: 2148: 2143: 2139: 2132: 2129: 2119: 2116: 2113: 2108: 2105: 2102: 2098: 2094: 2092: 2084: 2081: 2078: 2072: 2065: 2057: 2053: 2049: 2044: 2041: 2038: 2034: 2024: 2018: 2008: 2005: 2002: 1998: 1994: 1989: 1985: 1978: 1975: 1965: 1962: 1959: 1954: 1951: 1948: 1944: 1940: 1938: 1930: 1927: 1924: 1918: 1902: 1898: 1893: 1890: 1881: 1877: 1870: 1863: 1857: 1844: 1840: 1834: 1830: 1826: 1821: 1818: 1815: 1811: 1806: 1797: 1793: 1786: 1781: 1778: 1775: 1770: 1767: 1764: 1760: 1748: 1744: 1737: 1731: 1721: 1712: 1711: 1696: 1694: 1689: 1685: 1675: 1668: 1657: 1653: 1649: 1645: 1635: 1631: 1624: 1613: 1609: 1605: 1600: 1594: 1590: 1586: 1579: 1575: 1571: 1564: 1560: 1556: 1550: 1542: 1531: 1527: 1520: 1513: 1509: 1505: 1501: 1496: 1483: 1477: 1474: 1471: 1468: 1465: 1459: 1456: 1452: 1448: 1442: 1438: 1434: 1429: 1426: 1423: 1419: 1414: 1402: 1398: 1394: 1389: 1376: 1373: 1368: 1364: 1360: 1357: 1354: 1349: 1345: 1341: 1338: 1335: 1330: 1326: 1322: 1317: 1313: 1309: 1304: 1300: 1296: 1293: 1285: 1270: 1252: 1246: 1242: 1236: 1225: 1212: 1209: 1206: 1199: 1193: 1188: 1183: 1179: 1165: 1152: 1148: 1141: 1135: 1132: 1129: 1126: 1123: 1119: 1115: 1112: 1109: 1106: 1103: 1099: 1092: 1089: 1086: 1079: 1075: 1072: 1064: 1052: 1038: 1036: 1032: 1028: 1024: 1020: 1016: 1012: 1008: 1005:, created by 1004: 1000: 999:real analysis 996: 984: 979: 977: 972: 970: 965: 964: 962: 961: 954: 951: 949: 946: 944: 941: 939: 936: 934: 931: 929: 926: 924: 921: 920: 912: 911: 904: 901: 899: 896: 894: 891: 889: 886: 885: 877: 876: 865: 862: 860: 857: 855: 852: 851: 850: 849: 839: 838: 827: 824: 822: 819: 817: 814: 812: 809: 807: 806:Line integral 804: 802: 799: 797: 794: 793: 792: 791: 787: 786: 781: 778: 776: 773: 771: 768: 766: 763: 762: 761: 760: 756: 755: 749: 748:Multivariable 743: 742: 731: 728: 726: 723: 721: 718: 716: 713: 711: 708: 706: 703: 702: 701: 700: 696: 695: 690: 687: 685: 682: 680: 677: 675: 672: 670: 667: 665: 662: 661: 660: 659: 653: 647: 646: 635: 632: 630: 627: 625: 622: 620: 617: 615: 611: 609: 606: 604: 601: 599: 596: 594: 591: 589: 586: 585: 584: 583: 580: 577: 576: 571: 568: 566: 563: 561: 558: 556: 553: 551: 548: 545: 541: 538: 537: 536: 535: 529: 523: 522: 511: 508: 506: 503: 501: 498: 496: 493: 490: 486: 483: 481: 478: 475: 471: 467: 466:trigonometric 463: 460: 458: 455: 453: 450: 448: 445: 444: 443: 442: 438: 437: 432: 429: 427: 424: 422: 419: 417: 414: 411: 407: 404: 402: 399: 398: 397: 396: 392: 391: 386: 383: 381: 378: 376: 373: 372: 371: 370: 364: 358: 357: 346: 343: 341: 338: 336: 333: 331: 328: 326: 323: 321: 318: 316: 313: 311: 308: 306: 303: 301: 298: 297: 296: 295: 292: 289: 288: 283: 280: 278: 277:Related rates 275: 273: 270: 268: 265: 263: 260: 258: 255: 254: 253: 252: 248: 247: 240: 237: 235: 234:of a function 232: 230: 229:infinitesimal 227: 226: 225: 222: 219: 215: 212: 211: 210: 209: 205: 204: 198: 192: 191: 185: 182: 180: 177: 175: 172: 171: 166: 163: 161: 158: 157: 153: 150: 149: 148: 147: 128: 122: 119: 113: 107: 104: 101: 98: 91: 84: 81: 75: 70: 66: 57: 56: 53: 50: 49: 45: 44: 37: 29: 21: 8937: 8805:Riemann form 8789: 8616:Itô integral 8451:Substitution 8442:Integration 8357: 8297: 8266: 8263:Apostol, Tom 8247: 8227:. Retrieved 8218: 8193: 8189: 8179: 8146: 8142: 8132: 8120: 8108: 8097: 8093: 8081: 8048: 8044: 8038: 8031:Apostol 1974 8026: 8006: 7996: 7968: 7961: 7949: 7945: 7939: 7896: 7876: 7823: 7812: 7808: 7802: 7798: 7795: 7788: 7773: 7769: 7658: 7654: 7587: 7580: 7573: 7570: 7440: 7432: 7420: 7416: 7339: 7131: 7124: 7117: 7110: 7106: 7102: 7096: 7018: 6880: 6876:vector space 6873: 6789: 6785: 6778: 6774: 6738: 6726: 6565: 6561: 6546: 6542: 6539: 6531: 6526: 6516: 6501: 6499:point in ). 6496: 6485: 6474: 6466: 6462: 6458: 6446: 6440: 6436: 6423: 6416: 6411: 6407: 6396: 6392: 6388: 6376: 6370: 6366: 6357: 6346: 6338: 6334: 6327: 6321: 6316: 6308: 6304: 6296: 6288: 6284: 6277: 6269: 6265: 6252: 6242: 6238: 6231: 6223: 6219: 6215: 6206: 6199: 6191: 6187: 6163: 6159: 6155: 6148: 6141: 6137: 6133: 6126: 6123: 6121:as follows: 6109:, there are 6104: 6101: 6094: 6090: 6086: 6079: 6075: 6067: 6062: 6058: 6050: 6040: 6036: 6028: 6023: 6019: 6011: 6007: 5994: 5990: 5981: 5977: 5962: 5958: 5943: 5935: 5931: 5916: 5912: 5905: 5901: 5894: 5890: 5867: 5863: 5852: 5848: 5845: 5835: 5831: 5821: 5806: 5790: 5785: 5778: 5773: 5766: 5759: 5754: 5743: 5738: 5720: 5715: 5700: 5689: 5684: 5664: 5660: 5649: 5631: 5627:measure zero 5610: 5603:measure zero 5581: 5572:vector space 5565: 5386: 5341: 5337: 5126: 5123: 4992: 4969: 4965: 4958: 4945: 4938: 4935: 4931: 4924: 4902: 4877: 4864: 4851: 4834: 4793: 4778: 4768: 4760: 4615: 4523: 4516: 4509: 4502: 4492: 4417: 4413: 4399: 4395: 4392:and at most 4388: 4384: 4370: 4366: 4348: 4312: 4304: 4300: 4278: 4274: 4267: 4258: 4251: 4248: 4156: 4099: 4079: 4072: 4069: 3905: 3898: 3895: 3720: 3713: 3492: 3486: 3482: 3475: 3471: 3463: 3459: 3450: 3443: 3440: 3433: 3426: 3314: 3307: 3300: 3297: 3290: 3280: 3202: 3182: 3178: 3171: 3162: 3155: 3144: 3141: 2945: 2938: 2927: 2924: 2835: 2826: 2819: 2804: 2761: 2742: 2601: 2597: 2590: 2581: 2574: 2566: 2562: 2555: 2546: 2539: 2531: 2527: 2520: 2511: 2504: 2497: 2494: 2481: 2336: 2332: 2325: 2316: 2309: 2299: 2292: 2289: 2276: 2271: 2267: 2264:Riemann sums 2261: 2237: 1896: 1894: 1885: 1879: 1875: 1865: 1861: 1858: 1746: 1742: 1735: 1726: 1719: 1708: 1702: 1693:directed set 1690: 1683: 1670: 1663: 1655: 1651: 1647: 1640: 1633: 1629: 1622: 1611: 1607: 1603: 1598: 1592: 1588: 1584: 1577: 1573: 1569: 1562: 1558: 1554: 1551: 1537: 1529: 1525: 1518: 1511: 1507: 1503: 1499: 1497: 1400: 1396: 1393:sub-interval 1392: 1390: 1281: 1250: 1244: 1240: 1237: 1226: 1166: 1044: 1002: 992: 462:Substitution 415: 224:Differential 197:Differential 8830:Riemann sum 8466:Weierstrass 8229:27 February 8087:pp. 264–271 5921:, and thus 5652:oscillation 4909:, which is 4428:are within 4291:is between 3469:. The term 1710:Riemann sum 1699:Riemann sum 1251:signed area 995:mathematics 923:Precalculus 916:Miscellanea 881:Specialized 788:Definitions 555:Alternating 393:Definitions 206:Definitions 8955:Categories 8581:incomplete 8444:techniques 8241:References 6261:open cover 6099:as well). 6053:complement 5999:is compact 5973:open cover 5767:For every 5705:, then by 5592:continuous 5378:Properties 4832:, and let 4443:is within 4310:). Choose 4154:on is 1. 2954:such that 2607:, we have 2346:, we have 2268:integrable 1873:and width 1638:such that 1599:refinement 1273:Definition 903:Variations 898:Stochastic 888:Fractional 757:Formalisms 720:Divergence 689:Identities 669:Divergence 214:Derivative 165:Continuity 8351:integrals 8349:Types of 8342:Integrals 8304:EMS Press 8163:0002-9890 8065:0002-9890 7848:→ 7734:∞ 7729:∞ 7726:− 7722:∫ 7716:∞ 7713:→ 7702:≠ 7687:∞ 7682:∞ 7679:− 7675:∫ 7616:∞ 7611:∞ 7608:− 7604:∫ 7595:we have: 7524:∫ 7480:∫ 7474:∞ 7471:→ 7370:− 7366:∫ 7360:∞ 7357:→ 7316:− 7274:− 7270:∫ 7217:− 7213:∫ 7149:− 7145:∫ 7051:− 7047:∫ 7041:∞ 7038:→ 6972:∫ 6964:∞ 6961:→ 6953:∞ 6950:− 6947:→ 6910:∞ 6905:∞ 6902:− 6898:∫ 6842:∫ 6836:… 6819:∫ 6803:∫ 6682:∫ 6676:∞ 6673:→ 6637:∞ 6634:→ 6611:∫ 6583:∫ 6488:countable 5967:has zero 5731:countable 5519:∫ 5515:β 5479:∫ 5475:α 5447:β 5429:α 5412:∫ 5383:Linearity 5282:∫ 5237:− 5226:∫ 5186:− 5170:∫ 5087:− 5070:… 4734:δ 4665:δ 4660:− 4589:δ 4563:δ 4558:− 4468:, choose 4407:. So let 4195:→ 4129:→ 4044:− 4031:ε 4002:− 3967:∗ 3947:− 3870:− 3854:ε 3846:δ 3824:− 3768:≤ 3746:≤ 3714:Suppose, 3682:− 3613:− 3550:− 3243:≤ 3221:≤ 3113:− 3102:− 3081:⋯ 3060:− 3024:− 2994:− 2978:ε 2962:δ 2869:∈ 2725:ε 2711:− 2690:− 2644:− 2626:∑ 2464:ε 2450:− 2429:− 2383:− 2365:∑ 2204:− 2133:∈ 2117:− 2099:∑ 2050:− 1979:∈ 1963:− 1945:∑ 1827:− 1779:− 1761:∑ 1677:for some 1545:for each 1475:− 1460:∈ 1435:− 1358:⋯ 1339:⋯ 1180:∫ 1113:≤ 1107:≤ 997:known as 893:Malliavin 780:Geometric 679:Laplacian 629:Dirichlet 540:Geometric 120:− 67:∫ 8939:Category 8671:Volumes 8576:complete 8473:By parts 8265:(1974), 8004:(2006). 7988:56214595 7910:See also 7122:, 1 for 7109:) = sgn( 6768:for any 6527:stronger 6519:monotone 6176:are the 6168:, where 6003:subcover 5735:covering 5713:so that 4975:for all 4952:for all 4447:of some 4096:Examples 2495:For all 2290:For all 1257:and the 1041:Overview 1019:interval 1015:function 1011:integral 933:Glossary 843:Advanced 821:Jacobian 775:Exterior 705:Gradient 697:Theorems 664:Gradient 603:Integral 565:Binomial 550:Harmonic 410:improper 406:Integral 363:Integral 345:Reynolds 320:Quotient 249:Concepts 85:′ 52:Calculus 8675:Washers 8306:, 2001 8210:2688673 8171:2316325 8073:2301737 7889:. The 6784:, ..., 6124:Denote 6072:} (for 6033:}, for 5874:compact 5621:and by 5570:on the 4988:cofinal 4875:, like 4845:is not 4828:be the 4353:of the 4273:, ..., 4257:, ..., 4212:be the 3728:. Then 3718:, that 3449:, ..., 3438:times. 3313:, ..., 3177:, ..., 3161:, ..., 2932:, then 2825:, ..., 2596:, ..., 2580:, ..., 2561:, ..., 2545:, ..., 2526:, ..., 2510:, ..., 2331:, ..., 2315:, ..., 1741:, ..., 1725:, ..., 1620:, with 1524:, ..., 1269:-axis. 928:History 826:Hessian 715:Stokes' 710:Green's 542: ( 464: ( 408: ( 330:Inverse 305:Product 216: ( 8680:Shells 8254: 8208: 8169: 8161: 8071: 8063: 8014: 7986: 7976: 7585:to be 7134:< 0 7127:> 0 6574:, and 6430:, and 6107:> 0 5988:Since 5810:, the 5658:, Let 5638:Proof 4454:, and 4317:. The 4315:> 0 3303:> 1 3265:where 2948:> 1 2941:> 0 2500:> 0 2302:> 0 2295:> 0 1017:on an 1001:, the 770:Tensor 765:Matrix 652:Vector 570:Taylor 528:Series 160:Limits 8461:Euler 8206:JSTOR 8167:JSTOR 8069:JSTOR 7932:Notes 7428:∞ − ∞ 6883:limit 6795:then 6551:is a 6497:every 5586:on a 2943:. If 1681:with 1597:is a 1535:with 1238:When 1013:of a 593:Ratio 560:Power 474:Euler 452:Discs 447:Parts 315:Power 310:Chain 239:total 8252:ISBN 8231:2014 8159:ISSN 8061:ISSN 8012:ISBN 7984:OCLC 7974:ISBN 7954:here 7916:Area 7865:are 7423:− 1) 6490:has 6465:) = 6395:) = 6172:and 6158:/ 2( 6146:and 6136:/ 2( 6074:1 ≤ 6051:The 6035:1 ≤ 5880:and 5601:has 5399:and 5391:and 4767:1 − 4479:and 4394:1 · 4383:0 · 4298:and 4265:and 4157:Let 4100:Let 4026:< 3849:< 3843:< 3394:< 3375:< 3356:< 3343:< 3272:and 3169:and 2965:< 2722:< 2588:and 2553:and 2518:and 2461:< 2323:and 1703:Let 1567:and 1401:norm 1397:mesh 1361:< 1355:< 1342:< 1336:< 1323:< 1310:< 1133:< 1127:< 1051:real 1045:Let 674:Curl 634:Abel 598:Root 8198:doi 8151:doi 8053:doi 7824:In 7805:+ 1 7706:lim 7464:lim 7451:to 7430:). 7350:lim 7120:= 0 7031:lim 6938:lim 6777:= ( 6666:lim 6627:lim 6540:If 6502:An 6495:at 6180:of 6097:+ 1 6082:− 1 5975:of 5872:is 5814:of 4972:+ 1 4911:1/2 4696:and 4616:If 4307:+ 1 4281:− 1 4075:− 1 3489:+ 1 3466:+ 1 3457:at 3436:− 1 3293:= 1 3196:of 3185:− 1 3147:= 1 3142:If 2968:min 2930:= 0 2925:If 2862:sup 2798:of 2786:of 2757:net 2604:− 1 2569:− 1 2534:− 1 2339:− 1 2238:If 2126:sup 1972:inf 1882:+ 1 1752:is 1749:− 1 1713:of 1601:of 1532:− 1 1411:max 1399:or 1065:as 300:Sum 8957:: 8302:, 8296:, 8204:. 8194:40 8192:. 8188:. 8165:. 8157:. 8147:78 8145:. 8141:. 8067:. 8059:. 8049:43 8047:. 7982:. 7869:. 7821:. 7807:− 7793:. 7641:1. 6889:: 6469:/2 6461:− 6439:− 6399:/2 6391:− 6369:− 6320:− 6213:. 6162:− 6154:= 6140:− 6132:= 6093:, 6089:= 6078:≤ 6048:. 6039:≤ 5956:, 5861:, 5820:1/ 5805:1/ 5789:1/ 5777:1/ 5758:1/ 5742:1/ 5719:1/ 5698:. 5688:1/ 5582:A 4964:= 4944:= 4849:, 4776:. 4526:/2 4522:+ 4512:/2 4508:− 4092:. 4082:/2 3913:, 3911:∈ 3726:∈ 3491:− 3481:)( 3200:. 2838:/2 2802:. 2759:. 1884:− 1686:∈ 1669:= 1646:= 1625:∈ 1610:, 1591:, 1576:, 1561:, 1543:∈ 1510:, 1498:A 1282:A 1037:. 472:, 468:, 8716:e 8709:t 8702:v 8334:e 8327:t 8320:v 8258:. 8233:. 8212:. 8200:: 8173:. 8153:: 8101:, 8075:. 8055:: 8020:. 7990:. 7852:R 7843:n 7838:R 7813:k 7809:x 7803:k 7799:x 7784:f 7780:f 7756:. 7753:x 7750:d 7744:n 7740:f 7710:n 7699:x 7696:d 7692:f 7664:) 7661:n 7659:f 7657:( 7638:= 7635:x 7632:d 7626:n 7622:f 7593:n 7588:n 7583:) 7581:x 7579:( 7576:n 7574:f 7557:. 7554:x 7551:d 7547:) 7544:x 7541:( 7538:f 7533:b 7528:a 7520:= 7517:x 7514:d 7510:) 7507:x 7504:( 7499:n 7495:f 7489:b 7484:a 7468:n 7453:f 7443:n 7441:f 7421:x 7419:( 7417:f 7402:, 7399:x 7396:d 7392:) 7389:x 7386:( 7383:f 7378:a 7373:a 7354:a 7322:. 7319:a 7313:= 7306:x 7303:d 7299:) 7296:x 7293:( 7290:f 7285:a 7280:a 7277:2 7262:, 7259:a 7256:= 7249:x 7246:d 7242:) 7239:x 7236:( 7233:f 7228:a 7225:2 7220:a 7198:a 7184:0 7181:= 7178:x 7175:d 7171:) 7168:x 7165:( 7162:f 7157:a 7152:a 7132:x 7125:x 7118:x 7113:) 7111:x 7107:x 7105:( 7103:f 7083:. 7080:x 7077:d 7073:) 7070:x 7067:( 7064:f 7059:a 7054:a 7035:a 7005:. 7002:x 6999:d 6995:) 6992:x 6989:( 6986:f 6981:b 6976:a 6958:b 6944:a 6934:= 6931:x 6928:d 6924:) 6921:x 6918:( 6915:f 6860:. 6856:) 6850:n 6846:f 6839:, 6832:, 6827:1 6823:f 6815:( 6811:= 6807:f 6793:) 6790:n 6786:f 6782:1 6779:f 6775:f 6770:n 6754:n 6749:R 6713:. 6710:x 6707:d 6701:n 6697:f 6691:b 6686:a 6670:n 6662:= 6658:x 6655:d 6648:n 6644:f 6631:n 6620:b 6615:a 6607:= 6604:x 6601:d 6597:f 6592:b 6587:a 6572:f 6566:n 6562:f 6557:f 6547:n 6543:f 6477:ε 6467:ε 6463:m 6459:M 6457:( 6453:2 6447:ε 6441:m 6437:M 6432:f 6427:2 6424:ε 6417:i 6414:) 6412:ε 6410:( 6408:I 6406:{ 6397:ε 6393:a 6389:b 6387:( 6383:1 6377:ε 6371:a 6367:b 6361:1 6358:ε 6353:f 6347:i 6344:) 6342:1 6339:ε 6337:( 6335:J 6322:s 6317:i 6314:) 6312:1 6309:ε 6307:( 6305:J 6300:) 6297:i 6294:) 6292:1 6289:ε 6287:( 6285:J 6278:i 6275:) 6273:1 6270:ε 6268:( 6266:J 6256:1 6253:ε 6246:1 6243:ε 6239:X 6232:i 6229:) 6227:1 6224:ε 6222:( 6220:J 6218:{ 6210:2 6207:ε 6200:i 6197:) 6195:1 6192:ε 6190:( 6188:I 6186:{ 6182:f 6174:M 6170:m 6166:) 6164:m 6160:M 6156:ε 6152:2 6149:ε 6144:) 6142:a 6138:b 6134:ε 6130:1 6127:ε 6115:ε 6105:ε 6095:k 6091:k 6087:i 6080:k 6076:i 6068:i 6065:) 6063:ε 6061:( 6059:J 6057:{ 6046:k 6041:k 6037:i 6029:i 6026:) 6024:ε 6022:( 6020:I 6018:{ 6012:ε 6008:X 5995:ε 5991:X 5982:ε 5978:X 5963:ε 5959:X 5954:ε 5946:f 5940:. 5936:ε 5932:X 5927:ε 5923:f 5917:ε 5913:X 5906:ε 5902:X 5895:ε 5891:X 5882:b 5878:a 5868:ε 5864:X 5859:ε 5853:ε 5849:X 5841:f 5836:n 5834:/ 5832:c 5827:f 5822:n 5816:f 5807:n 5801:f 5797:c 5791:n 5786:X 5779:n 5774:X 5760:n 5755:X 5750:c 5744:n 5739:X 5727:c 5721:n 5716:X 5711:n 5696:n 5690:n 5685:X 5683:{ 5679:f 5675:f 5671:ε 5665:ε 5661:X 5656:ε 5552:. 5549:x 5546:d 5542:) 5539:x 5536:( 5533:g 5528:b 5523:a 5512:+ 5509:x 5506:d 5502:) 5499:x 5496:( 5493:f 5488:b 5483:a 5472:= 5469:x 5466:d 5462:) 5459:) 5456:x 5453:( 5450:g 5444:+ 5441:) 5438:x 5435:( 5432:f 5426:( 5421:b 5416:a 5401:β 5397:α 5393:g 5389:f 5362:. 5356:Q 5351:I 5324:. 5321:x 5318:d 5314:) 5311:x 5308:( 5302:Q 5297:I 5291:1 5286:0 5278:= 5275:x 5272:d 5268:) 5265:x 5262:( 5256:Q 5251:I 5245:1 5240:1 5232:2 5222:+ 5219:x 5216:d 5212:) 5209:x 5206:( 5200:Q 5195:I 5189:1 5181:2 5174:0 5145:Q 5140:I 5110:. 5106:] 5102:1 5099:, 5094:n 5090:1 5084:n 5077:[ 5073:, 5067:, 5063:] 5057:n 5054:2 5049:, 5044:n 5041:1 5035:[ 5031:, 5027:] 5021:n 5018:1 5013:, 5010:0 5006:[ 4995:n 4983:i 4981:t 4977:i 4970:i 4966:x 4961:i 4959:t 4954:i 4948:i 4946:x 4941:i 4939:t 4915:g 4905:C 4903:I 4898:g 4894:ε 4890:ε 4886:g 4880:C 4878:I 4873:g 4867:C 4865:I 4860:g 4854:C 4852:I 4843:C 4837:C 4835:I 4826:C 4808:Q 4803:I 4781:s 4774:ε 4769:ε 4747:. 4743:] 4737:2 4729:+ 4724:i 4720:t 4716:, 4711:j 4707:x 4702:[ 4691:, 4687:] 4681:j 4677:x 4673:, 4668:2 4655:i 4651:t 4646:[ 4634:i 4632:t 4627:j 4625:x 4620:i 4618:t 4602:. 4598:] 4592:2 4584:+ 4579:i 4575:t 4571:, 4566:2 4553:i 4549:t 4544:[ 4532:i 4530:t 4524:δ 4519:i 4517:t 4510:δ 4505:i 4503:t 4497:i 4495:t 4488:δ 4483:j 4481:x 4476:i 4474:t 4470:δ 4465:j 4463:x 4458:i 4456:t 4451:j 4449:x 4445:δ 4440:i 4438:t 4434:δ 4430:δ 4425:i 4423:t 4418:n 4416:/ 4414:ε 4409:δ 4405:ε 4400:n 4398:/ 4396:ε 4389:n 4387:/ 4385:ε 4378:i 4376:t 4371:n 4369:/ 4367:ε 4362:ε 4357:i 4355:t 4351:n 4344:ε 4339:i 4337:t 4332:i 4330:t 4326:f 4321:i 4319:t 4313:ε 4305:i 4301:x 4295:i 4293:x 4288:i 4286:t 4279:n 4275:t 4271:0 4268:t 4261:n 4259:x 4255:0 4252:x 4230:Q 4225:I 4199:R 4192:] 4189:1 4186:, 4183:0 4180:[ 4177:: 4171:Q 4166:I 4152:f 4148:f 4133:R 4126:] 4123:1 4120:, 4117:0 4114:[ 4111:: 4108:f 4090:ε 4086:s 4080:ε 4073:m 4056:. 4050:) 4047:1 4041:m 4038:( 4035:2 4022:) 4016:1 4013:+ 4010:j 4006:y 3997:1 3994:+ 3991:i 3987:x 3982:( 3977:| 3972:) 3962:i 3958:t 3954:( 3950:f 3943:) 3938:i 3934:t 3930:( 3926:f 3922:| 3906:i 3899:t 3882:, 3876:) 3873:1 3867:m 3864:( 3861:r 3858:2 3838:1 3835:+ 3832:j 3828:y 3819:1 3816:+ 3813:i 3809:x 3797:j 3795:y 3781:, 3776:j 3772:M 3765:) 3760:i 3756:t 3752:( 3749:f 3741:j 3737:m 3723:i 3721:t 3700:. 3696:) 3690:i 3686:x 3677:1 3674:+ 3671:j 3667:y 3662:( 3657:) 3652:i 3648:t 3644:( 3640:f 3637:+ 3633:) 3627:1 3624:+ 3621:j 3617:y 3608:1 3605:+ 3602:i 3598:x 3593:( 3588:) 3583:i 3579:t 3575:( 3571:f 3568:= 3564:) 3558:i 3554:x 3545:1 3542:+ 3539:i 3535:x 3530:( 3525:) 3520:i 3516:t 3512:( 3508:f 3498:) 3495:i 3493:x 3487:i 3483:x 3478:i 3476:t 3474:( 3472:f 3464:j 3460:y 3453:n 3451:x 3447:0 3444:x 3434:m 3429:δ 3413:. 3408:2 3405:+ 3402:j 3398:y 3389:1 3386:+ 3383:i 3379:x 3370:1 3367:+ 3364:j 3360:y 3351:i 3347:x 3338:j 3334:y 3323:δ 3317:m 3315:y 3311:0 3308:y 3301:m 3291:m 3286:s 3281:f 3276:j 3274:M 3269:j 3267:m 3251:j 3247:M 3240:) 3235:i 3231:t 3227:( 3224:f 3216:j 3212:m 3198:s 3194:ε 3190:δ 3183:n 3179:t 3175:0 3172:t 3165:n 3163:x 3159:0 3156:x 3151:δ 3145:m 3127:} 3122:) 3116:1 3110:m 3106:y 3097:m 3093:y 3088:( 3084:, 3078:, 3074:) 3068:1 3064:y 3055:2 3051:y 3046:( 3042:, 3038:) 3032:0 3028:y 3019:1 3015:y 3010:( 3006:, 3000:) 2997:1 2991:m 2988:( 2985:r 2982:2 2972:{ 2952:δ 2946:m 2939:r 2934:f 2928:r 2911:. 2907:| 2903:) 2900:x 2897:( 2894:f 2890:| 2884:] 2881:b 2878:, 2875:a 2872:[ 2866:x 2858:2 2855:= 2852:r 2842:s 2836:ε 2829:m 2827:y 2823:0 2820:y 2815:ε 2800:s 2796:ε 2792:δ 2788:s 2784:ε 2780:δ 2776:δ 2772:ε 2768:s 2764:s 2753:s 2749:s 2745:f 2728:. 2718:| 2714:s 2707:) 2703:) 2698:i 2694:x 2685:1 2682:+ 2679:i 2675:x 2671:( 2668:) 2663:i 2659:t 2655:( 2652:f 2647:1 2641:n 2636:0 2633:= 2630:i 2621:( 2616:| 2602:m 2598:r 2594:0 2591:r 2584:m 2582:y 2578:0 2575:y 2567:n 2563:t 2559:0 2556:t 2549:n 2547:x 2543:0 2540:x 2532:m 2528:r 2524:0 2521:r 2514:m 2512:y 2508:0 2505:y 2498:ε 2489:s 2485:f 2467:. 2457:| 2453:s 2446:) 2442:) 2437:i 2433:x 2424:1 2421:+ 2418:i 2414:x 2410:( 2407:) 2402:i 2398:t 2394:( 2391:f 2386:1 2380:n 2375:0 2372:= 2369:i 2360:( 2355:| 2344:δ 2337:n 2333:t 2329:0 2326:t 2319:n 2317:x 2313:0 2310:x 2300:δ 2293:ε 2284:s 2280:f 2248:f 2244:f 2240:f 2220:. 2217:) 2212:i 2208:x 2199:1 2196:+ 2193:i 2189:x 2185:( 2182:) 2179:t 2176:( 2173:f 2168:] 2163:1 2160:+ 2157:i 2153:x 2149:, 2144:i 2140:x 2136:[ 2130:t 2120:1 2114:n 2109:0 2106:= 2103:i 2095:= 2088:) 2085:P 2082:, 2079:f 2076:( 2073:U 2066:, 2063:) 2058:i 2054:x 2045:1 2042:+ 2039:i 2035:x 2031:( 2028:) 2025:t 2022:( 2019:f 2014:] 2009:1 2006:+ 2003:i 1999:x 1995:, 1990:i 1986:x 1982:[ 1976:t 1966:1 1960:n 1955:0 1952:= 1949:i 1941:= 1934:) 1931:P 1928:, 1925:f 1922:( 1919:L 1905:f 1888:i 1886:x 1880:i 1876:x 1871:) 1868:i 1866:t 1864:( 1862:f 1845:. 1841:) 1835:i 1831:x 1822:1 1819:+ 1816:i 1812:x 1807:( 1803:) 1798:i 1794:t 1790:( 1787:f 1782:1 1776:n 1771:0 1768:= 1765:i 1747:n 1743:t 1739:0 1736:t 1729:n 1727:x 1723:0 1720:x 1715:f 1705:f 1684:j 1679:j 1673:j 1671:s 1666:i 1664:t 1658:) 1656:i 1654:( 1652:r 1648:y 1643:i 1641:x 1636:) 1634:i 1632:( 1630:r 1623:i 1618:i 1614:) 1612:t 1608:x 1606:( 1604:P 1595:) 1593:s 1589:y 1587:( 1585:Q 1580:) 1578:s 1574:y 1572:( 1570:Q 1565:) 1563:t 1559:x 1557:( 1555:P 1547:i 1540:i 1538:t 1530:n 1526:t 1522:0 1519:t 1514:) 1512:t 1508:x 1506:( 1504:P 1484:. 1481:] 1478:1 1472:n 1469:, 1466:0 1463:[ 1457:i 1453:, 1449:) 1443:i 1439:x 1430:1 1427:+ 1424:i 1420:x 1415:( 1377:b 1374:= 1369:n 1365:x 1350:i 1346:x 1331:2 1327:x 1318:1 1314:x 1305:0 1301:x 1297:= 1294:a 1267:x 1263:x 1259:x 1255:f 1247:) 1245:x 1243:( 1241:f 1233:S 1229:S 1213:. 1210:x 1207:d 1203:) 1200:x 1197:( 1194:f 1189:b 1184:a 1169:S 1153:. 1149:} 1145:) 1142:x 1139:( 1136:f 1130:y 1124:0 1120:, 1116:b 1110:x 1104:a 1100:: 1096:) 1093:y 1090:, 1087:x 1084:( 1080:{ 1076:= 1073:S 1059:f 1055:S 1047:f 982:e 975:t 968:v 546:) 491:) 487:( 476:) 412:) 220:) 132:) 129:a 126:( 123:f 117:) 114:b 111:( 108:f 105:= 102:t 99:d 95:) 92:t 89:( 82:f 76:b 71:a

Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.

Index